Class 12 RD Sharma Solutions – Chapter 22 Differential Equations – Exercise 22.11 | Set 1
Last Updated :
28 Mar, 2021
Question 1. The surface area of a balloon being inflated changes at a rate proportional to time t. If initially its radius is 1 unit and after 3 seconds it is 2 units, find the radius after time t.
Solution:
Let us considered radius = r
and the surface area of the balloon at a particular time ‘t’ = S
Surface area is given by,
S = 4πr2 …..(1)
We have,
(dS/dt)∝ t
(dS/dt) = kt (where k is proportional constant)
On differentiating eq(1), we get
d/dt(4πr2) = kt
8πr(dr/dt) = kt
ktdt = 8πrdr
On integrating both sides, we get
∫ktdt = ∫8πrdr
kt2/2 = 8π(r2/2) + c …..(2)
At t = 0, r = 1 unit and at t = 3sec, r = 2units
0 = 4π + c
c = -4π
And,
k(3)2/2 = 8π(22/2) – 4π
(9/2)k = 12π
k = (8π/3)
On putting the values of k in equation (2)
(8π/3)(t2/2) = 8π(r2/2) – 4π
4t2/3 = 4r2-4
r2 = 1 + (t2/3)
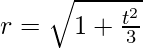
Hence, the radius after time t = 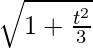
Question 2. A population grows at the rate of 5% per year. How long does it take for the population to double?
Solution:
Let us considered the initial population = P0
and the population at a particular time ‘t’ = P’
We have,
dP/dt = 5%P
dP/dt = 5P/100
dP/P = 0.05dt
On integrating both sides, we get
∫(dP/P) = ∫0.05dt
Log|P| = 0.05t + c
At t = 0, P = P0
log|P0| = c
Log|P| = 0.05t + log|P0|
Log|P/P0| = 0.05t
Now we find the time population becomes double,
P = 2P0
Log|2P0/P0| = 0.05t
Log|2| = 0.05t
t = 20Log|2| years
Question 3. The rate of growth of a population is proportional to the number present population of a city doubled in the past 25 years, and the present population 100000, when will the city have a population of 500000?
Solution:
Let us considered
The initial population = P0
the population at a particular time ‘t’ = P
and the growth of population = g’
We have,
dP/dt = gP
dP/P = gdt
On integrating both sides, we get
∫(dP/P) = g∫dt
Log|P| = gt + c
At t = 0, P = P0
log|P0| = c
Log|P| = gt + log|P0|
Log|P/P0| = gt
Population of city is doubled in 25 years.
At t = 25, P = 2P0
Log|2P0/P0| = 25g
g = Log|2|/25
g = 0.0277
Log|P/P0| = 0.0277t
For P = 500000 and P0 = 100000
Log|500000/100000| = 0.0277t
t = Log|5|/0.0277
t = 58.08 year
t = 58 year
Question 4. In a culture, the bacteria count is 100000. The number is increased by 10% in 2 hours. In how many hours will the count reach 200000, if the rate of growth of bacteria is proportional to the number of present?
Solution:
Let us assume the number of bacteria count at a particular time ‘t’ = P
We have,
dP/dt ∝ P
(dP/dt) = kP (where k is proportional constant)
(dP/P) = kdt
On integrating both sides, we get
∫(dP/P) = ∫kdt
Log|P| = kt + c
At t = 0, P = 100000
Log|100000| = c
Log|P| = kt + Log|100000|
After t = 2 hours number is increases by 10%.
Therefore, P = 100000 + (100000)(5/100)
P = 110000
Log|110000| = 2k + Log|100000|
k = (1/2)Log|11/10|
Log|P| = (t/2)Log|11/10| + Log|100000| …(i)
Putting the value of k in equation (i)
Let at t = T P = 200000
Log|200000| = (T/2)Log|11/10| + Log|100000|
Log|2| = (T/2)Log|11/10|
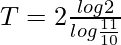
Question 5. If the interest is compounded continuously at 6% per annum, how much worth RS 1000 will be after 10 years? How long will it take to double RS. 1000?
Solution:
Let us assume be the initial amount = P0
and the amount at a particular time ‘t’ = P
dP/dt = 6%P
dP/dt = 6P/100
dP/P = 0.06dt
On integrating both sides, we get
∫(dP/P) = ∫0.06dt
Log|P| = 0.06t + c
At t = 0, P = P0
log|P0| = c
Log|P| = 0.06t + log|P0|
Log|P/P0| = 0.06t
At t = 10 years find the amount
Log|P/P0| = 0.06 × 10
Log|P/P0| = 0.6
P/P0 = e0.6
P = P0 × 1.8221
P = 1000 × 1.8221
P = 1822
At what time amount becomes double,
P = 2P0
Log|2P0/P0| = 0.06t
Log|2| = 0.06t
t = 16.66Loge|2|
t = 11.55 years
Question 6. The rate of increase in the number of bacteria in a certain bacteria culture proportional to the number present, Given the number triples in 5 hrs, find how many bacteria will be present after 10 hours. Also, find the time necessary for the number of bacteria to be 10 times the number of initial present.
Solution:
Let us considered
The initial count of bacteria = P0
the count of bacteria at a particular time ‘t’ = P
and the growth of bacteria = g times.
We have,
dP/dt ∝ P
dP/dt = gP
dP/P = gdt
On integrating both sides, we get
∫(dP/P) = g∫dt
Log|P| = gt + c
At t = 0, P = P0
log|P0| = c
Log|P| = gt + log|P0|
Log|P/P0| = gt
At t = 5 hours, P = 3P0
Log|3P0/P0| = 5g
g = Loge|3|/5
g = 0.219722
Log|P/P0| = 0.219722t
At t = 10 hours find the numbers of bacteria.
Log|P/P0| = 0.219722 × 10
|P/P0| = e2.19722
|P/P0| = 9
P = 9P0
At ‘T’ time, a number of bacteria become 10 times.
At t = T, P = 10P0
Log|10P0/P0| = Loge|3|/5T
Log|10| = T(Loge|3|/5)

Question 7. The population of a city increases at a rate proportional to the number of inhabitants present at any time t. If the population of the city was 200000 in 1990 and 250000 in 2000. What will be the population in 2010?
Solution:
Let us considered
The initial population = P0
the population at a particular time ‘t’ = P
and the growth of population = g times.
We have,
dP/dt ∝ P
dP/dt = gP
dP/P = gdt
On integrating both sides, we get
∫(dP/P) = g∫dt
Log|P| = gt + c …(i)
At t = 1990, P = 200000 and at t = 2000, P = 250000
Log|200000| = 1990g + c …(ii)
Log|250000| = 2000g + c …(iii)
On subtracting eq (iii) from (ii)
10g = Log|250000/200000|
g = (1/10)Log|5/4|
On putting the value of ‘g’ in equation (i)
Log|200000| = 1990 × (1/10)Log|5/4| + c
c = Log|200000| – 199 × Log|5/4|
Population in 2010,
Log|P| = (1/10)Log|5/4| × 2010 + Log|200000| – 199 × Log|5/4|
Log|P| = 201Log|5/4| – 199Log|5/4| + Log|200000|
Log|P| = Log|5/4|201 – Log|5/4|199 + Log|200000|
Log|P| = Log|(5/4)201(4/5)199| + log|200000|
Log|P| = Log|5/4|2 + log|200000|
Log|P| = Log|(25/16)200000|
Log|P| = Log|312500|
P = 312500
Question 8. If the marginal cost of manufacturing a certain item is given by C'(x) = (dC/dx) = 2 + 0.15x. Find the total cost function C(x), given that C(0) = 100
Solution:
We have,
dC/dx = 2 + 0.15x
dC = (2 + 0.15x)dx
On integrating both sides, we get
∫dC = ∫(2 + 0.15x)dx
C(x) = 2x + (0.15/2)x2 + c1
At C(0) = 100, we have
100 = 2(0) + (0.15/2)(0)2 + c1
c1 = 100
C(x) = 0.075x2 + 2x + 100
Question 9. A bank pays interest by continuous compounding, that is, by treating the interest rate as the instantaneous rate of change of principal. Suppose in an account interest accrues at 8% per year, compounded continuously. Calculate the percentage increase in such an account over one year.
Solution:
Let us considered
The initial population = P0
and the population at a particular time ‘t’ = P
We have,
dP/dt = (8/100)P
dP/dt = (2/25)P
dP/P = (2/25)dt
On integrating both sides, we get
∫(dP/P) = (2/25)∫dt
Log|P| = (2/25)t + c …(i)
At t = 0, P = P0
Log|P0| = 0 + c
c = Log|P0|
On putting the value of c in equation (i)
Log|P| = (2/25)t + Log|P0|
Log|P/P0| = (2t/25)
Amount after 1 year,
Log|P/P0| = (2/25)
e(2/25) = |P/P0|
e0.08 = |P/P0|
1.0833 = |P/P0|
P = 1.0833P0
Percentage increase = [(P – P0)/P0] × 100%
= [(1.0833P0 – P0)/P0] × 100%
= 0.0833 × 100%
= 8.33%
Question 10. In a simple circuit of resistance R, self-inductance L and voltage E, the current i at any time is given by L(di/dt) + Ri = E. If E is constant and initially no current passes through the circuit, prove that i = (E/R){1 – e-(R/L)t}
Solution:
We have,
L(di/dt) + Ri = E
(di/dt) + (R/L)i = E/L
The given equation is a linear differential equation of the form
(dy/dx) + Py = Q
Where, P = (R/L), Q = E/L
So, I.F = e∫Pdi
= e∫(R/L)di
= e(R/L)t
The solution of a differential equation is,
i(I.F) = ∫Q(I.F)dt + c
e(R/L)t × i = (E/L)∫e(R/L)tdt + c
e(R/L)t × i = (E/L)(L/R)e(R/L)t + c
e(R/L)t × i = (E/R)e(R/L)t + c …(i)
At t = 0, i = 0
e0 × 0 = (E/R)e0 + c
c = -(E/R)
On putting the value of c in equation (i)
e(R/L)t × i = (E/R)e(R/L)t – (E/R)
i = (E/R) – (E/R)e-(R/L)t
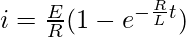
Question 11. The decay rate of the radius at any time t is proportional to its mass at that time. Find the time when the mass will be halved of its initial mass.
Solution:
Let us considered
initial radius = R0
and radius at a particular time ‘t’ = R
We have,
dR/dt ∝ R
dR/dt = -kR
dR/R = -kdt
On integrating both sides, we get
∫(dR/R) = -k∫dt
Log|R| = -kt + c …(i)
At t = 0, R = R0
Log|R0| = 0 + c
c = Log|R0|
Log|R| = -kt + Log|R0|
kt = Log|R0/R|
At time ‘T’ mass becomes R0/2
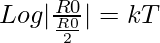
Log|2| = kT
T = (1/k)Log|2|
Share your thoughts in the comments
Please Login to comment...