Class 12 RD Sharma Solutions – Chapter 33 Binomial Distribution – Exercise 33.1 | Set 3

Question 37. An experiment succeeds twice as often as it fails. Find the probability that in the next 6 trials there will be at least 4 successes.

Solution:

Let us consider X be the number of successes out of 6 experiment. Also, p denotes the probability of success and q denotes the probability of failure.

According to the question, successes are twice failures.

So,  p = 2q

Also, p + q = 1

=> 3q = 1

=> q = 1/3

And p = 1 – 1/3 = 2/3

So, the binomial distribution(n) = 6

Hence, the probability of getting r success is,

P(X = r) = , r = 0, 1, 2 . . . . . 6

P(at least 4 successes) = P(X > 4)

= P(X = 4) + P(X = 5) + P(X = 6)

= 

= 

= 

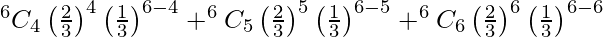
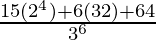
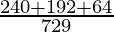
= 496/729

Question 38. In a hospital, there are 20 kidney dialysis machines and the chance of any one of them to be out of service during a day is 0.02. Determine the probability that exactly 3 machines will be out of service on the same day.

Solution:

Let us considered X be the number of machines out of service during a day. Also, p be the probability of any machine out of service during a day and q be the probability that machine will be in service on the same day.

Then, X has a binomial distribution with n = 20.

Hence, p = 0.02 and q = 0.98

Therefore, 

P(X = r) = 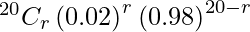, r = 0, 1, 2 . . . . . 20

The probability of exactly 3 machines will be out of the service on the same day is

P(X = 3) =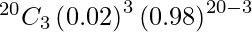 

= 1140 (0.000008) (0.7093)

= 0.006469

Question 39. The probability that a student entering a university will graduate is 0.4. 

(i) Find the probability that out of 3 students of the university none will graduate.

Solution:

Let us consider X denotes the number of students that graduate from among 3 students. Also, p denotes the probability that a student entering a university will graduate.

Here, n = 3, p = 0.4 and q = 0.6

Hence, 

P(X = r) = 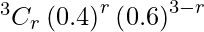 , r = 0, 1, 2, 3

So, the probability that none of the student will graduate is 

P(X = 0) = q³ 

= (0.6)³

= 0.216

(ii) Find the probability that out of 3 students of the university only one will graduate.

Solution:

Let us consider X denotes the number of students that graduate from among 3 students. Also, p denotes the probability that a student entering a university will graduate.

Here , n = 3, p = 0.4 and q = 0.6.

Hence, 

P(X = r) = 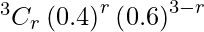 , r = 0, 1, 2, 3

So, the probability that only one student will graduate is 

P(X = 1) = 3 (0.4) (0.36) 

= 0.432

(iii) Find the probability that out of 3 students of the university all will graduate.

Solution:

Let us consider X denotes the number of students that graduate from among 3 students. Also, p be the probability that a student entering a university will graduate.

Here, n =3, p = 0.4 and q = 0.6

Hence, 

P(X = r) = 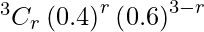, r = 0, 1, 2, 3

So, the probability that all the students will graduate is 

P(X = 3) = p³ 

= (0.4)³

= 0.064

Question 40. Ten eggs are drawn successively, with replacement, from a lot containing 10% defective eggs. Find the probability that there is at least one defective egg.

Solution:

Let us consider X denotes the number of defective eggs drawn from 10 eggs. Also, p denotes the probability that a drawn egg is defective.

Then, X has a binomial distribution with n = 10. 

=> p = 10% = 10/100 = 1/10

And q = 1 – p = 9/10

Hence, 

P(X = r) = 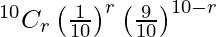 , r = 0, 1, 2 . . . . 10

So, the probability of t least one defective egg is 

= P(X > 1) = 1 – P(X = 0)

= 

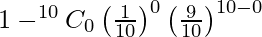
= 1 – (9/10)¹⁰

Question 41. In a 20-question true-false examination, suppose a student tosses a fair coin to determine his answer to each question. For every head he answers ‘true’ and for every tail, he answers ‘false’. Find the probability that he answers at least 12 questions correctly.

Solution:

Let us consider X be the number of correct answers. Also, p denotes the probability of a correct answer and p be the probability of a correct answer.

So, 

=> p = 1/2

So q = 1 – 1/2 = 1/2

Then, X has a binomial distribution with n = 20.

Hence, 

P(X = r) = 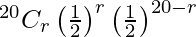 , r = 0, 1, 2, 3 . . . . . . 20

= 

So, the probability that the student answers at least 12 questions correctly is

P(X > 12) = P(X = 12) + P(X = 13) + . . . + P(X = 20)

= 

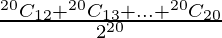
Question 42. Suppose X has a binomial distribution with n = 6 and p = 1/2. Show that X = 3 is the most likely outcome. 

Solution:

According to the question, x has a binomial distribution with n = 6 and p = 1/2.

So, q = 1 – p = 1 – 1/2 = 1/2

Hence, 

P(X = r) = 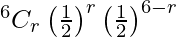 , r = 0, 1, 2, 3, 4, 5, 6

= ⁶C_r (1/2)⁶

=> P(X = r) = ⁶C_r (1/2)⁶

Now, by substituting r = 0, 1, 2, 3, 4, 5 and 6, we get 

P(X = 0) = ⁶C₀ (1/2)⁶

= 1/64

P(X = r) = ⁶C₁ (1/2)⁶ 

= 6/64

P(X = r) = ⁶C₂ (1/2)⁶

= 15/64

P(X = r) = ⁶C₃ (1/2)⁶

= 20/64

P(X = r) = ⁶C₄ (1/2)⁶

= 15/64

P(X = r) = ⁶C₅ (1/2)⁶

= 6/64

P(X = r) = ⁶C₆ (1/2)⁶

= 1/64

So, the tabular form is:

X	0	1	2	3	4	5	6
P(X)	1/64	6/64	15/64	20/64	15/64	6/64	1/64

Now, on comparing the probabilities, we get that X = 3 is the most likely outcome as P(X = 3) has the greatest value.

Hence proved.

Question 43. In a multiple-choice examination with three possible answers for each of the five questions out of which only one is correct, what is the probability that a candidate would get four or more correct answers just by guessing?

Solution:

Let us consider X denotes the number of right answers in the 5 questions. Also, p denotes the probability of guessing right answer and q denotes the probability of guessing wrong answer.

Here X can take values 0, 1, 2, 3, 4 and 5. So, X has a binomial distribution with n = 5.

Here p = 1/3

And q = 2/3

Hence, 

P(X = r) = 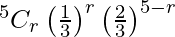 , r = 0, 1, 2, . . . 5

So, the probability that the student guesses 4 or more correct answers

P(X > 4) = P(X = 4) + P(X = 5)

= 

= 

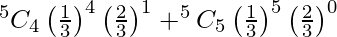
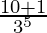
= 11/243

Question 44. A person buys a lottery ticket in 50 lotteries, in each of which his chance of winning a prize is 1/100.

(i) What is the probability that he will win a prize at least once.

Solution:

Let us consider X be the number of times the person wins the lottery. Also, p denotes the probability of winning a prize.

Then, X has a binomial distribution with n = 50.

=> p = 1/100

And q = 1 – 1/100 = 99/100

Hence, 

P(X = r) = 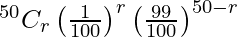 , r = 0, 1, 2 . . . 50

So, the probability of winning at least once is 

P(X > 0) = 1 – P(X – 0)

= 1 – (99/100)⁵⁰

(ii) What is the probability that he will win a prize exactly once.

Solution:

Let us consider X be the number of times the person wins the lottery. Also, p denotes the probability of winning a prize.

Then, X has a binomial distribution with n = 50.

=> p = 1/100

And q = 1 – 1/100 = 99/100

Hence, 

P(X = r) = 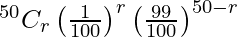, r = 0, 1, 2 . . . 50

So the probability of winning at least twice is 

P(X > 2) = 1 – P(X = 0) – P(X = 1)

= 

= 

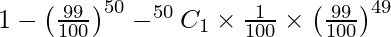
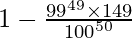
(iii) What is the probability that he will win a prize at least twice.

Solution:

Let us consider X be the number of times the person wins the lottery. Also, p be the probability of winning a prize.

Then, X has a binomial distribution with n = 50.

=> p = 1/100

And q = 1 – 1/100 = 99/100

Hence, 

P(X = r) = 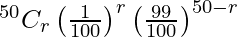, r = 0, 1, 2 . . . 50

So, the probability of winning at least twice is 

P(X > 2) = 1 – P(X = 0) – P(X = 1)

= 

= 

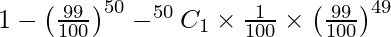
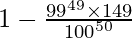
Question 45. The probability of a shooter hitting a target is 3/4. How many minimum number of times must he/she fire so that the probability of hitting the target at least once is more than 0.99?

Solution:

Let us consider the shooter fire n times and X be the number of times the shooter hits the target.

Then, X has a binomial distribution with p = 3/4 and q = 1/4 such that,

Hence, 

P(X = r) =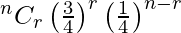 

P(X = r) = 

Given that P (X > 1) > 0.99

=> 1 – P(X = 0) > 0.99

=> 1 – 1/4ⁿ > 0.99

=> 1/4ⁿ < 0.01

=> 4ⁿ > 1/0.01

=> 4ⁿ > 100

So, the shooter must fire at least 4 times.

Question 46. How many times must a man toss a fair coin so that the probability of having at least one head is more than 90%?

Solution:

Let us considered the man tosses a fair coin n times and X be the number of heads in n tosses.

As p = 1/2 and q = 1/2, 

So, 

P(X = r) = 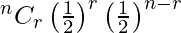 , r = 0, 1, 2, 3 . . . . n

It is given that P(X > 1) > 0.9

=> 1 – P(X = 0) > 0.9

=> 

=>(1/2ⁿ) < 1/10

=> 2^n > 10

=> n = 4, 5, 6 . . . . 

So, the man must toss the coin at least 4 times.

Question 47. How many times must a man toss a fair coin so that the probability of having at least one head is more than 80%?

Solution:

Let us consider X denotes the number of heads and n be the minimum number of times that a man must toss a fair coin. 

So that probability of X ≥ 1 is more than 80 %.

Here X has a binomial distribution with p = 1/2 and q = 1/2.

P(X = r) = ⁿC_r (1/2)ⁿ

We have P(X > 1) = 1 – P(X = 0) 

= 

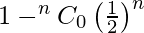
= 1 – (1/2)ⁿ

And for P(X > 1) > 80%

=> 1 – 1/2ⁿ > 0.80  

=> (1/2ⁿ) < 1 – 0.80 = 0.20

=> 2ⁿ > 1/0.2 = 5

We know, 2² < 5 while 2³ > 5.

So we get n = 3.

So, the man must toss the coin at least 3 times.

Question 48. A pair of dice is thrown 4 times. If getting a doublet is considered a success, find the probability distribution of the number of successes.

Solution:

Let us consider p be the probability of getting a doublet in a single throw of a pair of dice. Also, X be the number of getting doublets in 4 throws of a pair of dice. 

So, p = 6/36 = 1/6

And q = 1 – p = 1 – 1/6 = 5/6

Then, X has a binomial distribution with n = 4.

Hence, the probability of getting r doublets

P(X = r) = 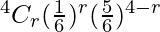, r = 0, 1, 2, 3, 4

If X = 0, then P(X = 0) =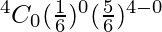 

=> P = (5/6)⁴

When X = 1, then P =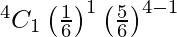 

= 

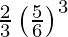
When X = 2, then P =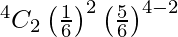 

= 

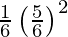
When X = 3, then P = 

= 

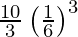
When X = 4, then P =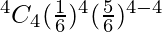 

= (1/6)⁴

= 1/1296

Question 49. From a lot of 30 bulbs that include 6 defective bulbs, a sample of 4 bulbs is drawn at random with replacement. Find the probability distribution of the number of defective bulbs.

Solution:

Let us considered X be the number of defective bulbs in a sample of 4 bulbs drawn successively with replacement.

Then, X has a binomial distribution with n = 4.

Here, p = 6/30 = 1/5

And q = 1 – 1/5 = 4/5

Then, 

P(X = r) =  , r = 0, 1, 2, 3, 4

P(X = 0) = (4/5)⁴

= 256/625

P(X = 1) =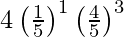 

= 256/625

P(X = 2) =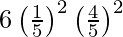 

= 96/625

P(X = 3) =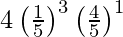 

= 16/625

P(X = 4) = (1/5)⁴

= 1/625

X	0	1	2	3	4
P(X)	256/625	256/625	96/625	16/625	1/625

Question 50. Find the probability that in 10 throws of a fair die, a score which is a multiple of 3 will be obtained in at least 8 of the throws. 

Solution:

Let us consider p be the probability of getting a success and q be the probability of getting a failure. Also, X be the number of success in a sample of 10 trials.

p = 2/6 = 1/3

So, q = 1 – p 

= 1 – 1/3

= 2/3

Then X has a binomial distribution with n = 10, p = 1/3 and q = 2/3.

P(X = r) =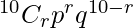 

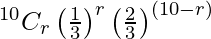= , r = 0, 1, 2, . . . , 10

So, the required probability is 

P(X > 8) = P(X = 8) + P(X = 9) + P(X = 10)

= 

= 

= 

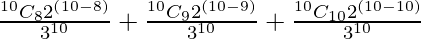
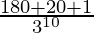
= 201/3¹⁰

Question 51. A die is thrown 5 times. Find the probability that an odd number will come up exactly three times. 

Solution:

Let us consider p be the probability of getting an odd number in a trial. Also, X be the number of success in a sample of 5 trials.

So, p = 3/6 = 1/2

Also, q = 1 – p = 1 – 1/2 = 1/2

Then, X has binomial distribution with n = 5 and p = q = 1/2.

P(X = r) = 

= 

= ⁵C₃ (1/2)⁵ , where r = 0, 1, 2, 3, 4, 5

So, the probability that an odd number will come up exactly three times. 

P(X = 3) = ⁵C₃ (1/2)⁵

= 10/32

= 5/16

Question 52. The probability of a man hitting a target is 0.25. He shoots 7 times. What is the probability of his hitting at least twice?

Solution:

Let us consider p be the probability of hitting the target. Also, X be the number of success in a sample of 7 trial.

So, p = = 0.25 = 1/4

Also q = 1 – p = 1 – 1/4 = 3/4

Then, X has a binomial distribution with parameters n = 7 and p = 1/4.

P(X = r) = 

= 

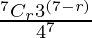= , where r  = 0, 1, 2, 3, 4, 5

So, the probability of his hitting at least twice is

P(X > 2) = 1 – [P(X = 0) + P(X = 1)]

= 

= 1 – [2187/16384 + 5103/16384]

= 1 – 7290/16384

= 9094/16384

= 4547/8192

Question 53. A factory produces bulbs. The probability that one bulb is defective is 1/50 and they are packed in boxes of 10. From a single box, find the probability: 

(i) that none of the bulbs is defective.

Solution:

Let us consider p be the probability of getting a defective bulb. Also, X be the number of success in a sample of 10 trials.

Here p = 1/50

Also, q = 1 – p = 1 – 1/50 = 49/50

Then, X has a binomial distribution with n = 10 and p = 1/50

P(X = r) =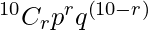 

= 

= , where r = 0, 1, 2, 3, . . . , 10

So, the probability that none of the bulb is defective

= P(X = 0) =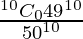 

= 49¹⁰/50¹⁰

(ii) that exactly two bulbs are defective.

Solution:

Let us consider p be the probability of getting a defective bulb. Also, X be the number of success in a sample of 10 trials.

Here p  = 1/50

Also, q = 1 – p = 1 – 1/50 = 49/50

Then, X has a binomial distribution with n = 10 and p = 1/50

P(X = r) =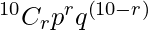 

= 

= , where r = 0, 1, 2, 3, . . . , 10

So, the probability that exactly two bulbs are defective

P(X = 2) = 

= 

(iii) that more than 8 bulbs work properly.       

Solution:

Let us consider p be the probability of getting a defective bulb. Also, X be the number of success in a sample of 10 trials.

Here p = 1/50

Also, q = 1 – p = 1 – 1/50 = 49/50

Then, X has a binomial distribution with n = 10 and p = 1/50

P(X = r) =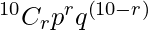 

= 

= , where r = 0, 1, 2, 3, . . . , 10

So, the probability that more than 8 bulbs work properly is

= P(X < 0)

= P(X = 0) + P(X = 1)

= 

= 

= 

= 

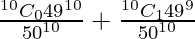
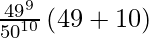
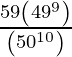
Question 54. A box has 20 pens of which 2 are defective. Calculate the probability that out of 5 pens drawn one by one with replacement, at most 2 are defective.

Solution:

Let us consider p be the probability of drawing a defective pen. Also, X be the number of defective pens drawn. 

Then,

=> p = 2/20 = 1/10

And q = 1 – p = 1 – 1/10 = 9/10

Then, X has a binomial distribution with n = 5

So, the probability of drawing r defective pens is

Now, P(X = r) = 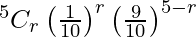, r = 0, 1, 2, 3, 4, 5

So, the probability of drawing at most 2 defective pens

= P(X  ≤ 2)

= P(X = 0) + P(X = 1) + P(X = 2)

= 

= 

= 

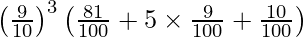
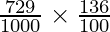
= 0.99144