Class 12 RD Sharma Solutions- Chapter 29 The Plane – Exercise 29.15 | Set 2
Last Updated :
18 Dec, 2021
Question 8. Find the image of the point (1, 3, 4) in the plane 2x – y + z + 3 = 0.
Solution:
According to the question we have to find the image of point P(1, 3, 4)
in the plane 2x – y + z +3 = 0
Now let us assume that Q be the image of the point.
Here, the direction ratios of normal to plane = 2, -1, 1
The direction ratios of PQ which is parallel to the normal to the plane
is proportional to 2, -1, 1 and the line PQ is passing through point P(1, 3, 4).
Thus, equation of the line PQ is:
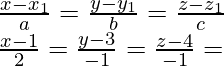
Now, the general point on the line PQ = (2λ + 1, -λ + 3, λ + 4)
Let Q = (2λ + 1, -λ + 3, λ + 4) -Equation(1)
Here, Q is the image of P, so R is the mid point of PQ
Coordinates of R = 
Point R is lies on the plane 2x – y + z + 3 = 0
= 2(λ + 1) – 
4λ + 4 + λ – 6 + λ + 8 + 6 = 0
6λ = -12
λ = -2
Now, put the value of λ in equation(1), we get
= (-4 + 1, 2 + 3, -2 + 4)
= (-3, 5, 2)
Hence, the image of point P(1, 3, 4) is (-3, 5, 2)
Question 9. Find the distance of the point with position vector 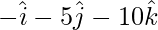 from the point of intersection of the line
from the point of intersection of the line 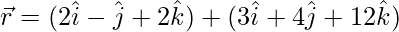 with the plane
with the plane 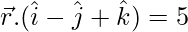 .
.
Solution:
According to the question we have to find distance of a point A with position
vector  from the point of intersection of
from the point of intersection of
line 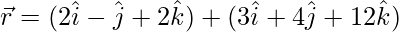
with plane 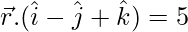
Let the point of intersection of line and plan be 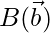
The line and the plane will intersect when,
=5\\ [(2+3λ)\hat{i}+(-1+4λ)\hat{j}+(2+12λ)\hat{k}](\hat{i}-\hat{j}+\hat{k})=5\\](https://www.geeksforgeeks.org/wp-content/ql-cache/quicklatex.com-6a0597c4ecdc7df7c6b78b933605d876_l3.png)
(2 + 3λ)(1) + (-1 + 4λ)(-1) + (2 + 12λ)(1) = 5
2 + 3λ + 1 – 4λ + 2 + 12λ = 5
11λ = 5 – 5
λ = 0
So, the point B is given by
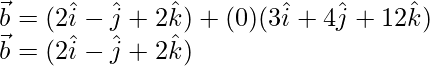

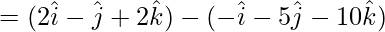
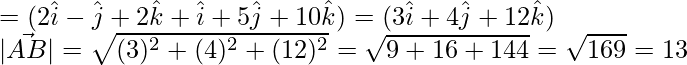
The required distance is 13 units.
Question 10. Find the length and the foot of the perpendicular from the point (1, 1, 2) to the plane 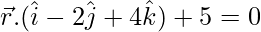 .
.
Solution:
Plane = x – 2y + 4z + 5 = 0 -Equation(1)
Point = (1, 1, 2)
D = 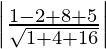
= 12/√21
The length of the perpendicular from the given point to the plane = 12/√21
Let us assume that the foot of perpendicular be (x, y, z).
So DR’s are in proportional
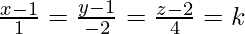
x = k + 1
y = -2k + 1
z = 4k + 2
Substitute (x, y, z) = (k + 1, -2k + 1, 4k + 2) in the plane equation(1)
k + 1 + 4k – 2 + 16k + 8 + 5 = 0
21k = -12
k = -12/21 = -4/7
Hence, the coordinate of the foot of the perpendicular = (3/7, 15/7, -2/7)
Question 11. Find the coordinates of the foot of the perpendicular and the perpendicular distance of the point P(3, 2, 1) from the plane 2x – y + z + 1 = 0. Find also the image of the point in the plane.
Solution:
Given:
Plane = 2x – y + z + 1 = 0 -Equation(1)
Point P = (3, 2, 1)
D =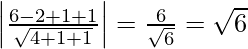
The perpendicular distance of the point P from the plane(D) = √6
Let us assume that the foot of perpendicular be (x, y, z).
So DR’s are in proportional
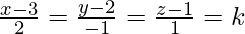
x = 2k + 3
y = -k + 2
z = k + 1
Substitute (x, y, z) = (2k + 3, -k + 2, k + 1) in the plane equation(1)
4k + 6 + k – 2 + k + 1 + 1 = 0
6k = -6
k = -6/6 = -1
The coordinate of the foot of the perpendicular = (1, 3, 0)
Question 12. Find the direction cosines of the unit vector perpendicular to the plane  passing through the origin.
passing through the origin.
Solution:
Given:
Equation of the plane 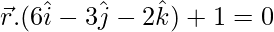
Thus, the direction ratios normal to the plane are 6, -3 and -2
Hence, the direction cosines to the normal to the plane are
= 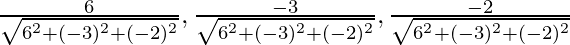
= 6/7, -3/7, -2/7
= -6/7, 3/7, 2/7
The direction cosines of the unit vector perpendicular to the plane
are same as the direction cosines of the unit vector perpendicular
to the plane are: -6/7, 3/7, 2/7
Question 13. Find the coordinates of the foot of the perpendicular drawn from the origin to the plane 2x – 3y + 4z – 6 = 0.
Solution:
According to the question,
Plane = 2x – 3y + 4z – 6 = 0
The direction ratios of the normal to the plane are 2, -3 and 4.
Thus, the direction ratios of the line perpendicular to the plane are 2, -3 and 4.
The equation of the line passing (x1, y1, z1) having direction ratios a, b and c is
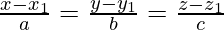
Thus, the equation of the line passing through the origin
with direction ratios 2, -3 and 4 is
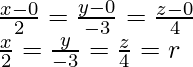
Here, r is same constant.
Any point on the line is of the form 2r, -3r, and 4r,
if the point P(2r, -3r, 4r) lies on the plane 2x – 3y + 4z – 6 = 0.
Thus, we have,
2(2r) – 3(-3r) + 4(4r) – 6 = 0
4r + 9r + 16r – 6 = 0
29r = 6
r = 6/29
Thus, the coordinates of the point of intersection of the perpendicular
from the origin and the plane are:
P(2×6/29, -3×629, 4×6/29) = P(12/29, -18/29, 24/29)
Question 14. Find the length and the foot of the perpendicular from the point (1, 3/2, 2) to the plane 2x – 2y + 4z +5 = 0.
Solution:
Given:
Point = (1, 3/2, 2)
Plane = 2x – 2y + 4z + 5 = 0
D = 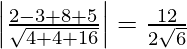
= √6
So, the length of the perpendicular from the point to the plane(D) = √6
Let the foot of perpendicular be (x, y, z). So, DR’s are in proportional
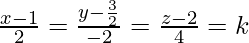
x = 2k + 1
y = -2k + 3/2
z = 4k + 2
So, using the values of x, y, z in equation of the plane we have,
2(2k + 1) – 2(-2k + 2/3) +4(4k + 2) + 5 = 0
4k + 2 + 4k – 3 + 16k + 8 + 5 = 0
24k = -12
k = -1/2
So, the coordinate of the foot of the perpendicular = (0, 5/2, 0)
Share your thoughts in the comments
Please Login to comment...