Question 14. Find the equation of the plane passing through the intersection of the planes x – 2y + z = 1 and 2x + y + z = 8 and parallel to the line with direction ratios proportional to 1, 2, 1. Also, find the perpendicular distance of (1, 1, 1) from this plane.
Solution:
As we know that the equation of the plane passing through the line of intersection of two planes are
(a1x + b1y + c1z + d1) + λ(a2x + b2y + c2z + d2) = 0
So, the equation of the plane passes through the intersection of the planes x – 2y + z = 1 and 2x + y + z = 8 is
(1 + 2λ)x + (-2 + λ)y + (1 + λ)z – 1 – 8λ = 0 ….(1)
Also, given that this plane is parallel to the line whose direction ratios are proportional to 1, 2, 1.
⇒ (1 + 2λ)1 + (-2 + λ)2 + (1 + λ)1 = 0
⇒ 1 + 2λ – 4 + 2λ + 1 + λ = 0
⇒ 5λ – 2 = 0
⇒ λ = 2/5
Now put the value of λ in (1), we have:
9x – 8y + 7z – 21 = 0 is the required equation.
And the perpendicular distance of plane from (1, 1, 1) is
= 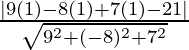
= 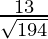 units.
units.
Question 15. Show when the line 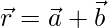 is parallel to the plane
is parallel to the plane 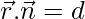 . Show that the line
. Show that the line 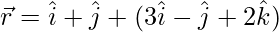 is parallel to the plane
is parallel to the plane 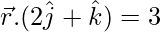 . Also, find the distance between the line and the plane.
. Also, find the distance between the line and the plane.
Solution:
The plane passes through the point with position vector 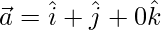 and is parallel to the vector
and is parallel to the vector 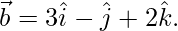
Given equation of the plane is 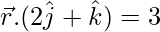 or
or 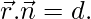
So, the normal vector is 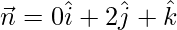 and d = 3.
and d = 3.
Now, 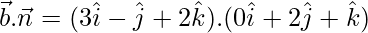
= 0 – 2 + 2 = 0
So, 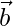 is perpendicular to
is perpendicular to 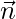
Hence, the given line is parallel to the given plane.
As we know that the distance between the line and parallel plane is the distance between any point on the line and the given plane.
So, Distance(d) = 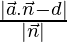
= 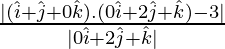
= 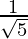 units.
units.
Question 16. Show that the line 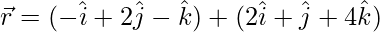 is parallel to the plane
is parallel to the plane 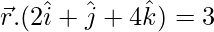 . Also, find the distance between the two.
. Also, find the distance between the two.
Solution:
The plane passes through the point with the position vector 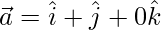 and is parallel to the vector
and is parallel to the vector 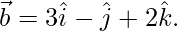 [Tex]\vec{b}[/Tex]
[Tex]\vec{b}[/Tex]
Given plane is 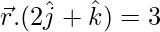 or
or 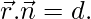
So, the normal vector is 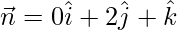 and d = 3.
and d = 3.
Now, 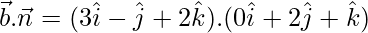
= 0 – 2 + 2 = 0
So, it is perpendicular to 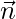 .
.
Hence, the given line is parallel to the plane.
As we know that the distance between a line and a parallel plane is the distance between any point on the line and the given plane.
so, Distance(d) = 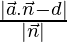
= 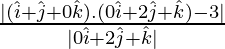
= 1/√6 units.
Question 17. Find the equation of the plane through the intersection of the planes 3x – 4y + 5z = 10 and 2x + 2y – 3z = 4 and parallel to the line x = 2x = 3z.
Solution:
As we know that the equation of the plane passing through the line of intersection of two planes are
(a1x + b1y + c1z + d1) + λ(a2x + b2y + c2z + d2) = 0
So, the equation of the plane passes through the intersection of the
planes 3x – 4y + 5z = 10 and 2x + 2y – 3z = 4 is
(3 + 2λ)x + (-4 + 2λ)y + (5 – 3λ)z – 10 – 4λ = 0 ….(1)
Given that the equation of line is x = 2x = 3z.
Now, dividing this equation by 6, we get,

So we get the direction ratios of this line are proportional to 6, 3, 2.
Now, the normal to the plane is perpendicular to the line whose direction ratios are 6, 3, 2.
⇒ (3 + 2λ)6 + (-4 + 2λ)3 + (5 – 3λ)2 – 10 – 4λ = 0
⇒ λ = -4/3
Now put the value of λ in eq(1), we get,
x – 20y + 27z = 14 is the required equation.
Question 18. Find the vector and cartesian forms of the equation of the plane passing through the point (1, 2, -4) and parallel to the lines 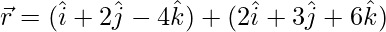 and
and 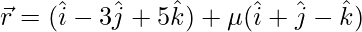 . Also, find the distance of the point from the plane obtained.
. Also, find the distance of the point from the plane obtained.
Solution:
Given that the equations of the lines are 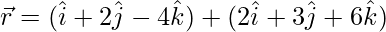
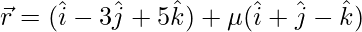
As we know that the vector equation of a plane passing through a point  and parallel to
and parallel to  and
and 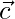 is
is
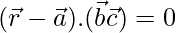
So, 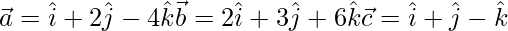
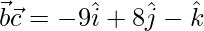
Now, the vector equation of the plane is 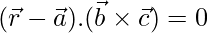
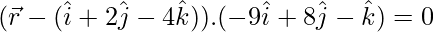
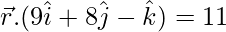
The cartesian equation of the plane is
-9x + 8y – z = 11
Now the distance of the point(9, -8, -10) from the plane is
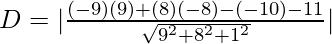
D = √146
Question 19. Find the equation of the plane passing through the points (3, 4, 1) and (0, 1, 0) and parallel to the line 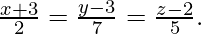
Solution:
When the plane passes through points (3, 4, 1), then the equation of the plane is
a(x – 3) + b(y – 4) + c(z – 1)=0 ….(1)
When this plane passes through points (0, 1, 0), then the equation of the plane is
a(0 – 3) + b(1 – 4) + c(0 – 1) = 0
⇒ 3a + 3b + 3c = 0 …..(2)
Also, given that the plane(i.e., eq(1)) is parallel to line.
So the normal of the plane(i.e., eq(1)) is perpendicular to the line so,
2a + 7b + 5c = 0 …..(3)
Now, on solving eq(1), (2), and (3), we get
8x – 13y + 15z + 13 = 0 is the required equation.
Question 20. Find the coordinates of the point where the line 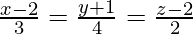 and intersects the plane x – y + z – 5 = 0. Also find the angle between the line and the plane.
and intersects the plane x – y + z – 5 = 0. Also find the angle between the line and the plane.
Solution:
Given that the equation of line is 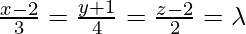
⇒ x = 3λ + 2, y = 4λ – 1, z = 2λ + 2 ….(1)
As we know that (x, y, z) intersect the plane x – y + z – 5 = 0,
So,
3λ + 2 – (4λ – 1) + 2λ + 2 – 5 = 0
⇒ λ = 0
Now, put this value in eq(1), we get
x = 2, y = -1, z = 2
The angle between the line and the plane is
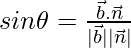
Here, 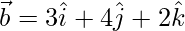
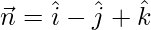
⇒ 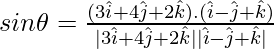
⇒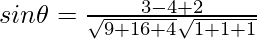
⇒ 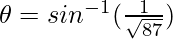
Question 21. Find the vector equation of the plane passing through (1, 2, 3) and perpendicular to the plane 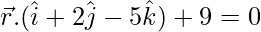 .
.
Solution:
Let us assume that the direction ratios are a, b, c
Given that the line passes through (1, 2, 3), so the equation of the line is
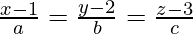 ….(1)
….(1)
And the line is perpendicular to the plane 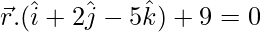
So, the line is parallel to the normal of the plane.
Now, the direction ratios are proportional to those of the given plane.
⇒ a = λ, b = 2λ, c = -5λ
Put these values in eq(1), we get
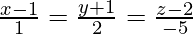
So, the vector form is
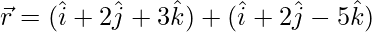 is the required equation.
is the required equation.
Question 22. Find the angle between the line 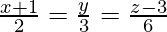 and the plane 10x + 2y – 11z = 3.
and the plane 10x + 2y – 11z = 3.
Solution:
Given that the equation of the line is 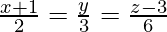 and the equation of the plane is 10x + 2y – 11z = 3
and the equation of the plane is 10x + 2y – 11z = 3
So, 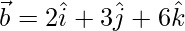
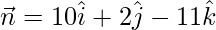
As we know that the angle between a line and a plane is
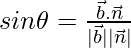
= 
⇒ θ = sin−1(−8/21)
Question 23. Find the vector equation of the line passing through (1, 2, 3) and parallel to the planes 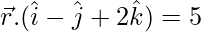 and
and 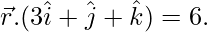
Solution:
Let us assume, ab, b, c are the direction ratios of the required line.
Given that the line is passes through (1, 2, 3). So the equation of the line is
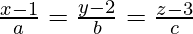 …(1)
…(1)
Also given that eq(1) is parallel to the planes 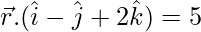 and
and  .
.
So, a – b + 2c = 0 …(2)
3a + b + z = 0 …(3)
Now on solving eq(2) and (3), we get
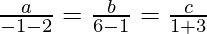

=> a = -3λ, b = 5λ, c = 4λ
Now put these values in eq(1), we get
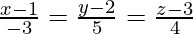 which is the cartesian form of the required line.
which is the cartesian form of the required line.
Question 24. Find the value of λ such that the line 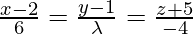 is perpendicular to the plane 3x − y − 2z = 7.
is perpendicular to the plane 3x − y − 2z = 7.
Solution:
Given that the equation of line is 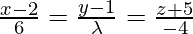 and
and
the equation of the plane is 3x − y − 2z = 7 and the line is perpendicular to the plane
So, the direction ratios of the given line are proportional to 6, λ ,-4.
and the direction ratios of the plane are 3, -1, -2.
Thus the line is parallel to the given plane, the line is perpendicular
to the normal of the given plane. So,
⇒ (6)(3) + (-1)(-4) + (-2 )(11) = 0
⇒ λ = 26
Question 25. Find the equation of the plane passing through the points (−1, 2, 0), (2, 2, −1) and parallel to the line 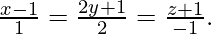
Solution:
The general equation of the plane passing through the point (−1, 2, 0) is
a(x+1) + b(y-2) + c( z – 0) = 0 ….(1)
This plane passes through the point (2, 2,−1), we get
a(2 + 1) + b(2 – 2) + c( -1 – 0) = 0
⇒ 3a – c = 0 ….(2)
Now, a, b, c are the direction ratio of the normal to the plane (1) and
the normal is perpendicular to the line, so
a + 2b + c = 0 ….(3)
Now on solving eq(2) and (3), we get

a = λ, b = -2 λ, c = 3λ
Now put all these values in eq(1), we get
λ(x + 1) – 2λ(y – 2) + 3λ(z – 0) = 0
x + 2y + 3z = 3
Thus, the equation of the required plane is x + 2y + 3z = 3.
Share your thoughts in the comments
Please Login to comment...