Question 1 (i). Verify Lagrange’s mean value theorem for the following function on the indicated interval. In each case find a point ‘c’ in the indicated interval as stated by the lagrange’s mean value theorem f(x) = x2 – 1 on [2, 3]
Solution:
Given that
f(x) = x2 – 1
Since the given f(x) = x2 – 1 is a polynomial function.
So, the given f(x) is continuous on [2, 3] and differentiable in (2, 3)
Hence, both the conditions of Lagrange’s mean value theorem are satisfied.
So, there exist a point c ∈ (2, 3) such that,
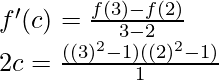
2c = (8 – 3)
c = 5/2 ∈ (2, 3)
Hence, the Lagrange’s mean value theorem is verified.
Question 1 (ii). Verify Lagrange’s mean value theorem for the following on the indicated interval. In each case find a point ‘c’ in the indicated interval as stated by the Lagrange’s mean value theorem f(x) = x3 – 2x2 – x + 3 on [0, 1].
Solution:
Given that
f(x) = x3 – 2x2 – x + 3
Since the given f(x) = x3 – 2x2 – x + 3 is a polynomial function.
So, the given f(x) is continuous on [0, 1] and differentiable in (0, 1)
Hence, both the conditions of Lagrange’s mean value theorem are satisfied.
So, there exist a point c ∈ (0, 1) such that,
![Rendered by QuickLaTeX.com f'(c)=\frac{f(1)-f(0)}{1-0}\\ 3c^2-4c-1=\frac{[(1)^2-2(1)^2-(1)+3]-3}{1}](https://www.geeksforgeeks.org/wp-content/ql-cache/quicklatex.com-f77aac287d21e590bbae00e61046a3a8_l3.png)
3c2 – 4ac – 1 = 1 – 3
3c2 – 4c + 1 = 0
3c2 – 3c – c + 1 = 0
3c(c – 1) – 1(c – 1) = 0
(3c – 1)(c – 1) = 0
c = 1/3 ∈ (0, 1)
Hence, the Lagrange’s mean value theorem is verified.
Question 1 (iii). Verify Lagrange’s mean value theorem for the following function on the indicated interval. In each case find a point ‘c’ in the indicated interval as stated by the Lagrange’s mean value theorem f(x) = x(x – 1) on [1, 2].
Solution:
Given that
f(x) = x(x – 1) ⇒ x2 – x
Since the given f(x) = x2 – x is a polynomial function.
So, the given f(x) is continuous on [1, 2] and differentiable in (1, 2)
Hence, both the conditions of Lagrange’s mean value theorem are satisfied.
So, there exist a point c ∈ (1, 2) such that,
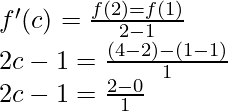
2c = 3
c = 3/2 ∈ (1, 2)
Hence, the Lagrange’s mean value theorem is verified.
Question 1 (iv). Verify Lagrange’s mean value theorem for the following function on the indicates interval. In each case find a point ‘c’ in the indicated interval as stated by the Lagrange’s mean value theorem f(x) = x2 – 3x + 2 on [-1, 2].
Solution:
Given that
f(x) = x2 – 3x + 2
Since the given f(x) = x2 – 3x + 2 is a polynomial function.
So, the given f(x) is continuous on [-1, 2] and differentiable in (-1, 2)
Hence, both the conditions of Lagrange’s mean value theorem are satisfied.
So, there exist a point c ∈ (-1, 2) such that,
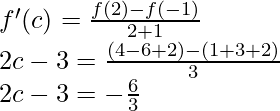
2c – 3 = -2
2c = 1
c = 1/2 ∈ (-1, 2)
Hence, the Lagrange’s mean value theorem is verified.
Question 1 (v). Verify Lagrange’s mean value theorem for the following function on the indicated interval. In each case find a point ‘c’ in the indicated interval as stated by the Lagrange’s mean value theorem f(x) = 2x2 – 3x + 1 on [1, 3].
Solution:
Given that,
f(x) = 2x2 – 3x + 1
Since the given f(x) = 2x2 – 3x + 1 is a polynomial function.
So, the given f(x) is continuous on [1, 3] and differentiable in (1, 3)
Hence, both the conditions of Lagrange’s mean value theorem are satisfied.
So, there exist a point c ∈ (1, 3) such that,
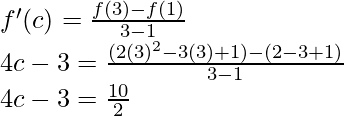
4c – 3 = 5
4c = 5 + 3
4c = 8
c = 2 ∈ (1, 3)
Hence, the Lagrange’s mean value theorem is verified.
Question 1 (vi). Verify Lagrange’s mean value theorem for the following function on the indicated interval. In each case find a point ‘c’ in the indicated interval as stated by the Lagrange’s mean value theorem f(x) = x2 – 2x + 4 on [1, 5].
Solution:
Given that,
f(x) = x2 – 2x + 4
Since the given f(x) = x2 – 2x + 4 is a polynomial function.
So, the given f(x) is continuous on [1, 5] and differentiable in (1, 5)
Hence, both the conditions of Lagrange’s mean value theorem are satisfied.
So, there exist a point c ∈ (1, 5) such that,
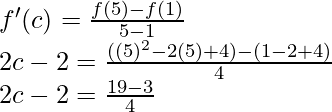
2c – 2 = 16/4
2c – 2 = 4
2c = 6
c = 3 ∈ (1, 5)
Hence, the Lagrange’s mean value theorem is verified.
Question 1 (vii). Verify Lagrange’s mean value theorem for the following function on the indicated interval. In each find a point ‘c’ in the indicated interval as stated by the Lagrange’s mean value theorem f(x) = 2x – x2 on [0, 1].
Solution:
Given that,
f(x) = 2x – x2
Since the given f(x) = 2x – x2 is a polynomial function.
So, the given f(x) is continuous on [0, 1] and differentiable in (0, 1)
Hence, both the conditions of Lagrange’s mean value theorem are satisfied.
So, there exist a point c ∈ (0, 1) such that,
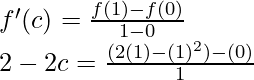
2 – 2c = 1
1 = 2c
c = 1/2 ∈ (0, 1)
Hence, the Lagrange’s mean value theorem is verified.
Question 1 (viii). Verify Lagrange’s mean value theorem for the following function on the indicated interval. In each find a point ‘c’ in the indicated interval as stated by the Lagrange’s mean value theorem f(x) = (x – 1)(x – 2)(x – 3) on [0, 4].
Solution:
Given that,
f(x) = (x – 1)(x – 2)(x – 3)
Since the given f(x) = (x – 1)(x – 2)(x – 3) is a polynomial function.
So, the given f(x) is continuous on [0, 4] and differentiable in (0, 4)
Hence, both the conditions of Lagrange’s mean value theorem are satisfied.
So, there exist a point c ∈ (0, 4) such that,
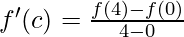
(c – 1)(c – 2) + (c – 2)(c – 3) + (c – 1)(c – 3)
c2 – 3c + 2 + c2 + 5c + 6 + c2 – 4c + 3 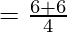
3c2 – 12c + 11 = 3
3c2 = 12c + 8 = 0

Hence, the Lagrange’s mean value theorem is verified.
Question 1 (ix). Verify Lagrange’s mean value theorem for the following function on the indicated interval. In each find a point ‘c’ in the indicated interval as stated by the Lagrange’s mean value theorem f(x) 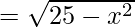 on [-3, 4].
on [-3, 4].
Solution:
Given that,
f(x) 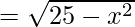
The given f(x) is exists, if
25 – x2 ≥ 0
x2 ≤ 25
x ≤ ±5
-5 < x < 5
So, f(x) has unique value ∀ x ∈(-5. 5), so the given f(x) is continuous on [-3, 4].
Now, differentiate f(x) = (25 – x2)1/2 with respect to x, we get
f'(x) = 1/2(25 – x2)1/2-1d(25 – x2)/dx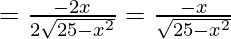
So, f'(x) exists∀ x ∈ (-3, 4), hence, f(x) is differential on (-3, 4).
Hence, both the conditions of Lagrange’s mean value theorem are satisfied.
So, there exist a point c ∈ (-3, 4) such that,
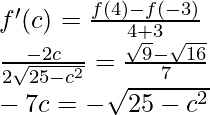
On squaring both sides, we get
49c2 = 25 – c2
c2 = 1/2
c = ±1/√2
Hence, the Lagrange’s mean value theorem is verified.
Question 1 (x). Verify Lagrange’s mean value theorem for the following function on the indicated interval. In each find a point ‘c’ in the indicated interval as stated by the Lagrange’s mean value theorem f(x) = tan-1x on [0, 1].
Solution:
Given that,
f(x) = tan-1x
Here, the given f(x) is is continuous on [0, 1] and differentiable on (0, 1)
Hence, both the conditions of Lagrange’s mean value theorem are satisfied.
So, there exist a point c ∈ (0, 1) such that,
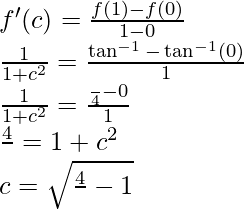
Hence, the Lagrange’s mean value theorem is verified.
Question 1 (xi). Verify Lagrange’s mean value theorem for the following function on the indicated interval. In each find a point ‘c’ in the indicated interval as stated by the Lagrange’s mean value theorem f(x) = x + 1/x on [1, 3].
Solution:
Given that,
f(x) = x + 1/x ⇒ (x2 + 1)/x
Here, the given f(x) is continuous on [1, 3] and differentiable on (1, 3)
Hence, both the conditions of Lagrange’s mean value theorem are satisfied.
So, there exist a point c ∈ (1, 3) such that,
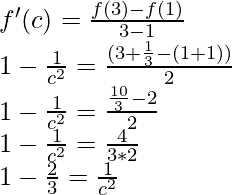
c2 = 3
c = √3 ∈ (1, 3)
Hence, the Lagrange’s mean value theorem is verified.
Question 1 (xii). Verify Lagrange’s mean value theorem for the following function on the indicated interval. In each find a point ‘c’ in the indicated interval as stated by the Lagrange’s mean value theorem f(x) = x (x + 4)2 on [1, 3].
Solution:
Given that,
f(x) = x (x + 4)2
Since the given f(x) = x (x + 4)2 is a polynomial function.
So, the given f(x) is continuous on [1, 3] and differentiable in (1, 3)
Hence, both the conditions of Lagrange’s mean value theorem are satisfied.
So, there exist a point c ∈ (1, 3) such that,
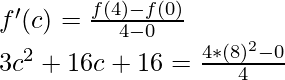
3c2 + 16c + 16 = 64
3c2 + 16c – 48 = 0
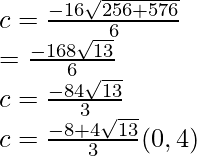
Hence, the Lagrange’s mean value theorem is verified.
Question 1 (xiii) Verify Lagrange’s mean value theorem for the following function on the indicated interval. In each find a point ‘c’ in the indicated interval as stated by the Lagrange’s mean value theorem f(x) = x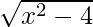 on [2, 4].
on [2, 4].
Solution:
Given that,
f(x) = x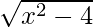
The given f(x) is exists, if
x2 – 4≥ 0
x2 ≤ 4
x ≤ ±2
-2 < x < 2
So, f(x) has unique value ∀ x ∈(-2. 2), so the given f(x) is continuous on [2, 4].
Now, differentiate f(x) = (x2 – 4)1/2 with respect to x, we get
f'(x) = 1/2(x2 – 4)1/2-1d(x2 – 4)/dx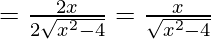
So, f'(x) exists∀ x ∈ (2, 4), hence, f(x) is differential on (2, 4).
Hence, both the conditions of Lagrange’s mean value theorem are satisfied.
So, there exist a point c ∈ (2, 4) such that,
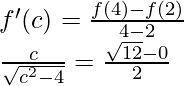
On squaring both sides, we get
⇒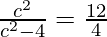
⇒4c2 = 12c2 – 48
⇒8c2 = 48
⇒c2 = 6
⇒c = √6 ∈ (2, 4)
Hence, the Lagrange’s mean value theorem is verified.
Question 1 (xiv). Verify Lagrange’s mean value theorem for the following function on the indicated interval. In each find a point ‘c’ in the indicated interval as stated by the Lagrange’s mean value theorem f(x) = x2 + x – 1 on [0, 4].
Solution:
Given that,
f(x) = x2 + x – 1
Since the given f(x) = x2 + x – 1 is a polynomial function.
So, the given f(x) is continuous on [0, 4] and differentiable in (0, 4)
Hence, both the conditions of Lagrange’s mean value theorem are satisfied.
So, there exist a point c ∈ (0, 4) such that,
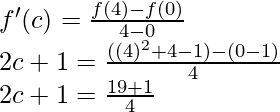
2c + 1 = 20/4
2c + 1 = 5
c = 2 ∈ (0, 4)
Hence, the Lagrange’s mean value theorem is verified.
Question 1 (xv) Verify Lagrange’s mean value theorem for the following function on the indicated interval. In each find a point ‘c’ in the indicated interval as stated by the Lagrange’s mean value theorem f(x) = sin x – sin2x – x on [0, π].
Solution:
Given that,
f(x) = sin x – sin2x – x
Since the given f(x) = sin x – sin2x – x is a polynomial function.
So, the given f(x) is continuous on [0, π] and differentiable in (0, π)
Hence, both the conditions of Lagrange’s mean value theorem are satisfied.
So, there exist a point c ∈ (0, π) such that,
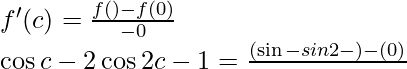
⇒cos c – 2cos2c = -1 + 1
⇒cos – 2(2cos2c – 1) = 0
⇒4cos2c – cosc – 2 = 0
⇒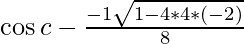
⇒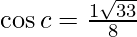
⇒
Hence, the Lagrange’s mean value theorem is verified.
Question 1 (xvi). Verify Lagrange’s mean value theorem for the following function on the indicated interval. In each find a point ‘c’ in the indicated interval as stated by the Lagrange’s mean value theorem f(x) = x3 – 5x2 – 3x on [1, 3]
Solution:
Given that,
f(x) = x3 – 5x2 – 3x
Since the given f(x) = x3 – 5x2 – 3x is a polynomial function.
So, the given f(x) is continuous on [1, 3] and differentiable in (1, 3)
Hence, both the conditions of Lagrange’s mean value theorem are satisfied.
So, there exist a point c ∈ (1, 3) such that,

Now, f'(c) = 3c2 – 10c – 3, f(1) = -7, f(3) = -27
3c2 – 10c – 3 = 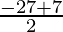
3c2 – 10c – 3 = -10
3c2 – 10c + 7 = 0
3c2 – 3c – 7c + 7 = 0
c = 7/3, where c = 7/3 ∈ (1, 3)
Hence, the Lagrange’s mean value theorem is verified.
Question 2. Discuss the applicable of Lagrange’s mean value theorem for the function f(x) = |x| on [-1, 1].
Solution:
Given that,
f(x) = |x|
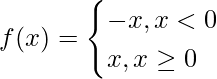
Now, we check differentiability at x = 0
LHD = 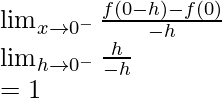
RHD = 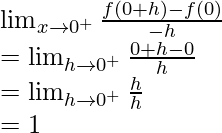
LHD ≠ RHD
Here, f(x) is not differentiable at x = 0 ∈ (-1, 1)
Hence, the Lagrange’s mean value theorem is not applicable for the given f(x) = \x\.
Question 3. Show that the lagrange’s mean value theorem is not applicable to the function f(x) = 1/x on [-1, 1].
Solution:
Given that,
f(x) = 1/x
On differentiating with respect to x, we get

So, we conclude that f'(x) does not exist at x = 0 ∈ (-1, 1)
So, f(x) is also not differentiable in (-1, 1)
Hence, the Lagrange’s mean value theorem is not applicable for the given f(x) = 1/x.
Question 4. Verify the hypothesis and conclusion of Lagrange’s mean value theorem for the function f(x) 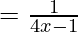 , 1 ≤ x ≤ 4.
, 1 ≤ x ≤ 4.
Solution:
Given that,
f(x) 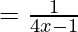
Here, the given f(x) has a unique value for each x ∈ [1, 4], so f(x) is continuous in [1, 4].
Now differentiate f(x) with respect to x, we get
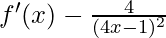
So, here f'(x) exits for all x ∈ (1, 4), hence, f(x) is differentiable on (1, 4)
Hence, both the conditions of Lagrange’s mean value theorem are satisfied.
So, there exist a point c ∈ (1, 4) such that,
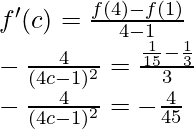
(4c – 1)2 = 45
4c – 1 = ±3√5
![Rendered by QuickLaTeX.com c=\frac{3\sqrt{5}+1}{4}∈[1,4]](https://www.geeksforgeeks.org/wp-content/ql-cache/quicklatex.com-f8ad62d30a8e2c861cb33b620667a225_l3.png)
Hence, the Lagrange’s mean value theorem is verified.
Question 5. Find a point on the parabola y = (x – 4)2, where the tangent is parallel to the chord joining (4, 0) and (5, 1).
Solution:
Given that,
f(x) = y = (x – 4)2⇒ x2 – 8x + 16
Also it is given that the tangent is parallel to the chord joining (4, 0) and (5, 1)
So, let us considered the chord joining the points are (4, 5)
Since the given f(x) = x2 – 8x + 16 is a polynomial function.
So, the given f(x) is continuous on [4, 5] and differentiable in (4, 5)
Hence, both the conditions of Lagrange’s mean value theorem are satisfied.
So, there exist a point c ∈ (4, 5) such that,

2c – 8 = 1
2c = 9
c = 9/2
So,
f(c) = (c – 4)2
f(c) = (9/2 – 4)2
f(c) = 1/4
Hence, (c, f(c)) = (9/2, 1/4) is the given point.
Question 6. Find a point on the curve y = x2 + x, where the tangent is parallel to the chord joining (0, 0) and (1, 2).
Solution:
Given that,
f(x) = y = x2 + x
Also it is given that the tangent is parallel to the chord joining (0, 0) and (1, 2)
So, let us considered the chord joining the points are (0, 1)
Since the given f(x) = x2 + x is a polynomial function.
So, the given f(x) is continuous on [0, 1] and differentiable in (0, 1)
Hence, both the conditions of Lagrange’s mean value theorem are satisfied.
So, there exist a point c ∈ (0, 1) such that,
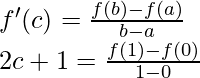
2c + 1 = 2
c = 1/2
So,
f(c) = c2 + c
f(c) = (1/2)2 + 1/2
f(c) = 3/4
Hence, (c, f(c)) = (1/2, 3/4) is the given point.
Question 7. Find a point on the probably y = (x – 3)2, where the tangent is parallel to the chord joining (3, 0) and (4, 1).
Solution:
Given that,
f(x) = y = (x – 3)2 = x2 – 6x + 9
Also it is given that the tangent is parallel to the chord joining (3, 0) and (4, 1)
So, let us considered the chord joining the points are (3, 4)
Since the given f(x) = x2 – 6x + 9 is a polynomial function.
So, the given f(x) is continuous on [3, 4] and differentiable in (3, 4)
Hence, both the conditions of Lagrange’s mean value theorem are satisfied.
So, there exist a point c ∈ (3, 4) such that,
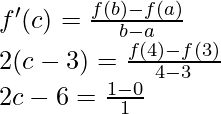
2c = 7
c = 7/2
So,
f(c) = (c – 3)2
f(c) = (7/2 – 3)2
f(c) = 1/4
Hence, (c, f(c)) = (7/2, 1/4) is the given point.
Question 8. Find the points on the curve y = x3 – 3x, which the tangent to the curve is parallel to the chord joining (1, -2) and (2, 2).
Solution:
Given that,
f(x) = y = x3 – 3x
Also it is given that the tangent is parallel to the chord joining (1, -2) and (2, 2)
So, let us considered the chord joining the points are (1, 2)
Since the given f(x) = x3 – 3x is a polynomial function.
So, the given f(x) is continuous on [1, 2] and differentiable in (1, 2)
Hence, both the conditions of Lagrange’s mean value theorem are satisfied.
So, there exist a point c ∈ (1, 2) such that,
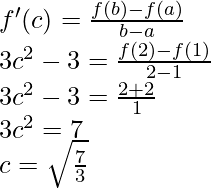
So,
f(c) = (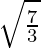 )3 – 3
)3 – 3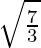
f(c) = 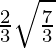
Hence, (c, f(c)) = (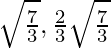 ) is the given point.
) is the given point.
Question 9. Find a point on the curve y = x3 + 1 where the tangent is parallel to the chord joining (1, 2) and (3, 28).
Solution:
Given that,
f(x) = y = x3 + 1
Also it is given that the tangent is parallel to the chord joining (1, 2) and (3, 28)
So, let us considered the chord joining the points are (1, 3)
Since the given f(x) = x3 + 1 is a polynomial function.
So, the given f(x) is continuous on [1, 3] and differentiable in (1, 3)
Hence, both the conditions of Lagrange’s mean value theorem are satisfied.
So, there exist a point c ∈ (1, 3) such that,
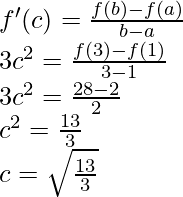
So,
f(c) = c3 + 1
f(c) = ( )3 + 1
)3 + 1
Hence, (c, f(c)) = ( , (
, ( )3 + 1) is the given point.
)3 + 1) is the given point.
Question 10. Let c be a curve defined parametrically as x = a cos3θ, y = a sin3θ, 0 ≤ θ ≤ π/2. Determine a point P on C, where the tangent to C is parallel to the chord joining the points (a, 0) and (0, a).
Solution:
Let us considered point P be (x, y)
So, given that,
x = a cos3θ ….(1)
y = a sin3θ ….(2)
Now we find the slope of tangent
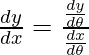 ….(3)
….(3)
So, differentiate eq(1), w.r.t. θ, we get,
dx/dθ = -3acos2θsinθ
Now, differentiate eq(2), w.r.t. θ, we get,
dx/dθ = 3a sin2θcosθ
Now put all these values in eq(3), we get
dy/dx = -3acos2θsinθ/3a sin2θcosθ = -tanθ
Now the two given points are (a, 0) and (0, a).
So, the slope of the given chord , m = a – 0/0 – a = -1
According to the question it is given that point C is parallel to the chord
So, slope of tangent = slope of the chord
-tanθ = -1
θ = π/4
Now put the value of θ in eq(1) and (2), we get the values of point P
x = a cos3(π/4) = a/2√2
y = a sin3(π/4) = a/2√2
So, the point P(a/2√2, a/2√2)
Question 11. Using Lagrange’s mean value theorem, prove that (b – a)sec2a < tanb – tana < (b – a)sec2b where 0 < a < b < π/2.
Solution:
Given that,
f(x) = tan x, x ∈ [a, b] such that 0 < a < b < π/2}
So, the given f(x) is continuous on [a, b] and differentiable in (a, b)
Hence, both the conditions of Lagrange’s mean value theorem are satisfied.
So, there exist a point c ∈ (a, b) such that,
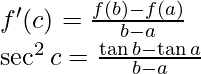 ….(i)
….(i)
So, c ∈ (a, b)
sec2a < 
Now, using eq(i), we get
(b – a)sec2a < (tan b – tan a) < (b – a)sec2b
Share your thoughts in the comments
Please Login to comment...