Class 11 RD Sharma Solutions – Chapter 28 Introduction to 3D Coordinate Geometry – Exercise 28.2 | Set 2

Read

Discuss

Question 13. Prove that the tetrahedron with vertices at points O(0,0,0), A(0,1,1), B(1,0,1) and C(1,1,0) is a regular one.

Solution:

Given: The points O(0,0,0), A(0,1,1), B(1,0,1) and C(1,1,0).

A regular tetrahedron has all equal sides and diagonals.

We know that the distance between two points (a, b, c) and (d, e, f) is given as follows:

$\sqrt{(a − d)^2 + (b − e)^2 + (c − f)^2}$

$AB = \sqrt{(0 − 1)^2 + (1 − 0)^2 + (1 −1)^2}$

$= \sqrt{2}$

$BC = \sqrt{(1 − 1)^2 + (0 −1)^2 + (1 −0)^2}$

$= \sqrt{2}$

$CA = \sqrt{(1 − 0)^2 + (1 −1)^2 + (0 −1)^2}$

$= \sqrt{2}$

$OA = \sqrt{(0 − 0)^2 + (0 −1)^2 + (0 −1)^2}$

$= \sqrt{2}$

$OB = \sqrt{(0 − 1)^2 + (0 − 0)^2 + (0 −1)^2}$

$= \sqrt{2}$

$OC = \sqrt{(0 − 1)^2 + (0 −1)^2 + (0 −0)^2}$

$= \sqrt{2}$

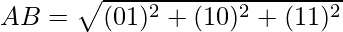
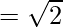
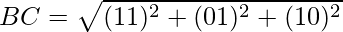
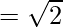
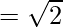
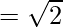
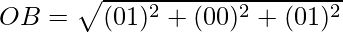
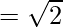
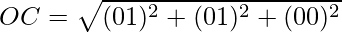
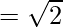
Clearly, OA = OB = OC = AB = BC = CA.

Hence O, A, B and C represent a regular tetrahedron.

Question 14. Show that the points (3,2,2), (-1,1,3), (0,5,6), (2,1,2) lie on a sphere whose centre is (1,3,4). Also find its radius.

Solution:

Given: The points A(3,2,2), B(-1,1,3), C(0,5,6), D(2,1,2) and E(1,3,4)

We know that the distance between two points (a, b, c) and (d, e, f) is given as follows:

$\sqrt{(a − d)^2 + (b − e)^2 + (c − f)^2}$

$EA = \sqrt{(1 − 3)^2 + (3 − 2)^2 + (4 − 2)^2}$

= 3

$EB = \sqrt{(1 + 1)^2 + (3 − 1)^2 + (4 − 3)^2}$

= 3

$EC = \sqrt{(1 − 0)^2 + (3 − 5)^2 + (4 − 6)^2}$

= 3

$ED = \sqrt{(1 − 2)^2 + (3 − 1)^2 + (4 − 2)^2}$

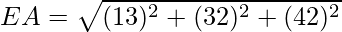
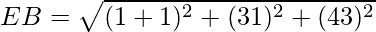
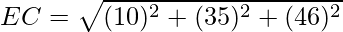
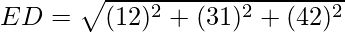
= 3

Since EA = EB = EC = ED, the points lie on a sphere with centre E.

Radius of the sphere = 3 units.

Question 15. Find the coordinates of the point which is equidistant from the four points O(0,0,0), A(2,0,0), B(0,3,0) and C(0,0,8).

Solution:

Given: Points O(0,0,0), A(2,0,0), B(0,3,0) and C(0,0,8).

Let the required point be P(x,y,z).

We are given that OP = PA ⇒ OP² = PA²

Using formula, we have:

$\sqrt{(x − 0)^2 + (y − 0)^2 + (z − 0)^2} = \sqrt{(x − 2)^2 + (y − 0)^2 + (z − 0)^2}$

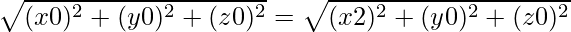
⇒ x² + y²+ z² = x² − 4x + 4 + y² +z²

⇒ 4x = 4

⇒ x = 1

Similarly, OP² = PB²

⇒ $\sqrt{(x − 0)^2 + (y − 0)^2 + (z − 0)^2} = \sqrt{(x − 0)^2 + (y − 3)^2 + (z − 0)^2}$

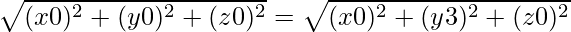
⇒ x² + y² + z² = x² + y² − 6y +9 +z²

⇒ 6y = 9

⇒ y = 3/2

Also, OP² = PC²

⇒ $\sqrt{(x − 0)^2 + (y − 0)^2 + (z − 0)^2} = \sqrt{(x − 0)^2 + (y − 0)^2 + (z − 8)^2}$

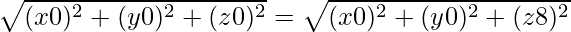
⇒ x² + y² + z² = x² + y² +z² − 16z + 64

⇒ 16z = 64

⇒ z = 4

Hence the point is P[1, 3/2, 4].

Question 16. If A(-2,2,3) and B(13,-3,13) are two points, find the locus of a point P which moves in a way such that 3PA = 2PB.

Solution:

Given: A(-2,2,3) and B(13,-3,13)

Let P = (x, y, z) be the required point.

We are given 3PA = 2PB

Using the formula,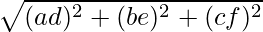 $\sqrt{(a − d)^2 + (b − e)^2 + (c − f)^2}$ , we have:

$3\sqrt{(x + 2)^2+(y − 2)^2+(z − 3)^2} = 2\sqrt{(x − 13)^2+(y + 3)^2+(z − 13)^2}$

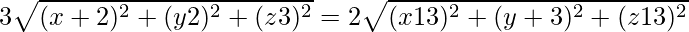
Squaring both sides, we have;

9(x² + 4x +4 + y² + 4 − 4y + z² + 9 − 6z) = 4(x² + 169 − 26x + y² +9 + 6y + z² + 169 − 26z)

⇒ 5(x² + y² +z²) + 140x − 60y + 50z − 1235 = 0.

Question 17. Find the locus of P if PA²+ PB² = 2k², where A and B are the points (3,4,5) and (-1,3,-7).

Solution:

Given: A(3,4,5) and B(-1,3,-7)

Let P(x, y, z) be the required point.

PA² + PB² = 2k². Using the formula,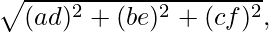 $\sqrt{(a − d)^2 + (b − e)^2 + (c − f)^2},$ we have:

$\sqrt{(x − 3)^2 + (y − 4)^2 + (z − 5)^2} + \sqrt{(x+1)^2 + (y − 3)^2 + (z+7)^2} = 2k^2$

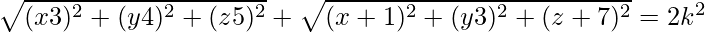
⇒ 2x² + 2y² + 2z² − 4x −14y + 4z + 109 − 2k² = 0

⇒ 2(x² + y² +z²) − 4x −14y + 4z + 109 − 2k2 = 0.

Question 18. Show that the points A(a, b, c), B(b, c, a) and C(c, a, b) are vertices of an equilateral triangle.

Solution:

Given: points A(a, b, c), B(b, c, a) and C(c, a, b)

Using formula, distance between two points (a, b, c) and (d, e, f) is given as follows:

$\sqrt{(a − d)^2 + (b − e)^2 + (c − f)^2}$

$AB = \sqrt{(a − b)^2 + (b − c)^2 + (c − a)^2}$

$= \sqrt{a^2 + b^2 - 2ab + b^2 + c^2 - 2bc + c^2 + a^2 - 2ac}$

$= \sqrt{2a^2 + 2b^2 + 2c^2 - 2ab - 2bc - 2ac}$

$BC = \sqrt{(b − c)^2 + (c − a)^2 + (a − b)^2}$

$= \sqrt{2a^2 + 2b^2 + 2c^2 - 2ab - 2bc - 2ac}$

$CA = \sqrt{(a − c)^2 + (b − a)^2 + (c − b)^2}$

$= \sqrt{2a^2 + 2b^2 + 2c^2 - 2ab - 2bc - 2ac}$

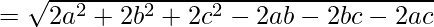
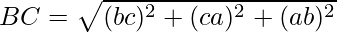
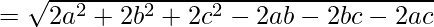
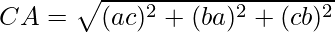
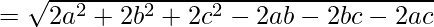
Since AB = BC = CA

ABC is an equilateral triangle.

Question 19. Are points A(3,6,9), B(10,20,30), and C(25,41,5) the vertices of a right-angled triangle?

Solution:

Given: A(3,6,9), B(10,20,30) and C(25,41,5)

Using formula, distance between two points (a, b, c) and (d, e, f) is given as follows:

$\sqrt{(a − d)^2 + (b − e)^2 + (c − f)^2}$

$AB = \sqrt{(3 − 10)^2 + (6 − 20)^2 + (9 − 30)^2}$

$= \sqrt{49 + 196 + 441}$

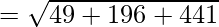
⇒ AB²= 586

$BC = \sqrt{(10 − 25)^2 + (20 + 41)^2 + (30 − 5)^2}$

$= \sqrt{225 + 3721 + 625}$

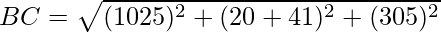
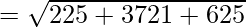
⇒ BC²= 4571

$CA = \sqrt{(3 − 25)^2 + (6 + 41)^2 + (9 − 5)^2}$

$= \sqrt{484 + 2209 + 16}$

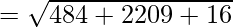
⇒ CA²= 2709

Since, AB² + BC² ≠ AC²

AB² + AC²≠ BC²

BC² + AC² ≠ AB²

ABC is not a right triangle.

Question 20. Verify that:

(i) (0,7,-10), (1,6,-6) and (4,9,-6) are the vertices of an isosceles triangle.

Solution:

Given: A(0,7,-10), B(1,6,-6) and C(4,9,-6)

Using formula, distance between two points (a, b, c) and (d, e, f) is given as follows:

$\sqrt{(a − d)^2 + (b − e)^2 + (c − f)^2}$

$AB = \sqrt{(0 − 1)^2 + (7 − 6)^2 + (-10 + 6)^2}$

$= \sqrt{1 + 1 + 16} = 3\sqrt{2}$

$BC = \sqrt{(1 − 4)^2 + (6 − 9)^2 + (6 − 6)^2}$

$= \sqrt{9 + 9} = 3\sqrt{2}$

$CA = \sqrt{(0 − 4)^2 + (7 − 9)^2 + (-10 + 6)^2}$

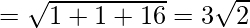
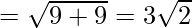
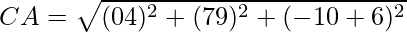
= 6

Since AB = BC, ABC is an isosceles triangle.

(ii) (0,7,-10), (-1,6,6) and (4,9,-6) are the vertices of a right-angled triangle.

Solution:

Given: Given: A(0,7,-10), B(1,6,-6) and C(4,9,-6)

Using formula, distance between two points (a, b, c) and (d, e, f) is given as follows:

$\sqrt{(a − d)^2 + (b − e)^2 + (c − f)^2}$

$AB = \sqrt{(0 + 1)^2 + (7 − 6)^2 + (10 - 6)^2}$

$= \sqrt{1 + 1 + 16} = 3\sqrt{2}$

$BC = \sqrt{(-1 + 4)^2 + (6 − 9)^2 + (6 − 6)^2}$

$= \sqrt{9 + 9} = 3\sqrt{2}$

$CA = \sqrt{(-4 − 0)^2 + (9 − 7)^2 + (6 + 10)^2}$

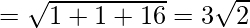
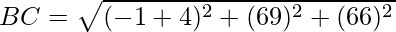
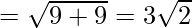
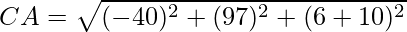
= 6

Since AB² + BC² = AC², ABC is a right triangle.

(iii) (-1,2,1), (1,-2,5), (4,-7,8) and (2,-3,4) are the vertices of a parallelogram.

Solution:

Given: A(-1,2,1), B(1,-2,5), C(4,-7,8) and D(2,-3,4)

Using formula, distance between two points (a, b, c) and (d, e, f) is given as follows:

$\sqrt{(a − d)^2 + (b − e)^2 + (c − f)^2}$

$AB = \sqrt{(-1 - 1)^2 + (2 + 2)^2 + (1 - 5)^2}$

$= \sqrt{36} = 6$

$BC = \sqrt{(1 − 4)^2 + (-2 + 7)^2 + (5− 8)^2}$

$= \sqrt{43}$

$CD = \sqrt{(4 − 2)^2 + (-7 + 3)^2 + (8 − 4)^2}$

$= \sqrt{36} = 6$

$DA = \sqrt{(2 + 1)^2 + (-3 − 2)^2 + (4− 1)^2}$

$= \sqrt{43}$

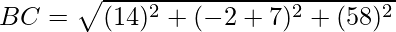
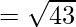
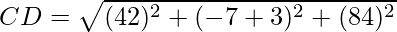
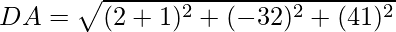
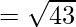
Since the opposite sides are equal, ABCD is a parallelogram.

(iv) (5,-1,1), (7,-4,7), (1,-6,10) and (-1,-3,4) are vertices of a rhombus.

Solution:

Given: A(5,-1,1), B(7,-4,7), C(1,-6,10) and D(-1,-3,4)

Using formula, distance between two points (a, b, c) and (d, e, f) is given as follows:

$\sqrt{(a − d)^2 + (b − e)^2 + (c − f)^2}$

$AB = \sqrt{(7 − 5)^2 + (-4 + 1)^2 + (7 − 1)^2}$

$= \sqrt{49} = 7$

$BC = \sqrt{(1 − 7)^2 + (-6 + 4)^2 + (10 − 7)^2}$

$= \sqrt{49} = 7$

$CD = \sqrt{(-1 − 1)^2 + (-3 + 6)^2 + (4 − 10)^2}$

$= \sqrt{49} = 7$

$AD = \sqrt{(-1 − 5)^2 + (-3 + 1)^2 + (4 − 1)^2}$

$= \sqrt{49} = 7$

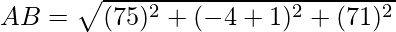
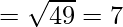
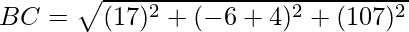
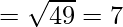
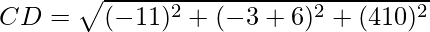
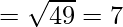
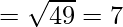
Since AB = BC = CA = AD

ABCD is a rhombus.

Question 21. Find the locus of the points which are equidistant from the points (1,2,3) and (3,2,-1).

Solution:

Let P(x, y, z) be the point equidistant from the points A(1,2,3) and B(3,2,-1).

Using formula, distance between two points (a, b, c) and (d, e, f) is given as follows:

$\sqrt{(a − d)^2 + (b − e)^2 + (c − f)^2}$

⇒ AP = BP or AP² = BP²

⇒ (x − 1)² + (y − 2)² + (z − 3)² = (x − 3)² + (y − 2)² + (z + 1)²

⇒ 4x − 8z = 14 − 14

⇒ x − 2z = 0.

Question 22. Show that the points A(1,2,3), B(-1.-2,-1), C(2,3,2) and D(7,4,6) are the vertices of a parallelogram ABCD.

Solution:

Using formula, distance between two points (a, b, c) and (d, e, f) is given as follows:

$\sqrt{(a − d)^2 + (b − e)^2 + (c − f)^2}$

$AB = \sqrt{(1 + 1)^2 + (2 + 2)^2 + (3 + 1)^2}$

$= \sqrt{36} = 6$

$BC = \sqrt{(-1 − 2)^2 + (-2 − 3)^2 + (-1− 2)^2}$

$= \sqrt{43}$

$CD = \sqrt{(2 − 4)^2 + (3 − 7)^2 + (2 − 6)^2}$

$= \sqrt{36} = 6$

$DA = \sqrt{(4 − 1)^2 + (7 − 2)^2 + (6− 3)^2}$

$= \sqrt{43}$

$AC = \sqrt{(1 -2)^2 + (2 - 3)^2 + (3 - 2)^2}$

$= \sqrt{3}$

$BD = \sqrt{(-1− 4)^2 + (-2 − 7)^2 + (-1 − 6)^2}$

$= \sqrt{155}$

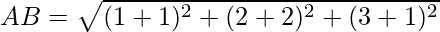
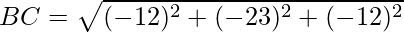
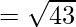
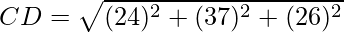
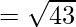
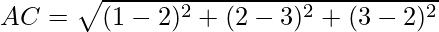
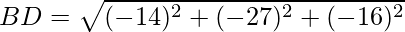
Since the opposite sides are equal, ABCD is a parallelogram.

Question 23: Find the locus of the point, the sum of whose distances from the points A(4,0,0) and B(-4,0,0) is equal to 10.

Solution:

Let P(x, y, z) be the required locus.

Given: PA + PB = 10. Using distance formula,

$\sqrt{(x − 4)^2+(y − 0)^2+(z − 0)^2} + \sqrt{(x + 4)^2+(y − 0)^2+(z − 0)^2} = 10$

$⇒ 4x + 25 = 5\sqrt{x^2 + y^2 + z^2 + 8x + 16}$

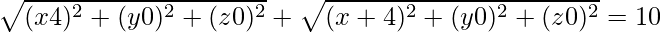
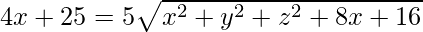
Squaring both sides, we get

16x² + 625 + 200x = 25(x² + y²+ z²+ 8x + 16)

⇒ 9x² + 25y² + 25z² – 225 = 0

Question 24. Find the equation of the set of points P such that its distances from the points A(3,4,-5) and B(-2,1,4) are equal.

Solution:

Given: A(3,4,-5) and B(-2,1,4)

Let P(x, y, z) be the required point, It is given that PA = PB.

Hence, PA² = PB²

Using distance formula, we have,

$⇒ \sqrt{(x − 3)^2+(y − 4)^2+(z + 5)^2} = \sqrt{(x + 2)^2+(y − 1)^2+(z − 4)^2}$

⇒ -6x + 9 – 8y + 16 + 10z + 25 = 4x + 4 – 2y +1 – 8z +16

⇒ 10x + 6y – 18z -29 = 0.

Whether you're preparing for your first job interview or aiming to upskill in this ever-evolving tech landscape, GeeksforGeeks Courses are your key to success. We provide top-quality content at affordable prices, all geared towards accelerating your growth in a time-bound manner. Join the millions we've already empowered, and we're here to do the same for you. Don't miss out - check it out now!

Last Updated : 30 Apr, 2021