Question 1. If sin A = 4/5 and cos B = 5/13, where 0 < A, B < π/2, find the values of the following:
(i) sin (A + B)
(ii) cos (A + B)
(iii) sin (A – B)
(iv) cos (A – B)
Solution:
Given that
sin A = 4/5 and cos B = 5/13
As we know, cos A = (1 – sin2A) and sin B = (1 – cos2B), where 0 <A, B < π/2
Now we find the value of cosA and sinB
cos A = √(1 – sin2A)
= √(1 – (4/5)2)
= √(1 – (16/25))
= √((25 – 16)/25)
= √(9/25)
= 3/5
sin B = √(1 – cos2B)
= √(1 – (5/13)2)
= √(1 – (25/169))
= √(169 – 25)/169)
= √(144/169)
= 12/13
(i) sin (A + B)
sin (A + B) = sinA cosB + cosA sinB
= 4/5 × 5/13 + 3/5 x 12/13
= 20/65 + 36/65
= (20 + 36)/65
= 56/65
(ii) cos (A + B)
cos (A + B) = cosA cosB – sinA sinB
= 3/5 × 5/13 – 4/5 x 12/13
= 15/65 – 48/65
= -33/65
(iii) sin (A – B)
sin (A – B) = sinA cosB – cosA sinB
= 4/5 × 5/13 – 3/5 x 12/13
= 20/65 – 36/65
= – 16/65
(iv) cos (A – B)
cos (A – B) = cos A cos B + sin A sin B
= 3/5 x 5/13 + 4/5 x 12/13
= 15/65 + 48/65
= 63/65
Question 2. (a) If sinA = 12/13 and sin B = 4/5, where π/2 < A <π and 0 < B π/2, find the following:
(i) sin (A + B)
(ii) cos (A + B)
Solution:
We have,
sinA = 12/13 and sinB = 4/5, where π/2 < A < and 0 < B < π/2
As we know, cosA = – √(1 – sin2A) and cosB = √(1 – sin2B)
Now we find the value of cosA and cosB
cosA = – √(1 – sin2A)
= – √(1 – (12/13)2)
= – √(1 – 144/169)
= – √((169 – 144)/169)
= – √(25/169)
= – 5/13
cosB = √(1 – sin2B)
= √(1 – (4/5)2)
= √(1 – 16/25)
= √((25 – 16)/25)
= √(9/25)
= 3/5
(i) sin (A + B)
Since, sin (A + B) = sinA cosB + cosA sinB
= 12/13 x 3/5 + (-5/13) x 4/5
= 36/65 – 20/65
= 16/65
(ii) cos (A + B)
Since, cos (A + B) = cos A cos B – sin A sin B
= – 5/13 x 3/5 – 12/13 x 4/5
= – 15/65 – 48/65
= – 63/65
(b) If sinA = 3/5, cosB = 12/13, where A and B, both lie in second quadrant, find the value of sin (A + B).
Solution:
We have,
sinA = 3/5, cosB = -12/13, where A and B, both lie in second quadrant.
As we know cosA = – √(1- sin2A) and sinB = √(1 – cos2B)
Now we find the value of cosA and sinB
cos A = – √(1 – sin2A)
= -√(1 – (3/5)2)
= -√(1 – 9/25)
= – √((25 – 9)/25)
= – √(16/25)
= – 4/5
sinB = √(1 – cos2B)
= √(1 – (-12/13)2)
= √(1 – 144/169)
= √((169 – 144)/169)
= √(25/169)
= 5/13
We need to find the value of sin (A + B)
Since, sin (A + B) = sinA cosB + cosA sinB
= 3/5 × (-12/13) + (-4/5) x 5/13
= -36/65 – 20/65
= -56/65
Question 3. If cosA = -24/25 and cosB = 3/5, where π < A < 3π/2 and 3π/2 < B < 2π, find the following:
(i) sin(A + B)
(ii) cos(A + B)
Solution:
We have,
cosA = -24/25 and cosB = 3/5, where π < A < 3π/2 and 3π/2 < B < 2π
As we know that A is present in third quadrant, B is
present in fourth quadrant, so the sine function is Negative.
By using the formulas, sinA = √(1 – cos2A) and sinB = -√(1 – cos2B)
We find the value of sinA and sinB
sinA = – √(1 – cos2A)
= – √(1 – (-24/25)2)
= – √(1 – 576/625)
= – √((625 – 576)/625)
= – √(49/625)
= – 7/25
sinB = – √(1 – cos2B)
= – √(1 – (3/5)²)
= – √(1 – 9/25)
= – √((25 – 9)/25)
= – √(16/25)
= – 4/5
(i) sin (A + B)
Since, sin (A + B) = sinA cosB + cosA sinB
= -7/25 x 3/5 + (-24/25) x (-4/5)
= -21/125 + 96/125
= 75/125
= 3/5
(ii) cos (A + B)
Since, cos (A + B) = cosA cosB – sinA sinB
= (-24/25) x 3/5 – (-7/25) × (-4/5)
= – 72/125 – 28/125
=- 100/125
= – 4/5
Question 4. If tanA = 3/4, cosB = 9/41, where π < A < 3π/2 and 0 < B < π/2, find tan(A + B).
Solution:
We have,
tanA = 3/4 and cosB = 9/41, where π < A < 3π/2 and 0 < B < π/2
As we know that, A is present in third quadrant, B is present in first quadrant
So, tan and sin functions are positive.
Now by using the formula,
sinB = √(1 – cos2B)
We find the value of sin B.
sinB = √(1 – cos2B)
= √(1 – (9/41)2)
= √(1 – 81/1681)
= √((1681 – 81)/1681)
= √(1600/1681)
= 40/41
As we know that, tanB = sinB/cosB, so
= (40/41)/(9/41)
= 40/9
Since, tan(A + B) = (tanA + tanB)/(1 – tanA tanB), so
= (3/4 + 40/9)/(1 – 3/4 x 40/9)
= (187/36)/(1 – 120/36)
= (187/36)/((36 – 120)/36)
= (187/36)/(- 84/36)
= -187/84
Hence, tan(A + B) = -187/84
Question 5. If sinA = 1/2, cosB = 12/13, where π/2 < A < π and 3π/2 < B < 2π, find tan(A – B).
Solution:
We have,
sinA = 1/2, cosB = 12/13, where π/2 < A < π and 3π/2 < B < 2π
As we know that, A is present in second quadrant and B is present in fourth quadrant.
So, the sine function is positive, cosine and tan functions are negative in second quadrant
and the sine and tan functions are negative, cosine function is positive in the fourth quadrant
Now by using the following formulas,
cosA = -√(1 – sin2A) and sinB = -√(1 – cos2B)
We find the value of cosA and sinB
cosA = – √(1 – sin2A)
= – √(1 – (1/2)2)
= – √(1 – 1/4)
= – √((4 – 1)/4)
= – √(3/4)
= – √3/2
sinB = – √(1 – cos2B)
= – √(1 – (12/13)2)
= – √(1 – 144/169)
= – √((169 – 144)/169)
= – √(25/169)
= – 5/13
As we know, tanA = sinA/cosA and tanB = sinB / cosB
tanA = (1/2)/(-√3/2) = -1/√3 and
tanB = (-5/13)/(12/13) = -5/12
Since, tan (A – B) = (tanA – tanB) / (1 + tanA tanB), so
= ((-1/√3) – (-5/12)) / (1 + (-1/√3) x (-5/12))
= ((-12 + 5√3)/12√3) / (1 + 5/12√3)
= ((-12 + 5√3)/12√3) / ((12√3 + 5)/12√3)
= (5√3 – 12)/(5 + 12√3)
Hence, tan (A – B) = (5√3 – 12)/(5 + 12√3)
Question 6. If sinA = 1/2, cosB = √3/2, where π/2 < A < π and 0 < B < π/2, find the following:
(i) tan(A + B)
(ii) tan(A – B)
Solution:
We have,
SinA = 1/2 and cosB = √3/2, where π/2 < A < π and 0 < B < π/2
As we know that, A is in second quadrant, B is in first quadrant.
So, all functions are positive in first quadrant and sine function is positive,
cosine and tan functions are negative in the second quadrant.
So, by using the following formulas,
cosA = – √(1 – sin2A) and sinB = √(1 – cos2B)
We find the value of cosA and sinB
cosA = – √(1 – sin2A)
= – √(1 – (1/2)2)
= – √(1 – 1/4)
= – √((4 – 1)/4)
= – √(3/4)
= – √3/2
sinB = √(1 – cos2B)
= √(1 – (√3/2)2)
= √(1 – 3/4)
= √((4 – 3)/4)
= √(1/4)
= 1/2
As we know that, tanA = sinA / cosA and tanB = sinB / cosB
So, tanA = (1/2)/(-√3/2) = -1/√3 and
tanB = (1/2)/(√3/2) = 1/√3
(i) Since, tan(A + B) = (tanA + tanB)/(1 – tanA tanB), so
= (-1/√3 + 1/√3)/(1 – (-1/√3) × 1/√3)
= 0/(1 + 1/3)
= 0
Hence, tan(A + B) = 0
(ii) Since, tan(A – B) = (tanA – tanB)/(1 + tanA tanB), so
= ((-1/√3) – (1/√3))/(1 + (-1/√3) x (1/√3))
= ((-2/√3)/(1 – 1/3)
= ((-2/√3)/(3 – 1)/3)
= ((-2/√3)/2/3)
= -√3
Hence, tan(A – B) = -√3
Question 7. Evaluate the following:
(i) sin 78° cos 18⁰- cos 78° sin 18⁰
(ii) cos 47° cos 13⁰ – sin 47⁰ sin 13⁰
(iii) sin 36° cos 9⁰+ cos 36° sin 9⁰
(iv) cos 80° cos 20⁰+ sin 80° sin 20⁰
Solution:
(i) sin 78° cos 18° – cos 78° sin 18°
Since, sinAcosB – cosAsinB = sin(A – B)
So
sin 78° cos 18° – cos 78° sin 18° = sin(78 – 18)°
= sin 60°
= √3/2
(ii) cos 47° cos 13° – sin 47° sin 13°
Since, cosA cosB – sinA sinB = cos(A + B)
So, cos 47° cos 13° – sin 47° sin 13° = cos (47 + 13)°
= cos 60°
= 1/2
(iii) sin 36° cos 9° + cos 36° sin 9°
Since, sin A cos B + cos A sin B = sin (A + B)
So, sin 36° cos 9° + cos 36° sin 9° = sin (36 + 9)°
= sin 45°
= 1/√2
(iv) cos 80° cos 20° + sin 80° sin 20⁰
Since, cos A cos B + sin A sin B = cos (A – B)
So, cos 80° cos 20° + sin 80° sin 20° = cos (80 – 20)°
= cos 60°
= 1/2
Question 8. If cosA = -12/13 and cotB = 24/7, where A lies in the second quadrant and B in the third quadrant, find the values of the following:
(i) sin(A + B)
(ii) cos(A + B)
(iii) tan(A + B)
Solution:
We have,
cosA = -12/13 and cotB= 24/7
It is given that, A lies in second quadrant, B in the third quadrant.
So, sine function is positive in second quadrant and both sine and cosine
functions are negative in third quadrant.
So, by using the following formulas,
sinA = √(1 – cos2A), sinB = 1/√(1 + cot2B) and cosB = -√(1 – sin2B),
We find the value of sinA and sinB
sinA = √(1 – cos2A)
= √(1 -(-12/13)2)
= √(1 – 144/169)
= √((169 – 144)/169)
= √(25/169)
= 5/13
sinB = -1/√(1 + cot2B)
= -1/√(1 + (24/7)2)
= -1/√(1 + 576/49)
= -1/√((49 + 576)/49)
= -1/√(625/49)
= -1/(25/7)
= -7/25
cosB = -√(1 – sin2B)
= -√(1 -(-7/25)2)
= -√(1 – (49/625))
= -√((625 – 49)/625)
= -√(576/625)
= -24/25
(i) sin(A + B)
Since, sin(A + B) = sinA cosB + cosA sinB
So,
= 5/13 x (-24/25) + (-12/13) x (-7/25)
= -120/325 + 84/325
= -36/325
(ii) cos(A + B)
Since, cos(A + B) = cosA cosB – sinA sinB
So,
= -12/13 x (-24/25) – (5/13) x (-7/25)
= 288/325 + 35/325
= 323/325
(iii) tan(A + B)
Since, tan(A + B) = sin(A + B) / cos(A + B)
So,
= (-36/325)/(323/325)
=-36/323
Question 9. Prove that: cos 7π/12 + cos π/12 = sin 5π/12 – sin π/12
Solution:
As we know that,
7π/12 = 105°, π/12 = 15°, 5π/12 = 75°
Now, L.H.S = cos 105° + cos 15°
= cos (90° + 15°) + sin (90° – 75°)
= -sin 15° + sin 75°
= sin 75° – sin 15°
= RHS
So, LHS = RHS
Hence proved.
Question 10. Prove that: (tanA + tanB) / (tanA – tanB) = sin(A + B) / sin(A – B)
Solution:
Let solve, LHS = (tanA + tanB) / (tanA – tanB)
= 
= 
As we know that,
sin(A ± B) = sinA cosB ± cosA sinB
So,
= {sin(A + B)} / {sin(A – B)}
= RHS
So, LHS = RHS
Hence proved.
Question 11. Prove that:
(i) (cos 11° + sin 11°)/(cos 11° – sin 11°) = tan 56°
(ii) (cos 9⁰+ sin 9″) / (cos 9″- sin 9°) = tan 54°
(iii) (cos 8° – sin 8°) / (cos 8° + sin 8°) = tan 37⁰
Solution:
(i) (cos 11° + sin 11°) / (cos 11° – sin 11°) = tan 56°
Let solve, LHS = (cos 11° + sin 11°)/(cos 11° – sin 11°)
Now divide the numerator and denominator by cos 11° we get,
(cos 11° + sin 11°)/(cos 11° – sin 11°) = (1 + tan 11°)/(1 – tan 11°)
= (1+ tan 11°)/(1 – 1 x tan 11°)
= (tan 45° + tan 11°)/(1 – tan 45° x tan 11°)
As we know that,
tan(A + B) = (tanA + tanB)/(1 – tanA tanB)
So,
(tan 45° + tan 11°)/(1 – tan 45° x tan 11°) = tan (45° + 11°)
= tan 56°
= RHS
So, LHS = RHS
Hence proved.
(ii) (cos 9° + sin 9°)/(cos 9° – sin 9°) = tan 54°
Let solve, LHS = (cos 9° + sin 9°)/(cos 9° – sin 9°)
Now divide the numerator and denominator by cos 9° we get,
(cos 9° + sin 9°)/(cos 9° – sin 9°) = (1 + tan 9°)/(1 – tan 9°)
= (1 + tan 9°) / (1 – 1 x tan 9°)
= (tan 45° + tan 9°)/(1 – tan 45° x tan 9°)
As we know that
tan(A + B) = (tanA + tanB)/(1 – tanA tanB)
So,
(tan 45° + tan 9°)/(1 – tan 45° x tan 9°) = tan (45° + 9°)
= tan 54°
= RHS
So, LHS = RHS
Hence proved.
(iii) (cos 8° – sin 8°)/(cos 8° + sin 8°) = tan 37⁰
Let solve, LHS = (cos 8° – sin 8°) / (cos 8° + sin 8°)
Now divide the numerator and denominator by cos 8° we get,
(cos 8° – sin 8°) / (cos 8° + sin 8°) = (1 – tan 8°)/(1 + tan 8°)
= (1 – tan 8°)/(1 + 1 x tan 8°)
= (tan 45° – tan 8°) / (1 + tan 45° x tan 8″)
As we know that
tan(A + B) = (tanA + tanB)/(1 – tanA tanB)
So,
(tan 45° – tan 8°)/(1 + tan 45° x tan 8°) = tan (45° – 8°)
= tan 37°
= RHS
LHS = RHS
Hence proved.
Question 12. Prove that:
(i) sin (π/3 – x) cos(π/6 + x) + cos (π/3 – x) sin(π/6 + x) = 1
(ii) sin (4π/9 + 7) cos(π/9 + 7) – cos (4π/9 + 7) sin (π/9 + 7) = √3/2
(iii) sin ( 3π/8 – 5) cos (π/8 + 5) + cos (3π/8 – 5) sin(π/8 + 5) = 1
Solution:
(i) sin (π/3 – x) cos(π/6 + x) + cos (π/3 – x) sin(π/6 + x) = 1
Let solve, LHS = sin (π/3 – x) cos(π/6 + x) + cos (π/3 – x) sin(π/6 + x)
As we know that
sin(A + B) = sinA cosB + cosA sinB
sin (π/3 – x) cos(π/6 + x) + cos (π/3 – x) sin(π/6 + x) = sin (π/3 – x + π/6 + x)
= sin ((2π + π)/6)
= sin (π/2)
= sin 90°
= 1
= RHS
LHS = RHS
Hence proved.
(ii) sin (4π/9 + 7) cos(π/9 + 7) – cos (4π/9 + 7) sin (π/9 + 7) = √3/2
Let solve, LHS = sin (4π/9 + 7) cos(π/9 + 7) – cos (4π/9 + 7) sin (π/9 + 7)
As we know that
sin(A – B) = sinA cosB – cosA sinB
sin (4π/9 + 7) cos(π/9 + 7) – cos (4π/9 + 7) sin (π/9 + 7) = sin (4π/9 + 7 – π/9 – 7)
= sin (3π/9)
= sin (π/3)
= sin 60°
= √3/2
= RHS
LHS = RHS
Hence proved.
(iii) sin ( 3π/8 – 5) cos (π/8 + 5) + cos (3π/8 – 5) sin(π/8 + 5) = 1
Let solve, LHS = sin ( 3π/8 – 5) cos (π/8 + 5) + cos (3π/8 – 5) sin(π/8 + 5)
As we know that
sin(A + B) = sinA cosB + cosA sinB
sin ( 3π/8 – 5) cos (π/8 + 5) + cos (3π/8 – 5) sin(π/8 + 5) = sin (3π/8 – 5 + π/8 + 5)
= sin ((3π + π)/8)
= sin (4π/8)
= sin (π/2)
= sin 90°
= 1
= RHS
LHS = RHS
Hence proved.
Question 13. Prove that: (tan 69° + tan 66°)/(1 – tan 69° tan 66°) = -1
Solution:
Let solve, LHS = (tan 69°+tan 66°)/(1-tan 69° tan 66°)
As we know that
tan(A + B) = (tanA + tanB) / (1 – tanA tanB)
= (tan 69° + tan 66°)/(1 – tan 69° tan 66°)
= tan (69 +66)°
= tan 135⁰
= – tan 45º
= -1
= RHS
LHS = RHS
Hence proved.
Question 14. (i) If tanA = 5/6 and tanB = 1/11, prove that (A + B) = π/4
(ii) If tanA = m/(m-1) and tanB = 1/(2m – 1), then prove that (A – B) = π/4
Solution:
(i) We have,
tanA = 5/6 and tanB = 1/11
As we know that
tan(A + B) = (tanA + tanB)/(1 – tanA tanB)
= [(5/6) + (1/11)] /[1 – (5/6) x (1/11)]
= (55 + 6)/(66 – 5)
= 61/61
= 1
= tan 45° or tan π/4
So, tan(A + B) = tan π/4
(A + B) = π/4
Hence proved.
(ii) We have,
tanA = m/(m – 1) and tanB = 1/(2m – 1)
As we know that
tan(A – B) = (tanA – tanB) / (1 + tanA tanB)
= 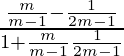
= (2m2 – m – m + 1)/(2m2 – m – 2m + 1 + m)
= (2m2 – 2m + 1)/(2m2 – 2m + 1)
= 1
= tan 45° or tan π/4
So, tan(A – B) = tan π/4
(A – B) = π/4
Hence proved.
Question 15. prove that:
(i) cos2 π/4 – sin2 π/12 = √3/4
(ii) sin2 (n + 1)A – sin2nA = sin (2n + 1)A sin A
Solution:
(i) cos2 π/4 – sin2 π/12 = √3/4
Let solve, LHS = cos2 π/4 sin2 π/12
As we know that
cos2 A – sin2 B = cos (A + B) cos (A – B)
So,
cos2 π/4 – sin2 π/12 = cos (π/4 + π/12) cos (π/4 – π/12)
= cos 4π/12 cos 2π/12
= cos π/3 cos π/6
= 1/2 x √3/2
= √3/4 = RHS
LHS = RHS
Hence proved.
(ii) sin2 (n + 1)A – sin2nA = sin (2n + 1)A sinA
Let solve, LHS = sin2 (n+1)A – sin2nA
As we know that
sin2A – sin2B = sin(A + B) sin(A – B)
Where, A = (n + 1)A and B = nA
So,
sin2(n + 1)A – sin2nA = sin((n + 1)A + nA) sin((n + 1)A – nA)
= sin(nA + A + nA) sin (nA + A – nA)
= sin(2nA + A) sinA
= sin(2n + 1)A sinA
= RHS
LHS = RHS
Hence proved.
Question 16. Prove that:
(i) {sin(A + B) + sin(A – B)}/{cos(A + B) + cos(A – B)} = tanA
Solution:
Prove: {sin(A + B) + sin(A – B)}/{cos(A + B) + cos(A – B)} = tanA
Proof:
Let solve, LHS = {sin(A + B) + sin(A – B)}/{cos(A + B) + cos(A – B)}
As we know that, sin (A ± B) = sinA cosB ± cosA sinB and cos(A ± B) = cosA cosB ± sinA sinB
So,
= 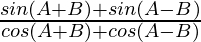
= 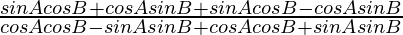
= (2cosA cosB)(2cosA cosB)
= tan A
= RHS
LHS = RHS
Hence proved.
(ii) {sin(A – B)/cosA.cosB} + {sin(B – C)/cosB.cosC} + {sin(C – A)/cosC.cosA} = 0
Solution:
Prove: {sin(A – B)/cosA.cosB} + {sin(B – C)/cosB.cosC} + {sin(C – A)/cosC.cosA} = 0
Proof:
Let solve, LHS = {sin(A – B)/cosA.cosB} + {sin(B – C)/cosB.cosC} + {sin(C – A)/cosC.cosA}
As we know that
sin(A – B) = sinA cosB – cosA sinB
So,
= {sin(A – B)/cosA.cosB} + {sin(B – C)/cosB.cosC} + {sin(C – A)/cosC.cosA}
= (sinA cosB – cosA sinB)/(cosA cosB) + (sinB cosC – cosB sinC)/(cosB cosC) +
(sinC cosA – cosC sinA)/(cosC cosA)
= (sinA cosB)/(cosA sinB) – (cosA sinB)/(cosB cosC) + (sinB cosC)/(cosB cosC) –
(cosB sinC)/(cosB cosC) + (sinC cosA)/(cosC cosA)
= tanA – tanB + tanB – tanC + tanC – tanA
= 0
= RHS
LHS = RHS
Hence proved.
(iii) {sin(A – B)/sinA.sinB} + {sin(B – C)/sinB.sinC} + {sin(C – A)/sinC.sinA} = 0
Solution:
Prove: {sin(A – B)/sinA.sinB} + {sin(B – C)/sinB.sinC} + {sin(C – A)/sinC.sinA} = 0
Proof:
Let solve, LHS = {sin(A – B)/sinA.sinB} + {sin(B – C)/sinB.sinC} + {sin(C – A)/sinC.sinA}
As we know that
sin(A – B) = sinA cosB – cosA sinB
= {sin(A – B)/sinA.sinB} + {sin(B – C)/sinB.sinC} + {sin(C – A)/sinC.sinA}
= (sinA cosB – cosA sinB)/(sinA sinB) + (sinB cosC – cosB sinC)/(sinB sinC) +
(sinC cosA- cosC sinA)/(sinC sinA)
= (sinA.cosB)/(sinA.sinB) – (cosA.sinB)/(sinA.sinB) + (sinB.cosC)/(sinB.sinC) –
(cosB.sinC)/(sinB.sinC) +(sinC.cosA)/(sinC.sinA) – (cosC.sinA)/(sinC.sinA)
= cotB – cotA + cotC – cotB + cotA – cot C
= 0
= RHS
LHS = RHS
Hence proved.
(iv) sin2B = sin2A + sin2(A – B) – 2sinA cosB sin(A – B)
Solution:
Prove: sin2B = sin2A + sin2(A – B) – 2sinA cosB sin(A – B)
Proof:
Let’s solve RHS = sin2A + sin2(A – B) – 2 sinA cosB sin(A – B)
= sin2A+ sin(A -B)[sin(A – B) – 2 sinA cosB]
As we know that
sin(A – B) = sinA cosB – cosA sinB
So,
= sin2A + sin(A -B)[sinA cosB – cosA sinB – 2 sinA cosB]
= sin2A + sin(A-B)[-sinA cosB – cosA sinB]
= sin2A – sin(A -B)[sinA cosB + cosA sinB]
As we know that
sin (A +B) = sin A cos B + cos A sin B
So,
= sin2A – sin(A – B) sin(A + B).
= sin2A – sin2A + sin2B
= sin2B
= LHS
LHS = RHS
Hence proved.
(v) cos2A + cos2B – 2 cosA cosB cos(A + B) = sin2(A + B)
Solution:
Prove: cos2A + cos2B – 2 cosA cosB cos(A + B) = sin2(A + B)
Proof:
Let solve LHS = cos2A + cos2B – 2 cosA cosB cos(A + B)
= cos2A + 1 – sin2B – 2 cosA cosB cos(A + B)
= 1 + cos2A – sin2B – 2 cosA cosB cos(A + B)
As we know that cos2A – sin2B = cos(A + B) cos(A – B)
So,
= 1 + cos(A + B) cos(A – B) – 2 cosA cosB cos(A + B)
= 1 + cos(A + B)[cos(A – B) – 2 cosA cosB]
Also,
cos(A – B) = cosA cosB + sinA sinB
So,
= 1 + cos(A +B)[cosA cosB + sinA sinB – 2 cosA cosB]
= 1 + cos(A +B)[-cosA cosB + sinA sinB]
= 1 cos(A +B)[cosA cosB – sinA sinB]
Also,
cos(A + B) = cosA cosB – sinA sinB
So,
1 – cos2(A + B) = sin2(A + B) = RHS
LHS = RHS
Hence proved.
(vi) tan(A + B)/cot(A – B) = (tan2A – tan2B)/(1 – tan2A tan2B)
Solution:
Prove: tan(A + B)/cot(A – B) = (tan2A – tan2B)/(1 – tan2A tan2B)
Proof:
Let solve LHS = tan(A + B)/cot(A – B)
As we know that
tan (A ± B) = (tanA ± tanB) / (1 ± tanA tanB)
So,
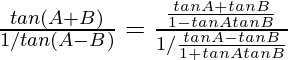
= 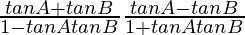
We know that, (x+y) (x – y) = x2– y2
So,
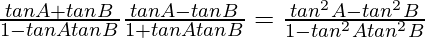
= RHS
LHS = RHS
Hence proved.
Share your thoughts in the comments
Please Login to comment...