Question 1. (i) Find the point of intersection of the lines:
2x – y + 3 = 0 and x + y – 5 = 0
Solution:
Given equation of lines are:
2x – y + 3 = 0 …..(1)
x + y – 5 = 0 ……(2)
2x – y + 3 = 0
⇒ y = 2x + 3
Now put this value in equation(2), we get
x + y – 5 = 0
x + (2x + 3) – 5 = 0
x + 2x + 3 – 5 = 0
3x – 2 = 0
x = 2/3
Now put the value of x in equation(1), we get
y = 2x + 3 = (2 × 2)/3 + 3 = 4/3 + 3 = 13/2
Hence, the points of intersection is (2/3, 13/3)
Question 1(ii). Find the point of intersection of the pairs of lines:
bx + ay = ab and ax + by = ab
Solution:
Given equation of lines are:
bx + ay = ab …..(1)
ax + by = ab …..(2)
bx + ay = ab ⇒ 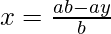
Now put this value in equation(2), we get
ax + by = ab
a((ab – ay)/(b)) + by = ab
a2b – a2y + b2y = ab2
y(b2 – a2) = ab(b – a)
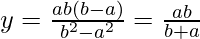
Now put the value of x in equation(1), we get
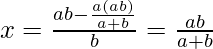
Hence, the points of intersection is (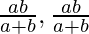 )
)
(iii) Find the point of intersection of the pairs of lines:
y = m1x + a/m1 and y = m2x + a/m2
Solution:
Given equation of lines are:
y = m1x + a/m1 …..(1)
y = m2x + a/m2 …..(2)
From equation (1) and (2)
m1x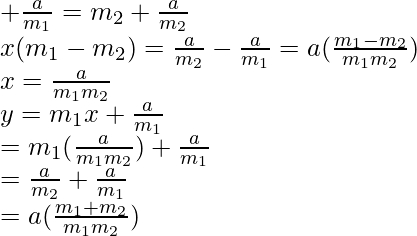
Hence, the point of intersection is 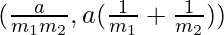
Question 2 (i). Find the coordinates of the vertices of a triangle, the equations of whose sides are x + y – 4 = 0, 2x – y + 3 = 0 and x – 3y + 2 = 0.
Solution:
Given equations:
x + y – 4 = 0 …..(1)
2x – y + 3 = 0 …..(2)
x – 3y + 2 = 0 …..(3)
On solving eq(1) and (2)
2x – (4 – x) + 3 = 0
2x – 4 + x + 3 = 0
3x – 1 = 0
x = 1/3
Now Put the value of x in eq(1), we get
1/3 + y – 4 = 0
y = 4 – 1/3 = 11/3
So, the first vertex is (1/3, 11/3)
On solving eq(2) and (3), and
Put y = 2x + 3 in eq(3)
x – 3(2x + 3) + 2 = 0
x – 6y – 9 + 2 = 0
-5x = 7
x = -7/5
y = 2x + 3 = 2(-7/5) + 3 = -14/5 + 3 = 1/5
So, the second vertex is (-7/5, 1/5)
Now we find the next vertex
x + y – 4 = 0
x – 3y + 2 = 0
x = 4 – y
4 – y – 3y + 2 = 0
4 – 4y + 2 = 0
-4y = -6
y = 3/2
x = 4 – y
4 – 3/2
8 – 3/2 = 5/2
Hence, the third vertex is (5/2, 3/2)
Question 2 (ii). Find the coordinates of the vertices if a triangle, the equation of whose sides are y(t1 + t2) = 2x + 2at1t2, y(t2 + t3) = 3x + 2at2t3 and y(t3 + t1) = 2x + 2a + t1t3
Solution:
Given equations:
y(t1 + t2) = 2x + 2at1t2 …..(1)
y(t2 + t3) = 2x + 2at2t3 …..(2)
y(t3 + t1) = 2x + 2at1t3 …..(3)
On solving eq (1) and (2), we get
(x1, y1) = (at22, 2at2)
On solving eq (2) and (3), we get
(x2, y2) = (at32, 2at3)
On solving eq(1) and (3), we get
(x3, y3) = (at12, 2at1)
Hence, the vertices of the triangle are (at22, 2at2), (at32, 2at3), and (at12, 2at1).
Question 3 (i). Find the area of the triangle formed by the lines
y = m1 + c1, y = m2 + c2, x = 0
Solution:
Given equations
y = m1x + c1 …..(1)
y = m2x + c2 …..(2)
x = 0 …..(3)
On solving eq (1) and (2), we get
(x1, y1) = (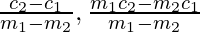 )
)
On solving eq (2) and (3), we get
(x2, y2) = (0, c2)
On solving eq (1) and (3), we get
(x3, y3) = (0, c1)
Area of triangle formed by above vertices is
![Rendered by QuickLaTeX.com =\frac{1}{2}[(\frac{c_2-c_1}{m_1-m_2} \times c_1)-(\frac{c_2-c_1}{m_1-m_2}\times c_2)]\\ =\frac{(c_2-c_1)^2}{2(m_1-m_2)}](https://www.geeksforgeeks.org/wp-content/ql-cache/quicklatex.com-9c63d5b1f5c4a6c3365530c10abce475_l3.png) sq. units
sq. units
Question 3 (ii). Find the area of the triangle formed by the lines
y = 0, x = 2, and x + 2y = 3
Solution:
Given equations
y = 0 …..(1)
x = 2 …..(2)
x + 2y = 3 …..(3)
On solving eq (1) and (2), we get
Point (x1, y1) = (2, 0)
On solving eq (2) and (3), we get
2 + 2y = 3
y = 1/2
y = 2
Point (x2, y2) = (2, 1/2)
On solving eq (1) and (3), we get
Point (x3, y3) = (3, 0)
As we know that the area of triangle is (A) = 1/2[x1(y2 – y3) + x2(y3 – y1) + x3(y1 – y2)]
A = 1/2[2(1/2 – 0) + 2(0 – 0) + 3( 0 – 1/2)]
A = 1/2[|1 – 3/2|]
A = 1/4 sq. units
Question 3 (iii). Find the area of the triangle formed by the lines
x + y – 6 = 0, x – 3y – 2 = 0 and 5x – 3y + 2 = 0
Solution:
Given equations
x + y – 6 = 0 …..(1)
x – 3y – 2 = 0 …..(2)
5x – 3y + 2 = 0 …..(3)
On solving eq (1) and (2), we get
(x1, y1) = (5, 1)
On solving eq (2) and (3), we get
(x2, y2) = (-1, -1)
On solving eq (3) and (1), we get
(x3, y3) = (2, 4)
As we know that the area of triangle is (A) = 1/2[x1(y2 – y3) + x2(y3 – y1) + x3(y1 – y2)]
A = 1/2[|-25 – 3 + 4|]
A = 12 sq. units
Question 4. Find the equation of the medians of a triangle, the equation of whose sides are:
3x + 2y + 6 = 0, 2x – 5y + 4 = 0, x – 3y – 6 = 0
Solution:
Let us considered ABC is a triangle, in which A, B, and C are the vertices of the triangle
So, according to the question the equations are:
3x + 2y + 6 = 0 …..(1)
2x – 5y + 4 = 0 …..(2)
x – 3y – 6 = 0 …..(3)
On solving eq (1) and (2), we get
x = -2 and y = 0
So the coordinates of A is (-2, 0)
On solving eq (3) and (2), we get
x = -42 and y = -16
So the coordinates of B is (-42, -16)
On solving eq (1) and (3), we get
x = -6/11 and y = -24/11
So the coordinates of C is (-6/11, -24/11)
Now let us assume that P, Q, and R be the mid-point of side AB, BC and AC
So, the coordinates of the mid-points are:
P = ((-2 -42)/2, (0 – 16)/2) = (-22, -8)
Q = ((-42 – 6/11)/2, (-16 – 24/11)/2) = (-234/11, -100/11)
R = ((-2 -6/11)/2, (0 – 24/11)/2) = (-14/11, -12/11)
Now we find the median of AP is
= y + 8/x + 22 = 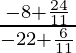
= y + 8/x + 22 = -88 + 24/-242 + 6 = 16/59
= 16x – 59y + 352 – 572 = 0
= 16x – 59y – 120 = 0
Similarly, the median of BQ is 25x – 53y + 50 = 0
And the median of CR is 41x – 112y – 70 = 0
Question 5. Prove that lines y = √3x + 1, y = 4, and y = -√3x + 2 form an equilateral triangle.
Solution:
Let us considered ABC is a triangle in which A, B, and C are the vertices of the triangle
The equation of lines are:
y = √3x + 1 …..(1)
y = 4 …..(2)
y = -√3x + 2 …..(3)
On solving eq (1) and (2), we get
4 = √3x + 1
x = 4 – 1/√3 = 3/√3 = √3
So, the coordinates of point A is (√3, 4)
On solving eq (2) and (3), we get
4 = -√3x + 2
√3x = -2
x = -2/√3
x = -2√3/3
So, the coordinates of point B is (-2√3/3, 4)
On solving eq (1) and (3), we get
√3x + 1 = -√3x + 2
2√3x = 1
x = 1/2√3 = √3/6
y = √3(√3/6 + 1) = 3/2
So, the coordinates of point C is(√3/6, 3/2)
Now we have,
AB = 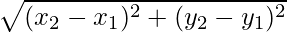

= 5√3/3 units
BC 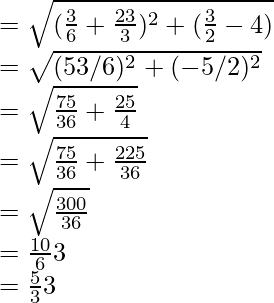
AC 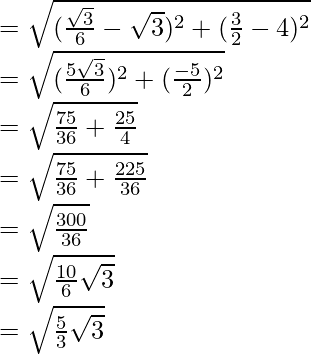
Here, AB = BC = AC
Hence, ABC triangle is equilateral
Question 6 (i). Classify 2x + y – 1 = 0 and 3x + 2y + 5 = 0 as coincident, parallel or intersecting.
Solution:
The equations of lines are:
2x + y – 1 = 0
3x + 2y + 5 = 0
Now on writing above equations in the form y = mx + c
y = -2x + 1,
y = -3x/2 – 5/2
So, the slope of both the equations are
m1 = -2 and m2 = -3/2
Now
m1 ≠ m2 and m1m2 ≠ -1
Hence, the lines are intersecting
Question 6(ii). Classify x – y = 0 and 3x – 3y + 5 = 0 as coincident, parallel or intersecting.
Solution:
The equations of lines are:
x – y = 0
3x – 3y + 5 = 0
Now on writing above equations in the form y = mx + c
y = x
y = x + 5/3
So, the slope of both the equations are
m1 = 1 and m2 = 1
Slopes of both lines are equal
Hence, the lines are parallel
Question 6 (iii). Classify 3x + 2y – 4 = 0 and 6x + 4y – 8 = 0 as coincident, parallel or intersecting.
Solution:
The equations of lines are:
3x + 2y – 4 = 0
6x + 4y – 8 = 0
Now on writing above equations in the form y = mx + c
y = -3x/2 + 2
y = -3x/2 + 2
So, the slope of both the equations are
m1 = -3/2 and m2 = -3/2
So, m1 = m2 = -3/2
Hence, the lines are coincident
Question 7. Find the equation of the joining the point (3, 5) to the point of intersection of the lines 4x + y – 1 = 0 and 7x – 3y – 35 = 0.
Solution:
Given equation of lines are
4x + y – 1 = 0 …..(1)
7x – 3y – 35 = 0 …..(2)
From eq(1), we get
y = 1 – 4x
Now put the value of y in eq(2), we get
7x – 3(1 – 4x) – 35 = 0
7x – 3 + 12x – 35 = 0
19x = 38
x = 2
y = 1 – 4x = 1 – 8 = -7
Now we have to find the equation of line the joining the point P(2, -7) and Q(3, 5)
The equation of line PQ is
y – y1 = m(x – x1)
y – y1 = 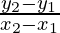 (x – x1)
(x – x1)
y – (-7) = 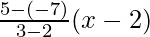
y + 7 = 12(x – 2)
y – 12x = -31
12x – y – 31 = 0
Hence, the equation of line is 12x – y – 31 = 0
Question 8. Find the equation of the line passing through the point of intersection of the lines 4x – 7y – 3 = 0 and 3x – 3y + 1 = 0 that has equal intercepts on the axes.
Solution:
Given equation of lines are
4x – 7y = 3
2x – 3y = -1
On solving the above equations, we get the point of intersection,
x = -B, y = -5
Now, the point of intersection of given lines is (-8, -5) equation
of line making equal intercepts (a) on the coordinates axes is,
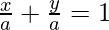
x + y = a
-8 – 5 = a
a = -13
Hence, the equation of line is x + y = -13
Question 9. Show that area of the triangle formed by the lines y = m1x, y = m2x, and y = c is equal to c2/4(√33 + √11), where m1, m2 are the roots of the equation x2 + (√3 + 2)x + √3 – 1 = 0.
Solution:
Given equation of lines are
y = m1x …..(1)
y = m2x …..(2)
y = c …..(3)
On solving eq (1) and (2), we get A(0, 0)
On solving eq (1) and (3), we get A(c/m1, c)
On solving eq (2) and (3), we get A(c/m2, c)
Now we find the area of triangle when three vertices are given is
= 1/2(x2(y2 – y3) + x2(y3 – y1) + x3(y1 – y2))
![Rendered by QuickLaTeX.com =\frac{1}{2}[|\frac{c^2}{m_1}-\frac{c^2}{m_2}|]=\frac{c^2}{2}[|\frac{m_2-m_1}{m_1m_2}|]](https://www.geeksforgeeks.org/wp-content/ql-cache/quicklatex.com-d6543ebb859259d99e170d0d65a6c7e2_l3.png)
Given m1 and m2 are roots of x2 + (√3 + 2)x + √3 – 1 = 0
Product of roots = m1m2 = √3 – 1
|m2-m1|=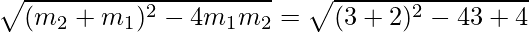
|m2-m1|=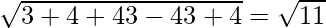
Area = ![Rendered by QuickLaTeX.com \frac{c^2}{2}[\frac{√11}{√3-1}]](https://www.geeksforgeeks.org/wp-content/ql-cache/quicklatex.com-105b73f3a69f09a561fd1e0d8041556d_l3.png)
On rationalizing denominator, we get
![Rendered by QuickLaTeX.com \frac{c^2}{4}[√33+√11]](https://www.geeksforgeeks.org/wp-content/ql-cache/quicklatex.com-f985a63f1a3230a9d6c580fd736f86cf_l3.png)
Hence proved
Question 10.If the straight line 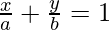 passes through the point of intersection of the lines x + y = 3 and 2x – 3y = 1 and is parallel to x – y – 6 = 0, find a and b.
passes through the point of intersection of the lines x + y = 3 and 2x – 3y = 1 and is parallel to x – y – 6 = 0, find a and b.
Solution:
Given equation of lines are
x + y = 3
2x – 3y = 1
On solving both the equations we get the intersection point (2, 1)
It is given that the line x/a + y/b = 1 is parallel to line x – y – 6 = 0
so, the slope = 1
Now we find the equation of line passing through (2, 1) and having slope = 1 is
y – y1 = m(x – x1)
y – 1 = 1(x – 2)
y – 1 = x – 2
y – x = -2 + 1
y – x = -1
x – y = 1
On comparing with x/a + y/b = 1, we get
a = 1, b = -1
Share your thoughts in the comments
Please Login to comment...