Class 11 RD Sharma Solutions – Chapter 1 Sets – Exercise 1.4 | Set 2
Last Updated :
11 Feb, 2021
Question 9. Write down all subsets of the following subsets:
(i) {a}
(ii) {0,1}
(iii){a,b,c}
(iv){1,{1}}
(v) {ϕ}
Solution:
We know, if A is a set and B is a subset of A, then B is a subset of A, then B is called a proper subset of A if B ⊆ A and B≠A, ϕ, illustrated by B ⊆ A or B ⊂ A.
For any set S with n elements, the power set has 2n elements.
(i) Since n=1, the power set has 21 = 2 elements.The subsets are {a} and ϕ, but the set has no proper subsets.
(ii) Since n=2, the power set has 22 = 4 elements. The elements of the power subset are ϕ, {0}, {1}, {0,1}.
(iii)Since n=3, the power set has 23 = 8 elements.The elements of the power subset are ϕ, {a}, {b}, {c}, {a,b}, {b,c}, {a,c}, {a,b,c}.
(iv) Since n=2, the power set has 22 = 4 elements. The elements of the power subset are ϕ, {1}, {{1}}, {1,{1}}.
(v)Since n=1, the power set has 21 = 2 elements, which are ϕ and {ϕ}.
Question 10. Write down all proper subsets of the following subsets:
(i) {1,2}
(ii) {1,2,3}
(iii){1}
Solution:
We know, if A is a set and B is a subset of A, then B is a subset of A, then B is called a proper subset of A if B ⊆ A and B≠A, ϕ, illustrated by B ⊆ A or B ⊂ A.
(i) The proper subsets are {1}, {2}
(ii) The proper subsets are {1}, {2}, {3}, {1,3}, {2,3}, {1,2}
(iii) The subsets are {1} and ϕ, but the set has no proper subsets.
Question 11. What is the total number of proper subsets of a set consisting of n elements?
Solution
We know that, for any finite set A, having n elements, the total number of subsets of A has 2n elements. However, the number of proper subsets is 2n – 1, where A is not included.
Question 12. If A is any set, prove that A ⊆ ∅ <=> A = ∅
Solution:
In order to prove that two sets A and B are equal, we need to show the following:
A ⊆ B and B ⊆ A.
Since, ∅ is a subset of every set, therefore, A ⊆ ∅.
Therefore, ∅ ⊆ A.
Hence, A = ∅
Let us assume now, that A =∅
Now, every set is a subset of itself,
∅ = A ⊆ ∅
Hence, proved.
Question 13. Prove that A ⊆ B, B ⊆ C, and C ⊆ A => A = C
Solution:
We have, A ⊆ B, B ⊆ C and C ⊆ A, therefore, A ⊆ B ⊆ C ⊆ A.
Now, A is a subset of B and B is a subset of C,
So A is a subset of C, that is A ⊆ C.
Also, it is given, C ⊆ A.
We know, if A ⊆ C and C ⊆ A => A = C.
Hence, proved.
Question 14. How many elements has P(A)=?, if A = ∅.
Solution:
An empty set has zero elements.
Therefore, size of A = n = 0.
Power set of A P(A) = 2n = 20 = 1 element.
Question 15. What universal set(s) would you propose for the following?
(i) The set of right triangles.
(ii) The set of isosceles triangles.
Solution:
(i) The right triangle is a subset of the triangles. Therefore, the set of right triangles is a subset of set of triangles.
=> Set of right triangles ⊆ Set of triangles, which becomes Universal set (U) in this case.
(ii) The isosceles triangle is a special case of the triangles where two sides are equal. Therefore, the set of isosceles triangles is a subset of set of triangles.
=> Set of isosceles triangles ⊆ Set of triangles, which becomes Universal set (U) in this case.
Question 16. If X= {8n – 7n -1 : n ∈ N} and Y = {49(n-1): n ∈ N}. Prove that X ⊆ Y.
Solution:
To prove X ⊆ Y, we need to show that each element of X belongs to Y.
We have,
X= {8n – 7n -1 : n ∈ N}
Y = {49(n-1): n ∈ N}
So, let x ∈ X => x = 8m– 7m – 1 for some m ∈ N
=> x = (1 + 7 )m – 7m – 1
= {n \choose x}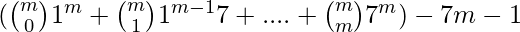
= 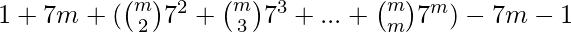
=({m \choose 0}1^m + {m \choose 1}1^{m-1} 7 +….+ {m \choose m}7^{m}) – 7m -1 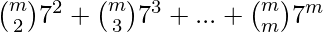
=49({m \choose 2}7^2 + {m \choose 3}7^{3} +…+ {m \choose m}7^{m-2}), m >=2
= 49tm, m >=2 where tm = {m \choose 2}7^2 + {m \choose 3}7^{3} +…+ {m \choose m}7^{m-2}
For m = 1, we have,
X = 8 – 7 x 1 – 1
= 0
Hence, X contains all positive integral multiples of 49.
Hence, Y contains all positive integral multiples of 49 and 0, for n =1.
Therefore,
X ⊆ Y.
Share your thoughts in the comments
Please Login to comment...