Class 11 RD Sharma Solutions – Chapter 20 Geometric Progressions- Exercise 20.3 | Set 1

Question 1. Find the sum of the following geometric progressions:

(i) 2, 6, 18, … to 7 terms

Solution:

Given G.P. has first term(a) = 2, common ratio(r) = 6/2 = 3 and number of terms(n) = 7

We know sum of n terms of a GP is given by S_n = a(rⁿ–1)/(r–1).

S₇ = 2(3⁷–1)/(3–1) 

= 2(3⁷–1)/2

= 2187–1

= 2186

Therefore, sum of 7 terms of the G.P. is 2186.

(ii) 1, 3, 9, 27, … to 8 terms

Solution:

Given G.P. has first term(a) = 1, common ratio(r) = 3 and number of terms(n) = 8

We know sum of n terms of a GP is given by S_n = a(rⁿ–1)/(r–1).

S₈ = 1(3⁸–1)/(3–1)

= 6560/2

= 3280

Therefore, sum of 8 terms of the G.P. is 3280.

(iii) 1, –1/2, 1/4, –1/8, ….

Solution:

Given G.P. has first term(a) = 1, common ratio(r) = –1/2 and number of terms(n) is infinite.

We know sum of n terms of an infinite GP is given by S = a/(1–r).

S = 1/[1 – (–1/2)]

= 1/(3/2)

= 2/3

Therefore, sum of infinite terms of the G.P. is 2/3.

(iv) (a² – b²), (a – b), (a–b)/(a+b), … to n terms

Solution:

Given G.P. has first term(a) = (a² – b²), common ratio(r) = (a – b)/(a² – b²) = 1/(a+b) and number of terms is n.

We know sum of n terms of an infinite GP is given by S_n = a(rⁿ–1)/(r–1).

S_n = 

= 

= 

Therefore, sum of n terms of the G.P. is .

(v) 4, 2, 1, 1/2 … to 10 terms

Solution:

Given G.P. has first term(a) = 4, common ratio(r) = 2/4 = 1/2 and number of terms(n) = 10.

We know sum of n terms of a GP is given by S_n = a(rⁿ–1)/(r–1).

S₁₀ = 

= 

= 

= 

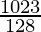
Therefore, sum of 10 terms of the G.P. is .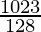

Question 2. Find the sum of the following geometric series:

(i) 0.15 + 0.015 + 0.0015 + … to 8 terms

Solution:

Given G.P. has first term(a) = 0.15, common ratio(r) = 0.015/0.15 = 1/10 and number of terms(n) = 8.

We know sum of n terms of a GP is given by S_n = a(rⁿ–1)/(r–1).

S₈ = 

= 0.15 (10/9) (1 – 1/10⁸)

= (1/6) (1 – 1/10⁸)

Therefore, sum of 8 terms of the G.P. is (1/6) (1 – 1/10⁸).

(ii) √2 + 1/√2 + 1/2√2 + …. to 8 terms

Solution:

Given G.P. has first term(a) = √2, common ratio(r) = (1/√2)/√2 = 1/2 and number of terms(n) = 8.

We know sum of n terms of a GP is given by S_n = a(rⁿ–1)/(r–1).

S₈ = √2[(1/2)⁸–1]/[(1/2)–1]

= √2(1–1/256)/(1/2)

= √2 (255/256) (2)

= (255√2)/128

Therefore, sum of 8 terms of the G.P. is (255√2)/128.

(iii) 2/9 – 1/3 + 1/2 – 3/4 + … to 5 terms

Solution:

Given G.P. has first term(a) = 2/9, common ratio(r) = (–1/3)/(2/9) = –3/2 and number of terms(n) = 5.

We know sum of n terms of a GP is given by S_n = a(rⁿ–1)/(r–1).

S₅ = 

= 

= 

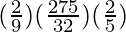
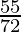
Therefore, sum of 5 terms of the G.P. is .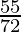

(iv) (x + y) + (x² + xy + y²) + (x³ + x²y + xy² + y³) + …. to n terms

Solution:

Given series can be written as,

S_n = (x + y) + (x² + xy + y²) + (x³ + x²y + xy² + y³) + . . . . to n terms

(x – y) S_n = (x + y) (x – y) + (x² + xy + y²) (x – y) . . . to n terms

(x – y) S_n = x² – y² + x³ + x²y + xy² – x²y – xy² – y³ . . . to n terms

(x – y) S_n = (x² + x³ + x⁴ + . . . n terms) + (y² + y³ + y⁴ + . . . n terms)

(x – y) S_n = x²[(xⁿ – 1)/(x – 1)] – y²[(yⁿ – 1)/(y – 1)]

S_n = [x²[(xⁿ – 1)/(x – 1)] – y²[(yⁿ – 1)/(y – 1)]]/(x – y)

Therefore, sum of n terms of series is [x²[(xⁿ – 1)/(x – 1)] – y²[(yⁿ – 1)/(y – 1)]]/(x – y).

(v) 3/5 + 4/5² + 3/5³ + 4/5⁴ + … to n terms

Solution:

Given series can be written as,

S_n = (3/5 + 3/5³ + . . . to n terms) + (4/5² + 4/5⁴ +  . . . to n terms)

= 

= 

= 

Therefore, sum of n terms of series is .

(vi)

Solution:

Given G.P. has first term(a) = 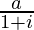, common ratio(r) =  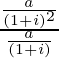=  and number of terms is n.

We know sum of n terms of a GP is given by S_n = a(rⁿ–1)/(r–1). 

S_n = 

= 

= –a i[1–(1+i)^-n]

Therefore, sum of n terms of G.P. is –a i[1–(1+i)^-n].

(vii) 1, –a, a², –a³, . . . . to n terms (a ≠ 1)

Solution:

Given G.P. has first term(a) = 1, common ratio(r) = –a and number of terms is n.

We know sum of n terms of a GP is given by S_n = a(rⁿ–1)/(r–1). 

S_n = [(–a)ⁿ–1]/(–a–1)

= [1–(–a)ⁿ]/(a+1)

Therefore, sum of n terms of G.P. is [1–(–a)ⁿ]/(a+1).

(viii) x³ + x⁵ + x⁷ + . . . . n terms

Solution:

Given G.P. has first term(a) = x, common ratio(r) = x⁵/x³ = x² and number of terms is n.

We know sum of n terms of a GP is given by S_n = a(rⁿ–1)/(r–1). 

= x³[x²ⁿ–1]/[x²–1]

 Therefore, sum of n terms of G.P. is x³[x²ⁿ–1]/[x²–1].

(ix) √7 + √21 + 3√7 + . . . . n terms

Solution:

Given G.P. has first term(a) = √7, common ratio(r) = √21/√7 = √3 and number of terms = n.

We know sum of n terms of a GP is given by S_n = a(rⁿ–1)/(r–1).

S_n = √7[(√3)ⁿ–1]/(√3–1)

Therefore, sum of n terms of G.P. is √7[(√3)ⁿ–1]/(√3–1).

Question 3. Evaluate the following:

(i)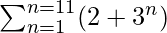 

Solution:

Given summation can be written as,

S₁₁ = (2+3¹) + (2+3²) + (2+3³) + . . . . + (2+3¹¹)

= 2(11) + (3¹ + 3² + 3³ + . . . . 3¹¹)

= 2(11) + 3(3¹¹–1)/(3–1)

= 22 + 265719

= 265741

Therefore, value of the summation is 265741.

(ii)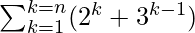 

Solution:

Given summation can be written as,

S_n = (2+3⁰) + (2²+3¹) + (2³+3²) + . . . . + (2ⁿ+3^n-1)

= (2¹ + 2² + 2³ + . . . . + 2ⁿ) + (3⁰ + 3¹ + 3² + . . . . + 3^n-1)

= 2(2ⁿ–1)/(2–1) + 3⁰(3ⁿ–1)/(3–1)

= 2(2ⁿ–1) + (3ⁿ–1)/2

Therefore, value of the summation is 2(2ⁿ–1) + (3ⁿ–1)/2.

(iii)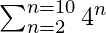

Solution:

Given summation can be written as,

S_10-2+1 = S₉ = 4² + 4³ + 4⁴ + . . . . 4¹⁰

 = 4²(4⁹–1)/(4–1)

= 16[4⁹–1]/3

Therefore, value of the summation is 16[4⁹–1]/3. 

Question 4. Find the sum of the series:

(i) 5 + 55 + 555 + … to n terms

Solution:

We have S_n = 5 + 55 + 555 + ….. up to n terms.

Multiplying and dividing by 9, we get

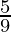=  [9+99+999+…to n terms]

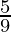=  [(10–1)+(10²–1)+(10³–1)…to n terms]

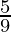=  [(10+10²+10³+….n terms) – (1+1+1+…..n terms)]

= 

= 

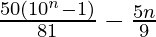
Therefore, the sum of the series up to n terms is .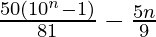 

(ii) 7 + 77 + 777 + … to n terms

Solution:

We have S_n = 7 + 77 + 777 + … to n terms.

Multiplying and dividing by 9, we get,

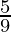=  [9+99+999+…to n terms]

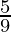=  [(10–1)+(10²–1)+(10³–1)…to n terms]

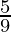=  [(10+10²+10³+….n terms) – (1+1+1+…..n terms)]

= 

= 

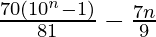
Therefore, the sum of the series up to n terms is .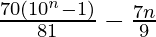

(iii) 9 + 99 + 999 + … to n terms

Solution:

We have S_n = 9 + 99 + 999 + … to n terms. It can be written as,

= (10–1)+(10²–1)+(10³–1)…to n terms

= (10+10²+10³+….n terms) – (1+1+1+…..n terms)

= 

Therefore, the sum of the series up to n terms is .

(iv) 0.5 + 0.55 + 0.555 + … to n terms

Solution:

We have S_n = 0.5 + 0.55 + 0.555 + … to n terms. It can be written as,

= 

= 

= 

= 

= 

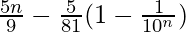
Therefore, the sum of the series up to n terms is .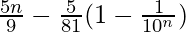

(v) 0.6 + 0.66 + 0.666 + … to n terms

Solution:

We have S_n = 0.6 + 0.66 + 0.666 + … to n terms. It can be written as,

= 

= 

= 

= 

= 

Therefore, the sum of the series up to n terms is .

Question 5. How many terms of the G.P. 3, 3/2, 3/4, … be taken together to make 3069/512?

Solution:

Given G.P. has first term(a) = 3, common ratio(r) = (3/2)/3 = 1/2 and sum of terms(S_n) = 3069/512.

We know sum of n terms of a G.P. is given by S_n = a(rⁿ–1)/(r–1).

=> 3069/512 = 3[1–(1/2)ⁿ] / [1–(1/2)]

=> 2(2ⁿ–1)/(2ⁿ) = 1023/512

=> 1023(2)ⁿ = 1024(2)ⁿ – 1024

=> 2ⁿ = 1024

=> n = 10

Therefore, 10 terms of the G.P. should be taken together to make 3069/512.

Question 6. How many terms of the series 2 + 6 + 18 + …. must be taken to make the sum equal to 728?

Solution: 

Given G.P. has first term(a) = 2, common ratio(r) = 6/2 = 3 and sum of terms(S_n) = 728.

We know sum of n terms of a G.P. is given by S_n = a(rⁿ–1)/(r–1).

=> 728 = 2[3ⁿ–1]/[3–1]

=> 3ⁿ–1 = 728

=> 3ⁿ = 729

=> n = 6

Therefore, 6 terms of the G.P. must be taken together to make the sum equal to 728.

Question 7. How many terms of the sequence √3, 3, 3√3,… must be taken to make the sum 39+ 13√3 ?

Solution:

Given G.P. has first term(a) = 2, common ratio(r) = 3/√3 = 1/√3 and sum of terms(S_n) = 39+ 13√3.

We know sum of n terms of a G.P. is given by S_n = a(rⁿ–1)/(r–1).

=> 39+13√3 = √3[3^n/2–1]/(√3–1)

=> (39+13√3)(√3–1) = √3(3^n/2–1)

=> 39√3–39+39–13√3 = 3^(n+1)/2–√3

=> 3^(n+1)/2 = 27√3 

=>  3^n/2 √3 = 27√3 

=> 3^n/2 = 27 

=> n/2 = 3

=> n = 6

Therefore, 6 terms of the G.P. must be taken to make the sum 39+ 13√3.

Question 8. The sum of n terms of the G.P. 3, 6, 12, … is 381. Find the value of n.

Solution:

Given G.P. has first term(a) = 3, common ratio(r) = 6/3 = 2 and sum of terms(S_n) = 381.

We know sum of n terms of a G.P. is given by S_n = a(rⁿ–1)/(r–1).

=> 381 = 3(2ⁿ–1)/(2–1)

=> 2ⁿ – 1 = 127

=> 2ⁿ = 128

=> n = 7

Therefore, value of n is 7.

Question 9. The common ratio of a G.P. is 3, and the last term is 486. If the sum of these terms be 728, find the first term.

Solution:

Given G.P. has common ratio(r) = 3, last term(a_n) = 486 and sum of terms(S_n) = 728.

We know nth term of a G.P. is given by a_n = ar^n-1.

=> 486 = a(3)^n-1

=> 486 = a(3)ⁿ/3

=> a(3)ⁿ = 1458  . . . . (1)

We know sum of n terms of a G.P. is given by S_n = a(rⁿ–1)/(r–1).

=> 728 = a(3ⁿ–1)/(3–1)

=> 1456 = a(3)ⁿ–a

Using (1) in the equation, we get,

=> a = 1458 – 1456 = 2

Therefore, first term of the G.P. is 2.

Question 10. The ratio of the sum of the first three terms is to that of the first 6 terms of a G.P. is 125 : 152. Find the common ratio.

Solution:

We know sum of n terms of a G.P. is given by S_n = a(rⁿ–1)/(r–1).

According to the question, we have,

=> 

=> (r³–1)/(r⁶–1) = 125/152

=> 1/(r³+1) = 125/152

=> 125r³ + 125 = 152

=> r³ = 27/125

=> r = 3/5

Therefore, the common ratio is 3/5.

Question 11. The 4th and 7th term of a G.P. are 1/27 and 1/729 respectively. Find the sum of n terms of the G.P.

Solution:

We know nth term of a G.P. is given by a_n = ar^n-1.

According to the question, we have,

=> ar³ = 1/27   . . . . (1)

=> ar⁶ = 1/729 . . . . (2)

Dividing (2) by (1), we get,

=> r³ = 27/729 = 1/27

=> r = 1/3

Putting r = 1/3 in (1), we get,

=> a(1/3)³ = 1/27

=> a(1/27) = 1/27

=> a = 1

We know sum of n terms of a G.P. is given by S_n = a(rⁿ–1)/(r–1).

=> S_n = 1[(1/3)ⁿ–1]/[(1/3)–1]

= 3[1–(1/3)ⁿ]/2

Therefore, sum of n terms of the G.P. is 3[1–(1/3)ⁿ]/2.