Class 11 RD Sharma solutions – Chapter 29 Limits – Exercise 29.7 | Set 1

Question 1. Lim_x→0[sin3x/5x]

Solution:

We have,
Lim_x→0[sin3x/5x]
= (1/5)Lim_x→0[sin3x/3x] × 3
= (3/5)Lim_x→0[sin3x/3x]
As we know that, Lim_x→0[sinx/x] = 1
= (3/5)

Question 2. Lim_x→0[sinx°/x]

Solution:

We have,
Lim_x→0[sinx°/x]
As we know that x° = [(πx)/180]
= Lim_x→0[sin{(πx)/180}/x]
= $\lim_{x\to0}\frac{sin\frac{πx}{180}}{\frac{πx}{180}}×\frac{π}{180}$
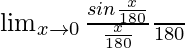
= (π/180) × 1
= (π/180)

Question 3. Lim_x→0[x²/sinx²]

Solution:

We have,
Lim_x→0[x²/sinx²]
= $Lim_{x→0}[\frac{1}{\frac{sinx^2}{x^2}}]$
= $[\frac{1}{ Lim_{x→0}\frac{sinx^2}{x^2}}]$
As we know that, Lim_x→0[sinx/x] = 1
= 1/1
= 1

Question 4. Lim_x→0[sinx.cosx/3x]

Solution:

We have,
Lim_x→0[(sinx.cosx)/3x]
= Lim_x→0[(sinx.cosx)/ × 3x]
= 1/3 Lim_x→0[(sinx)/x] × Lim_x→0[(cosx)]
As we know that Lim_x→0[sinx/x] = 1, and Lim_x→0cos0 = 1
= (1/3) × 1 × 1
= 1/3

Question 5. Lim_x→0[(3sinx – 4sin³x)/x]

Solution:

We have,
Lim_x→0[(3sinx – 4sin³x)/x]
As we know that 3sinx – 4sin³x = sin3x
= Lim_x→0[(sin3x)/3x] × 3
= 3 × Lim_x→0[(sin3x)/3x]
As we know that, Lim_x→0[sinx/x] = 1
= 3 × 1
= 3

Question 6. Lim_x→0[tan8x/sin2x]

Solution:

We have,
Lim_x→0[tan8x/sin2x]
= $\lim_{x\to0}[\frac{tan8x}{8x}×8x][\frac{1}{\frac{sin2x}{2x}×2x}]$
= $\lim_{x\to0}[\frac{tan8x}{8x}][\frac{1}{\frac{sin2x}{2x}}]×\frac{8x}{2x}$
As we know that Lim_x→0[sin2x/2x] = 1 and Lim_x→0[tan8x/8x] = 1
= 8/2
= 4

Question 7. Lim_x→0[tan(mx)/tan(nx)]

Solution:

We have,
Lim_x→0[tan(mx)/tan(nx)]
= $\lim_{x\to0}[\frac{tanmx}{mx}×mx][\frac{1}{\frac{tannx}{nx}×nx}]$
= $\lim_{x\to0}[\frac{tanmx}{mx}][\frac{1}{\frac{tannx}{nx}}]×\frac{mx}{nx}$
As we know that Lim_x→0[tanx/x] = 1
= m × 1/n × 1
= m/n

Question 8. Lim_x→0[sin5x/tan3x]

Solution:

We have,
Lim_x→0[sin5x/tan3x]
= $\lim_{x\to0}[\frac{sin5x}{5x}×5x][\frac{1}{\frac{tan3x}{3x}×3x}]$
= $\lim_{x\to0}[\frac{sin5x}{5x}][\frac{1}{\frac{tan3x}{3x}}]×\frac{5x}{3x}$
As we know that Lim_x→0[sin5x/5x] = 1 and Lim_x→0[tan3x/3x] = 1
= 5/3 × 1
= 5/3

Question 9. Lim_x→0[sin(xⁿ)/(xⁿ)]

Solution:

We have,
Lim_x→0[sin(xⁿ)/(xⁿ)]
It is in the form of 0/0
So, let xⁿ= y
x→0 than y→0
Lim_y→0[siny/y]
As we know that Lim_y→0[siny/y] = 1
= 1

Question 10. Lim_x→0[(7xcosx – 3sinx)/(4x + tanx)]

Solution:

We have,
Lim_x→0[(7xcosx – 3sinx)/(4x + tanx)]
= Lim_x→0[x(7cosx-3sinx/x)/x(4 + tanx/x)]
= Lim_x→0[(7cosx-3sinx/x)/(4 + tanx/x)]
= $\frac{7\lim_{x\to0}cosx-3\lim_{x\to0}\frac{sinx}{x}}{4+\lim_{x\to0}\frac{tanx}{x}}$
As we know that Lim_x→0[sinx/x] = 1 and Lim_x→0[tanx/x] = 1
= (7 – 3)/(4 + 1)
= 4/5

Question 11. Lim_x→0[{cos(ax) – cos(bx)}/{cos(cx) – cos(dx)}]

Solution:

We have,
Lim_x→0[{cos(ax) – cos(bx)}/{cos(cx) – cos(dx)}]
= $\lim_{x\to0}[\frac{-2sin(\frac{ax+bx}{2})sin(\frac{ax-bx}{2})}{-2sin(\frac{cx+dx}{2})sin(\frac{cx-dx}{2})}]$
= $\lim_{x\to0}[\frac{\frac{sin(\frac{ax+bx}{2})}{(\frac{ax+bx}{2})}×(\frac{ax+bx}{2})\frac{sin(\frac{ax-bx}{2})}{(\frac{ax-bx}{2})}×(\frac{ax-bx}{2})}{\frac{sin(\frac{cx+dx}{2})}{(\frac{cx+dx}{2})}×(\frac{cx+dx}{2})\frac{sin(\frac{cx-dx}{2})}{(\frac{cx-dx}{2})}×(\frac{cx-dx}{2})}]$
= $\lim_{x\to0}[\frac{\frac{(ax+bx)}{2}\frac{(ax-bx)}{2}}{\frac{(cx+dx)}{2}\frac{(cx-dx)}{2}}]$
= $\lim_{x\to0}[\frac{x^2(a+b)(a-b)}{x^2(c+d)(c-d)}]$
= (a²– b²)/(c²– d²)

Question 12. Lim_x→0[(tan²3x)/x²]

Solution:

We have,
Lim_x→0[(tan²3x)/x²]
= Lim_x→0[(tan3x)/x]²
= Lim_x→0[(tan3x)/3x]²× 9
As we know that Lim_x→0[tanx/x] = 1
= 1 × 9
= 9

Question 13. Lim_x→0[(1 – cosmx)/x²]

Solution:

We have,
Lim_x→0[(1 – cosmx)/x²]
= $\lim_{x\to0}[\frac{2sin^2\frac{mx}{2}}{x^2}]$
= $2\lim_{x\to0}[\frac{sin\frac{mx}{2}}{x}]^2$
= $2\lim_{x\to0}[\frac{sin\frac{mx}{2}}{\frac{mx}{2}}]^2×(\frac{m}{2})^2$
As we know that Lim_x→0[sinx/x] = 1
= 2 × (m/2)²
= m²/2

Question 14. Lim_x→0[(3sin2x + 2x)/(3x + 2tan3x)]

Solution:

We have,
Lim_x→0[(3sin2x + 2x)/(3x + 2tan3x)]
= Lim_x→0[2x(1 + 3sin2x/2x)/3 x (1 + 2tan3x/3x)]
= (2/3)Lim_x→0[(1 + 3sin2x/2x)/3 x (1 + 2tan3x/3x)]
= $\frac{2}{3}\lim_{x\to0}\frac{1+3\lim_{x\to0}\frac{sin2x}{2x}}{1+2\lim_{x\to0}\frac{tan3x}{3x}}$
As we know that Lim_x→0[sinx/x] = 1 and Lim_x→0[tanx/x] = 1
= (2/3) × (4/3)
= 8/9

Question 15. Lim_x→0[(cos3x – cos7x)/x²]

Solution:

We have,
= $\lim_{x\to0}[\frac{-2sin(\frac{3x+7x}{2})sin(\frac{3x-7x}{2})}{x^2}]$
= Lim_x→0[-2sin5x.sin(-2x)/x²]
= Lim_x→0[2sin5x.sin2x/x²]
= $2\lim_{x\to0}[\frac{sin5x}{5x}×5]×\lim_{x\to0}[\frac{sin2x}{2x}×2]$
As we know that Lim_x→0[sinx/x] = 1
= 2 × 5 × 2
= 20

Question 16. Lim_θ→0[sin3θ/tan2θ]

Solution:

We have,
Lim_θ→0[sin3θ/tan2θ]
= $\lim_{θ\to0}[\frac{sin3θ}{3θ}×3θ][\frac{1}{\frac{tan2θ}{2θ}×2θ}]$
= $\lim_{x\to0}[\frac{sin3θ}{3θ}][\frac{1}{\frac{tan2θ}{2θ}}]×\frac{3θ}{2θ}$
As we know that Lim_x→0[sinx/x] = 1 and Lim_x→0[tanx/x] = 1
= 3/2

Question 17. Lim_x→0[sinx²(1 – cosx²)/x⁶]

Solution:

We have,
Lim_x→0[sinx²(1 – cosx²)/x⁶]
= Lim_x→0[sinx²{2sin(x²/2}/x⁶]
= $2\lim_{x\to0}[\frac{sinx^2.sin^2(\frac{x^2}{2})}{x^6}]$
= $2\lim_{x\to0}(\frac{sinx^2}{x^2})(\frac{sin\frac{x^2}{2}}{\frac{x^2}{2}×2})^2$
= $2\lim_{x\to0}(\frac{sinx^2}{x^2})(\frac{sin\frac{x^2}{2}}{\frac{x^2}{2}})^2×\frac{1}{4}$
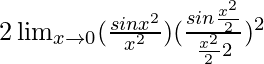
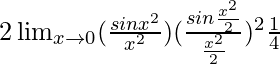
As we know that Lim_x→0[sinx/x] = 1
= 2 × (1/4)
= 1/2

Question 18. Lim_x→0[sin²(4x²)/x⁴]

Solution:

We have,
Lim_x→0[sin²(4x²)/x⁴]
= Lim_x→0[(sin(4x²))²/(x²)²]
= Lim_x→0[sin(4x²)/4x²]²× 4²
As we know that Lim_x→0[sinx/x] = 1
= 4²
= 16

Question 19. Lim_x→0[(xcosx + 2sinx)/(x²+ tanx)]

Solution:

We have,
Lim_x→0[(xcosx + 2sinx)/(x²+ tanx)]
= Lim_x→0[x(cosx + 2sinx/x)/x(x + tanx/x)]
= Lim_x→0[(cosx + 2sinx/x)/(x + tanx/x)]
= $\frac{\lim_{x\to0}cosx+2\lim_{x\to0}\frac{sinx}{x}}{\lim_{x\to0}x+\lim_{x\to0}\frac{tanx}{x}}$
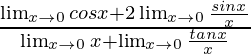
As we know that Lim_x→0[sinx/x] = 1 and Lim_x→0[tanx/x] = 1
= (1 + 2)/(0 + 1)
= 3

Question 20. Lim_x→0[(2x – sinx)/(x + tanx)]

Solution:

We have,
Lim_x→0[(2x – sinx)/(x + tanx)]
= Lim_x→0[x(2 – sinx/x)/x(1 + tanx/x)]
= Lim_x→0[(2 – sinx/x)/(1 + tanx/x)]
= $\frac{2-\lim_{x\to0}\frac{sinx}{x}}{1+\lim_{x\to0}\frac{tanx}{x}}$
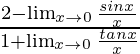
As we know that Lim_x→0[sinx/x] = 1 and Lim_x→0[tanx/x] = 1
= (2 – 1)/(1 + 1)
= 1/2

Question 21. Lim_x→0[(5xcosx + 3sinx)/(3x²+ tanx)]

Solution:

We have,
Lim_x→0[(5xcosx + 3sinx)/(3x²+ tanx)]
= Lim_x→0[x(5cosx + 3sinx/x)/x(3x + tanx/x)]
= Lim_x→0[(5cosx + 3sinx/x)/(3x + tanx/x)]
= $\frac{5\lim_{x\to0}cosx+3\lim_{x\to0}\frac{sinx}{x}}{3\lim_{x\to0}x+\lim_{x\to0}\frac{tanx}{x}}$
As we know that Lim_x→0[sinx/x] = 1 and Lim_x→0[tanx/x] = 1
= (5 cos0 + 3)/(0 + 1)
= 8

Question 22. Lim_x→0[(sin3x – sinx)/(sinx)]

Solution:

We have,
Lim_x→0[(sin3x – sinx)/(sinx)]
= $\lim_{x\to0}[\frac{2cos(\frac{3x+x}{2})sin(\frac{3x-x}{2})}{sinx}]$
= 2Lim_x→0[cos2x.sinx/sinx]
= 2Lim_x→0cos2x
= 2 × cos0
= 2 × 1
= 2

Question 23. Lim_x→0[(sin5x – sin3x)/(sinx)]

Solution:

We have,
Lim_x→0[(sin5x – sin3x)/(sinx)]
= $\lim_{x\to0}[\frac{2cos(\frac{5x+3x}{2})sin(\frac{5x-3x}{2})}{sinx}]$
= 2Lim_x→0[cos4x.sinx/sinx]
= 2Lim_x→0cos4x
= 2 × cos0
= 2 × 1
= 2

Question 24. Lim_x→0[(cos3x – cos5x)/x²]

Solution:

We have,
Lim_x→0[(cos3x – cos5x)/x²]
= $\lim_{x\to0}[\frac{-2sin(\frac{3x+5x}{2})sin(\frac{3x-5x}{2})}{x^2}]$
= Lim_x→0[-2sin4x.sin(-x)/x²]
= Lim_x→0[2sin4x.sinx/x²]
= $2\lim_{x\to0}[\frac{sin4x}{4x}×4]×\lim_{x\to0}[\frac{sinx}{x}]$
As we know that Lim_x→0[sinx/x] = 1
= 2 × 4 × 1
= 8

Question 25. Lim_x→0[(tan3x – 3x)/(3x – sin²x)]

Solution:

We have,
Lim_x→0[(tan3x – 3x)/(3x – sin²x)]
= Lim_x→0[x(tan3x/x – 3)/x(3 – sin²x/x)]
= Lim_x→0[(tan3x/x – 3)/(3 – sin²x/x)]
= $\lim_{x\to0}[\frac{\frac{tan3x}{3x}×3-2}{3-\frac{sinx}{x}×sinx}]$
As we know that Lim_x→0[sinx/x] = 1
= (3 – 2)/(3 – 0)
= 1/3

Question 26. Lim_x→0[{sin(2 + x) – sin(2 – x)}/x]

Solution:

We have,
Lim_x→0[{sin(2 + x) – sin(2 – x)}/x]
= $\lim_{x\to0}[\frac{2cos(\frac{2+x+2-x}{2})sin(\frac{2+x-2+x}{2})}{x}]$
= 2Lim_x→0[cos2.sinx/x]
= 2cos×2Lim_x→0[sinx/x]
As we know that Lim_x→0[sinx/x] = 1
= 2cos2

Question 27. Lim_h→0[{(a + h)²sin(a + h) – a²sina}/h]

Solution:

We have,
Lim_h→0[{(a + h)²sin(a + h) – a²sina}/h]
= Lim_h→0[{a²sin(a + h) + h²sin(a + h) + 2ahsin(a + h) – a²sina}/h]
= $\lim_{h\to0}[a^2(\frac{sin(a+h)-sina}{h}+\frac{h^2sin(a+h)}{h}+\frac{2ahsin(a+h)}{h}]$
= $\lim_{h\to0}[a^2\frac{2cos\frac{(a+h+a)}{2}sin\frac{(a+h-a)}{2}}{2×\frac{h}{2}}+hsin(a+h)+2asin(a+h)]$
= $\lim_{h\to0}[a^2cos(\frac{a+h+a}{2})+hsin(a+h)+2asin(a+h)$
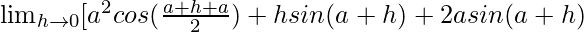
= a²cosa + 0 + 2asina
= a²cosa + 2asina

Question 28. Lim_x→0[{tanx – sinx}/{sin3x – 3sinx}]

Solution:

We have,
Lim_x→0[{tanx – sinx}/{sin3x – 3sinx}]
= $\lim_{x\to0}[\frac{\frac{sinx}{cosx}-sinx}{3sinx-4sin^3x-3sinx}]$
= $\frac{-1}{4}\lim_{x\to0}[\frac{sinx(\frac{1}{cosx}-1)}{sin^2x.sinx}]$
= $\frac{-1}{4}\lim_{x\to0}[\frac{(\frac{1-cosx}{cosx})}{1-cos^2x}]$
= $\frac{-1}{4}\lim_{x\to0}[\frac{(1-cosx)}{cosx(1-cosx)(1+cosx)}]$
= $\frac{-1}{4}\lim_{x\to0}[\frac{1}{cosx(1+cosx)}]$
= (-1/4)(1/2)
= -(1/8)

Question 29. Lim_x→0[{sex5x – sec5x}/{sec3x – secx}]

Solution:

We have,
Lim_x→0[{sex5x – sec5x}/{sec3x – secx}]
= $\lim_{x\to0}\frac{\frac{1}{cos5x}-\frac{1}{cos3x}}{\frac{1}{cos3x}-\frac{1}{cosx}}$
= $\lim_{x\to0}(\frac{cos3x-cos5x}{cosx-cos3x})(\frac{cosx.cos3x}{cos3x.cos5x})$
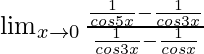
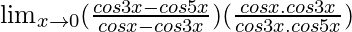
= $\lim_{x\to0}[\frac{-2sin4x.sin(-x)}{-2sin2x.sin(-x)}][\frac{cosx}{cos5x}]$
= $\lim_{x\to0}[\frac{sin4x}{sin2x}][\frac{cosx}{cos5x}]$
= $\lim_{x\to0}[\frac{\frac{sin4x}{4x}×4x}{\frac{sin2x}{2x}×2x}][\frac{cosx}{cos5x}]$
As we know that Lim_x→0[sinx/x] = 1
= (4x/2x)(cos0/cos0)
= 2

Question 30. Lim_x→0[{1 – cos2x}/{cos2x – cos3x}]

Solution:

We have,
Lim_x→0[{1 – cos2x}/{cos2x – cos3x}]
= $\lim_{x\to0}[\frac{2sin^2x}{-2sin(\frac{3x+7x}{2})sin(\frac{3x-7x}{2})}]$
= $\lim_{x\to0}[\frac{2sin^2x}{2sin(5x)sin(3x)}]$
= $\lim_{x\to0}[\frac{sinx.sinx}{sin(5x)sin(3x)}]$
= $\lim_{x\to0}[\frac{(\frac{sinx}{x}×x)(\frac{sinx}{x}×x)}{(\frac{sin5x}{5x}×5x)(\frac{sin3x}{3x}×3x)}$
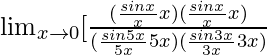
As we know that Lim_x→0[sinx/x] = 1
= (1/5 × 3)
= 1/15

Question 31. Lim_x→0[(1 – cos2x + tan²x)/(xsinx)]

Solution:

We have,
Lim_x→0[(1 – cos2x + tan²x)/(xsinx)]
= Lim_x→0[(2sin²x + tan²x)/(xsinx)]
Dividing numerator and denominator by x²
= $\lim_{x\to0}\frac{2(\frac{sinx}{x})^2 \times x^2+(\frac{tanx}{x})^2\times x^2}{\lim_{x\to0}(\frac{sinx}{x})\times x^2}$
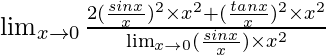
As we know that Lim_x→0[sinx/x] = 1 and Lim_x→0[tanx/x] = 1
= (2 × 1 × x² + 1) + (1 × x²) /(1 × x²)
= 3

My Personal Notes

Last Updated : 04 May, 2021

Related Articles

Class 11 RD Sharma solutions – Chapter 29 Limits – Exercise 29.7 | Set 1

Question 1. Lim_x→0[sin3x/5x]

Question 2. Lim_x→0[sinx°/x]

Question 3. Lim_x→0[x²/sinx²]

Question 4. Lim_x→0[sinx.cosx/3x]

Question 5. Lim_x→0[(3sinx – 4sin³x)/x]

Question 6. Lim_x→0[tan8x/sin2x]

Question 7. Lim_x→0[tan(mx)/tan(nx)]

Question 8. Lim_x→0[sin5x/tan3x]

Question 9. Lim_x→0[sin(xⁿ)/(xⁿ)]

Question 10. Lim_x→0[(7xcosx – 3sinx)/(4x + tanx)]

Question 11. Lim_x→0[{cos(ax) – cos(bx)}/{cos(cx) – cos(dx)}]

Question 12. Lim_x→0[(tan²3x)/x²]

Question 13. Lim_x→0[(1 – cosmx)/x²]

Question 14. Lim_x→0[(3sin2x + 2x)/(3x + 2tan3x)]

Question 15. Lim_x→0[(cos3x – cos7x)/x²]

Question 16. Lim_θ→0[sin3θ/tan2θ]

Question 17. Lim_x→0[sinx²(1 – cosx²)/x⁶]

Question 18. Lim_x→0[sin²(4x²)/x⁴]

Question 19. Lim_x→0[(xcosx + 2sinx)/(x²+ tanx)]

Question 20. Lim_x→0[(2x – sinx)/(x + tanx)]

Question 21. Lim_x→0[(5xcosx + 3sinx)/(3x²+ tanx)]

Question 22. Lim_x→0[(sin3x – sinx)/(sinx)]

Question 23. Lim_x→0[(sin5x – sin3x)/(sinx)]

Question 24. Lim_x→0[(cos3x – cos5x)/x²]

Question 25. Lim_x→0[(tan3x – 3x)/(3x – sin²x)]

Question 26. Lim_x→0[{sin(2 + x) – sin(2 – x)}/x]

Question 27. Lim_h→0[{(a + h)²sin(a + h) – a²sina}/h]

Question 28. Lim_x→0[{tanx – sinx}/{sin3x – 3sinx}]

Question 29. Lim_x→0[{sex5x – sec5x}/{sec3x – secx}]

Question 30. Lim_x→0[{1 – cos2x}/{cos2x – cos3x}]

Question 31. Lim_x→0[(1 – cos2x + tan²x)/(xsinx)]

Data Structures and Algorithms - Self Paced

CBSE Class 12 Computer Science

GATE CS & IT 2024

Related Articles

Class 11 RD Sharma solutions – Chapter 29 Limits – Exercise 29.7 | Set 1

Question 1. Limx→0[sin3x/5x]

Question 2. Limx→0[sinx°/x]

Question 3. Limx→0[x2/sinx2]

Question 4. Limx→0[sinx.cosx/3x]

Question 5. Limx→0[(3sinx – 4sin3x)/x]

Question 6. Limx→0[tan8x/sin2x]

Question 7. Limx→0[tan(mx)/tan(nx)]

Question 8. Limx→0[sin5x/tan3x]

Question 9. Limx→0[sin(xn)/(xn)]

Question 10. Limx→0[(7xcosx – 3sinx)/(4x + tanx)]

Question 11. Limx→0[{cos(ax) – cos(bx)}/{cos(cx) – cos(dx)}]

Question 12. Limx→0[(tan23x)/x2]

Question 13. Limx→0[(1 – cosmx)/x2]

Question 14. Limx→0[(3sin2x + 2x)/(3x + 2tan3x)]

Question 15. Limx→0[(cos3x – cos7x)/x2]

Question 16. Limθ→0[sin3θ/tan2θ]

Question 17. Limx→0[sinx2(1 – cosx2)/x6]

Question 18. Limx→0[sin2(4x2)/x4]

Question 19. Limx→0[(xcosx + 2sinx)/(x2 + tanx)]

Question 20. Limx→0[(2x – sinx)/(x + tanx)]

Question 21. Limx→0[(5xcosx + 3sinx)/(3x2 + tanx)]

Question 22. Limx→0[(sin3x – sinx)/(sinx)]

Question 23. Limx→0[(sin5x – sin3x)/(sinx)]

Question 24. Limx→0[(cos3x – cos5x)/x2]

Question 25. Limx→0[(tan3x – 3x)/(3x – sin2x)]

Question 26. Limx→0[{sin(2 + x) – sin(2 – x)}/x]

Question 27. Limh→0[{(a + h)2sin(a + h) – a2sina}/h]

Question 28. Limx→0[{tanx – sinx}/{sin3x – 3sinx}]

Question 29. Limx→0[{sex5x – sec5x}/{sec3x – secx}]

Question 30. Limx→0[{1 – cos2x}/{cos2x – cos3x}]

Question 31. Limx→0[(1 – cos2x + tan2x)/(xsinx)]

Please Login to comment...

Data Structures and Algorithms - Self Paced

CBSE Class 12 Computer Science

GATE CS & IT 2024

Question 1. Lim_x→0[sin3x/5x]

Question 2. Lim_x→0[sinx°/x]

Question 3. Lim_x→0[x²/sinx²]

Question 4. Lim_x→0[sinx.cosx/3x]

Question 5. Lim_x→0[(3sinx – 4sin³x)/x]

Question 6. Lim_x→0[tan8x/sin2x]

Question 7. Lim_x→0[tan(mx)/tan(nx)]

Question 8. Lim_x→0[sin5x/tan3x]

Question 9. Lim_x→0[sin(xⁿ)/(xⁿ)]

Question 10. Lim_x→0[(7xcosx – 3sinx)/(4x + tanx)]

Question 11. Lim_x→0[{cos(ax) – cos(bx)}/{cos(cx) – cos(dx)}]

Question 12. Lim_x→0[(tan²3x)/x²]

Question 13. Lim_x→0[(1 – cosmx)/x²]

Question 14. Lim_x→0[(3sin2x + 2x)/(3x + 2tan3x)]

Question 15. Lim_x→0[(cos3x – cos7x)/x²]

Question 16. Lim_θ→0[sin3θ/tan2θ]

Question 17. Lim_x→0[sinx²(1 – cosx²)/x⁶]

Question 18. Lim_x→0[sin²(4x²)/x⁴]

Question 19. Lim_x→0[(xcosx + 2sinx)/(x²+ tanx)]

Question 20. Lim_x→0[(2x – sinx)/(x + tanx)]

Question 21. Lim_x→0[(5xcosx + 3sinx)/(3x²+ tanx)]

Question 22. Lim_x→0[(sin3x – sinx)/(sinx)]

Question 23. Lim_x→0[(sin5x – sin3x)/(sinx)]

Question 24. Lim_x→0[(cos3x – cos5x)/x²]

Question 25. Lim_x→0[(tan3x – 3x)/(3x – sin²x)]

Question 26. Lim_x→0[{sin(2 + x) – sin(2 – x)}/x]

Question 27. Lim_h→0[{(a + h)²sin(a + h) – a²sina}/h]

Question 28. Lim_x→0[{tanx – sinx}/{sin3x – 3sinx}]

Question 29. Lim_x→0[{sex5x – sec5x}/{sec3x – secx}]

Question 30. Lim_x→0[{1 – cos2x}/{cos2x – cos3x}]

Question 31. Lim_x→0[(1 – cos2x + tan²x)/(xsinx)]