Triangles are super important shapes. It’s crucial to know the different types of triangles, which are classified based on their sides and angles.
Triangles show up in everyday life in various ways. They’re in traffic signals, the front and back views of pyramids, and even in the mysterious Bermuda Triangle. Triangles are important because they provide a stable base, making them useful in building foundations and trusses. Majorly, there are 6 types of triangles.
In this article, you will read in detail about the different types of triangles based on sides and based on angles.
What is a Triangle?
Triangles are simple shapes with three sides, formed when three lines intersect. They’re a basic part of geometry, having three sides, three angles, and three vertices. In the figure below, check out triangle ABC. It has vertices A, B, and C, and sides AB, AC, and BC.
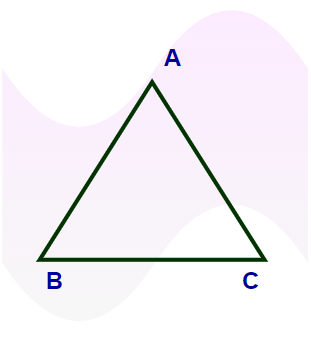
The angle sum property of a triangle is a fundamental concept in geometry, stating that the sum of the measures of all three interior angles of any triangle is always equal to 180 degrees.
What are the Different Types of Triangles?
Triangles can be classified base don various factors. Most important are on the basis of Sides and on the basis of angles.
Types of Triangles based on sides
On the basis of sides, There are 3 types of triangles
- Equilateral Triangle
- Isosceles Triangle
- Scalene Triangle
Let’s discuss these types in detail.
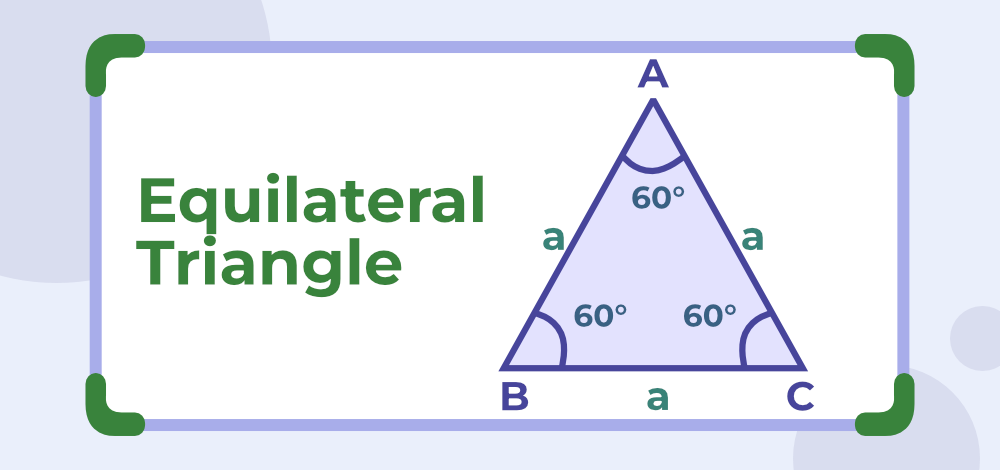
Equilateral Triangles
Equilateral triangles are triangles where all sides and angles are equal. Because all angles are the same, each angle in an equilateral triangle is 60°. Another name for an equilateral triangle is an equiangular triangle. Here length of the sides and angles is equal to each other.
Isosceles Triangles
An isosceles triangle is a triangle where two sides are equal, and the third side is not equal to the other two. The angles opposite to the equal sides of this triangle are also equal.

Scalene Triangles
A scalene triangle is one where none of the sides are equal, and none of the angles are equal either. However, the general properties of triangles still apply to scalene triangles. Hence, the sum of all the interior angles is always equal to 180°

Classification of Triangles
Triangles are the most fundamental shape in geometry. Based on the following two major factors, we can classify triangles:
- Interiors Angles
- Sides of Triangles
Let’s understand these different classifications in detail.
Types of Triangles based on angles
Based on the interior angles of a triangle, we can classify the triangle into three types:
- Acute Angled Triangle
- Right Angled Triangle
- Obtuse Angles Triangle
Let’s discuss these types in detail.
Acute Angled Triangle
An Acute angled Triangle is one where all the interior angles of the Triangle are less than 90°. For Instance, an Equilateral Triangle is an acute-angled triangle (all angles are less than 90°).

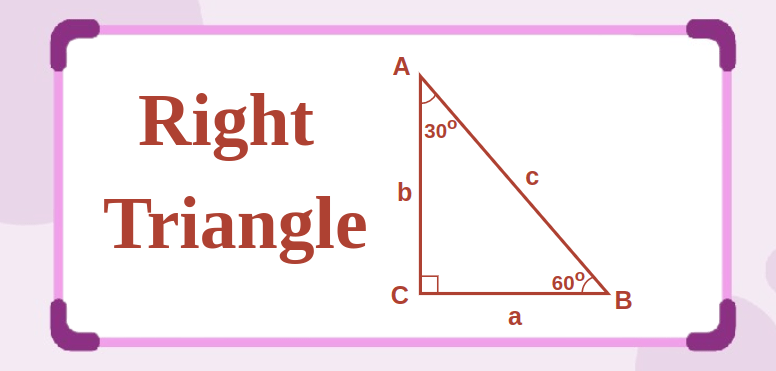
Right Angled Triangle
A Right Angled Triangle is one where one of the angles is always equal to 90°. Pythagoras’ Theorem applies to right-angled triangles. It says that the square of the hypotenuse (the longest side) equals the sum of the squares of the base and perpendicular.
Obtuse Angled Triangle
In an obtuse-angled triangle, one side measures more than 90°. Here, one of the three angles is greater than 90°, making the other two angles less than 90°.

Classification Based on the Sides and Angles of Triangle
There are various other types of triangles based on both angles and sides of the triangle, some of these types are:
- Isosceles Right Triangle
- Obtuse Isosceles Triangle
- Acute Isosceles Triangle
- Right Scalene Triangle
- Obtuse Scalene Triangle
- Acute Scalene Triangle
Let’s discuss these types of triangles in detail.
Isosceles Right Triangle
An isosceles right triangle, also called a right isosceles triangle, has two main characteristics. First, it possesses two sides of equal length, often referred to as the legs. Second, it contains one angle measuring exactly 90 degrees, known as the right angle.
For example, look at triangle ABC. Sides AB and BC both measure 8 centimeters (AB = BC = 8cm), and angle B is a right angle (∠B = 90°). This configuration perfectly exemplifies an isosceles right triangle.
Obtuse Isosceles Triangle
An Obtuse Isosceles Triangle is a triangle with two sides equal and one interior angle measuring more than 90°. For instance, in triangle PQR, sides PQ = PR = 10 cm, and ∠P = 110°, it’s an example of an Obtuse Isosceles Triangle.
Acute Isosceles Triangle
An Acute Isosceles Triangle is a triangle with two equal sides, and all interior angles measuring less than 90°. For instance, in triangle LMN, sides LM = LN = 8 cm, and ∠M = ∠N = 70°, it’s an example of an Acute Isosceles Triangle.

Right Scalene Triangle
A Right triangle is a triangle with all sides of different lengths and one interior angle to be 90°.
For example, picture a triangle ABC:
Here, AC = 6 cm, BC = 8 cm and AB = 10 cm. Plus, angle C measures 90 degrees, making it a perfect example of a Right Scalene Triangle.
Obtuse Scalene Triangle
An Obtuse Scalene Triangle is a triangle with all sides of different lengths and one obtuse angle. For example, imagine a triangle named DEF where all three sides are different lengths. On top of that, one of the angles inside, angle F, opens extra wide at 135 degrees, more than a right angle! That’s what makes DEF an Obtuse Scalene Triangle – different side lengths and a super-wide angle.
Acute Scalene Triangle
An Acute Scalene Triangle is a triangle with all angles less than a right angle and all sides of different lengths.
Picture triangle GHI, where each side is of different length: GH = 5 cm, HI = 7 cm and IG = 9 cm. And all three angles are sharp, none wider than a right angle – with ∠I = 60 degrees. That makes GHI an Acute Scalene Triangle – different sides and all sharp angles!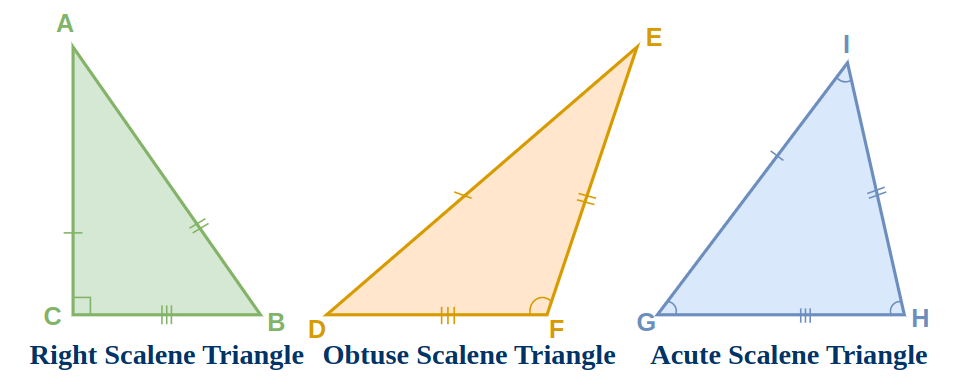
Also, Read
FAQs on Types of Triangles
What is a Triangle in Maths?
A triangle is a two-dimensional geometric shape that is formed by connecting three vertices with three line segments while making three interior angles as well.
What are Different Basis on which Triangles can be Classified?
There are various types of triangles based on different parameters of classification i.e.,
- Based on Sides
- Based on Interior Angles
How are Triangles Classified Based on Sides?
Based on the sides, triangles can be classified as follows:
- Equilateral Triangle
- Isosceles Triangle
- Scalene Triangle
What are Different Types of Triangles Based on Angles?
Based on the measurement of interior angles, triangles can be classified as follows:
- Acute Angle Triangle
- Right Angle Triangle
- Obtuse Angle Triangle
Are There any Triangles that have both Equal sides and Equal angles?
Yes, there is a triangle where each angle and side is equal i.e., equilateral triangle.
Can a Triangle be both Obtuse and Isosceles?
Yes, a triangle can be both obtuse as well as Isoceles and is called Obtuse Isosceles Triangle.
Which Triangle has no Equal Sides?
A scalene triangle is a triangle which has no equal sides.
What are 7 Properties of riangle?
7 properties of triangle are,
- Sum of all the angles of a triangle is 180°
- Sum of the length of the two sides of a triangle is greater than the length of the third side.
- Difference between the two sides of a triangle is less than the length of the third side.
- Side opposite the greater angle is the longest side of a triangle.
- The exterior angle of a triangle is always equal to the sum of the interior opposite angles.
- Area of a triangle = ½ × Base × Height
What are 5 Properties of a Right Angle Triangle?
5 properties of triangle are,
- Sides of right angle follow Pythagoras Theorem
- One angle in right angle is always 90°
- Other two angles of right angle are always acute angle.
- Sum of all the angles of a triangle is 180°
- Sum of the length of the two sides of a triangle is greater than the length of the third side.
Share your thoughts in the comments
Please Login to comment...