Division of Line Segment in Given Ratio – Constructions | Class 10 Maths

Line is a straight one-dimensional figure that has no thickness. In geometry, a line extends endlessly in both directions. It is described as the shortest distance between any two points. A-line can also be understood as multiple points connected to each other in one specific direction without a gap between them.

Line Segment is a part of a line that is bounded by two different endpoints and contains every point on the line between its endpoints in the shortest possible distance.

Rules to write down the coordinates of the point which divides the join of two given points P(x₁,y₁) and Q(x₂,y₂) internally in a given ratio m₁:m₂

1. Draw the line segment joining the given points P and Q
2. Write down the coordinates of p and Q at extremities
3. Let R(x, y) be the input which divides PQ internally in the ratio m₁:m₂
4. For x-coordinate of R, multiply x₂ with m₁ and x₁ with m₂  and add the products. Divide the sum by m₁+m₂
5. For y-coordinate of R, multiply y₂ with m₁ and y₁ with m₂  and add the products. Divide the sum by m₁+m₂

Derivation of Formula

Let P(x₁, y₁) and Q(x₂, y₂) be the two ends of a given line in a coordinate plane, and R(x,y) be the point on that line which divides PQ in the ratio m₁:m₂ such that 

PR/RQ=m₁/m₂              …(1)

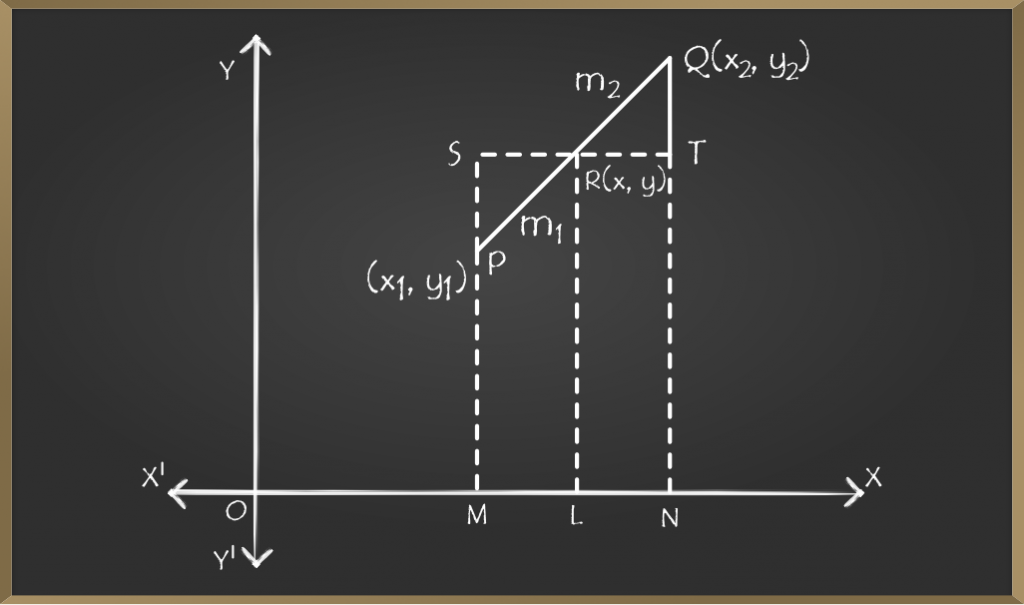
Drawing lines PM, QN, and RL perpendicular on the x-axis and through R draw a straight line parallel to the x-axis to meet MP at S and NQ at T.

Hence, from the figure, we can say,

SR = ML = OL – OM = x – x₁          …(2)

RT = LN = ON – Ol = x₂ – x            …(3)

PS = MS – MP = LR – MP = y – y₁    …(4)

TQ = NQ – NT = NQ – LR = y₂ – y   …(5)

Now ∆SPR is similar to ∆TQR

SR / RT = PR / RQ

By using equation 2, 3, and 1, we know, 

x – x₁ / x₂ – x = m₁ / m₂

m₂x – m₂x₁ = m₁x₂ – m₁x

m₁x + m₂x = m₁x₂ + m₂x₁

(m₁ + m₂)x = m₁x₂ + m₂x₁

x = (m₁x₂ + m₂x₁) / (m₁ + m₂)

Now ∆SPR is similar to ∆TQR,

Therefore,

PS/TQ = PR/RQ

By using equation 4, 5, and 1, we know,

y – y₁ / y₂ – y = m₁/m₂

m₂y – m₂y₁ = m₁y₂ – m₁y

m₁y + m₂y = m₁y₂ + m₂y₁

(m₁ + m₂)y = m₁y₂ + m₂y₁

y = (m₁y₂ + m₂y₁)/(m₁ + m₂)

Hence, the coordinate of R(x,y) are,

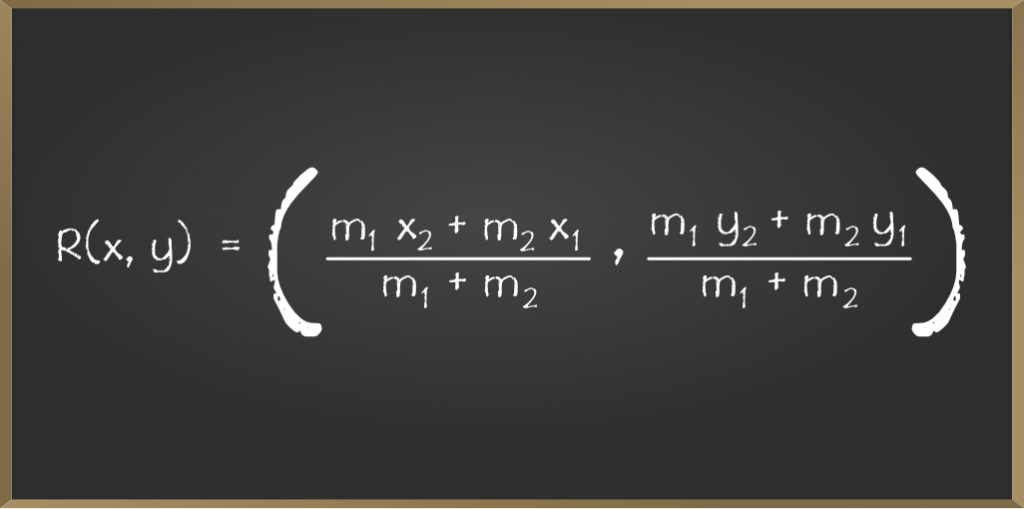
Sample Problems based on the Formula

Problem 1: Calculate the co-ordinates of the point P which divides the line joining A(-3,3) and B(2,-7) in the ratio 2:3

Solution: 

Let (x, y) be th co-ordinates of the point P which divides the line joining A(-3, 3) and B(2, -7) in the ratio 2:3, then

Applying the formulae,

x = (m₁x₂ + m₂x₁) / (m₁ + m₂)

x = {2 x 2 + 3 x (-3)} / (2 + 3)

x = (4 – 9) / 5

x = -5 / 5

x = -1

y = (m₁y₂ + m₂y₁) / (m₁ + m₂)

y = {2 x (-7) + 3 x 3} / (2 + 3)

y = (-14 + 9) / 5

y = -5 / 5

y = -1

Hence the co-ordinates of point P are (-1, -1).

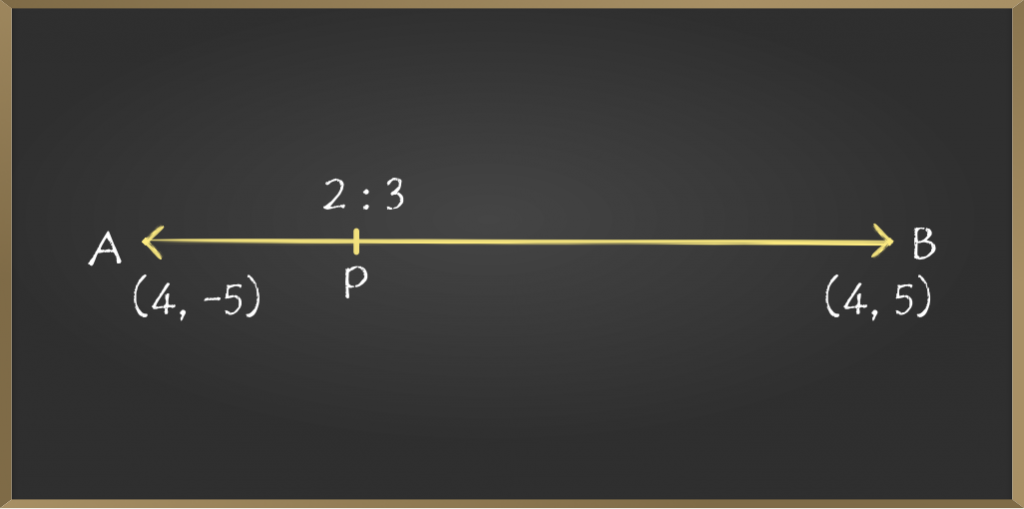
Problem 2: If the line joining the points A(4,-5) and B(4,5) is divided by point P such that AP/AB=2/5, find the coordinates of P.

Solution:

Given AP/AB = 2/5

5AP = 2AB = 2(AP + PB)

3AP = 2PB

AP/PB = 2/3

AP:PB = 2:3

Thus the point P divides the line segment joining the points A(4, -5) and B(4, 5) in the ratio 2:3 internally.

Hence the coordinates of P are,

P_x = (2 x 4 + 3 x 4)/(2 + 3)

    = (8 + 12)/5

    = 20/5 

    = 4

P_y = (2 x 5 + 3 x (-5))/(2 + 3)

   = (10 – 15)/5

   = -5/5

   = -1

Coordinates of P are (4, -1).

Problem 3: In what ratio does the point P(2, -5) divide the line segment joining the points A(-3, 5) and B(4, -9).

Solution:

Let P(2, -5) divide the line segment joining the points A(-3, 3) and B(4, -9) in the ratio k:1, i.e. AP:PB = k:1

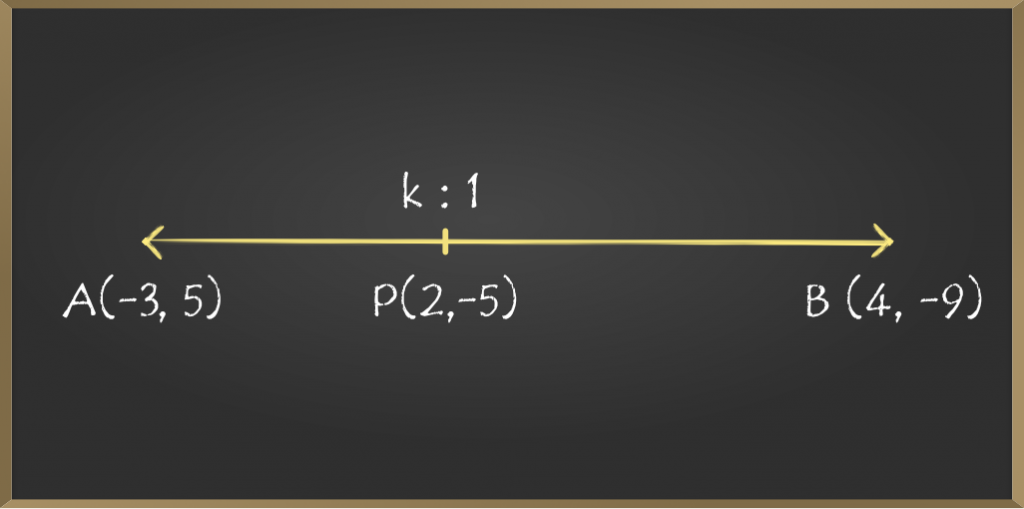
Therefore, Coordinates of P are,

P_x = (k.4 + 1.(-3)) / (k + 1)

P_y = (k.(-9) + 1 x 5)/(k + 1) 

But P is (2, -5) therefore,

(4k – 3) / (k + 1) = 2 and (-9k + 5) / (k + 1) = -5

Solving any of the two equation we get k = 5/2

Therefore, the required ratio is 5/2:1 i.e. 5:2(internally)