Class 11 NCERT Solutions- Chapter 15 Statistics – Miscellaneous Exercise On Chapter 15
Last Updated :
03 Mar, 2021
Question 1. The mean and variance of eight observations are 9 and 9.25, respectively. If six of the observations are 6, 7, 10, 12, 12 and 13, find the remaining two observations.
Solution:
Given,
We are provided with six of the observations 6, 7, 10, 12, 12 and 13.
Let us assume the missing observations to be a and b.
Now, Mean 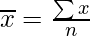 = 9
= 9
9 = (6 + 7 + 10 + 12 + 12 + 13 + a + b)/8
But, 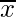
Solving for a + b, we get,
a + b = 12
Also,
Variance 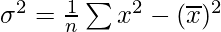
Equating 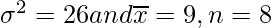
We have,
9.25 = 1/8(62 + 72 + 102 + 122 + 122 + 132 + a2 + b2) – 92
=> 9.25 + 81 = 1/8(36 + 49 + 100 + 144 + 144 + 169 + a2 + b2)
=> 90.25 * 8 = 642 + a2 + b2
=> a2 + b2 = 80
We have, b = 12 – a
On substituting the value,
a2 + (12 – a)2= 80
=> 2a2 – 24a + 64 = 0
On dividing by 2, we get
a2 – 12a + 32 = 0
Therefore, a = 4, 8
Now, for a = 4, b = 8
And, for a = 8, b = 4
Question 2. The mean and variance of 7 observations are 8 and 16, respectively. If five of the observations are 2, 4, 10, 12, 14. Find the remaining two observations.
Solution:
Given,
We are provided with five of the observations 2, 4, 10, 12, 14.
Let us assume the missing observations to be a and b.
Now, Mean
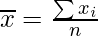
But,
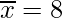
8 = (2 + 4 + 10 + 12 + 14 + a + b)/(7)
Solving for a + b, we get,
a + b = 14
Also,
Variance = 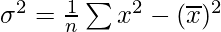
Equating
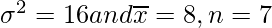
We have,
16 = 1/7(22 + 42 + 102 + 122 + 142 + a2 + b2) – 64
=> 16 + 64 = 1/7(4 + 16 + 100 + 144 + 196 + a2 + b2)
=> 560 = 460 + a2 + b2
=> a2+b2 = 100
We have, b = 14 – a
On substituting the value, we get
a2 + (14 – a)2= 100
=> 2a2 – 28a + 96 = 0
On dividing by 2, we get
a2 – 14a + 48 = 0
=> (a – 8)(a – 6) = 0
Therefore, a = 6, 8
Now, for a = 6, b = 8
And, for a = 8, b = 6
Question 3. The mean and standard deviation of six observations are 8 and 4, respectively. If each observation is multiplied by 3, find the new mean and new standard deviation of the resulting observations.
Solution:
Mean of six observations = 8
Standard deviation of six observations = 4
Let the six observations be x1, x2, x3, x4, x5, x6
Therefore,
Mean of observations, 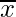 = (x1 + x2 + x3 + x4 + x5 + x6)/6 = 8
= (x1 + x2 + x3 + x4 + x5 + x6)/6 = 8
If each observation is multiplied by 3 and the resultant observations are yi then,
yi = 3xi
xi = (1/3)yi, where i = 1….6
So, new mean
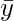 = (y1 + y2 + y3 + y4 + y5 + y6)/6
= (y1 + y2 + y3 + y4 + y5 + y6)/6
= 3(x1 + x2 + x3 + x4 + x5 + x6)/6
= 3 * 8
= 24
Standard Deviation 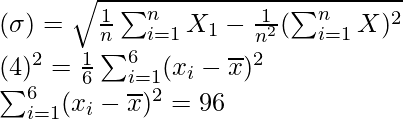
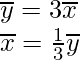
Substituting values, we get,
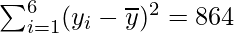
Therefore,
Variance of new observation = 1/6 x 864
= 144
Standard Deviation = √144
= 12
Question 4. Given that x̅ is the mean and σ2 is the variance of n observations x1, x2, …,xn . Prove that the mean and variance of the observations ax1, ax2, ax3, …., axn are ax̅ and a2σ2, respectively, (a ≠ 0).
Solution:
Let us assume the observations to be x1, …xn
Mean of n observations = 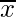
Variance of n observations = 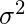
We know,
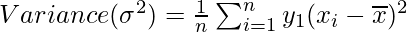
y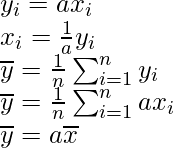
Now,
Mean of the observations, ax1, ax2, …..axn = 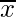
By substituting values, we get,
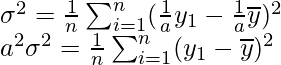
Variance = 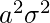
Question 5. The mean and standard deviation of 20 observations are found to be 10 and 2, respectively. On rechecking, it was found that an observation 8 was incorrect. Calculate the correct mean and standard deviation in each of the following cases: (i) If wrong item is omitted. (ii) If it is replaced by 12
Solution:
(i) On omission of wrong item
n = 20
Incorrect mean = 20
Incorrect SD = 2
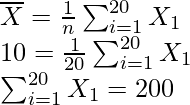
Now,
Incorrect sum of observations = 200
Correct sum of observations = 200 – 8 = 192
Therefore,
Correct mean = Correct sum/19
= 192/19
= 10.1
Standard Deviation 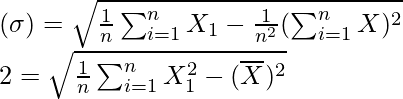
4 = 1/20 Incorrect 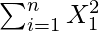 – 100
– 100
Incorrect 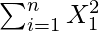 = 2080
= 2080
Therefore,
Correct 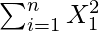 = Incorrect
= Incorrect 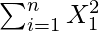 – (8)2
– (8)2
= 2080 – 64
= 2016
Calculating correct standard deviation,
Correct SD = 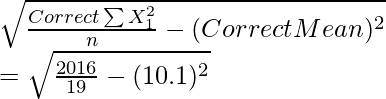
= √(1061.1 – 102.1)
= 2.02
(ii) If it is replaced by 12,
Incorrect sum of observations, n = 200
Correct sum of observations n = 200 – 8 + 12
n = 204
Correct Mean = Correct Sum / 20
= 204/20
= 10.2
Standard Deviation 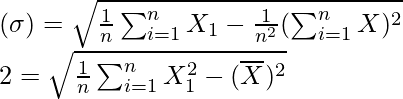
4 = 1/20 Incorrect 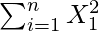 – 100
– 100
Incorrect 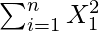 = 2080
= 2080
Therefore, Correct 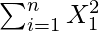 = Incorrect
= Incorrect 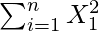 – (8)2 + (12)2
– (8)2 + (12)2
= 2080 – 64 + 144
= 2160
Calculating correct standard deviation,
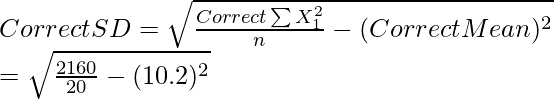
= √(108 – 104.04)
= 1.98
Question 6. The mean and standard deviation of marks obtained by 50 students of a class in three subjects, Mathematics, Physics and Chemistry are given below:
Which of the three subjects shows the highest variability in marks and which shows the lowest?
| Subject | Mathematics | Physics | Chemistry |
| Mean | 42 | 32 | 40.9 |
| Standard Deviation | 12 | 15 | 20 |
Solution:
Given values,
Mean of Mathematics = 42
Standard deviation of Mathematics = 12
Mean of Physics = 32
Standard deviation of physics = 15
Mean of Chemistry = 40.9
Standard deviation of chemistry = 20
Now,
Coefficient of Variation (C.V) = 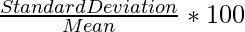
CV for Mathematics = 12/42 x 100 = 28.57
CV for Physics = 15/32 x 100 = 46.87
CV for Chemistry = 20/40.9 x 100 = 48.89
The Highest Variability of the subject is of Chemistry.
Question 7. The mean and standard deviation of a group of 100 observations were found to be 20 and 3, respectively. Later on it was found that three observations were incorrect, which were recorded as 21, 21 and 18. Find the mean and standard deviation if the incorrect observations are omitted.
Solution:
Given:
n = 100
Incorrect mean, (x̅) = 20
Incorrect standard deviation (σ) = 3
Therefore, 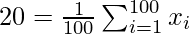
On solving, we get
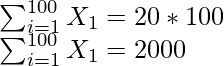
Incorrect sum of observations = 2000
Now, Correct sum of observations = 2000 – 21- 21 – 18
= 1940
Correct mean = Correct Sum / 97
= 1940/97
= 20
Also,
Standard Deviation 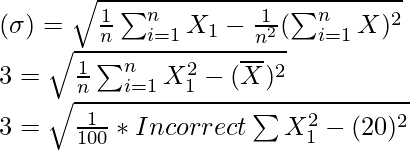
Incorrect 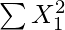 = 100(9 + 400)
= 100(9 + 400)
Incorrect 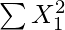 = 40900
= 40900
Correct 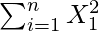 = Incorrect
= Incorrect 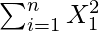 – (21)2 – (21)2 – (18)2
– (21)2 – (21)2 – (18)2
= 40900 – 441 – 441 – 324
= 40900 – 1206
= 39694
Therefore,
Correct S.D = 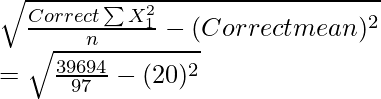
= √(409.216 – 400)
= 3.036
Share your thoughts in the comments
Please Login to comment...