Question 1. Show that the sum of (m + n)th and (m – n)th terms of an A.P. is equal to twice the mth term.
Solution:
Let the first term and common difference of the A.P. be a and d respectively.
(m+n)th term of the A.P. = a+(m+n−1)d
(m−n)th term of the A.P. = a+(m−n−1)d
Thus, L.H.S = a+(m+n−1)d + a+(m−n−1)d
= 2a+(m+n−1+m−n−1)d
= 2a+(2m−2)d
= 2[a+(m−1)d] = 2am
= R.H.S.
Hence, proved.
Question 2. If the sum of three numbers in A.P., is 24 and their product is 440, find the numbers.
Solution:
Let three numbers be a − d, a, a + d.
Sum of these numbers = 24
=> a−d+a+a+d = 24
=> 3a = 24
=> a = 8
Product of these numbers = 440
=> (a−d) a (a+d) = 440
=> (8−d) 8 (8+d) = 440
=> 64−d2 = 55
=> d2 = 9
=> d = ±3
When a=8, d=3, the numbers are
8−3, 8, 8+3, i.e., 5, 8, 11
When a=8, d=−3, the numbers are
8+3, 8, 8-3, i.e., 11, 8, 5
Question 3. Let the sum of n, 2n, 3n terms of an A.P. be S1, S2, and S3, respectively, show that S3 = 3(S2 – S1).
Solution:
According to the question,
S1 = Sum of n terms =n[2a+(n−1)d]/2
S2 = Sum of 2n terms = 2n[2a+(2n−1)d]/2
S3 = Sum of 3n terms = 3n[2a+(3n−1)d]/2
Therefore, R.H.S.= 3(S2−S1)
= ![Rendered by QuickLaTeX.com 3\left[\frac{2n}{2}(2a+(2n−1)d)−\frac{n}{2}(2a+(n−1)d)\right]](https://www.geeksforgeeks.org/wp-content/ql-cache/quicklatex.com-698050eba9bc403455579bc33d3e53a3_l3.png)
= 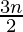 [4a+(4n−2)d−2a−(n−1)d]
[4a+(4n−2)d−2a−(n−1)d]
= 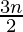 [4a−2a+(4n−2−n+1)d]
[4a−2a+(4n−2−n+1)d]
= 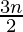 [2a+(3n−1)d]
[2a+(3n−1)d]
= S3 = L.H.S.
Hence, proved.
Question 4. Find the sum of all numbers between 200 and 400 which are divisible by 7.
Solution:
The numbers between 200 and 400 divisible by 7 form an A.P. with common difference(d)=7 and first term (a)=203.
Last term of this series will be, an = 399
We also know, an = a+(n−1)d
=> 399 = 203+(n−1)7
=> (n–1)7 = 196
=> n−1 = 28
=> n = 29
Sum of the series =  (a + an)
(a + an)
= 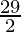 (203+399) = 8729
(203+399) = 8729
Therefore, required sum is 8729.
Question 5. Find the sum of integers from 1 to 100 that are divisible by 2 or 5.
Solution:
Let S1 be the sum of integers from 1 to 100 divisible by 2.
For S1, Common difference(d)=2 and first term(a)=2
Last term will be, an = 100. Therefore,
=> 100 = 2+(n–1)2
=> n = 50
Hence, S1 =  (a + an)
(a + an)
= 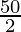 [2+100] = 2550
[2+100] = 2550
Let S2 be the sum of integers from 1 to 100 divisible by 5.
For S2, Common difference(d)=5 and first term(a)=5
Last term will be, an = 100
=> 100 = 5+(n–1)5
=> n = 20
Hence, S2 = 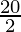 [5+100] = 1050
[5+100] = 1050
Let S3 be the sum of integers from 1 to 100 divisible by both 2 and 5.
For S3, Common difference(d)=10 and first term(a)=10
Last term will be, an = 100
=> 100 = 10+(n–1)10
=> n = 10
Hence, S3 = 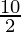 [10+100] = 550
[10+100] = 550
Required sum = S1 + S2 – S3
= 2550 + 1050 – 550
= 3050
Question 6. Find the sum of all two-digit numbers which when divided by 4, yields 1 as remainder.
Solution:
Two-digit numbers, which when divided by 4, yield 1 as remainder are:
13, 17, 21 … 97.
This series forms an A.P. with first term(a) = 13 and common difference(d) = 4.
Let n be the number of terms of the A.P.
We know the nth term of an A.P. is, an= a+(n–1)d
=> 97 = 13 + (n–1)4
=> 4(n–1) = 84
=> n = 22
Now, the sum of n terms of an A.P. is given by,
Sn =  [a + an]
[a + an]
= 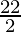 [13+97] = 1210
[13+97] = 1210
Therefore, required sum is 1210.
Question 7. If f is a function satisfying f(x + y) = f(x) f(y) for all x, y ∈ N such that, f(1) = 3 and 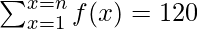 , find the value of n.
, find the value of n.
Solution:
We are given that,
f(x + y) = f(x) × f(y) for all x, y ∈ N … (1)
f(1) = 3
Putting x = y = 1 in (1), we have
f(1 + 1) = f(2) = f(1) f(1) = 3 × 3 = 9
Similarly,
f(1+1+1) = f(3) = f(1+2) = f(1) f(2) = 3 × 9 = 27
And, f(4) = f(1+3) = f(1) f(3) = 3 × 27 = 81
We have, f(1), f(2), f(3).., i.e., 3, 9, 27, …,
This series forms a G.P. with the first term(a)=3 and common ratio(r)=3.
We know that sum of terms in G.P is given by,
Sn = 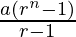
And also it’s given that the sum of terms of the function is 120.
=> 120 = 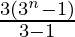
=> 3n–1 = 80
=> n=4
Therefore, number of terms is 4.
Question 8. The sum of some terms of G.P. is 315 whose first term and the common ratio are 5 and 2, respectively. Find the last term and the number of terms.
Solution:
Let the total number of terms be n.
We know that, sum of terms of a G.P. is,
Sn = 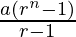
Also, we know that the first term(a) is 5 and common ratio(r) is 2.
=> 315 = 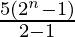
=> 2n–1 = 63
=> n=6
The last term of the G.P = 6th term = ar6-1 = (5)(2)5 = 160
Therefore, last term of the G.P. is 160 and the number of terms is 6.
Question 9. The first term of a G.P. is 1. The sum of the third term and fifth term is 90. Find the common ratio of G.P.
Solution:
Let the first term and common ratio of the G.P. be a and r respectively.
We have, a = 1. So,
a3 = ar2 = r2 …. (1)
a5 = ar4 = r4 .. …. (2)
According to the question, we have
a3 + a5 = 90
From (1) and (2),
r2 + r4 = 90
r4 + r2 – 90 = 0
Solving for r2, we get,
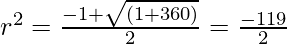
r2 = –10 or r2 = 9
Taking only real roots, we get,
r = ±3
Therefore, the common ratio of the G.P. is ±3.
Question 10. The sum of three numbers in G.P. is 56. If we subtract 1, 7, 21 from these numbers in that order, we obtain an arithmetic progression. Find the numbers.
Solution:
Suppose the three numbers in G.P. are a, ar, and ar2.
According to question, we have
=> a + ar + ar2 = 56
=> a (1 + r + r2) = 56
=> a = 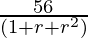 …. (1)
…. (1)
Also, we are given that,
a – 1, ar – 7, ar2 – 21 forms an A.P.
which implies,
=> (ar – 7) – (a – 1) = (ar2 – 21) – (ar – 7)
=> ar – a – 6 = ar2 – ar – 14
=> ar2 – 2ar + a = 8
=> a (r – 1)2 = 8 ….. (2)
Putting value of a from (1) in (2), we get,
=> 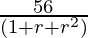 (r – 1)2 = 8
(r – 1)2 = 8
=> 7(r2 – 2r + 1) = 1 + r + r2
=> 6r2 – 15r + 6 = 0
=> (6r – 3) (r – 2) = 0
=> r = 2, 1/2
Now if r = 2, then a = 8 and the three numbers in G.P. are 8, 16, and 32.
If r = 1/2, then a = 32 and the three numbers in G.P. are 32, 16, and 8.
Therefore, in both the cases, the three numbers are 8, 16, and 32.
Question 11. A G.P. consists of an even number of terms. If the sum of all the terms is 5 times the sum of terms occupying odd places, then find its common ratio.
Solution:
Suppose the terms in the G.P. are a1, a2, a3, a4, … a2n.
Number of terms = 2n
According to the question, we have
=> a1 + a2 + a3 + …+ a2n = 5 [a1 + a3 + … + a2n–1]
=> a1 + a2 + a3 + … + a2n – 5 [a1 + a3 + … + a2n–1] = 0
=> a2 + a4 + … + a2n = 4 [a1 + a3 + … + a2n – 1] …. (1)
Now, let the terms of our G.P. be a, ar, ar2, ar3, …..
Using sum of terms of a G.P., equation (1) becomes,
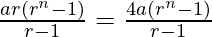
After solving we get,
ar = 4a
r = 4
Thus, the common ratio of the G.P. is 4.
Question 12. The sum of the first four terms of an A.P. is 56. The sum of the last four terms is 112. If its first term is 11, then find the number of terms.
Solution:
Suppose the terms in A.P. are a, a + d, a + 2d, a + 3d, … a + (n – 2) d, a + (n – 1)d.
According to the question, we have,
a = 11,
Sum of first four terms = a + (a + d) + (a + 2d) + (a + 3d) = 4a + 6d,
Sum of last four terms = [a + (n – 4) d] + [a + (n – 3) d] + [a + (n – 2) d] + [a + n – 1) d]
= 4a + (4n – 10) d
Then according to the given condition,
=> 4a + 6d = 56
=> 4(11) + 6d = 56
=> d = 2
Therefore, 4a + (4n –10)d = 112
=> 4(11) + (4n – 10)2 = 112
=> (4n – 10)2 = 68
=> 4n = 44
=> n = 11
Therefore, the number of terms of the A.P. is 11.
Question 13. If, 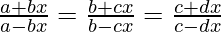 , then show that a, b, c and d are in G.P.
, then show that a, b, c and d are in G.P.
Solution:
We are given,
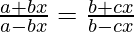
On cross multiplication, we have,
=> (a+bx)(b-cx) = (b+cx)(a-bx)
=> ab–acx+b2x–bcx2 = ab–b2x+acx–bcx2
=> 2b2x = 2acx
=> b2 = ac
=> b/a = c/b ….. (1)
We are also given,
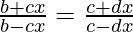
On cross multiplication, we have,
=> (b+cx)(c−dx) = (c+dx)(b−cx)
=> bc−bdx+c2x-cdx2 = bc+bdx−c2x−cdx2
=> 2c2x = 2bdx
=> c2 = bd
=> c/d = d/c ….. (2)
From (1) and (2), we get
b/a = c/b = d/c
Therefore, a, b, c and d are in G.P.
Question 14. Let S be the sum, P the product, and R the sum of reciprocals of n terms in a G.P. Prove that P2Rn = Sn.
Solution:
Suppose the terms in the G.P. are a, ar, ar2, ar3, … arn – 1…
According to the question, we have,
S = a(rn−1)/(r−1) ….. (1)
P = an × r1+2+….+n-1
= an r n(n-1)/2
R = 1/a + 1/ar + 1/ar2 + …. + 1/arn-1
= 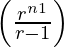 [Tex]\left(\frac{1}{ar^{n-1}}\right)[/Tex]
[Tex]\left(\frac{1}{ar^{n-1}}\right)[/Tex]
So, P2Rn = a2n r n(n-1) = 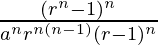
= 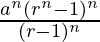
= ![Rendered by QuickLaTeX.com \left[\frac{a(r^n-1)}{r-1}\right]^{n}](https://www.geeksforgeeks.org/wp-content/ql-cache/quicklatex.com-5c6948bdfa8a3ed633010ea67b4a93b3_l3.png)
From (1), we get,
P2Rn = Sn
Hence, proved
Question 15. The pth, qth and rth terms of an A.P. are a, b, c respectively. Show that (q – r) a + (r – p) b + (p – q) c = 0.
Solution:
Let us take t and d to be the first term and the common difference of the A.P. respectively.
The nth term of the A.P. is given by, an = t + (n – 1) d
Therefore,
pth term will be, ap = t+ (p – 1) d = a ….. (1)
qth term will be, aq = t + (q – 1) d = b ….. (2)
rth term will be, ar = t + (r – 1) d = c ….. (3)
On subtracting equation (2) from (1), we get
=> (p – 1 – q + 1) d = a – b
=> (p – q) d = a – b
=> d = 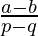 ….. (4)
….. (4)
On subtracting equation (3) from (2), we get
=> (q – 1 – r + 1) d = b – c
=> (q – r) d = b – c
=> d =  .…. (5)
.…. (5)
Equating both the values of d obtained in (4) and (5), we get
=> 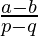 =
= 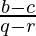
=> (a–b)(q–r) = (b–c)(p–q)
=> bp–cp+cq–aq+ar–br = 0
Rearranging terms, we get,
=> (–aq+ar)+(bp–br)+(–cp+cq) = 0
=> a(q–r)+b(r–p)+c(p–q) = 0
Hence, proved
Question 16. If a(1/b+1/c), b(1/c+1/a), c(1/a+1/b) are in A.P., prove that a, b, c are in A.P.
Solution:
We are given, a(1/b+1/c), b(1/c+1/a), c(1/a+1/b) are in A.P.
=> b(1/c+1/a)–a(1/b+1/c) = c(1/a+1/b)–b(1/c+1/a)
=> 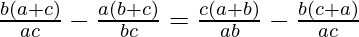
Multiplying both sides by abc and rearranging, we get,
=> b2a–a2b+b2c–a2c = c2a–b2a+c2b–b2c
=> ab(b–a)+c(b2–a2) = a(c2–b2)+bc(c–b)
=> (b–a)(ab+bc+ca) = (c–b)(ab+bc+ca)
=> b–a = c–b
Therefore, a, b and c are in A.P.
Share your thoughts in the comments
Please Login to comment...