Class 11 NCERT Solutions- Chapter 11 Conic Section – Exercise 11.1

In each of the following Exercise 1 to 5, find the equation of the circle with

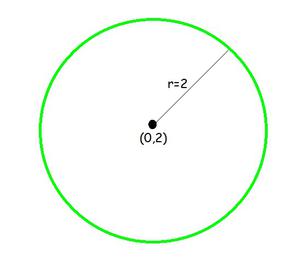
Question 1: Centre (0, 2) and radius 2

Solution:

Given: Centre (0, 2) and radius (r) = 2

The equation of a circle with centre as (h, k) and radius as r is given as (x – h)² + (y – k)² = r²

As, Centre (h, k) = (0, 2) and radius (r) = 2

Thus, the equation of the circle is

(x – 0)² + (y – 2)² = 2² [by using formula (a – b)² = a² – 2ab + b²]

x² + y² + 4 – 4y = 4

x² + y² – 4y = 0

Therefore, the equation of the circle is x² + y² – 4y = 0

Question 2: Centre (–2, 3) and radius 4

Solution:

Given: Centre (-2, 3) and radius (r) = 4

The equation of a circle with centre as (h, k) and radius as r is given as (x – h)² + (y – k)² = r²

As, centre (h, k) = (-2, 3) and radius (r) = 4

Thus, the equation of the circle is

(x + 2)² + (y – 3)² = (4)² [by using formula (a – b)² = a² – 2ab + b²]

x² + 4x + 4 + y² – 6y + 9 = 16

x² + y² + 4x – 6y – 3 = 0

Therefore, the equation of the circle is x² + y² + 4x – 6y – 3 = 0

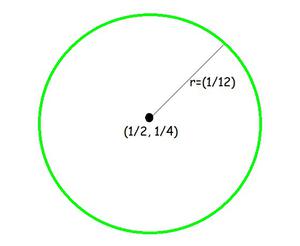
Question 3: Centre (1/2, 1/4) and radius (1/12)

Solution:

Given: Centre (1/2, 1/4) and radius 1/12

The equation of a circle with centre as (h, k) and radius as r is given as (x – h)² + (y – k)² = r²

So, centre (h, k) = (1/2, 1/4) and radius (r) = 1/12

Thus, the equation of the circle is

(x – 1/2)² + (y – 1/4)² = (1/12)² [by using formula (a – b)² = a² – 2ab + b²]

x² – x + 1/4 + y² – y/2 + 1/16 = 1/144

x² – x + 1/4 + y² – y/2 + 1/16 = 1/144

144x² – 144x + 36 + 144y² – 72y + 9 – 1 = 0

144x² – 144x + 144y² – 72y + 44 = 0

36x² + 36x + 36y2 – 18y + 11 = 0

36x² + 36y2 – 36x – 18y + 11= 0

Therefore, the equation of the circle is 36x² + 36y² – 36x – 18y + 11= 0

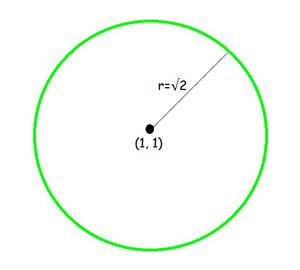
Question 4: Centre (1, 1) and radius √2

Solution:

Given: Centre (1, 1) and radius √2

The equation of a circle with centre as (h, k) and radius as r is given as (x – h)² + (y – k)² = r²

So, centre (h, k) = (1, 1) and radius (r) = √2

Thus, the equation of the circle is

(x-1)² + (y-1)² = (√2)² [by using formula (a – b)² = a² – 2ab + b²]

x² – 2x + 1 + y² -2y + 1 = 2

x² + y² – 2x -2y = 0

Therefore, the equation of the circle is x² + y² – 2x -2y = 0

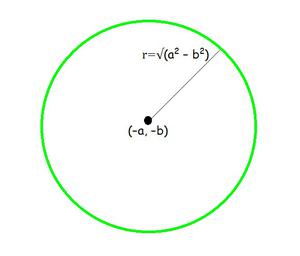
Question 5: Centre (–a, –b) and radius √(a² – b²)

Solution:

Given: Centre (-a, -b) and radius √(a² – b²)

The equation of a circle with centre as (h, k) and radius as r is given as (x – h)² + (y – k)² = r²

So, centre (h, k) = (-a, -b) and radius (r) = √(a² – b²)

Thus, the equation of the circle is

(x + a)² + (y + b)² = (√(a² – b²)²) [by using formula (a + b)² = a² + 2ab + b²]

x² + 2ax + a² + y² + 2by + b² = a² – b²

x² + y² +2ax + 2by + 2b² = 0

Therefore, the equation of the circle is x² + y² +2ax + 2by + 2b² = 0

In each of the following Exercise 6 to 9, find the centre and radius of the circles.

Question 6: (x + 5)² + (y – 3)² = 36

Solution:

Given equation:  (x + 5)² + (y – 3)² = 36

(x – (-5))² + (y – 3)² = 6²

The equation is of the form (x – h)² + (y – k)² = r² where, h = -5, k = 3 and r = 6

Therefore, the centre is (-5, 3) and its radius is 6.

Question 7: x² + y² – 4x – 8y – 45 = 0

Solution:

Given equation: x² + y² – 4x – 8y – 45 = 0.

x² + y² – 4x – 8y – 45 = 0

(x² – 4x) + (y² -8y) = 45

(x² – 2(x) (2) + 2²) + (y² – 2(y) (4) + 4²) – 4 – 16 = 45

(x – 2)² + (y – 4)² = 65

(x – 2)² + (y – 4)² = (√65)²

The equation is of the form (x-h)² +(y-k)² = r² ,where h = 2, k = 4 and r = √65

Therefore, the centre is (2, 4) and its radius is √65.

Question 8: x² + y² – 8x + 10y – 12 = 0

Solution:

Given equation: x² + y² -8x + 10y -12 = 0.

x² + y² – 8x + 10y – 12 = 0

(x² – 8x) + (y² + 10y) = 12

(x² – 2(x) (4) + 4²) + (y² – 2(y) (5) + 5²) – 16 – 25 = 12

(x – 4)² + (y + 5)² = 53

(x – 4)² + (y – (-5))² = (√53)²

The equation is of the form (x-h)² +(y-k)² = r² ,where h = 4, k= -5 and r = √53

Therefore, the centre is (4, -5) and its radius is √53.

Question 9: 2x² + 2y² – x = 0

Solution:

Given equation: 2x² + 2y² – x = 0.

2x² + 2y² – x = 0

(2x² – x) + 2y² = 0

(x² – 2 (x) (1/4) + (1/4)²) + y² – (1/4)² = 0

(x – 1/4)² + (y – 0)² = (1/4)²

The equation is of the form (x-h)² +(y-k)² = r², where, h = 1/4, k = 0, and r = 1/4

Therefore, the centre is (1/4, 0) and its radius is 1/4.

Question 10: Find the equation of the circle passing through the points (4,1) and (6,5) and whose centre is on the line 4x + y = 16.

Solution:

The equation of the circle is (x – h)²+ (y – k)² = r²

As the circle passes through points (4,1) and (6,5)

So, When the circle passes through (4,1)

(4 – h)² + (1 – k)² = r² ……………..(1)

When the circle passes through (6,5)

(6 – h)²+ (5 – k)² = r² ………………(2)

Given that, the centre (h, k) of the circle lies on line 4x + y = 16,

4h + k =16 ………………… (3)

From equation (1) and (2), we get

(4 – h)²+ (1 – k)² =(6 – h)² + (5 – k)²

16 – 8h + h² +1 -2k +k² = 36 -12h +h²+15 – 10k + k²

16 – 8h +1 -2k + 12h -25 -10k

4h +8k = 44

h + 2k = 11 ……………. (4)

Now let us multiply equation (3) by 2, and subtracting it with equation (4), we get

(h + 2k) – 2(4h + k) = 11 – 32

h + 2k – 8h – 2k = -21

-7h = -21

h = 3

Substitute this value of h in equation (4), we get

3 + 2k = 11

2k = 11 – 3

2k = 8

k = 4

We get h = 3 and k = 4

When we substitute the values of h and k in equation (1), we get

(4 – 3)²+ (1 – 4)² = r²

(1)² + (-3)² = r²

1+9 = r²

r = √10

Now, the equation of the circle is,

(x – 3)² + (y – 4)² = (√10)²

x² – 6x + 9 + y² – 8y + 16 =10

x² + y² – 6x – 8y + 15 = 0

Therefore, the equation of the circle is x² + y² – 6x – 8y + 15 = 0

Question 11: Find the equation of the circle passing through the points (2, 3) and (–1, 1) and whose centre is on the line x – 3y – 11 = 0.

Solution:

The equation of the circle is (x – h)² + (y – k)² = r²

As the circle passes through points (2,3) and (-1,1)

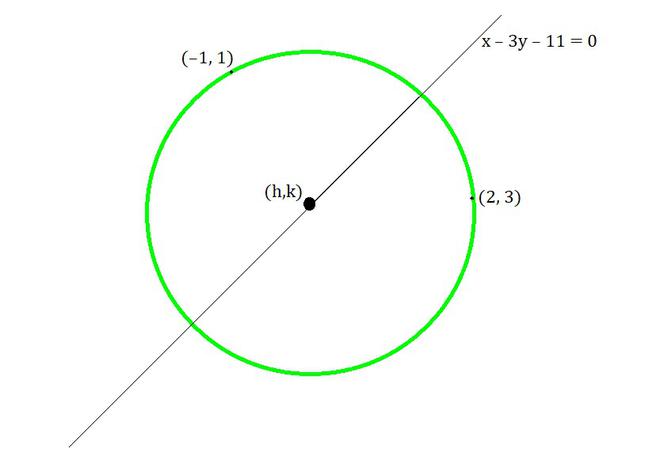
So, When the circle passes through (2,3)

(2 – h)²+ (3 – k)² =r² ……………..(1)

When the circle passes through (-1,1)

(-1 – h)²+ (1– k)² =r² ………………(2)

Given that, the centre (h, k) of the circle lies on line x – 3y – 11= 0,

h – 3k =11 ………………… (3)

From the equation (1) and (2), we get

(2 – h)²+ (3 – k)² =(-1 – h)² + (1 – k)²

4 – 4h + h² +9 -6k +k² = 1 + 2h +h²+1 – 2k + k²

4 – 4h +9 -6k = 1 + 2h + 1 -2k

6h + 4k =11 ……………. (4)

Now let us multiply equation (3) by 6, and subtracting it with equation 4, we get

6h+ 4k – 6(h-3k) = 11 – 66

6h + 4k – 6h + 18k = 11 – 66

22 k = – 55

k = -5/2

Substitute this value of k in equation (4), we get

6h + 4(-5/2) = 11

6h – 10 = 11

6h = 21

h = 21/6

h = 7/2

We get h = 7/2 and k = -5/2

On substituting the values of h and k in equation (1), we get

(2 – 7/2)² + (3 + 5/2)² = r²

[(4-7)/2]² + [(6+5)/2]² = r²

(-3/2)² + (11/2)² = r²

9/4 + 121/4 = r²

130/4 = r²

Now, the equation of the circle is,

(x – 7/2)² + (y + 5/2)² = 130/4

[(2x-7)/2]² + [(2y+5)/2]² = 130/4

4×2 -28x + 49 +4y² + 20y + 25 =130

4x² +4y² -28x + 20y – 56 = 0

4(x² +y² -7x + 5y – 14) = 0

x² + y² – 7x + 5y – 14 = 0

Therefore, the equation of the circle is x² + y² – 7x + 5y – 14 = 0

Question 12: Find the equation of the circle with radius 5 whose centre lies on x-axis and passes through the point (2, 3).

Solution:

The equation of the circle is (x – h)²+ (y – k)² = r²

Given the radius of the circle is 5 and it’s centre lies on the x-axis, k = 0 and r = 5.

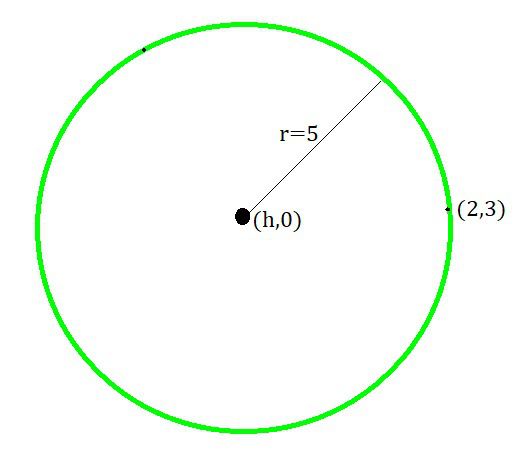
So now, the equation of the circle is (x – h)² + y² = 25.

Also given that the circle passes through the point (2, 3).

Therefore,

(2 – h)²+ 3² = 25

(2 – h)² = 25-9

(2 – h)² = 16

2 – h = ± √16 = ± 4

If 2-h = 4, then h = -2

If 2-h = -4, then h = 6

Now, when h = -2, the equation of the circle is

(x + 2)² + y² = 25

x² + 4x + 4 + y² = 25

x² + y² + 4x – 21 = 0

Now, when h = 6, the equation of the circle is

(x – 6)² + y² = 25

x² -12x + 36 + y² = 25

x² + y² -12x + 11 = 0

Therefore, the equation of the circle is x² + y² – 4x + 21 = 0 and x² + y² -12x + 11 = 0

Question 13: Find the equation of the circle passing through (0,0) and making intercepts a and b on the coordinate axes.

Solution:

The equation of the circle is (x – h)²+ (y – k)² = r²

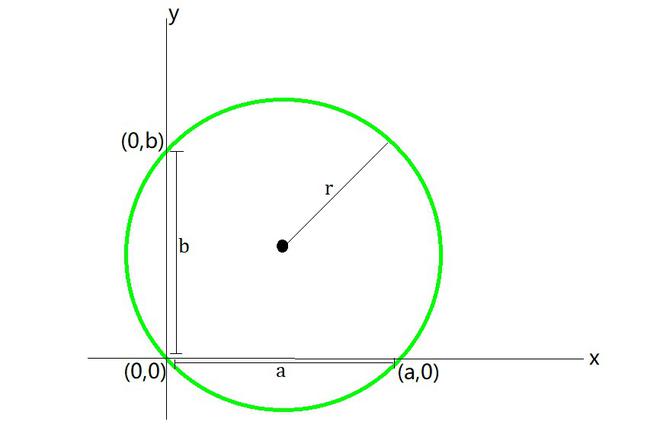
When the circle passes through (0, 0),we get,

(0 – h)²+ (0 – k)² = r²

h² + k² = r²

The equation of the circle is (x – h)² + (y – k)² = h² + k².

Given that the circle intercepts points a and b on the coordinate axes.

Since, the circle passes through points (a, 0) and (0, b).

So the equations are, 

(a – h)²+ (0 – k)² = h² +k² ……………..(1)

(0 – h)²+ (b– k)² = h² +k² ………………(2)

From equation (1), we get

a² – 2ah + h² + k² = h² + k²

a² – 2ah = 0

a(a – 2h) =0

a = 0 or (a -2h) = 0

As, a ≠ 0; hence, (a -2h) = 0

h = a/2

From equation (2), we get

h² – 2bk + k² + b² = h² + k²

b² – 2bk = 0

b(b– 2k) = 0

b= 0 or (b-2k) =0

As, a ≠ 0; hence, (b -2k) = 0

k = b/2

Now, substituting the value of h and k, we get

(x – a/2)² + (y – b/4)² = (a/2)² + (b/2)²

[(2x-a)/2]² + [(2y+b)/2]² = (a2 + b2)/4

4x² – 4ax + a² +4y² – 4by + b² = a² + b²

4x² + 4y² -4ax – 4by = 0

4(x² +y² -7x + 5y – 14) = 0

x² + y² – ax – by = 0

Therefore, the equation of the circle is x² + y² – ax – by = 0

Question 14: Find the equation of a circle with centre (2,2) and passes through the point (4,5).

Solution:

Given the centre of the circle as (h, k) = (2,2)

Also given that the circle passes through the point (4,5), 

the radius (r) of the circle is the distance between the points (2,2) and (4,5).

r = √[(2-4)² + (2-5)²]

= √[(-2)² + (-3)²]

= √[4+9]

= √13

Now, the equation of the circle is

(x– h)²+ (y – k)² = r²

(x –h)² + (y – k)² = (√13)²

(x –2)² + (y – 2)² = (√13)²

x² – 4x + 4 + y² – 4y + 4 = 13

x² + y² – 4x – 4y = 5

Therefore, the equation of the circle is x² + y² – 4x – 4y = 5

Question 15: Does the point (–2.5, 3.5) lie inside, outside or on the circle x² + y² = 25?

Solution:

Given equation of the circle is x² +y² = 25.

x² + y² = 25

(x – 0)² + (y – 0)² = 5²

The equation is of the form (x – h)² + (y – k)² = r² ,where, h = 0, k = 0 and r = 5.

Now the distance between the point (-2.5, 3.5) and the centre (0,0) is

= √[(-2.5 – 0)² + (-3.5 – 0)²]

= √(6.25 + 12.25)

= √18.5

= 4.3 [which is < 5]

Since, the distance between the point (-2.5, -3.5) and the centre (0, 0) of the circle is less than the radius of the circle.

Therefore, the point (-2.5, -3.5) lies inside the circle.