Limits have been really useful in the field of calculus, they become a solid foundation for defining many concepts like continuity, differentiability, integrals, and derivatives. These concepts further help us analyze a lot of functions and their behavior in calculus. Limits have been the foundation for almost all the concepts in calculus. Thus, it becomes essential to learn how to calculate the limits for different types of functions and how to deal with the indeterminate forms of the limits. Let’s see different methods which help us to calculate the limits for complex functions and expressions.
Limits
Consider a function f(x), and a point x = c, the limit at this point is defined as the value the function seems to take on when one approaches this value of x = c from either the left-hand side or the right-hand side. Limit of the function at a particular point is defined as,
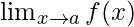
Most of the limits can be calculated by simple substitution of the point x = a in the function. This is called the direct substitution method. Sometimes while calculating the limits, we might come across some expressions which are undefined. These are indeterminate forms of the limit.
For example, let’s consider a function f(x) =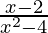 . The goal is to find the limit of this function at x = 2.
. The goal is to find the limit of this function at x = 2.
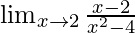
Notice that through direct substitution, this limit takes the form 0/0. This is undefined and it is called indeterminate form. Similarly, ∞/∞, 1∞ are also called indeterminate forms. To solve such forms, a number of strategies are employed.
Strategies of Solving Limits
There are several strategies and methods used to find the limits of the function. Which method will be used for which function depends on several factors. For example, type of function(trigonometric, exponential, polynomial, etc), the indeterminate form encountered(∞/∞, 1∞, 0/0, and so on). There are no set rules for these things, one should practice, and it comes with experience as one goes on solving limits for different kinds of functions. Let’s see some strategies to solve limits.
Direct Substitution
Many of the limits can be evaluated by simply substituting the value of the point in the function. The necessary condition for using this approach is function should be continuous, and the limit should not give some indeterminate form as the output.
Example: Consider a function f(x) = x2 + 4x + 13. Find 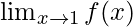
Solution:
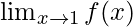
⇒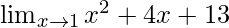
⇒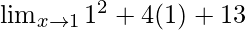
⇒1 +4 + 13
⇒ 18
Factoring and Cancelling
Sometimes in some functions, when using the substitution method, the limit takes the form of 0/0. Often in these cases, there are some common factors in numerator and denominator that can be factorized and cancelled.
Example: Consider a function f(x) = 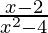 . Find
. Find 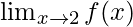
Solution:
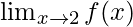
⇒
Using substitution method,
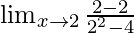
⇒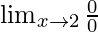
Now using the factoring and canceling method.
⇒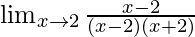
⇒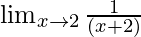
⇒ 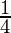
Special Case with Sine Function
Sometimes while evaluating the 0/0 form, if the sine function is present. This identity, 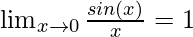 comes in handy.
comes in handy.
Example: Consider a function f(x) = 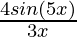 . Find
. Find 
Solution:
This limit is of the form 0/0.

⇒
⇒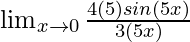
⇒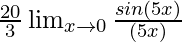
Using the identity mentioned above,
⇒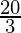
Multiplying by Reciprocal of the Highest Power
In the case of ∞/∞ form in the limits and polynomial functions. This method can be used to solve the limit. In this case, both numerator and denominator are divided by the highest power of x appearing in the function.
Example: Consider a function f(x) = 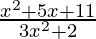 . Find
. Find 
Solution:
This limit is of the form ∞/∞.

⇒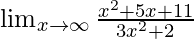
⇒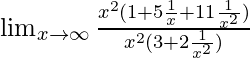
⇒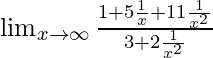
⇒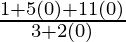
⇒
L’Hospital Rule
This rule is useful for indeterminate forms such as 0/0 or ∞/∞. There is no boundation on the class of functions on which it can be applied. It can be applied for any type of function which evaluates in indeterminate forms with the substitution method. In this rule, the numerator and denominator are differentiated until the limit comes in the determinate form.
Example: The function mentioned above, f(x) = 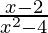 . Find out the
. Find out the  using L’Hospital rule.
using L’Hospital rule.
Solution:


Differentiating the numerator and denominator.
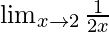
Now this limit is not in indeterminate form,

⇒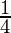
Let’s look at some more examples of these methods.
Sample Problems
Question 1: Consider a function f(x) = x3 + 4x2+ 1. Find 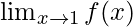
Solution:
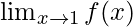
⇒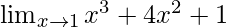
⇒
⇒1 + 4 + 1
⇒ 6
Question 2: Consider a function f(x) = 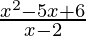 . Find
. Find 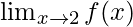
Solution:
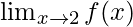
⇒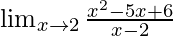
Using substitution method,
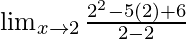
⇒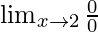
Now using the factoring and canceling method.
⇒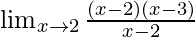
⇒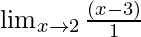
⇒ -1
Question 3: Consider a function f(x) =  . Find
. Find 
Solution:
This limit is of the form ∞/∞.

⇒
⇒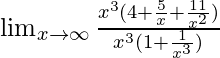
⇒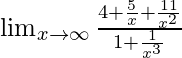
⇒ 4
Question 4: The function mentioned above, f(x) = 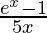 . Find out the
. Find out the 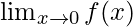 using L’Hospital rule.
using L’Hospital rule.
Solution:
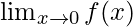
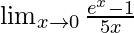
Differentiating the numerator and denominator.
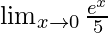
Now this limit is not in indeterminate form,
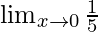
⇒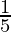
Question 5: Consider a function f(x) = 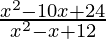 . Find
. Find 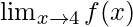
Solution:
This limit is of the form ∞/∞.

⇒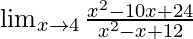
⇒
⇒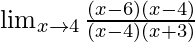
⇒ 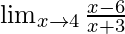
⇒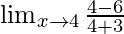
⇒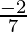
Question 6: Consider a function f(x) = 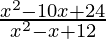 . Find
. Find 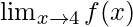
Solution:
This limit is of the form ∞/∞.

⇒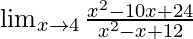
⇒
⇒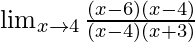
⇒ 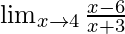
⇒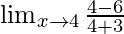
⇒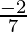
Question 7: Consider a function f(x) = 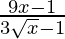 . Find
. Find 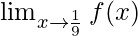
Solution:
This limit is of the form ∞/∞.
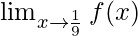
⇒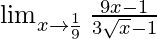
⇒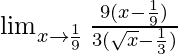
⇒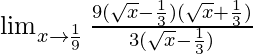
⇒ 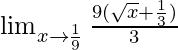
⇒
⇒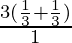
⇒ 2
Share your thoughts in the comments
Please Login to comment...