Quadratic Equations: Definition, Formulas, and Examples

Quadratic Equations are the polynomial equations of degree two. Quadratic Equations are very common in mathematics and their family of curve represent the conic section. The word quadratic is derived from the Latin word “quadratus” which means square. Thus, the name quadratic equation implies that these are equations with square terms.

Quadratic Equations are used to define various things they define the path and motion of multiple objects. Suppose we throw a stone in the sky then its trajectory is defined using the quadratic equations. As quadratic equations are the equation with two degrees they can have a maximum of two roots. The roots of quadratic equations are the values that satisfy the quadratic equation.

Lets’s learn more about quadratic equation formula, their roots, and how to solve quadratic equations in detail in this article.

Table of Content

• Definition
• Roots of a Quadratic Equation
• Quadratic Formula
• Nature of Roots
• Sum and Product of Roots
• Quadratic Equation Formulas
• How to Solve Quadratic Equations
  • Factorization Method
  • Completing Squares Method
  • Quadratic Formula
  • Graph Method to Find the Roots
• Quadratic Equations Having Common Roots
• Maximum and Minimum Value
• Solved Examples
• FAQs

What is Quadratic Equation?

An algebraic equation of the second degree is called the quadratic equation. The standard form of the quadratic equation is ax² + bx + c = 0. The most important condition for a quadratic equation to exist is that the coefficient of the highest degree term i.e. the coefficient of ‘x²‘ can never be zero. In standard form, we can imply that a  ≠ 0. Quadratic Equation is an important topic for Class 10 Students.

Quadratic Equation Definition

Quadratic equation is a type of polynomial equation of the second degree, which means it contains a variable raised to the power of 2 (squared) as its highest exponent. The general form of a quadratic equation is:

ax² + bx + c = 0

Related Resources,

Quadratic Equation Class 10

Some examples of quadratic equations are,

• 3x² – 11x + 23 = 0
• 5x² = 0
• 11x² -13 = 0

Standard Form of Quadratic Equation

The standard form of the Quadratic Equation is,

ax² + bx + c = 0

Where,

• x is the variable of the equation,
• a, b, and c are real numbers and constants and a ≠ 0.

In general, any second-degree polynomial P(x), when put like P(x) = 0 represents a quadratic equation.

Example: Rahul and Ravi together have 45 candies. Both of them lost 5 candies each. The product of number of candies both of them have now is 124.  We are asked to find out the number of candies each one had in the beginning. Formulate a quadratic equation for this problem. 

Solution:

Let’s say Rahul had “x” candies. 

Then Ravi must have “45 – x” candies because both of them had 45 candies. 

Now after losing the candies, we are given that product of the number of candies they have is 124, i.e.

x(45 – x) = 124

⇒ 45x – x² = 124 

⇒ x² – 45x + 124 = 0

This is the required quadratic equation.

Roots of a Quadratic Equation

The values of the x_o which satisfies the quadratic equation q(x) are called the roots of the quadratic equation. This implies that for any x_o if q(x) = 0. Then x_o is the root of the q(x).

For example, the roots of the quadratic equation q(x): 3x² – 10x – 8 = 0 are x = -2/3 and x = 4 as

For x = -2/3,

q(-2/3) = 3(-2/3)² – 10(-2/3) – 8 
⇒ q(-2/3)  = 4/3 + 20/3 – 8 
⇒ q(-2/3) = 0

For x = 4, 

q(4) = 3(4)² – 10(4) – 8
⇒ q(4)  = 32 – 40 -8
⇒ q(4)  = 0

We use various methods to find the solution to the quadratic equation. Quadratic Formula is the most common way to solve quadratic equations which is discussed below in the article.

Note: The quadratic equation is a two degrees polynomial i.e., it can have a maximum of 2 roots.

Quadratic Formula

The easiest and most efficient way to calculate the roots of the quadratic equation is by using the quadratic formula. It provides the roots of the quadratic equation in the least steps. Also, not all the methods of solving quadratic equations work on all types of equations, but quadratic formulas can be used to solve any type of quadratic equation.

The quadratic formula to solve the general quadratic equation ax² + bx + c = 0 is,

Steps for using Quadratic Formula

Follow the steps to find the roots of the quadratic equation using the Quadratic Formula,

Step 1: Arrange the given quadratic equation in standard form.

Step 2: Compare the given equation with the standard quadratic equation to find the values of a, b and c.

Step 3: Use the quadratic formula, x = [-b ± √(b² – 4ac)]/2a

Step 4: Simplify the value obtained in the above step to get the roots of the quadratic equation.

Example: Find the roots of the quadratic equation x² – 5x – 6 = 0

Solution:

Given equation, x² – 5x – 6 = 0

comparing with ax² + bx + c = 0 we get,

a = 1, b = -5, c = -6

Using x = [-b ± √(b² – 4ac)]/2a

x = [-(-5) ± √(-5)² – 4(1)(-6)] / 2(1)

⇒ x = [5 ± √(25 + 24)] / 2

⇒ x = [5 ± √(49)] / 2

Taking Positive Sign,
 x = (5 + 7) / 2 = 12 /2 = 6

OR

Taking Negative Sign
x = (5 – 7) / 2 = -2 /2 = -1

Thus, the roots of the equation x² – 5x – 6 = 0 are x = 6 and x = -1

Proof of Quadratic Formula

Take the standard quadratic equation as, ax² + bx + c = 0, where a ≠ 0 now,

ax² + bx = -c 

x² + bx/a = -c/a

Completing the perfect square on the left-hand side we get,

x²+ bx/a + (b/2a)² = -c/a + (b/2a)²

⇒ (x + b/2a)² = -c/a + b²/4a²

⇒ (x + b/2a⁾² = (b² – 4ac)/4a²

Taking square root on both sides we get,

x + b/2a = ±√(b² – 4ac)/2a

x = [-b ± √(b² – 4ac)]/2a

Thus, the quadratic formula is calculated.

Nature of Roots of Quadratic Equation

We can easily find the nature of the roots of the quadratic equations without actually finding the roots. Generally, we represent the roots of quadratic equations with α and β symbols.

We use the concept of discriminant to find the nature of the roots. The discriminant of the quadratic equation ax² + bx + c = 0 is calculated using the formula,

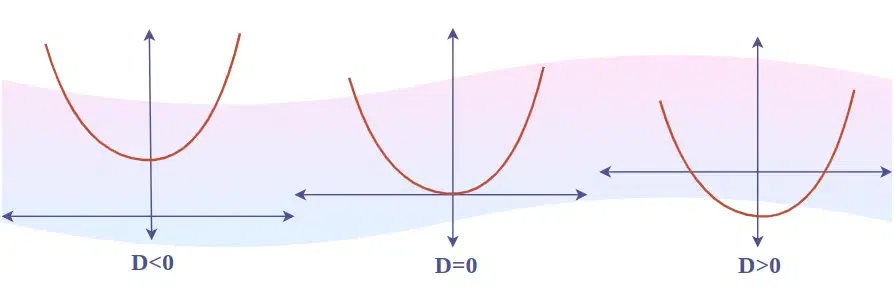
(Discriminant) D = b² – 4ac

Using discriminant we can find the nature of the root as,

• If D > 0, the roots of the quadratic equation are real and distinct.
• If D = 0, the roots of the quadratic equation are real and equal.
• if D < 0, the roots of the quadratic equation do not exist or the roots are imaginary.

Sum and Product of Roots of Quadratic Equation

For any given quadratic equation we can easily find the sum and product of the roots of the quadratic equation without actually calculating the roots of the quadratic equation.

For the given quadratic equation ax² + bx + c = 0 the sum and the product of the roots are found with the help of the coefficient of x², coefficient of x, and the constant term. For example, if the given quadratic equation is ax² + bx + c = 0, then the sum and product of the roots are given using the formula,

• Sum of Roots: α + β = -b/a = -Coefficient of x/ Coefficient of x²
• Product of Roots: αβ = c/a = Constant term/ Coefficient of x²

Example: Find the sum and the product of the roots of equation 2x² + 5x + 3 = 0.

Solution:

Given quadratic equation, 2x² + 5x + 3  = 0

Comparing with, ax² + bx + c = 0

We get, a = 2, b = 5, c =3

• Sum of Roots = α + β = -b/a = -5/2
• Product of Roots = αβ = c/a = 3/2

Writing Quadratic Equations with Roots

If the roots of the quadratic equation α, and β are given then we can easily write the quadratic equation by using the formula,

(x – α)(x – β) = 0

Example: Find the quadratic equation whose roots are, 1 and 2.

Solution:

Given, α = 1 and β = 2

Then the required quadratic equation is,

(x – α)(x – β) = 0

⇒ (x – 1)(x – 2) = 0

⇒ x² – 2x -x + 2 = 0

⇒ x² – 3x + 2 = 0

This is the required quadratic equation.

We can also find the quadratic equation if the sum and the product of the quadratic equation are given. Suppose the sum (S) and the product (P) of the quadratic equation are (α + β) and αβ respectively. Then the quadratic equation is given using the formula,

• x² – (Sum)x + (Product) = 0
• x² – (α + β)x + αβ = 0

Example: Find the quadratic equation whose sum of the roots is, 3 and the product of the root are 2.

Solution:

Given, α + β = 3 and αβ = 2

Then the required quadratic equation is,

x² – (α + β)x + αβ = 0

⇒ x² – 3x + 2 = 0

This is the required quadratic equation.

Formulas Related to Quadratic Equations

Various formulas are used for solving quadratic equations and finding the values and nature of the roots. Some of the important formulas of the quadratic equation are,

• The standard form of the quadratic equation is ax² + bx + c = 0
• Quadratic formula used for finding the roots of the quadratic equation is x = [-b ± √(b² – 4ac)]/2a
• Discriminant of the quadratic equation is found using the formula D = b² – 4ac
  • If D > 0 the roots are real and distinct.
  • If D = 0 the roots are real and equal.
  • If D < 0 the real roots do not exist, or the roots are imaginary.
• Sum of the roots of a quadratic equation is given by α + β = -b/a.
• Product of the Root of the quadratic equation is given by αβ = c/a.
• If the sum (α + β) and the product (αβ) of the quadratic equation then the quadratic equation is given using x² – (α + β)x + αβ = 0.

How to Solve Quadratic Equations

Let’s assume a quadratic equation P(x) = 0. The points which satisfy this equation are called solutions or zeros of this quadratic equation. There are three types of methods to find the solution of a quadratic equation: 

• Factorization Method
• Completing Squares Method
• Shree Dharacharya or Quadratic Formula
• Graph Method to Find the Roots

Let’s look at all these methods of finding the roots of the quadratic equations one by one through examples. 

Factorization Method of Solving Quadratic Equations

A quadratic equation can be considered a factor of two terms. Like ax² + bx + c = 0 can be written as (x – x₁)(x – x₂) = 0 where x₁ and x₂ are roots of quadratic equation.

Steps of Solving Quadratic Equations Using Factorization

We can use the following steps to solve quadratic equations using factorization:

Step 1: Find two numbers such that the product of the numbers is ‘ac’ and the sum is ‘b’.

Step 2: Then write x coefficient as the sum of these two numbers and split them such that you get two terms for x.

Step 3: Factor the first two as a group and the last two terms as another group.

Step 4: Take common factors from these and on equating the two expressions with zero after taking common factors and rearranging the equation we get the roots.

Let’s consider an example of this for better understanding.

Example: Find out the solutions of the given quadratic equation using the factorization method. 

2x² – 3x + 1 = 0

Solution:

Given, 2x² – 3x + 1 = 0

⇒ 2x² – 2x – x + 1 = 0

⇒ 2x(x – 1) – 1(x -1) = 0

⇒ (2x – 1)(x-1) = 0

Now this equation is zero when either of these two terms or both of these terms are zero

So, 

Putting 2x – 1 = 0, we get x = 1/2

Similarly, x – 1 = 0, we get x = 1 

Thus, we get two roots x = 1 and 1/2

Method of Completing the Square

Any equation ax² + bx + c = 0 can be converted in the form (x + m)² – n² = 0. After this take the square roots and get the roots of the equation. Completing the square is just a way to readjust the given quadratic equation in such a way that they come in the form of squares. 

Let’s see this through an example.

Example: Find the root of the given equation through complete the square method. 

x² + 4x – 5 = 0

Solution:

Given, x² + 4x – 5 = 0 

Solving by Completing Square Method

x² + 4x – 5 = 0

⇒ x² + 4x + 4 – 9 = 0

⇒ (x + 2)² – 3² = 0

⇒ (x + 2)² = 3²

Taking square root both sides, 

x + 2 = 3 and x + 2 = -3 

⇒ x = 3 -2 and x = -3 -2

⇒ x = 1 and x = -5

Learn more about Completing Square Method

Quadratic Formula of Solving Quadratic Equations

This formula says, for a quadratic equation in general form, ax² + bx + c = 0 If b² – 4ac > 0, 

Then roots are given by 

Example: Find the roots of equation 3x² – 5x + 2 = 0.

Solution: 

For finding out the roots using Shree Dharacharya formula, 

We need to check If b² – 4ac > 0,

In this particular equation, a = 3, b = -5 and c = 2. 

b² – 4ac = (-5)² – 4(3)(2) = 25 – 24 = 1 > 0 

Thus, the roots of the quadratic equation exist, which are

Graphical Solution of Quadratic Equation

Suppose the general form of the quadratic equation is ax² + bx + c = 0, where a ≠ 0. The quadratic equation is a polynomial equation of 2 degrees so it comes under the conic section. Further simplifying the standard form of quadratic equation,

 y = ax² + bx + c 

⇒ y = a[(x + b/2a)² – (D/4a²)]

⇒ y – D/4a = a[(x + b/2a)²]

This resembles a parabola and we can draw its curve easily the points where this curve cut the x-axis are the solution of the quadratic equation.

Learn more about Solving Quadratic Equations

Quadratic Equations Having Common Roots

We can have two quadratic equations which have the same roots. Suppose two quadratic equations are a₁x² + b₁x + c₁ = 0, and a₂x² + b₂x + c₂ = 0, then these equation have common roots if (a₁b₂ – a₂b₁) (b₁c₂ – b₂c₁) = (a₂c₁ – a1c₂)²

Let’s consider an example based on above mentioned condition.

Example: Check whether the equation 3x² + 7x – 6 = 0  and the equation 6x² + 14x – 12 = 0 have common roots or not.

Solution:

Given equations, 3x² + 7x – 6 = 0 and 6x² + 14x – 12 = 0

Comparing with a₁x² + b₁x + c₁ = 0 and a₂x² + b₂x + c₂ = 0 we get,

a₁ = 3, b₁ = 7 and c₁ = -6

a₂ = 6, b₂ = 14 and c₂ = -12

Using the above condition we get,

(a₁b₂ – a₂b₁) (b₁c₂ – b₂c₁) = (a₂c₁ – a1c₂)²

⇒ (42 – 42)(-84  + 84) = (-36+36)²

⇒ 0 = 0

Thus, the above equations have common roots.

Maximum and Minimum Value of Quadratic Equation

For any quadratic equation q(x) = ax² + bx + c the maximum and minimum values of the quadratic can easily be calculated. We know that we can easily plot a graph of the quadratic equations and it comes out to be a parabola.

Now, performing the necessary calculation we can state that,

• If a > 0, q(x) is minimum at x = -b/2a
• If a < 0, q(x) is maximum at x = -b/2a

Thus we can easily get the range of the quadratic equation.

• When a > 0, Range of q(x) is [q(-b/2a), ∞)
• When a < 0, Range of q(x) is (-∞, q(-b/2a)]

Applications of Quadratic Equations

Quadratic Equation can be used to various real life problems whose equations when formed is of degree two. The applications of Quadratic Equations are mentioned below:

• Quadratic Equation is used to solve questions where the curve is parabolic in nature.
• Quadratic Equations are used to solve word problems related to area and surface area.
• Quadratic Equation is used to solve questions of Projectile Motion
• Quadratic Equation is used to solve problems of Speed, Distance and Time.

Related Resources,

• Solving Cubic Equation
• Algebraic Expression
• Factorization of Polynomials

Quadratic Equation Examples

Example 1: Check whether the following equation is a quadratic equation or not.  (x – 2)(x + 1) = (x – 1)(x + 3) 

Solution:

We know that a quadratic equation must be of degree 2. 

Let’s simplify and check the given equation. 

 (x – 2)(x + 1) = (x – 1)(x + 3)

⇒ x² + x – 2x – 2 = x² + 3x – x – 3

⇒ x² – x – 2 = x² + 2x – 3

⇒ -x – 2 = 2x – 3 

⇒ -3x + 1 = 0  

This equation is of degree 1. Thus, it cannot be a quadratic equation. 

Example 2: Find the roots of the following quadratic equation using the factorization method. 

2x² – x – 6 = 0

Solution: 

Given Quadratic Equation,

2x² – x – 6 = 0
⇒ 2x² – 4x. +3x – 6 = 0
⇒ 2x (x – 2) +3(x – 2) = 0 
⇒ (2x + 3) (x – 2) = 0 

Now, 

2x + 3 = 0 
x = -3/2

OR

x – 2 = 0 
x = 2

Thus, this equation has roots x = 2 and -3/2

Example 3: Find the root of the given equation through complete the square method. 

x² + 6x + 9 = 0

Solution:

Given, x² + 6x + 9 = 0

⇒ x² + 6x + 9 = 0
⇒ x² + 2(3x) + 3² = 0
⇒ (x + 3)² = 0 

Taking square root, 

x + 3 = 0 
x = – 3

x = -3,-3

Example 4: Find the roots of the equation x + 1/x = 3

Solution:

Given Equation,

x + 1/x = 3

Simplify the above equation and using quadratic formula,

(x² + 1)/x = 3
x² + 1 = 3x
x² – 3x + 1 = 0

Comparing with ax² + bx + c = 0

a = 1, b = -3 and c = 1

Now, Checking if b² – 4ac > 0

b² – 4ac  = 9 – 4(1)(1)  = 5 > 0 

Applying Quadratic Formula,

Example 5: Find the quadratic equation having the roots 4 and 9 respectively.

Solution:

The quadratic equation having the roots α, β, is (x – α)(x – β) = 0

Given,

α = 4, and β = 9

Therefore the required quadratic equation is,

(x – 4)(x – 9) = 0

x² – 9x – 4x + 36 = 0

x² – 13x + 36 = 0

Thus, the required quadratic equation is x² – 13x + 36 = 0

Example 6: The quad equation 3x² + 5x + 9 = 0 has roots α, and β. Find the quadratic equation having the roots 1/α, and 1/β.

Solution:

Given equation 3x² + 5x + 9 = 0

Comparing with ax² + bx + c = 0

a = 3, b = 5 and c = 9

α + β = -b/a = -5/3

αβ = c/a = 9/3 = 3

Roots of the new equation are 1/α and 1/β.

Sum of Roots = 1/α + 1/β = (α + β)/α β = (-5/3)×(1/3) = -5/9

Product of Roots = 1/α β = 1/3

Thus, the required quadratic equation is,

x² – Sumx + Product = 0

x² – (-5/9)x + 1/3 = 0

Simplifying,

9x² + 5x + 3 = 0

Quadratic Equation Questions

Q1. Check if √3 and -√3 are are the roots of the Quadratic Equation x² + √3x – 6 = 0.

Q2. Find the Roots of the Quadratic Equation 6x² – 31x + 40 = 0 by Factorization Method.

Q3. Find the Roots of the Quadratic Equation x² + 12x = -35 by Completing the Square Method.

Q4. Find the Roots of the Quadratic Equation 4 – 11x = 3×2 by Sri Dharcharya Formula.

Q5. Ram’s Father is twice as old as Ram. Four Years later the product of their ages is 120. Find their present ages.

FAQs on Quadratic Equation

1. What is a Quadratic Equation?

A polynomial equation of the second degree in one variable is called the quadratic equation. The general form of the quadratic equation is ax² + bx + c = 0. Here, a and b are the coefficients of x² and x terms respectively, and c is the constant term.

2. What is the Quadratic Formula?

The general formula to solve a quadratic equation of the form ax² + bx + c = 0 is 

x = [-b ± √(b² – 4ac)]/2a

This is called the quadratic formula.

3. What is Discriminant of Quadratic Equation?

For any quadratic equation of the form ax² + bx + c = 0, we calculate the value b² – 4ac this is called the discriminant of the quadratic equation. It is denoted by D. It is used to tell the nature of the roots of the quadratic equation.

4. What are Some Real-Life Applications of Quadratic Equations?

Quadratic Equations are highly used in various scenarios some of their real-life application are,

• They find or equate the price of the commodity with their demand.
• Quadratic equations are used to determine the rate and efficiency of the various machines.
• They are used to solve speed and distance problems.
• They are used in navigational systems and the calculation of the position of objects in space.

5. How to Find the Value of the Discriminant?

The general form of the quadratic equation is ax² + bx + c = 0 and its discriminant is calculated using the formula, D = b² – 4ac where D is the discriminant of the equation.

6. What are the 3 Quadratic Equations or Formulas?

The three quadratic equations are,

• Standard Form: y = ax² + bx + c
• Factored Form: y = (ax + c)(bx + d)
• Vertex Form: y = a(x + b)² + c

7. What is another name for Quadratic Equation?

As the quadratic equation uses the variable x along with x² it is also called as the polynomial equation of two degrees.

8. What is Biqudratic Equation?

The equation with power or degree four is called a biquadratic equation. The general form of the biquadratic equation is,

ax⁴ + bx³ + cx² + dx + e = 0

9. What is Shri Dharacharya Formula?

Quadratic formula is also called as Shri Dharacharya Formula. For any quadratic equation ax² + bx + c = 0 we can find its root using the Dharacharya formula as x = [-b ± √(b² – 4ac)]/2a

10. What are the Different ways of Solving a Quadratic Equation?

There are four ways of solving a Quadratic Equation. These are Factorization Method, Graphing Method, Completing Square Method and Quadratic Formula Method. All these four methods of solving a Quadratic Equation has been discussed in the article under their headings.

11. How is Quadratic Equation different from Linear Equation?

Quadratic Equation differs from Linear Equation in the manner that Quadratic Equation has degree two and can have maximum of two solutions while Linear Equation has degree one and can have only one solution at maximum.

12. What is Quadratic Equation Calculator?

Quadratic Equation Calculator is an online calculator that is used to calculate the roots of a Quadratic Equation. In such calculator you need to input the values of a, b and c according to the standard from of Quadratic Equation ax² + bx + c = 0 and you will get roots as output.

13. How to Solve Quadratic Equation Graphically?

While solving Quadratic Equation ax² + bx + c = 0 Graphically we need to find where the curve of the quadratic equation cut the x-axis. The coordinate at which the curve of the quadratic equation cut the x-axis are the roots of the quadratic equations.