Integers

Integers are groups of numbers that are defined as the union of positive numbers, and negative numbers, and zero is called an Integer. ‘Integer’ comes from the Latin word ‘whole’ or ‘intact’. Integers do not include fractions or decimals.

Integers are denoted by the symbol “Z“. You will see all the arithmetic operations, like addition, subtraction, multiplication, and division, on integers. The examples of integers are 0, 1, -4, -12, 100, etc.

In this article, we have covered everything about what are integers in maths, integers definition, types of integers, etc. with respect to Integers classes 6 and 7.

What is an Integers in Maths?

So, What is an Integer? If a set is constructed using all-natural numbers, whole numbers, and negative numbers, then that set is referred to as an Integer set. Integers range from negative infinity to positive infinity.

Integers include positive numbers, negative numbers, and zero. Integers are represented by the symbol Z such that,

Z = {… -7, -6, -5, -4, -3, -2, -1, 0, 1, 2, 3, …}

• Positive Numbers: Numbers greater than zero are called positive numbers. Example: 1, 2, 3 4 . . .
• Negative Numbers: Numbers less than zero are called negative numbers. Example: -1, -2, -3 -4..
• Zero (0) is neither positive nor negative.

Integers Definition

Integers are a set of whole numbers that include both positive and negative numbers, as well as zero. In mathematical terms, integers are numbers without any fractional or decimal parts.

Types of Integers

Integers are classified into three categories:

• Zero (0)
• Positive Integers (i.e. Natural numbers)
• Negative Integers (i.e. Additive inverses of Natural Numbers)

1. Zero

Zero is a unique number that does not belong to the category of positive or negative integers. It is considered a neutral number and is represented as “0” without any plus or minus sign.

2. Positive Integers

Positive integers, also known as natural numbers or counting numbers, are often represented as Z+. Positioned to the right of zero on the number line, these integers encompass the realm of numbers greater than zero.

Z+ → 1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12, 13, 14, 15, 16, 17, 18, 19, 20, 21, 22, 23, 24, 25, 26, 27, 28, 29, 30,….

3. Negative Integers

Negative integers mirror the values of natural numbers but with opposing signs. They are symbolized as Z–. Positioned to the left of zero on the number line, these integers form a collection of numbers less than zero.

Z– → -1, -2, -3, -4, -5, -6, -7, -8, -9, -10, -11, -12, -13, -14, -15, -16, -17, -18, -19, -20, -21, -22, -23, -24, -25, -26, -27, -28, -29, -30,…..

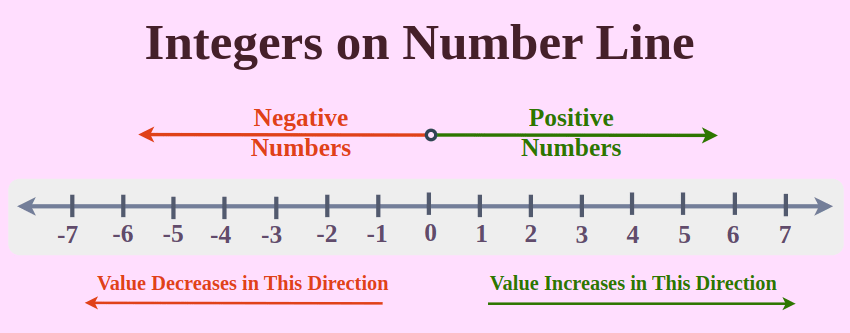
How to Represent Integers on Number Line?

As we have discussed previously, it is possible to visually represent the three categories of integers – positive, negative, and zero – on a number line.

Zero serves as the midpoint for integers on the number line. Positive integers occupy the right side of zero, while negative integers populate the left side. Refer to the diagram below for a visual representation.

Rules of Integers

1. Addition of Positive Integers: When two positive integers are added together, the result is always an integer. This principle underscores the fundamental nature of integers as encompassing positive values beyond the realm of natural numbers.
2. Addition of Negative Integers: Similarly, the sum of two negative integers results in an integer. This mirrors the concept of integers extending into the realm of negative values, broadening the spectrum of numerical representation.
3. Multiplication of Positive Integers: The product of two positive integers yields an integer. This rule reaffirms the idea that the multiplication of positive values remains rooted within the integer domain, offering a bridge between arithmetic operations and this distinct number set.
4. Multiplication of Negative Integers: Similarly, when two negative integers are multiplied, the outcome is an integer. This rule showcases the symmetrical nature of integers, where even multiplicative interactions among negative values lead back to the realm of integers.
5. Sum of an Integer and Its Inverse: The intriguing aspect of integers reveals itself when considering the sum of an integer and its inverse. Remarkably, this sum always equals zero, illuminating the unique property that integers inherently possess. The interplay between positive and negative values results in a harmonious equilibrium at the integer zero.
6. Product of an Integer and Its Reciprocal: Delving deeper into the properties of integers, the product of any integer and its reciprocal, which is defined as one divided by the integer, is consistently equal to 1. This principle showcases the unity that exists within the intricate realm of integers.

Arithmetic Operations on Integers

The four basic Maths operations performed on integers are:

• Addition of Integers
• Subtraction of Integers
• Multiplication of Integers
• Division of Integers

1. Addition of Integers

Addition of integers is similar to finding the sum of two integers. Read the rules discussed below to find the sum of integers.

Example: Add the given integers: 

1. 3 + (-9)
2. (-5) + (-11)

Solution:

1. 3 + (-9) = -6
2. (-5) + (-11) = -16

2. Subtraction of Integers

Subtraction of integers is similar to finding the difference between two integers. Read the rules discussed below to find the difference between integers.

Example: Add the given integers: 

1. 3 – (-9)
2. (-5) – (-11)

Solution:

1. 3 – (-9) = 3 + 9 = 12
2. (-5) – (-11) = -5 + 11 = 6

3. Multiplication of Integers

Multiplication of integers is achieved by following the rule:

• When both integers have same sign, the product is positive.
• When both integers have different signs, the product is negative.

Product of Sign	Resultant Sign	Example
(+) × (+)	+	9 × 3 = 27
(+) × (–)	–	9 × (-3) = -27
(–) × (+)	–	(-9) × 3 = -27
(–) × (–)	+	(-9) × (-3) = 27

4. Division of Integers

Division of integers is achieved by following the rule:

• When both integers have the same sign, the division is positive.
• When both integers have different signs, the division is negative.

Division of Sign	Resultant Sign	Example
(+) ÷ (+)	+	9 ÷ 3 = 3
(+) ÷ (–)	–	9 ÷ (-3) = -3
(–) ÷ (+)	–	(-9) ÷ 3 = -3
(–) ÷ (–)	+	(-9) ÷ (-3) = 3

Properties of Integers

Integers have various properties, the major properties of integers are:

• Closure Property
• Associative Property
• Commutative Property
• Distributive Property
• Identity Property
• Additive Inverse
• Multiplicative Inverse

Closure Property

Closure property of integers states that if two integers are added or multiplied together their result is always an integer. For integers p and q

• p + q = integer
• p x q = integer

Example:

(-8) + 11 = 3 (An integer)
(-8) × 11 = -88 (An integer)

Commutative Property

Commutative property of integers states that for two integers p and q

• p + q = q + p
• p x q = q x p

Example:

(-8) + 11 = 11 + (-8) = 3
(-8) × 11 = 11 × (-8) = -88

But the commutative property is not applicable to the subtraction and division of integers.

Associative Property

Associative  property of integers states that for integers p, q, and r

• p + (q + r) = (p + q) + r
• p × (q × r) = (p × q) × r

Example:

5 + (4 + 3) = (5 + 4) + 3 = 12
5 × (4 × 3) = (5 × 4) × 3 = 60

Distributive Property

Distributive  property of integers states that for integers p, q, and r

p × (q + r) = p × q + p × r

For Example, Prove: 5 × (9 + 6) = 5 × 9 + 5 × 6

LHS = 5 × (9 + 6) 
        = 5 × 15
        = 75

RHS = 5 × 9 + 5 × 6 
        = 45 + 30
        = 75

Thus, LHS = RHS Proved.

Identity Property

Integers hold Identity elements both for addition and multiplication. Operation with the Identity element yields the same integers, such that

• p + 0 = p
• p × 1 = p

Here, 0 is Additive Identity, and 1 is Multiplicative Identity.

Additive Inverse

Every integer has its additive inverse. An additive inverse is a number that in addition to the integer gives the additive identity. For integers, Additive Identity is 0. For example, take an integer p then its additive inverse is (-p) such that

• p + (-p) = 0.

Multiplicative Inverse

Every integer has its multiplicative inverse. A multiplicative inverse is a number that when multiplied to the integer gives the multiplicative identity. For integers, Multiplicative Identity is 1. For example, take an integer p then its multiplicative inverse is (1/p) such that

p × (1/p) = 1.

Applications of Integers

Integers extend beyond numbers, finding applications in real life. Positive and negative values represent opposing situations. For instance, they indicate temperatures above and below zero. They facilitate comparisons, measurements, and quantification. Integers feature prominently in sports scores, ratings for movies and songs, and financial transactions like bank credits and debits.

Resources related to Integers:

• Rational Number
• Irrational Number
• Real Numbers
• Properties of Real Numbers

Solved Examples on Integers

Example 1: Can we say that 7 is both a whole number and a natural number?

Solution:

Yes, 7 is both whole number and natural number.

Example 2: Is 5 a whole number and a natural number?

Solution:  

Yes, 5 is both a natural number and whole number.

Example 3: Is 0.7 a whole number?

Solution: 

No, it is a decimal.

Example 4: Is -17 a whole number or a natural number?

Solution: 

No, -17 is neither natural number nor whole number.

Example 5: Categorize the given numbers among Integers, whole numbers, and natural numbers,

• -3, 77, 34.99, 1, 100

Solution:

Numbers	Integers	Whole Numbers	Natural Numbers
-3	Yes	No	No
77	Yes	Yes	Yes
34.99	No	No	No
1	Yes	Yes	Yes
100	Yes	Yes	Yes

Practice Questions on Integers

1. Write three consecutive integers.
2. Which of the following numbers is the largest: -6, 2, -3, or 0?
3. Calculate the product of -7 and 9.
4. Find the sum of -15, 20, and -8.
5. And some word problems.
6. If the temperature drops by 10 degrees Celsius and then rises by 7℃, what is the net change in temperature?
7. A submarine is at a depth of 120 meters below sea level. If it rises 80 meters, what will its new depth be?

FAQs on Integers

1. What are Integers in Math ?

The union of zero, natural numbers, and their additive inverse is called Integers. It is mathematically denoted by the symbol Z.

2. What are Consecutive Integers?

Consecutive Integers are integers that are adjacent to each other on a number line. The difference between the two consecutive integers is “1”.

3. Write the Examples of Integers.

Examples of integers are -1, -9, 0, 1, 87, etc.

4. What are the different types of Integers?

There are three different types of integers:

• Zero
• Positive Integers
• Negative integers

5. Can Integers be Negative?

Yes, integers can be negative. Negative Integers are -1, -4, and -55, etc.

6. State whether the given statement is True or False: “All Natural Numbers are Whole Numbers”?

True, all natural numbers are whole numbers but not vice versa.

7. What are the applications of Integers?

The Applications of Integers are mentioned above in this article.

8. What is a Positive Integer?

An integer is said to be positive if it is greater than zero. For example: 2, 50, 28 etc.

9. What are the Properties of Integers ?

These are the properties of Integers:

• Closure Property
• Associative Property
• Commutative Property
• Distributive Property
• Additive Inverse Property
• Multiplicative Inverse Property
• Identity Property

All the properties of Integers are explained in detail in the above article.

10. What is the definition of Integers?

The set of integers is the collection of all whole numbers, including both positive whole numbers (natural numbers), their additive inverses (negative whole numbers), and zero. Mathematically, the set of integers can be expressed as {…, -3, -2, -1, 0, 1, 2, 3, …}.

11. Is zero an integer?

Yes, zero is considered an integer. In the set of integers, zero is the integer that separates the positive integers (natural numbers) from the negative integers. Therefore, the set of integers includes all positive whole numbers (1, 2, 3, …), zero (0), and all negative whole numbers (… -3, -2, -1).