Rational Numbers are numbers written in terms of the ratio of two integers, where the denominator is not zero. Rational numbers are real numbers that can be written in the form of p/q, where q ≠ 0. Rational Numbers are the most fundamental set of the collection of all numbers. A rational number represents the fraction of two integers where the denominator can’t be 0. In this article, we have explained the Rational and Irrational Numbers, their Definitions, Types, Examples, and more.
Examples of Rational Numbers include 12/21, 34/2, -22 etc. In other words, a rational number is any number that can be written in the form of a/b, where a and b are integers and b is not equal to zero.
In this article, we have provided everything related to Rational numbers including, definitions, examples, types, list of rational numbers, and how to identify rational numbers.
.png)
What are Rational Numbers?
Rational numbers can be defined as numbers represented in the form of p/q where p and q are both integers and q ≠ 0. Also, any fraction where the denominator is not equal to zero is considered to be a rational number. Thus, rational numbers are a group of numbers that includes fractions, decimals, whole numbers, and natural numbers, also the collection of all rational numbers is denoted by Q.
- All natural numbers, whole numbers, integers, and fractions are rational numbers.
- Every rational number can be represented on a number line.
- 0 is neither a positive nor a negative rational number.

Rational Number Definition
A number in the form of p/q where p and q are integers and q ≠ 0 is called a Rational Number. For Example, 2/3, -3/4, 22/7 etc.
Rational Numbers Examples
Examples of Rationl Numbers includes the following:
- 0 (which can be written as 0/1)
- 19 (which can be written as 19/1)
- 2/9
- √(64) which gives 4 or 4/1
- -6/7
- 0.333333 = 1/3
- -0.9 = -9/10
Curious about the nature of π? Read – Is π a rational or irrational number?
Note: In Set Theory, Rational Number is represented as Q. If Q is a set of Rational Numbers then set of Natural Numbers (N), Whole Numbers (W) and Integers (Z) are all subsets of Rational Number Set.
Representation of Rational Numbers
Rational Numbers can be represented in following two ways:
- In Fraction Form
- In Fraction form we represents in terms of Numerator and Denominator
- In Decimal Form
- In Decimals form we represents in terms of Terminating and Repeating Patterns
Rational Numbers Types
Rational Numbers can be classified into following Types
- Standard Form
- Positive Rational Number
- Negative Rational Number
- Terminating Rational Number
- Non Terminating and Repeating Rational Number
Standard Form of Rational Number
The standard form of a rational number is defined as having no common factors other than one between the dividend and divisor, and hence the divisor is positive.
For instance, 12/36 is a rational number. However, it can be simplified to 1/3; the divisor and dividend only share one common element. We could say that rational number ⅓ is in a standard form.
Positive Rational Number
Positive Rational Numbers are those in which both numerators and denominators are either positive or negative. In case both numerators and denominators are negative, -1 can be eliminated as common factor which gives us ultimately Positive Rational Number
Example of Positive Rational Numbers are 2/5, -3/-5 etc.
Negative Rational Number
Negative Rational Numbers are those in which either of Numerator or denominator is negative integer.
Example of Negative Rational Number includes -1/2, 3/-4
Terminating Rational Number
Terminating Decimals are the Rational numbers whose decimal representations end or terminate after a certain number of digits.
Rational Number has terminating expansion if the denominator is in the form of 2m × 5n where either of m and n can be zero
Example of Terminating Decimal
Non Terminating and Repeating Rational Number
Repeating Decimals are the Rational numbers whose decimal representations have a repeating pattern.
The decimal expansion of non terminating rational number doesn’t end. Same digit or group of digits is repeated after fixed interval
Example of Non Terminating and Repeating Rational Number
How to Identify Rational Numbers?
All the rational numbers follow the following rules, thus using the help of these rules we can identify the rational numbers
- Rational numbers are represented in the form of p/q, where q≠0.
- Ratio p/q can be further simplified in simple form or decimal expansion.
- Non-terminating decimals with repeating decimal values are also considered rational numbers as they can be represented in the form of p/q.

Example: Which of the following numbers are rational numbers?
a) -1.75
b) 2/3
c) √5
d) π
Solution:
a) -1.75 is a rational number as it it has a terminating decimal expansion.
b) 2/3 is also a rational number as it can be expressed in the form of a ratio of two integers.
c) √5 is an irrational number because it has a decimal expansion with infinitely many digits without any repeatation.
d) π is also an irrational number as it has a decimal expansion with infinitely many digits without any repeaatation.
Thus, only (a) and (b) are the rational numbers out of all the given numbers.
List of Rational Numbers in Number system
The following are the classification of rational numbers in Number system .
- All Natural Numbers are Rational Numbers
- All Whole Numbers are Rational Numbers
- All Integers are Rational Numbers
- All Fractions are Rational Numbers
- All Terminating Decimals are Rational Numbers
- All Non-Terminating and Repeating Decimals are Rational Numbers.
- Square roots of all perfect squares are Rational Numbers
- Cube Roots of all perfect cubes are Rational Numbers.
Note: All real numbers are not rational numbers but all the rational numbers are real numbers
Arithmetic Operations on Rational Numbers
There are four most common operations for Rational Numbers, which includes the following
- Addition
- Subtraction
- Multiplication
- Division

Addition of Rational Numbers
Addition of two rational numbers can be done using the following step-by-step method where the addition of 3/4 and 1/6 is explained as an example.
Step 1: Find the common denominator (LCD) for both the rational number. i.e.,
Common denominator for 4 and 6 is 12.
Step 2: Convert both the rational number to equivalent fractions using the common denominator. i.e.,
3/4 = (3 × 3)/(4 × 3) = 9/12
1/6 = (1 × 2)/(6 × 2) = 2/12
Step 3: Add numerators of the equivalent fractions obtained in step 2. i.e.,
9/12 + 2/12 = (9 + 2)/12 = 11/12
Step 4: Simplify the resulting fraction if possible. i.e.,
11/12 is already in its simplest form.
Thus, Addition of 3/4 and 1/6 is 11/12 .
Subtraction of Rational Numbers
Subtraction of two Rational Numbers can be done using the following step-by-step method where subtraction of 1/3 and 2/5 is explained.
Step 1: Find the common denominator (LCD) for both the rational number. i.e.,
Common denominator for 3 and 5 is 15.
Step 2: Convert both the rational numbers to equivalent fractions with the common denominator. i.e.,
1/3 = (1 × 5)/(3 × 5) = 5/15
2/5 = (2 × 3)/(5 × 3) = 6/15
Step 3: Subtract numerators of equivalent fractions obtained in step 2. i.e.,
5/15 – 6/15 = (5 – 6)/15 = -1/15
Step 4: Simplify the resulting fraction if possible. i.e.,
-1/15 is already in its simplest form.
Therefore, 1/3 – 2/5 = -1/15.
Multiplication of Rational Numbers
Multiplication of two rational numbers can be achieved by simply multiplying the numerator and denominator of the given Rational Numbers. Step by step method with an example of multiplication of -11/3 and 4/5 is as follows:
Step 1: Write both rational number in with multiplication sign(×) in between. i.e. -11/3 × 4/5
Step 2: Multiply the numerator and denominator individually. i.e.,(-11 × 4)/(3 × 5)
Step 3: We get the result of the multiplication. i.e., -44/15
Division of Rational Numbers
Division of two Rational numbers can be achieved in the following steps(where the division of 3/5 and 4/7 is explained):
Step 1: Write both rational number in with division sign in between. i.e., 3/5 ÷ 4/7
Step 2: Change “÷” with “×” and take reciprocal of the second rational number. i.e., 3/5 × 7/4
Step 3: Multiply the numerator and denominator of the resulting fractions. i.e., (3 × 7)/(5 × 4)
Step 4: We get the result of the division. i.e., 21/20
Equivalent Rational Numbers
Equivalent Rational Numbers are those which when reduced to the simplest form the their values are equal. They can be obtained by multiplying or dividing a the numerator and denominator by a fixed number. We can learn it through the following example.
Example: Find the first three equivalent rational numbers of 5/6
Solution:
First three equivalent rational numbers of 5/6 are
(5×2)/(6×2) = 10/12
(5×3)/(6×3) = 15/18
(5×4)/(6×4) = 20/24
Example: Check if 20/16 is equivalent to 10/8
Solution:
Simplest form of 20/16 is 5/4
Simplest form of 10/6 is 5/4
Hence, 20/16 is equivalent to 10/8
Decimal Expansion of Rational Numbers
There are two decimal forms of Rational Numbers, terminating and recurring.
Terminating Decimal
Rational number whose decimal expansion has finite terms after decimal is called a terminating decimal. For Example, 1/4 = 0.25, 8/5 = 1.6 etc. A rational number has terminating decimal expansion if the denominator is in the form of 2m ⨯ 5n where m and n can be zero. Terminating Decimal Examples include 1/4 = 0.25, 25/4 = 6.25, 1/2 = 0.5 etc.
Non-Terminating and Repeating(Recurring) Decimal
Rational number whose decimal expansion is non-terminating decimals i.e. which does not have finite terms after decimals and the repeating of digits is periodic i.e. repeat after the fixed interval is called Non-Terminating and Repeating Decimals. They are also called Recurring Decimals. A rational number which is not in the form of 2m ⨯ 5n where m and n can be zero i.e. it may be some other prime factors or maybe some extra in addition to 2 and 5 then the expansion is non-terminating and repeating. Examples of Recurring Decimals are 1/3 = 0.333…, 1.232323…
Multiplicative Inverse of a Rational Number
The multiplicative inverse of a rational number is a fundamental concept in mathematics, particularly in the realm of rational numbers. It is also known as the reciprocal of a rational number.
In essence, for any non-zero rational number a/b, its multiplicative inverse is b/a. The product of a rational number and its multiplicative inverse is always 1.
This concept plays a pivotal role in various mathematical operations involving rational numbers, including division, simplification, and solving equations. For instance, when dividing one rational number by another, we often multiply the dividend by the reciprocal of the divisor to simplify the operation.
The multiplicative inverse of a rational number a/b exists if a is not equal to zero. If a were equal to zero, the rational number would be undefined.
Rational Numbers Properties
Rational Numbers show several properties under the different operations (two of such common operations are addition and multiplication), which are as follows:
- Closure Property
- Commutative Property
- Associative Property
- Identity Property
- Inverse Property
- Distributive Property
Closure Property
- Closure Property for Addition: Rational numbers are closed under addition, i.e., for any two rational numbers a and b, the sum a + b is also a rational number.
- Closure Property for Multiplication: Rational numbers are closed under multiplication, i.e., for any two rational numbers a and b, their product ab is also a rational number
Example: Verify Closure Property for the sum and product of 3/4 and -1/2
Solution:
For a = 3 / 4 and b = (-1) / 2
Now, a + b = 3 / 4 + (-1) / 2
⇒ a + b = (3- 2)/ 4
⇒ a + b = 1/4, is Rational Number.
Also, a × b = 3/4 × (-1)/2 = -3/8, which is also Rational number.
Commutative Property
- Commutative Property for Addition: Rational numbers hold commutative property under addition operation, i.e., for any two rational numbers a and b, a + b = b + a.
- Commutative Property for Multiplication: Rational numbers hold commutative property under multiplication operation as well, i.e., for any two rational numbers a and b, ab = ba.
Example: For a = (-7) / 8 and b = 3 / 5, verify commutative property.
Solution:
Now, a + b = -7/8 + 3/5
⇒ a + b = (-7 x 5 + 3 x 8)/40 = (-35 + 24) / 40
⇒ a + b = (-11) / 40
And, b + a = 3/5 + (-7)/8
⇒ b + a = (3 x 8 + (-7) x 5)/ 40 = (24 – 35)/40
⇒ b + a = -11/40 = a + b
Now, ab = (-7)/8 x 3/5 = (-7 x 3)/(8 x 5)
⇒ ab = -21/40
And, ba = 3/5 x (-7)/8 = (3 x 7 )/(5 x 8)
⇒ ba =(-21)/40 = ab
Associative Property
- Associative Property for Addition: Rational Numbers are associative under addition operation, i.e., for any three Rational Numbers a, b, and c, a + (b + c) = (a + b) + c
- Associative Property for Multiplication: Rational Numbers are associative under multiplication operation as well, i.e., for any three Rational numbers a, b, and c, a(bc) = (ab)c
Example: For three Rational numbers a, b, c where a = -1/2, b = 3/5, c = -7/10, verify associative property.
Solution:
Now, a + b = -1/2 + 3/5 = (-5 + 6)/10 = 1/10
and (a + b) + c = 1/10 + (-7)/10
⇒ (a + b) + c = (1 – 7)/10 = -6/10 = -3/5
Also, b + c = 3/5 + (-7)/10
⇒ b + c = (6 – 7)/10 = -1/10
and, a + (b + c) = -1/2 + (-1)/10
⇒ a + (b + c) = (-5 – 1)/10= -6/10 = -3/5
Thus, (a + b) + c = a + (b + c) is true for Rational Numbers.
Similarly, for multiplication
a × b = -1/2 × 3/5 = -3/10
and, (a × b)× c = -3/10 × -7/10= -3× (-7)/100
⇒ (a × b)× c = 21/100
Also, b× c = 3/5 × (-7)/10 = -21/50
and, a × ( b × c ) = -1/2 × (-21)/50
⇒ a × ( b × c ) = 21/100
Thus, (a× b)× c = a × ( b × c ) is true for Rational Numbers.
Identity Property
- Identity Property for Addition: For any rational number a, there exists a unique rational number 0 such that 0 + a = a = a + 0, where 0 is called the identity of the rational number under the addition operation.
- Identity Property for Multiplication: For any rational number a, there exists a unique rational number 1 such that a × 1 = a = a × 1, where 1 is called the identity of the rational number under the multiplication operation.
Inverse Property
- Additive Inverse property: For any rational number a, there exists a unique rational number -a such that a + (-a) = (-a) + a = 0, and -a is called the inverse of element a under the operation of addition. Also, 0 is the additive identity.
- Multiplicative Inverse property: For any rational number b, there exists a unique rational number 1/b such that b × 1 / b = 1 / b × b = 1, and 1/b is called the inverse of the element b under the multiplication operation. Here, 1 is the multiplicative identity.
Example: Find the Additive and Multiplicative Inverse of -11/23
Solution:
For a = -11/23
a + (-a) = -11/23 – (-11)/23
a + (-a) = -11/23 + 11/23 = (-11 + 11)/23 = 0
Similarly, (-a) + a = 0
Thus, 11/23 is the additive inverse of -11/23.
Now, for b = -17/29
b × 1/b = -17/29 × -29/17 = 1
Similarly, 1 / b × b = 29/17 × -17/29 = 1
Thus, -29 / 17 is the multiplicative inverse of -17/23.
Distributive Property
Distributive property for any two operations holds if one distributes over the other. For example, multiplicative is distributive over addition for the collection of rational numbers, for any three rational numbers a, b, and c the distributive law of multiplication of addition is
a × (b +c) = (a× b) + (a × c), and it is true for all the rational numbers.
Example: For rational number a, b, c i.e., a = -7 / 9, b = 11 / 18 and c = -14 / 27, verify distributive.
Solution:
Now, b + c = 11/18 + (-14)/27
⇒ b + c = 33/54 + (-28)/54 = (33 – 28)/54 = 5/54
and, a × ( b + c ) = -7/9 × 5/54
⇒ a × ( b + c ) = (-7 × 5)/(9 × 54) = -35/486 . . .(1)
Also, a × b = -7/9 × 11/18
⇒ a × b = (-7 × 11)/9 × 18 = -77/9 × 9 × 2
and a × c = (-7)/ 9 ×(-14)/27
⇒ a × c = (7 × 14)/9 × 9 × 3 = 98/9 × 9 × 3
Now, (a × b) + (a × c) = (-77/9 × 9 × 2 ) + ( 98/9 × 9 × 3)
⇒ (a × b) + (a × c) = (-77 × 3 + 98 × 2)/9 × 9 × 2 × 3
⇒ (a × b) + (a × c) = (-231 + 196)/486 = -35/486 . . .(2)
(1) and(2) shows that a × ( b + c ) = ( a × b ) + ( a × c ).
Hence, multiplication is distributive over addition for the collection Q of rational numbers.
Finding Rational Numbers between Two Rational Numbers
Between two rational numbers there exists infinite rational numbers. However, we can find a rational number between two rational numbers using the formula 1/2(a + b) where a and b are rational numbers. Let’s say we have to find rational numbers between 2 and 3 then a rational number between 2 and 3 is given as 1/2(2 + 3) = 5⨯1/2 = 5/2
However, other methods also exist to find rational numbers between two rational numbers.
Method 1: To find rational numbers between two rational numbers with like denominators.
In this, we need to multiply the numerator and denominator of rational numbers with a larger number to create a gap between numerators.
Once the gap is created write the in-between rational numbers just increasing the numerator by 1 and keeping the denominators same.
Example: Find 10 rational numbers between 4/5 and 6/5.
Solution:
In this case, we see that we can only find only one rational number between 4/5 and 6/5 which is 5/5. But here we need to find 10 rational numbers.
Hence, we would multiply the numerator and denominator in both the rational number by 10. Hence we have to find 10 rational numbers between (4⨯10)/(5⨯10) and (6⨯10)/(5⨯10) i.e. 40/50 and 60/50.
Hence, ten rational numbers between 40/60 and 50/60 are 41/50, 42/50, 43/50, 44/50, 45/50, 46/50, 47/50, 48/50, 49/50, 50/50.
If we need more we would multiply by a larger number. For simplicity, you can multiply by 10, 100, etc.
Method 2: To find a rational number between two rational numbers with unlike denominators
In this, we first convert the unlike denominators to like decimals then follow the same method as followed in the case of like denominators
Example: Find 5 rational numbers between 4/3 and 6/5
Solution:
Here we will first make the denominators like, by taking the LCM of denominators 3 and 5. Hence, the LCM of 3 and 5 is 15. Therefore our new equivalent rational numbers will be (4⨯5)/(3⨯5) and (6⨯3)/(5⨯3) i.e. 20/15 and 18/15.
Still, we see that gap is of two only between 18 and 20. Hence, we will multiply with a larger number say 5.
Hence, we have to find a rational number between 20⨯5/15/⨯5 and 18⨯5/15⨯5 i.e. 100/75 and 90/75. Hence, 5 rational numbers between 90/75 and 100/75 are 91/75, 92/75, 93/75, 94/75 and 95/75.
Method 3: To find ‘n’ rational numbers between two rational numbers x and y with unlike denominators such that x < y
In this case, first calculate, d = (y – x)/(n + 1) then find the rational numbers between two rational numbers as (x + d), (x + 2d), (x + 3d),…..,(x + nd)
Example: Find five rational numbers between 1/3 and 2/5.
Solution:
x = 1/3, y = 2/5, n = 5
d = (y – x)/(n + 1) = (2/5 – 1/3)/(5 + 1) = 1/15/6 = 1/90
Five rational numbers between 1/3 and 2/5 are given as
(x + d), (x + 2d), (x + 3d), (x + 4d) and (x + 5d)
(1/3 + 1/90), (1/3 + 2/90), (1/3 + 3/90), (1/3 + 4/90) and (1/3 + 5/90)
(31/90), (32/90), (33/90), (34/90) and (35/90)
Representing Rational Numbers on Real Line
Rational Numbers are Real Numbers. Hence they can be represented on real line. There are following steps that need to be followed to represent rational numbers on real line.
Step 1: First find if the number is positive or negative if positive then it will be plotted on the RHS of zero and if positive it will be on the LHS of zero.
Step 2: Identify if the given rational number is proper or improper. If proper then it will lie between 0 and 1 in case of positive and 0 and -1 in case of negative rational number.
Step 3: If improper then convert it into a mixed fraction. In this case, the rational number will lie just beyond the whole number part.
Step 4: Now after steps 1, 2, and 3 we have to plot only the proper fraction part. To plot this cut the area between the two successive desired numbers by drawing lines n-1 times where n is the denominator of the proper fraction part.
Step 5: Now count the lines equal to the value of the numerator. This will represent the desired rational number on a real line.
Let’s see some examples:
Example 1: Represent 2/5 on Real Line
Solution:

Example 2: Represent -2/5 on Real Line
Solution:
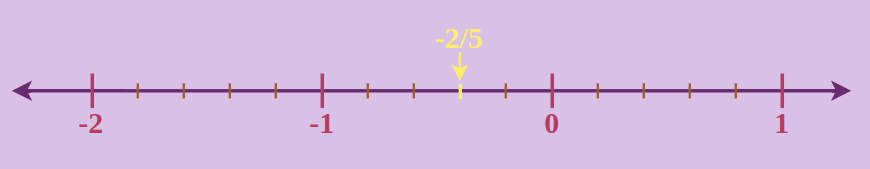
Example 3: Represent 4/3 on Real Line
Solution:
4/3 = 1(1/3)
.png)
Example 4: Represent -4/3 on Real Line
Solution:
-4/3 = -{1(1/3)}

Rational and Irrational Numbers
Irrational Numbers are those which can’t be represented in the form of p/q where q ≠ 0. The decimal expansion of irrational numbers is non-terminating and non-repeating. Let’s learn a comparison between the rational and irrational numbers in the table given below:
|
Rational Numbers
|
Irrational Numbers
|
|
It can be represented in the form of p/q where q ≠ 0
|
It can’t be represented in the form of p/q where q ≠ 0
|
|
Its Decimal Expansion is either terminating or non-terminating and repeating
|
Its Decimal Expansion is non-terminating and non-repeating
|
|
A set of rational numbers contains all types of numbers such as natural numbers, whole numbers, and integers.
|
Irrational Numbers doesn’t contain all types of numbers in itself
|
|
Examples include 2/3, -5/6, 0.25, 0.333, 22/7, etc.
|
Examples include √2,√3, 1.010010001, π, etc.
|
Rational Numbers Class 8 NCERT PDF
The Class 8 NCERT Mathematics textbook offers comprehensive coverage of rational numbers, providing clear explanations and numerous examples to illustrate what are rational numbers. Mastery of rational numbers is crucial for understanding the distinction between rational and irrational numbers and grasping the properties of rational numbers. In addition to rational numbers examples, the textbook may include worksheets and exercises to reinforce learning. By acquiring the Rational Numbers Class 8 NCERT PDF, students can access valuable resources to enrich their understanding of rational numbers and enhance their mathematical proficiency.
Rational Numbers – Solved Examples
Question 1: Check which of the following is irrational or rational: 1/2, 13, -4, √3, and π.
Solution:
Rational numbers are numbers that can be expressed in the form of p/q, where q is not equal to 0.
1/2, 13, and -4 are rational numbers as they can be expressed as p/q.
√3, and π are irrational numbers as they can not be expressed as p/q.
Question 2: Check if a mixed fraction, 3(5/6) is a rational number or an irrational number.
Solution:
Simplest form of 3(5/6) is 23/6
Numerator = 23, which is an integer
Denominator = 6, is an integer and not equal to zero.
So, 23/6 is a rational number.
Question 3: Determine whether the given numbers are rational or irrational.
(a) 1.33 (b) 0.1 (c) 0 (d) √5
Solution:
a) 1.33 is a rational number as it can be represented as 133/100.
b) 0.1 is a rational number as it can be represented as 1/10.
c) 0 is a rational number as it can be represented as 0/1.
d) √5 is an irrational number as it can not be represented as p/q.
Question 4: Simplify (2/3) × (6/8) ÷ (5/3).
Solution:
(2/3) × (6/8) ÷ (5/3) = (2/3) x (6/8) × (3/5)
= (2 × 6 × 3)/(3 × 8 × 5)
= 36/120 = 3/10
Question 5: Arrange following rational numbers in ascending order: 1/3, -1/2, 2/5, and -3/4.
Solution:
Common denominator for 3, 2, 5, and 4 is 60. Thus
1/3 = 20/60
-1/2 = -30/60
2/5 = 24/60
-3/4 = -45/60
With common denominator, rational number with greatest numerator is greatest.
⇒ -30/60 < -45/60 < 20/60 < 24/60
Thus, ascending order of given rational numbers is: -1/2 < -3/4 < 1/3 < 2/5
Rational Numbers Worksheet
Try out the following questions on rational numbers
Q1. Find two rational number between 2/3 and 3/4
Q2. Find the sum of -3/5 and 6/7
Q3. Find the first five equivalent rational numbers of -7/8
Q4. Represent 4/3 on Real Line
Q5. Find the Product of -19/3 and 2/57
Summary – Rational Numbers
Rational numbers are essentially any numbers that can be expressed as a fraction or ratio where both the numerator (the top number) and the denominator (the bottom number) are integers, and the denominator is not zero. This category includes a wide array of numbers such as natural numbers, whole numbers, integers, fractions, and even some decimal numbers if they terminate (come to an end) or repeat a pattern. Rational numbers are denoted by the letter Q and are part of the larger real number system, which also includes irrational numbers. Examples of rational numbers are straightforward fractions like 2/3, decimal numbers that end like 0.75, or even negative numbers like −1.75. They can also be non-terminating decimals as long as there is a repeating pattern, such as 0.333…. Identifying rational numbers is quite simple if you remember that they can always be written as a fraction p/q, where q is not zero. Rational numbers are fundamental in mathematics because they allow us to perform arithmetic operations like addition, subtraction, multiplication, and division, and they can represent a wide range of quantities in real life.
FAQs on Rational Numbers
What are rational numbers?
A rational number is a number that is in the form of p/q, where p and q are integers, and q is not equal to 0. Some examples of rational numbers include 1/3, 2/4, 1/5, 9/3, and so on.
What is difference between rational and irrational numbers?
A rational number is a number that is expressed as the ratio of two integers, where the denominator should not be equal to zero, whereas an irrational number cannot be expressed in the form of fractions. Rational numbers are terminating decimals but irrational numbers are non-terminating and non-recurring. An example of a rational number is 10/2, and an irrational number is a famous mathematical value Pi(π) which is equal to 3.141592653589…….
Is 0 a rational number?
Yes, 0 is a rational number because it is an integer that can be written in any form such as 0/1, 0/2, where b is a non-zero integer. It can be written in the form: p/q = 0/1. Hence, we conclude that 0 is a rational number.
Is Pi(π) a rational number?
No, Pi (π) is not a rational number. It is an irrational number and its value equals 3.142857…
Are fractions rational numbers?
Fractions are numbers that are represented in the form of (numerator/denominator) which is equivalent to p/q form so fractions are considered rational numbers. Example 3/4 is a fraction but is also a rational number.
Are all rational numbers integers?
No, all rational numbers are not integers but the opposite is true. i.e. “all integers are rational numbers.” For example, 1/2 is a rational number but not an integer whereas -7 is an integer and is also a rational number.
Can rational numbers be negative?
Yes, a rational number can be negative i.e. all negative number comes under rational numbers. Example -1.25 is a rational number.
Are all whole numbers rational numbers?
Yes, all whole number are considered as rational numbers. For example 1 is a whole number and is also a rational number.
How many rational numbers are between 1/2 and 1/3?
There are infinite rational numbers between any two rational number, thus there are infinite rational numbers between 1/2 and 1/3, some of those numbers are 11/24, 7/24, 19/48, 13/72, 3/8 etc.
By which Symbol rational number denoted?
Rational Numbers are denoted by “Q” in the mathematics.
How many rational numbers are there?
There are infinitely many rational numbers.
Find five rational numbers between 3/5 and 4/5.
To find five rational numbers between 3/5 and 4/5, we can divide the interval between these two numbers into equal parts and find the rational numbers within those intervals. One way to do this is to add or subtract a common fraction, like 1/25, from the given fractions. Thus, five rational numbers between 3/5 and 4/5 could be: 11/25, 12/25, 13/25, 14/25, and 16/25.
What is the meaning of rational numbers?
Rational numbers are numbers that can be expressed as the quotient or fraction of two integers, where the denominator is not equal to zero. In other words, any number that can be written in the form a/b, where a and b are integers and b ≠ 0, is a rational number. Rational numbers include integers, fractions, and terminating or repeating decimals.
How to classify rational and irrational numbers?
Rational numbers are those that can be expressed as fractions of integers, while irrational numbers cannot be expressed as fractions and have non-repeating, non-terminating decimal representations. To classify a number, one can determine if it can be written as a fraction. If it can, it’s rational; if not, it’s irrational.
How to identify rational numbers?
To identify rational numbers, you simply look for numbers that can be written as fractions of integers. For example, whole numbers like 5, -3, and 0 are rational because they can be expressed as simple fractions like 5/1, -3/1, and 0/1. Also, any fraction such as 3/4 or -2/7 is rational. In decimal form, rational numbers either end after a certain number of digits or repeat the same sequence endlessly. For instance, 0.25 (equal to 1/4) and 0.333… (equal to 1/3) are rational because their decimals stop or repeat.
Like Article
Suggest improvement
Share your thoughts in the comments
Please Login to comment...