Class 9 RD Sharma Solutions – Chapter 8 Introduction to Lines and Angles- Exercise 8.4 | Set 2
Last Updated :
06 Mar, 2023
Question 11. In the given figure, lines AB and CD are parallel and P is any point as shown in the figure. Show that ∠ABP+ ∠CDP= ∠DPB.

Solution:
Here in the given figure:

Given:
AB || CD
Now draw a line XY passing through point P and parallel to AB and CD.
Here,XY || CD, thus, ∠CDP and ∠1are alternate interior opposite angles. Therefore,
∠1 = ∠CDP ……(i)
Similarly, we have XY || AB, thus, ∠ABP and∠2are alternate interior opposite angles. Therefore,
∠2 = ∠ABP ….(ii)
On adding (i) and (ii)
∠1 + ∠2 = ∠CDP + ∠ABP
∠DPB = ∠CDP + ∠ABP
Thus proved.
Question 12. In the given figure, AB||CD and P is any point shown in the figure. Prove that:
∠ABP+ ∠BPD+ ∠CDP= 360°

Solution:
The given figure is as follows:

It is given that AB || CD
Let us draw a line XY passing through point P and parallel to AB and CD.
We have XY || CD, thus, ∠CDP and∠2are consecutive interior angles. Therefore,
∠2 + ∠CDP = 180° ……(i)
Similarly, we have XY || AB, thus,∠CDP and∠2 are consecutive interior angles. Therefore,
∠1 + ∠ABP = 180° ……(ii)
On adding equation (i) and (ii), we get:
∠2 + ∠CDP + ∠1 + ∠ABP = 180° + 180°
(∠2 + ∠1) + ∠CDP + ∠ABP = 360°
∠ABP + ∠BPD + ∠CDP = 360°
Hence proved.
Question 13. Two unequal angles of a parallelogram are in the ratio 2 : 3. Find all its angles in degrees.
Solution:
The parallelogram can be drawn as follows:

It is given that
∠A : ∠C = 2 : 3
Therefore, let:
∠A = 2x and ∠C = 3x
We know that opposite angles of a parallelogram are equal.
Therefore,
∠A = ∠D
∠D = 2x
Similarly
∠B = 3x
Also, if AB || CD, then sum of consecutive interior angles is equal to180°. .
Therefore,
∠A + ∠C = 180°
2x + 3x = 180°
5x = 180°
x =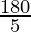
x = 36°
We have
∠A = 2x
∠A = 2(36°)
∠A = 72°
Also,
∠C = 3x
∠C = 3(36°)
∠C = 108°
Similarly,
∠D = 72°
And
∠B = 108°
Hence, the four angles of the parallelogram are as follows:
∠A = 72°, ∠B = 108°, ∠C = 72° and ∠D = 108°
Question 14. In each of the two lines is perpendicular to the same line, what kind of lines are they to each other?
Solution:
The figure can be drawn as follows:

Here, l ⊥ n and m ⊥n.
We need to find the relation between lines l and m
It is given that l ⊥n, therefore,
∠1 = 90° …….(i)
Similarly, we have m ⊥n, therefore,
∠2 = 90° …….(ii)
From (i) and (ii), we get:
∠1 = ∠2
But these are the pair of corresponding angles.
Theorem states: If a transversal intersects two lines in such a way that a pair of corresponding angles is equal, then the two lines are parallel.
Thus, we can say that l || m
Hence, the lines are parallel to each other.
Question 15. In the given figure, ∠1 = 60° and ∠2 =(23)rd23rdof a right angle. Prove that l||m.

Solution:
The figure is given as follows:
It is given that ∠1 = 60°
Also,
∠2 =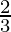 (90°)
(90°)
∠2 = 2(30°)
∠2 = 60°
Thus, we have ∠1 = ∠2
But these are the pair of corresponding angles.
Thus, l || m
Hence, proved.
Question 16. In the given figure, if l||m||n and ∠1 = 60°, find ∠2.

Solution:
The given figure is as follows:
We have l || m || n and ∠60°
Thus, we get ∠1 and ∠3 as corresponding angles.
Therefore,
∠3 = ∠1
∠3 = 60° ……….(i)
We have ∠3 and ∠4 forming a linear pair.
Therefore, they must be supplementary. That is;
∠3 + ∠4 = 180°
From equation (i)
60° + ∠4 = 180°
∠4 = 180° – 60°
∠4 = 120° ……(ii)
We have m || n
Thus, we get ∠2 and ∠4 as alternate interior opposite angles.
Therefore, these must be equal. That is,
∠2 = ∠4
From equation (ii), we get :
∠2 = 120°
Hence, the required value for ∠2 is 120°
Question 17. Prove that the straight lines perpendicular to the same straight line are parallel to one another.
Solution:
The figure can be drawn as follows:

Here, l ⊥ m and m ⊥ n.
We need to prove that l || m
It is given that l ⊥n, therefore,
∠1 = 90° …..(i)
Similarly, we have m ⊥ n, therefore,
∠2 = 90° ……(ii)
From (i) and (ii), we get
∠1 = ∠2
But these are the pair of corresponding angles.
Theorem states: If a transversal intersects two lines in such a way that a pair of corresponding angles is equal, then the two lines are parallel.
Thus, we can say that l || m.
Question 18. The opposite sides of a quadrilateral are parallel. If one angle of the quadrilateral is 60°, find the other angles.
Solution:
The quadrilateral can be drawn as follows:

Here, we have AB || CD and AC || BD.
Also, ∠ACD = 60°.
Thus, AB || CD.
Thus, ∠ACD and ∠BAC are consecutive interior angles.
Therefore, these two must be supplementary. i.e.
∠ACD + ∠BAC = 180°
60° + ∠BAC = 180°
∠BAC = 180° – 60°
∠BAC = 120°
Similarly, AC || BD .
Hence, ∠ACD and ∠CDB are consecutive interior angles.
Therefore, these two must be supplementary. i.e.
∠ACD + ∠CDB = 180°
60° + ∠CDB = 180°
∠CDB = 180° + 60°
∠CDB = 120°
Similarly, AB || CD.Thus, ∠ABD and ∠CDB are consecutive interior angles.
Therefore, these two must be supplementary. i.e.
∠ABD + ∠CDB = 180°
∠ABD + 120° = 180°
∠ABD = 180° – 120°
∠ABD = 60°
Thus, the other angles are as follows:
∠BAC = 120°
∠CDB = 120°
∠ABD = 60°
Question 19. Two lines AB and CD intersect at O. If ∠AOC+ ∠COB+ ∠BOD= 270°, find the measures of ∠AOC, ∠COB, ∠BOD and ∠DOA.
Solution:

Since, lines AB and CD intersect each other at point O.
Thus, ∠AOC and ∠BOD are vertically opposite angles.
Therefore,
∠AOC = ∠BOD …… (i)
Similarly,
∠COB = ∠AOD …… (ii)
Also, we have ∠AOC, ∠BOD, and ∠AOD forming a complete angle.
Therefore, ∠AOC + ∠BOD + ∠COB + ∠AOD = 360°
Given:
∠AOC + ∠COB + ∠BOD = 270°
Hence, we get
(∠AOC + ∠BOD + ∠COB) + ∠AOD = 360°
270° + ∠AOD = 360°
∠AOD = 360° – 270°
∠AOD = 90°
From (ii), we will get;
∠COB = 90°
As we know that ∠AOC and ∠COB form a linear pair. Therefore, these must be supplementary.
∠AOC + ∠COB = 180°
∠AOC + 90° = 180°
∠AOC = 180° – 90°
∠AOC = 90°
From (i), we get;
∠BOD = 90°
Question 20. In the given figure, p is a transversal to lines m and n, ∠2 = 120° and ∠5 = 60°. Prove that m||n.

Solution:
The given figure is;
Here we have that p is a transversal to lines m and n.
Also,
∠2 = 120° and ∠5 = 60°.
To prove: m || n
Here we have ∠2 = 120°.
Also, ∠2 and ∠4 are vertically opposite angles, therefore, these two must be equal. i.e.
∠4 = 120° ……(i)
Also, ∠5 = 60°
Adding this equation to (i), we will get :
∠4 + ∠5 = 120° + 60°
∠4 + ∠5 = 60°
But these are the consecutive interior angles.
Theorem states: If a transversal intersects two lines in such a way that a pair of consecutive interior angles is supplementary, then the two lines are parallel.
Thus, m || n.
Therefore, the lines are parallel to each other.
Share your thoughts in the comments
Please Login to comment...