Area Between Two Curves – Calculus

Area Between Two Curves in Calculus is one of the applications of Integration which helps us calculate the area bounded between two or more curves using the integration. As we know Integration in calculus is defined as the continuous summation of very small units. The topic “Area Between Two Curves” has applications in the various fields of engineering, physics, and economics. 

In this article, we will all the basics of the area between two curves in calculus such as the definition, formula, and method.

Area Between Two Curves Definition

The area between two curves is the area of the region that is bounded by two curves in a plane i.e., both curves are the boundaries of the area required. These two curves can be any function, including polynomials, trigonometric functions, exponential functions, or any other function. 

For example, let’s consider two functions f(x) = sin x and g(x) = x+1. The area bounded between f(x) and g(x) is illustrated in the following diagram:

How to Find the Area Between Two Curves?

To calculate the area between two curves, use the following steps:

Step 1: Determine the points of intersection between the two curves by substituting value of one variable from one curve to another curve.

Step 2: Decide which curve has higher values in between the points of intersections if there are more than two points of intersection then check this for each possible interval.

Step 3: Set up the integral for the area between the two curves using the difference of both curves.

Step 4: Evaluate the integral using integration techniques such as u-substitution or integration by parts.

Thus, the area between two curves is calculated.

Formula for Area between Two Curves

Let’s assume the two curves for which we need to calculate the area between them are f(x) and g(x) and for the required domain f(x) ≥ g(x). Then the formula for finding the area between two curves is given by:

Where,

• a and b are the limits of integration, which are the x-values where the curves intersect
• A is the area between both curves

If both the curves are defined as x₁ = f(y) and x₂ = g(y) and all the other assumptions remain the same as above, then the area between them is given as

Where,

• a and b are the limits of integration, which are the y-values where the curves intersect
• A is the area between both curves

Area Between Two Compound Curves

In the previous formulation, we assumed that f(x) ≥ g(x) in the interval [a, b]. But it’s not always the case, let’s consider another case, f(x) ≥ g(x) in [a, c] and f(x) ≤ g(x) in [c, b], here a < c < b. So, the bounded area in this region is given in the figure below, 

Area Required =

Area Between Two Polar Curves

Area between two polar curves can also be easily calculated using the same concept. The curve in polar coordinates is converted to a rectangle coordinate system.

Let’s take two polar curves r₀ = f(θ) and r_i = g(θ) as shown in the image added below, and the area enclosed between these two curves is found from α ≤ θ ≤ β where [α, β] is the bounded region. Now the area between the curves is given as,

A = 1/2 ∫_α ^β{(r₀)² – (r_i )²}.dθ

Sample Problems on Area Between Two Curves

Problem 1: Find the area bounded between two lines f(x) = 5x and g(x) = 3x from x =0 to x = 3.

Solution: 

The figure below shows both the lines, 

From the figure, we know

Area = 

⇒ Area = 

⇒ Area = 

⇒ Area = 

⇒ Area = 

⇒ Area = 9  sq. units

Problem 2: Find the area bounded between two curves f(x) = x³ and g(x) = x² between 0 and 1. 

Solution: 

The figure below shows both the curves, to find the bounded region, we first need to find the intersections. 

f(x) = g(x) 

⇒x³ = x²

⇒x²(x-1) = 0

⇒ x = 0 and 1

From the figure, we know

Area = 

⇒ Area = 

⇒ Area = 

⇒ Area = 

⇒ Area = 

⇒ Area = 1/3 – 1/4

⇒ Area = 1/12 sq. units

Problem 3: Find the area bounded between the parabola y² = 4x and x² + y² = 9. 

Solution: 

The figure below shows both the curves, to find the bounded region, we first need to find the intersections. 

x² + y² = 12

Figure 

Area = 

⇒ Area = 

⇒ Area = 

⇒ Area = 

⇒ Area = 

⇒ Area = 1/2 – 1/3

⇒ Area = 1/6  sq. units

Problem 4: Find the area bounded between the parabola y² = 4x and its latus rectum. 

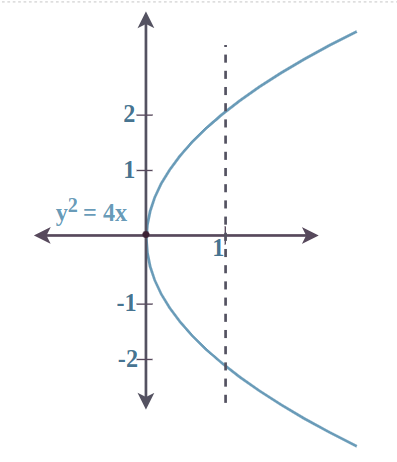
Solution: 

The figure below shows the parabola, and it’s latus rectum. Latus rectum is the line x = 1. We need to find the intersections, 

y² = 4 

y = 2 and -2

Area = 2(Area of the region bounded by the parabola and x = 1 and x-axis in the first quadrant) 

⇒ Area = 2()

⇒ Area = 2

⇒ Area = 4

⇒ Area = 4

⇒ Area = 

⇒ Area = 8/3 sq. units

Problem 5: The figure given below shows an ellipse 9x²+ y² = 36 and a chord PQ. Find the area enclosed between the chord and the ellipse in the first quadrant. 

Solution: 

The equation of ellipse is, 

⇒ 

So, now the equation of the chord becomes,

⇒ 

⇒ 3x + y = 6 

⇒ y = 6 – 3x

So, now the required area will be. 

A = 

⇒ A= 

⇒ A= 

⇒ A = 3π – 6 sq. units

FAQs on Area Between Two Curves

1. What is Area between two Curves.

The area between two curves is the region bounded by two curves in a plane and can be calculated by the integration of the absolute difference between both curves over the interval of interest.

2. How do you find the Area Between Two Curves?

To find the area between two curves, we need to integrate the absolute difference of both curves between the limit of integration which can be calculated using the intersection points of both graphs.

3. What is the Difference between Finding the Area Between Curves and Finding the Area Under a Curve?

The area between two curves is the area enclosed between two curves whereas the area under a curve is the area enclosed by the curve and x-axis between any given limits. Both can be calculated using the integration but in the area between curves, we need to calculate the points of intersection.

4. Can the Area Between Two Curves be Negative?

No, the area between two curves can’t be negative as it is the integral of the absolute difference of any two curves. Thus, it can have a positive value or zero value but not a negative value.

5. Can the area between two curves be infinite?

Yes, the area between two curves can be infinite if the curves intersect at infinity i.e., both curves have the same horizontal asymptote.

6. Can the Area between two curves be calculated if the curves do not intersect?

No, the area between two curves can’t be calculated if the curves don’t intersect, as there is no enclosed area between curves in such a case.