TCS Coding Practice Question | HCF or GCD of 2 Numbers
Last Updated :
27 Sep, 2022
Given two numbers, the task is to find the HCF of two numbers using Command Line Arguments. GCD (Greatest Common Divisor) or HCF (Highest Common Factor) of two numbers is the largest number that divides both of them. 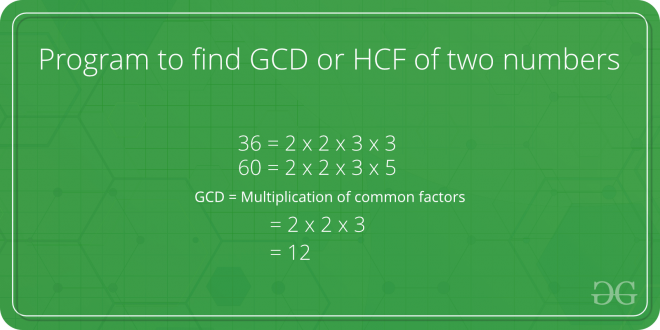 Examples:
Examples:
Input: n1 = 10, n2 = 20
Output: 10
Input: n1 = 100, n2 = 101
Output: 1
Approach:
- Since the numbers are entered as Command line Arguments, there is no need for a dedicated input line
- Extract the input numbers from the command line argument
- This extracted numbers will be in String type.
- Convert these numbers into integer type and store it in variables, say num1 and num2
- Find the HCF of the numbers. An efficient solution is to use Euclidean algorithm which is the main algorithm used for this purpose. The idea is, GCD of two numbers doesn’t change if smaller number is subtracted from a bigger number.
- Print or return the HCF
Program:
C
#include <stdio.h>
#include <stdlib.h> /* atoi */
int HCF(int a, int b)
{
if (b == 0)
return a;
return HCF(b, a % b);
}
int main(int argc, char* argv[])
{
int num1, num2;
if (argc == 1)
printf("No command line arguments found.\n");
else {
num1 = atoi(argv[1]);
num2 = atoi(argv[2]);
printf("%d\n", HCF(num1, num2));
}
return 0;
}
|
Java
class GFG {
static int HCF(int a, int b)
{
if (b == 0)
return a;
return HCF(b, a % b);
}
public static void main(String[] args)
{
if (args.length > 0) {
int num1 = Integer.parseInt(args[0]);
int num2 = Integer.parseInt(args[1]);
int res = HCF(num1, num2);
System.out.println(res);
}
else
System.out.println("No command line "
+ "arguments found.");
}
}
|
Output:
- In C:

- In Java:

Time Complexity: O(log(min(num1,num2)))
Auxiliary Space: O(1)
Share your thoughts in the comments
Please Login to comment...