Proof of De-Morgan’s laws in boolean algebra
Last Updated :
21 Sep, 2023
Statements :
1.

2.
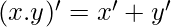
Proof:
Here we can see that we need to prove that the two propositions are complement to each other. We know that
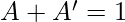
and
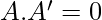
which are annihilation laws. Thus if we prove these conditions for the above statements of the laws then we shall prove that they are complement of each other.
For statement 1:
We need to prove that:
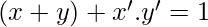
and
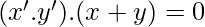
Case 1.
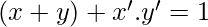
[Tex]LHS: (x+y)+x’.y’ =(x+y).(x+x’)+x’.y’
[/Tex]
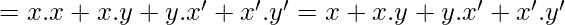
{Using distributive property}
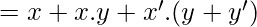
[Tex]=x+x.y+x’=x+x’+x.y
[/Tex]
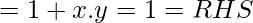
Hence proved.
Case 2.

[Tex]LHS: (x’.y’).(x+y)=x.(x’y’)+y.(x’.y’)
[/Tex]
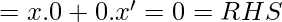
Hence proved.
For statement 2:
We need to prove that:
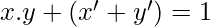
and

Case 1.
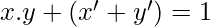
[Tex] LHS: x.y+(x’+y’)=(x+x’+y’).(y+x’+y’)
[/Tex]
{We know that A+BC=(A+B).(A+C)}
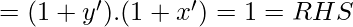
Hence proved.
Case 2.

[Tex]LHS: (x.y).(x’+y’)=x.x’.y+x.y.y’
[/Tex]
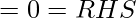
Hence Proved. This proves the De-Morgan’s theorems using identities of Boolean Algebra.
Proof of De-Morgan’s law of boolean algebra using Truth Table:
1) (x+y)’= x’. y’
x
| y
| x+y
| (x+y)’
| x’
| y’
| x’. y’
|
|
0
|
0
|
0
|
1
|
1
|
1
|
1
|
|
0
|
1
|
1
|
0
|
1
|
0
|
0
|
|
1
|
0
|
1
|
0
|
0
|
1
|
0
|
|
1
|
1
|
1
|
0
|
0
|
0
|
0
|
Hence the first De-morgan law of boolean algebra is proved (x+y)’= x’. y’
2) (x.y)’=x’+y’
x
| y
| x.y
| (x.y)’
| x’
| y’
| x’+y’
|
|
0
|
0
|
0
|
1
|
1
|
1
|
1
|
|
0
|
1
|
0
|
1
|
1
|
0
|
1
|
|
1
|
0
|
0
|
1
|
0
|
1
|
1
|
|
1
|
1
|
1
|
0
|
0
|
0
|
0
|
Hence the second De-morgan law of boolean algebra is proved (x.y)’=x’+y’
Share your thoughts in the comments
Please Login to comment...