Class 9 RD Sharma Solutions – Chapter 2 Exponents of Real Numbers- Exercise 2.2 | Set 2

Question 12. Determine (8x)^x, if 9^x+2 = 240 + 9^x.

Solution:

We have,

=> 9^x+2 = 240 + 9^x

=> 9^x+2 − 9^x = 240

=> 9^x (9² − 1) = 240

=> 9^x = 240/80

=> 3^2x = 3

=> 2x = 1

=> x = 1/2

Therefore, (8x)^x = [8 × (1/2)]^1/2

= 4^1/2

= 2

Question 13. If 3^x+1 = 9^x−2, find the value of 2^1+x.

Solution:

We have,

=> 3^x+1 = 9^x−2

=> 3^x+1 = (3²)^x−2

=> 3^x+1 = 3^2x−4

=> x + 1 = 2x − 4

=> x = 5

Therefore, 2^1+x = 2¹⁺⁵

= 2⁶

= 64

Question 14. If 3^4x = (81)⁻¹ and (10)^1/y = 0.0001, find the value of 2^−x+4y.

Solution:

We are given,

=> 3^4x = (81)⁻¹

=> 3^4x = (3⁴)⁻¹

=> 3^4x = (3)⁻⁴

=> 4x = −4

=> x = −1

And also, (10)^1/y = 0.0001

=> (10)^1/y = (10)⁻⁴

=> 1/y = −4

=> y = −1/4

Therefore, 2^−x+4y = 2^1+4(−1/4) 

= 2¹⁻¹ 

= 1

Question 15. If 5^3x = 125 and 10^y = 0.001. Find x and y.

Solution:

We are given,

=> 5^3x = 125

=> 5^3x = 5³

 => 3x = 3

=> x =1

Also, (10)^y = 0.001

=> 10^y = 10⁻³

=> y = −3

Therefore, the value of x is 1 and the value of y is –3.

Question 16. Solve the following equations:

(i) 3^x+1 = 27 × 3⁴

Solution:

We have,

=>  3^x+1 = 27 × 3⁴

=> 3^x+1 = 3³ × 3⁴

=> 3^x+1 = 3⁷

=> x + 1 = 7

=> x = 6

(ii) 

Solution:

We have,

=> 

=> 

=> 

=> 

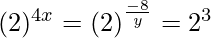
=> 4x = −8/y = 3

=> x = 3/4 and y = −8/3

(iii) 3^x−1 × 5^2y−3 = 225

Solution:

We have,

=> 3^x−1 × 5^2y−3 = 225

=> 3^x−1 × 5^2y−3 = 3² × 5²

=> x − 1 = 2 and 2y − 3 = 2 

=> x = 3 and 2y = 5

=> x = 3 and y = 5/2

(iv) 8^x+1 = 16^y+2 and (1/2)^3+x = (1/4)^3y

Solution:

We have,

=> 8^x+1 = 16^y+2

=> (2³)^x+1 = (2⁴)^y+2

=> 2^3x+3 = 2^4y+8

=> 3x + 3 = 4y + 8  . . . . (1)

Also, (1/2)^3+x = (1/4)^3y

=> (1/2)^3+x = [(1/2)²]^3y

=> (1/2)^3+x = (1/2)^6y

=> 3 + x = 6y

=> x = 6y − 3   . . . . (2)

Putting (2) in (1), we get,

=> 3(6y − 3) + 3 = 4y + 8

=> 18y − 9 + 3 = 4y + 8

=> 14y = 14

=> y = 1

Putting y = 1 in (2), we get,

x = 6(1) − 3 = 6 − 3 = 3

Therefore, the value of x is 1 and the value of y is –3.

(v) 4^x−1 × (0.5)^3−2x = (1/8)^x

Solution:

We have,

=> 4^x−1 × (0.5)^3−2x = (1/8)^x

=> (2²)^x−1 × (1/2)^3−2x = [(1/2)³]^x

=> 2^2x−2 × 2^2x−3 = 2^−3x

=> 2^{2x−2+2x−3} = 2^−3x

=> 2^4x−5 = 2^−3x

=> 4x − 5 = −3x

=> 7x = 5

=> x = 5/7

(vi) 

Solution:

We have,

=> 

=> 

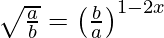
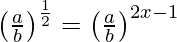
=> 1/2 = 2x − 1

=> 2x = 3/2

=> x = 3/4

Question: 17. If a and b are distinct positive primes such that,  find x and y.

Solution:

We have,

=> 

=> (a⁶ b⁻⁴)^1/3 = a^xb^2y

=> a^6/3 b^−4/3 = a^xb^2y

=> a² b^−4/3 = a^xb^2y

=> x = 2 and 2y = −4/3

=> x = 2 and y = −2/3

Question 18. If a and b are different positive primes such that,

(i) 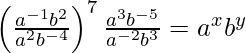, find x and y.

Solution:

We have,

=> 

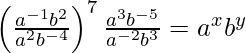
=> (a⁻¹⁻² b²⁺⁴)⁷ ÷ (a³⁺² b⁻⁵⁻³) = a^xb^y

=> (a⁻³ b⁶)⁷ ÷ (a⁵ b⁻⁸) = a^xb^y

=> (a⁻²¹ b⁴²) ÷ (a⁵ b⁻⁸) = a^xb^y

=> (a⁻²¹⁻⁵ b⁴²⁺⁸) = a^xb^y

=> (a⁻²⁶ b⁵⁰) = a^xb^y

=> x = −26, y = 50

(ii) (a + b)⁻¹(a⁻¹ + b⁻¹) = a^xb^y, find x+y+2.

Solution:

We have,

=> (a + b)⁻¹(a⁻¹ + b⁻¹) = a^xb^y

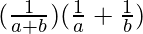=>  = a^xb^y

=>  = a^xb^y

=> 1/ab = a^xb^y

=> a⁻¹b⁻¹ = a^xb^y

=> x = −1 and y = −1

So, x+y+2 = −1−1+2 = 0.

Question 19. If 2^x × 3^y × 5^z = 2160, find x, y and z. Hence compute the value of 3^x × 2^−y × 5^−z.

Solution:

We are given,

=> 2^x × 3^y × 5^z = 2160

=> 2^x × 3^y × 5^z = 2⁴ × 3³ × 5¹

=> x = 4, y = 3, z = 1

Therefore, 3^x × 2^−y × 5^−z = 3⁴ × 2⁻³ × 5⁻¹

= (81) (1/8) (1/5)

= 81/40

Question 20. If 1176 = 2^a × 3^b × 7^c, find the values of a, b and c. Hence, compute the value of 2^a × 3^b × 7^-c as a fraction.

Solution:

We are given,

=> 1176 = 2^a × 3^b × 7^c

=> 2³ × 3¹ × 7² = 2a × 3b × 7c

=> a = 3, b = 1, c = 2 

Therefore, 2^a × 3^b × 7^−c = 2³ × 3¹ × 7⁻²

= (8) (3) (1/49)

= 24/49

Question 21. Simplify

(i) 

Solution:

We have,

= 

= (x^a+b−c)^a−b (x^b+c−a)^b−c (x^c+a−b)^c−a

= 

= 

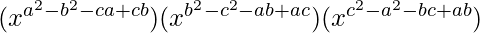
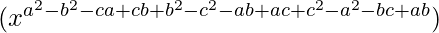
= x⁰

= 1

(ii) 

Solution:

We have,

=> 

=> 

=> 

=> 

=> 

=> 

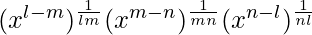
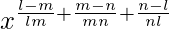
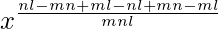
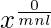
=> x⁰

= 1

Question 22. Show that .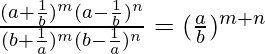

Solution:

We have,

L.H.S. = 

= 

= 

= 

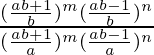
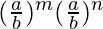
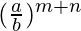
= R.H.S.

Hence proved.

Question 23. (i) If a = x^m+ny^l, b = x^n+ly^m and c = x^l+myⁿ, prove that a^m−n b^n−l c^l−m = 1.

Solution:

Given, a = x^m+ny^l, b = x^n+ly^m and c = x^l+myⁿ. 

We have,

L.H.S. = a^m−n b^n−l c^l−m

= (x^m+ny^l)^m−n(x^n+ly^m)^n−l(x^l+myⁿ)^l−m

= 

= 

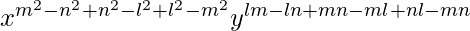
= x⁰y⁰

= 1

= R.H.S.

Hence proved.

(ii) If x = a^m+n, y = a^n+l and z = a^l+m, prove that x^myⁿz^l = xⁿy^lz^m.

Solution:

Given, x = a^m+n, y = a^n+l and z = a^l+m.

We have,

L.H.S. = x^myⁿz^l

= (a^m+n)^m (a^n+l)ⁿ (a^l+m)^l

= 

= 

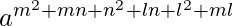
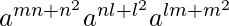
= (a^m+n)ⁿ (a^n+l)^l (a^l+m)^m

= xⁿy^lz^m

= R.H.S.

Hence proved.