This exercise has been deleted as per new NCERT Syllabus
Question 1. The ages of two friends Ani and Biju differ by 3 years. Ani’s father Dharam is twice as old as Ani and Biju is twice as old as his sister Cathy. The ages of Cathy and Dharam differ by 30 years. Find the ages of Ani and Biju.
Solution:
The age difference between Ani and Biju = 3 yrs.
Case 1: Either Biju is 3 years older than that of Ani,
y – x = 3
Case 2: or Ani is 3 years older than Biju.
x – y = 3
Given, Ani’s father’s age is 30 yrs more than that of Cathy’s age.
Let’s take,
Ani’s age = x
and Biju’s age = y
So, Dharam’s age will be = 2x
And the age of Biju sister (Cathy) will be = [Tex]\frac{y}{2}[/Tex]
CASE 1 (y > x)
According to the given condition,
y – x = 3 -(1)
and, 2x−y/2 = 30
Multiply it by 2, we get
4x – y = 60 -(2)
Add eq(1) and (2)
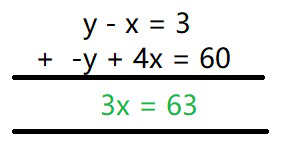
3x = 63
x = 63/3
x = 21
Now putting x = 21 in eq(1), we get
y – 21 = 3
y = 24
Therefore, the age of Ani = 21 years
And the age of Biju is = 24 years.
CASE 2: (x > y)
According to the given condition,
x – y = 3 -(1)
and, 2x−y/2 = 30
Multiply it by 2, we get
4x – y = 60 -(2)
Now, by using Elimination method,
Subtract eq(1) from eq(2)
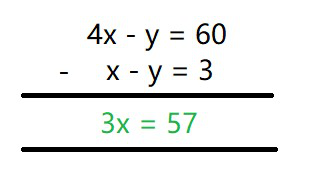
3x = 57
x = 57/3
x = 19
Now putting x = 19 in eq(1), we get
19 – y = 3
y = 19 – 3
y = 16
Therefore, the age of Ani = 19 years
And the age of Biju is = 16 years.
Question 2. One says, “Give me a hundred, friend! I shall then become twice as rich as you”. The other replies, “If you give me ten, I shall be six times as rich as you”. Tell me what is the amount of their (respective) capital? [From the Bijaganita of Bhaskara II]
[Hint : x + 100 = 2(y – 100), y + 10 = 6(x – 10)].
Solution:
Let there be two persons as A and B.
Let’s take,
Money person A has = ₹ x
Money person B has = ₹ y
So, According to the given conditions, we have
x + 100 = 2(y – 100)
x – 2y = – 300 -(1)
And
6(x – 10) = (y + 10)
6x -y = 70 -(2)
Now, by using Elimination method,
Multiply eq(2) by 2 and subtract eq(1) from it
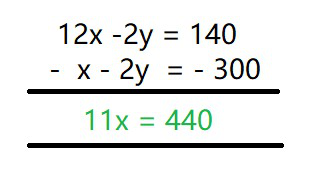
11x = 440
x = 40
Now putting x = 40 in eq(1), we get
40 – 2y = – 300
2y = 300 + 40
y = 340/2
y = 170
Hence,
Person A had Rs 40 and person B had Rs 170 with them.
Question 3. A train covered a certain distance at a uniform speed. If the train would have been 10 km/h faster, it would have taken 2 hours less than the scheduled time. And, if the train were slower by 10 km/h; it would have taken 3 hours more than the scheduled time. Find the distance covered by the train.
Solution:
Let the speed of the train = x km/hr
The time taken by the train to travel a distance = t hours
The distance to travel = d km.
As we know,
Distance traveled by train = Speed of the train × Time taken to travel that distance
d = xt -(1)
According to the given equation,
d = (x + 10) × (t – 2)
d = xt + 10t – 2x – 20
as d = xt, we get
10t – 2x = 20 -(2)
and 2nd condition,
d = (x – 10) × (t + 3)
d = xt – 10t + 3x – 30
as d = xt, we get
10t – 3x = -30 -(3)
Now, by using Elimination method,
Subtract eq(3) from eq(2), we get
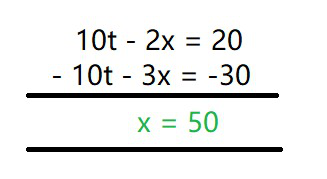
x = 50 km/h
Now putting x = 50 in eq(1), we get
10t – 2(50) = 20
10t = 120
t = 12 hours
Now, distance will be = 50 × 12 = 600 km
Hence, the distance covered by the train is 600 km.
Question 4. The students of a class are made to stand in rows. If 3 students are extra in a row, there would be 1 row less. If 3 students are less in a row, there would be 2 rows more. Find the number of students in the class.
Solution:
Let the number of rows = x
and, the number of students in a row = y.
Total number of students = Number of rows x Number of students in a row = xy -(1)
Here, total no. of student will be same always
According to the given condition,
Total number of students = (x – 1)(y + 3)
xy = (x – 1)(y + 3)
xy = xy – y + 3x – 3
3x – y = 3 -(2)
and,
Total Number of students = (x + 2 ) ( y – 3 )
xy = xy + 2y – 3x – 6
3x – 2y = -6 -(3)
Now, by using Elimination method,
Subtract eq(3) from eq(2), we get
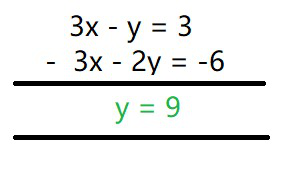
y = 9
Now putting y = 9 in eq(1), we get
3x – 9 = 3
3x = 12
x = 4
Number of total students in a class = xy
= 4 x 9
= 36
Hence, No. of students = 36
Question 5. In a ∆ABC, ∠C = 3 ∠B = 2 (∠A + ∠B). Find the three angles.
Solution:
Here, according to the given conditions
3 ∠B = 2 (∠A + ∠B)
3 ∠B = 2∠A + 2∠B
∠B = 2∠A -(1)
and, ∠C = 3∠B
∠C = 3 (2∠A)
∠C = 6∠A -(2)
As we know,
∠A + ∠B + ∠C = 180° (Angle sum property)
∠A + (2∠A) + (6∠A) = 180° -(From eq(1) and eq(2))
9∠A = 180
∠A = 20°
∠B = 2∠A = 2(20°) = 40°
∠C = 6∠A = 6(20°) = 120°
Hence, the three angles are 20°, 40° and 120°.
Question 6. Draw the graphs of the equations 5x – y = 5 and 3x – y = 3. Determine the coordinates of the vertices of the triangle formed by these lines and the y axis.
Solution:
5x – y = 5 -(1)
3x – y = 3 -(2)
y-axis, x = 0 -(3)
To get the co-ordinates of the vertices of the triangle formed, lets get there intersection point,
Intersection of eq(1) and (2)
Now, by using Elimination method,
Subtract eq(2) from (1), we get
2x = 2
x = 1
Now putting x = 1 in eq(1), we get
5(1) – y = 5
y = 0
So, Intersection of eq(1) and (2) vertices are (1, 0)
Now, Intersection of eq(1) and (3)
As x =0 substitutes it in eq(1), we get
5(0) – y = 5
y = -5
So, Intersection of (I) and (III) vertices are (0, -5)
Now, Intersection of eq(2) and (3)
As x =0 substitutes it in eq(2), we get
3(0) – y = 3
y = -3
So, Intersection of eq(2) and (3) vertices are (0,-3)

Hence, the co-ordinates of the vertices of the triangle formed are (1, 0), (0, -5) and (0, -3)
Question 7. Solve the following pair of linear equations:
(i) px + qy = p – q
qx – py = p + q
Solution:
px + qy = p – q -(1)
qx – py = p + q -(2)
Multiplying p to equation (1) and q to equation (2), we get
p2x + pqy = p2 − pq -(3)
q2x − pqy = pq + q2 -(4)
Add eq(3) and (4), we get
p2x + q2 x = p2 + q2
(p2 + q2) x = p2 + q2
x = [Tex]\frac{(p^2 + q^2)}{p^2 + q^2} [/Tex]
x = 1
Now putting x = 1 in eq(1), we get
p(1) + qy = p – q
qy = p-q-p
qy = -q
y = -1
(ii) ax + by = c
bx + ay = 1 + c
Solution:
ax + by = c -(1)
bx + ay = 1 + c -(2)
Multiplying a to equation (1) and b to equation (2), we get
a2x + aby = ac -(3)
b2x + aby = b + bc -(4)
Subtract equation eq(4) from equation (3),
(a2 – b2) x = ac − bc– b
x = [Tex]\frac{(ac − bc– b)}{(a^2 – b^2)}[/Tex]
x = [Tex]\frac{(c(a-b) –b)}{(a^2 – b^2)}[/Tex]
Now putting the value of x in eq(1), we get
ax + by = c
[Tex]a{\frac{(c(a-b) –b)}{(a^2 – b^2)}} [/Tex] +by = c
[Tex]\frac{(ac(a-b) –b)}{(a^2 – b^2)} [/Tex]+by = c
by = [Tex]\frac{c–ac(a−b)−ab}{(a^2 – b^2)}[/Tex]
by = [Tex]\frac{(c–ac(a−b)−ab)}{(a^2 – b^2)}[/Tex]
y = [Tex]\frac{(c(a-b)+a)}{(a^2 – b^2)}[/Tex]
(iii) [Tex]\frac{x}{a} – \frac{y}{b} = 0[/Tex]
ax + by = a2+ b2.
Solution:
[Tex]\frac{x}{a} – \frac{y}{b} = 0 [/Tex] -(1)
Multiplying equation (1) with ab, we get
bx − ay = 0 -(new 1)
Multiplying a and b to equation (1) and (2) respectively, we get
b2x − aby = 0 -(3)
a2x + aby = a3 + ab3 -(4)
Add eq(3) and (4), we get
b2x + a2x = a3 + ab2
x (b2 + a2) = a (a2 + b2)
x = a
Now putting x = a in eq(1), we get
b(a) − ay = 0
ab − ay = 0
ay = ab,
y = b
(iv) (a – b)x + (a + b) y = a2– 2ab – b2
(a + b)(x + y) = a2+ b2
Solution:
(a – b)x + (a + b) y = a2– 2ab – b2 -(1)
(a + b)(x + y) = a2+ b2 -(2)
Subtract equation (2) from equation (1), we get
(a − b) x − (a + b) x = (a2 − 2ab − b2) − (a2 + b2)
x(a − b − a − b) = − 2ab − 2b2
− 2bx = − 2b (b + a)
x = b + a
Substituting, x = b + a in equation (1), we get
(a + b)(a − b) +y (a + b) = a2− 2ab – b2
a2 − b2 + y(a + b) = a2− 2ab – b2 -(Using the identity (a + b)(a – b) = a2 – b2)
(a + b) y = − 2ab
y = [Tex]\frac{-2ab}{a+b}[/Tex]
(v) 152x – 378y = – 74
–378x + 152y = – 604
Solution:
x = [Tex]\frac{(189y-137)}{76} [/Tex] -(1)
− 378x + 152y = − 604
Dividing it by 2, we get
− 189x + 76y = − 302 -(2)
Substitute x in equation (2), we get
−189[Tex](\frac{(189y-137)}{76}) [/Tex]+76y=−302
− (189)2y + 189 × 37 + (76)2 y = − 302 × 76
189 × 37 + 302 × 76 = (189)2 y − (76)2y
6993 + 22952 = (189 − 76) (189 + 76) y
29945 = (113) (265) y
y = 1
Using equation(1), we get
x = [Tex]\frac{189-37}{76}[/Tex]
x = 152/76
x = 2
Question 8. ABCD is a cyclic quadrilateral (see Figure). Find the angles of the cyclic quadrilateral.

Solution:
It is known that the sum of the opposite angles of a cyclic quadrilateral is 180°
Thus, we have
∠C +∠A = 180
4y + 20− 4x = 180
− 4x + 4y = 160
x − y = − 40 -(1)
And, ∠B + ∠D = 180
3y − 5 − 7x + 5 = 180
− 7x + 3y = 180 -(2)
Multiplying 3 to equation (1), we get
3x − 3y = − 120 -(3)
Adding equation (2) to equation (3), we get
− 7x + 3x = 180 – 120
− 4x = 60
x = −15
Substituting this value in equation (1), we get
x − y = − 40
-y−15 = − 40
y = 40-15
y = 25
∠A = 4y + 20 = 20 + 4(25) = 120°
∠B = 3y − 5 = − 5 + 3(25) = 70°
∠C = − 4x = − 4(− 15) = 60°
∠D = 5 – 7x
∠D= 5 − 7(−15) = 110°
Hence, ∠A, ∠B, ∠C and ∠D are equal to 120°, 70°, 60° and 110° respectively.
Share your thoughts in the comments
Please Login to comment...