Derivative Formulas in Calculus

Derivative Formulas in Calculus are one of the important tools of calculus as Derivative formulas are widely used to find derivatives of various functions with ease and also, help us explore various fields of mathematics, engineering, etc.

This article explores all the derivative formulas closely including the general derivative formula, derivative formulas for logarithmic and exponential functions, derivative formulas for trigonometric ratios, derivative formulas for inverse trigonometric ratios, and derivative formulas for hyperbolic functions. Derivative Formula is important for Class 12 students for their Board Exams. We will also solve some examples of derivatives using the different derivative formulas. Let’s closely traverse the topic of Derivative Formula.

What is Derivative?

The derivatives represent the rate of function with respect to any variable. The derivative of a function f(x) is denoted as f'(x) or (d/dx) [f(x)]. The process of finding derivatives is called differentiation.

The most fundamental derivative formula is the definition of a derivative, which is defined as:

f'(x) = lim_h→0 [(f(x + h) – f(x))/h]

There are various derivative formulas including general derivative formulas, derivative formulas for trigonometric functions, and derivative formulas for inverse trigonometric functions, etc.

What are Derivative Formulas?

Derivative Formulas are those mathematical expressions which help us calculate the derivative of some specific function with respect to its independent variable. In simple words, the formulas which helps in finding derivatives are called as derivative formulas. There are multiple derivative formulas for different functions.

Examples of Derivative Formula

Some examples of formulas for derivatives are listed as follows:

• Power Rule: If f(x) = xⁿ, where n is a constant, then the derivative is given by:

f'(x) = nx^n-1

• Constant Rule: If f(x) = c, where c is a constant, then the derivative is zero:

f'(x) = 0

• Exponential Functions: If f(x) = e^x, then:

f'(x) = e^x

Let’s discuss all the Formulas related to Derivative in a structured manner.

Basic Derivative Formulas – Derivative Rules in Calculus

Some of the most basic formulas to find derivative are:

• Constant Rule
• Power Rule
• Sum Difference Rule
• Product Rule
• Quotient Rule
• Chain Rule

Let’s discuss these rules in detail:

Constant Rule for Derivatives

The constant rule for derivatives is given by:

(d/dx) constant = 0

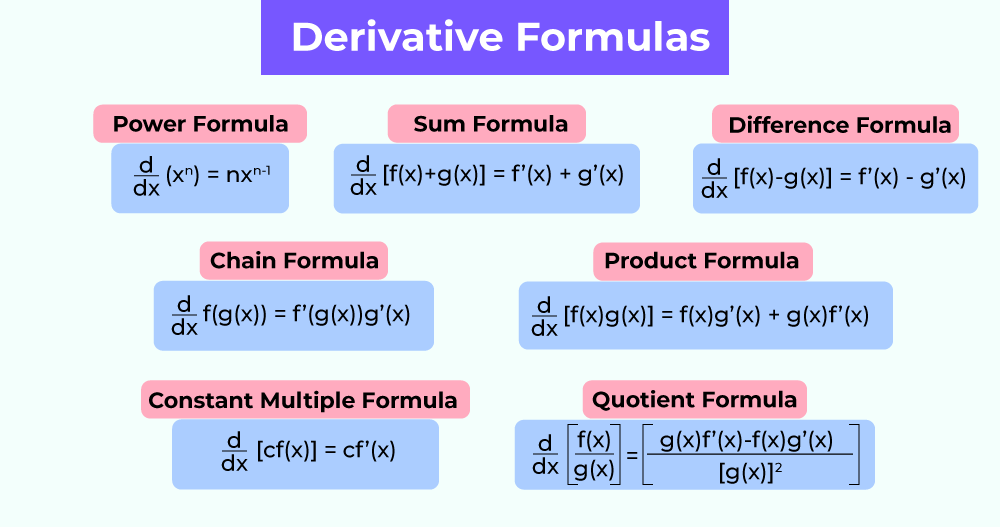
Power Rule for Derivatives

The power rule for derivatives is given by:

(d/dx) xⁿ = nx^n-1

Sum Difference Rule for Derivatives

The sum and difference rule for derivatives is given by:

(d/dx) [f(x) ± g(x)] = (d/dx) f(x) ± (d/dx) g(x)

Product Rule for Derivatives

The product rule for derivatives is given by:

(d/dx) [f(x). g(x)] = f'(x). g(x) + f(x). g'(x)

Quotient Rule for Derivatives

The quotient rule for derivatives is given by:

(d/dx) [f(x)/g(x)] = [f'(x). g(x) – f(x). g'(x)]/[g(x)]²

Chain Rule for Derivatives

The chain rule for derivative is given by:

(d/dx) [f(g(x))] = (d/dx) [f(g(x))] × (d/dx) [g(x)]

List of Derivative Formulas

The derivative formulas for the different functions are listed below:

Exponential and Logarithmic Derivative Formulas

The derivative formulas for the exponential and logarithmic functions are listed below:

• (d/dx) e^x = e^x
• (d/dx) a^x = a^x ln a
• (d/dx) ln x = (1/x)
• (d/dx) log_ax= (1/x lna)

Read More,

• Logarithms
• Derivative of Exponential Functions

Trigonometric Derivative Formulas

The derivative formulas for the trigonometric functions are listed below:

• (d/dx) sin x = cos x
• (d/dx) cos x = -sin x
• (d/dx) tan x = sec² x
• (d/dx) cot x = -cosec²x
• (d/dx) sec x = sec x tan x
• (d/dx) cosec x = – cosec x cot x

Learn more about Differentiation of Trigonometric Functions.

Derivative Formula for Inverse Trigonometric Functions

The derivative formulas for the inverse trigonometric functions are listed below:

• (d/dx) sin^-1 x = 1/[√(1 – x²)]
• (d/dx) cos^-1 x = 1/[√(1 – x²)]
• (d/dx) tan^-1 x = 1/(1 + x²)
• (d/dx) cot^-1 x = -1/(1 + x²)
• (d/dx) sec^-1 x = 1/[|x|√(x² – 1)]
• (d/dx) cosec^-1 x = -1/[|x|√(x² – 1)]

Read more, Derivative of Inverse Trig Functions.

Derivative of Hyperbolic Functions

The derivative formulas for the trigonometric functions are listed below:

• (d/dx) sinh x = cosh x
• (d/dx) cosh x = sinh x
• (d/dx) tanh x = sech² x
• (d/dx) coth x = -cosech²x
• (d/dx) sech x = -sech x tanh x
• (d/dx) cosech x = -cosech x coth x

Some Other Derivative Formulas

There are some other functions like implicit functions, parametric functions and higher order derivatives whose derivative formulas are listed below:

Implicit Derivative Formula

The method of finding the derivative of an implicit function is called implicit differentiation. Let’s take an example to understand implicit differentiation.

Example: Find derivative of xy = 2

Solution:

(d/dx) [xy] = (d/dx) 2

⇒ x(dy/dx) + y(dx/dx) = 0

⇒ x(dy/dx) + y(1) = 0

⇒ x(dy/dx) + y = 0

⇒ x(dy/dx) = -y

⇒ (dy/dx) = -y/x

From given equation y = 2/x

(dy/dx) = -(2/x)/x

⇒ (dy/dx) = -(2/x²)

Learn more about Implicit Differentiation.

Parametric Derivative Formula

If the function y(x) is expressed in the terms of third variable t and x and y can be represented in the x = f(t) and y = g(t) then, this type of function is called as parametric function.

If y is function of x and x = f(t) and y = g(t) are two differentiable functions of parameter t then, derivative of parametric function is given by:

(dy/dx) = (dy/dt)/(dx/dt), such that (dx/dt) ≠ 0

Read more about Parametric Differentiation.

Higher Order Derivative Formula

Finding the derivative of a function for more than one time gives the higher order derivative of a function.

n^th Derivative = dⁿy/(dx)ⁿ

Read more about Higher Order Derivative.

How to find the Derivatives?

To find the derivatives of a function we follow the below steps:

• First check the type of the function whether it is algebraic, trigonometric etc.
• After finding the type apply the corresponding derivative formulas on the function.
• The resultant value gives the derivative of the function using the derivatives formula.

Applications of Derivative Formula

There are many applications of the derivative formulas. Some of these applications are listed below:

• Derivatives are used to find the rate of change in any quantity.
• It can be used to find maxima and minima.
• It is used in increasing and decreasing functions.

Read More,

• Differentiation Formulas
• Differentiation and Integration Formula
• Logarithmic Differentiation

Derivative Rules in Calculus

Solved Examples on Derivative Formula

Example 1: Find the derivative of x⁵.

Solution:

Let y = x⁵

⇒ y’ = (d/dx) [x⁵]

⇒ y’ = 5(x^5-1)

⇒ y’ = 5x⁴

Example 2: Find the derivative of log₂x.

Solution:

Let y = log₂x

⇒ y’ = (d/dx) [log₂x]

⇒ y’ = 1/ [x ln2]

Example 3: Find the derivative of the function f(x) = 8 . 6^x

Solution:

f(x) = 8 . 6^x

⇒ f'(x) = (d/dx) [8 . 6^x]

⇒ f'(x) = 8 . (d/dx) [6^x]

⇒ f'(x) = 8[6x ln 6]

Example 4: Find the derivative of the function f(x) = 3sinx + 2x

Solution:

f(x) = 3 sinx + 2x

⇒ f'(x) = (d/dx)[3 sinx + 2x]

⇒ f'(x) = (d/dx)[3 sinx] + (d/dx)[2x]

⇒ f'(x) = 3(d/dx)[sinx] + 2(d/dx)(x)

⇒ f'(x) = 3 cosx + 2(1)

⇒ f'(x) = 3 cosx + 2

Example 5: Find the derivative of the function f(x) = 5cos^-1x + e^x

Solution:

f(x) = 5cos^-1x + e^x

⇒ f'(x) = (d/dx)[5cos^-1x + e^x]

⇒ f'(x) = (d/dx)[5cos^-1x] + (d/dx)[e^x]

⇒ f'(x) = 5(d/dx)[cos^-1x] + (d/dx)[e^x]

⇒ f'(x) = 5[-1/√(1 – x²)] + e^x

⇒ f'(x) = [-5/√(1 – x²)] + e^x

Practice Problems on Derivative Formula

Problem 1: Evaluate: (d/dx) [x⁴].

Problem 2: Find the derivative of y = 5cos x.

Problem 3: Find the derivative of y = cosec x + cot x.

Problem 4: Find the derivative of f(x) = 4^x + log₃x + tan^-1 x.

Problem 5: Evaluate: (d/dx) [40].

Problem 6: Find the derivative of f(x) = x⁵ + 5x³ + 1 .

Derivative Formula – FAQs

What is Derivative?

The value that represents the rate of change of function with respect to any variable is called the derivative.

How are the Derivatives Represented?

The derivatives are represented as (d/dx) or if f(x) is a function then, derivative of f(x) is represented as f'(x).

How is the Derivative of a Constant Calculated?

The derivative of a constant is always zero. In mathematical notation, if ‘C’ is a constant, then dC/dx = 0.

Write the General Derivative Formula of xⁿ.

The general formula for derivative of xⁿ = nx^n-1.

How to Calculate the Derivatives of Function?

To calculate the derivatives of a function, we can apply derivatives formula according to given function.

What is the Formula for Derivative of Logarithmic Function?

The derivative of the natural logarithm function, ln(x), is 1/x. In mathematical notation, if y = ln(x), then dy/dx = 1/x.

Which Formula is used to Find Derivative of Exponential Functions?

The derivative of an exponential function, y = a^x (where ‘a’ is a constant), is found using the formula dy/dx = a^x × ln(a).

What are Higher-Order Derivatives?

Higher-order derivatives are derivatives of a function taken more than once. The second derivative is the derivative of the first, the third is the derivative of the second, and so on.

What is Derivative Formula for e^x?

The derivative of the function f(x) = e^x (where ‘e’ is Euler’s number, approximately 2.71828) is simply f'(x) = e^x.

Write Derivative Formula for u/v.

The derivative of the quotient of two functions u(x) and v(x) is given by the quotient rule:

d(u/v)/dx = (v × du/dx – u × dv/dx)/(v²)

What is Derivative Formula for 1/x?

The derivative of the function f(x) = 1/x is given by:

f'(x) = -1/x²