Differentiation and Integration Formula

Differentiation and Integration are two mathematical operations used to find change in a function or a quantity with respect to another quantity instantaneously and over a period, respectively. Differentiation is an instantaneous rate of change and it breaks down the function for that instant with respect to a particular quantity while Integration is the average rate of change that causes the summation of continuous data of a function over the given period or range. Both are inverse of each other. The concept of Differentiation and Integration can be best understood by the following example:

Let’s say you are traveling from A to B, then the speed at each instant between A and B is given by differentiating the distance with that particular instant of time, but you can’t keep noting down the speed at each instant hence if someone asks you with what speed you completed your journey then you need to tell a single speed magnitude and not the speed of each instant, hence for this you will integrate all the data of velocities from the initial time to final time.

What is Differentiation?

Differentiation is a method to find the instantaneous rate of change of a function or curve with respect to other quantities. Mathematically, the Slope of the tangent at a point on the curve is called the Derivative of the Curve or Function and differentiation is a method to find that derivative. In differentiation, we compute the rate at which a dependent variable ‘y‘ changes with respect to the change in the independent variable ‘x’. This rate of change is called the derivative of ‘y’ with respect to ‘x’, where y is a function of x given as y = f(x).

How to Differentiate a Function

The differentiation of a function is simply the Derivative of the Function at all differentiable points in its domain. For Example, if f(x) is differentiable at x = a in its domain. The Derivative of f(x) at x = a is given as

[Tex]\bold{\left.\frac{d}{dx}(f(x))\right|_{x=a} =f'(a)=\lim_{h\rightarrow 0}\frac{f(a+h)-f(a)}{h}}[/Tex]

where h is the change in independent variable x.

For example, if need to find differentiation of a function f(x) = x².

Then differentiation of the function is given as f'(x) = 2x.

Here, in the above step, we reduce the power to 1.

Derivative of Some Standard Functions

The derivative of standard functions can be found by the formulas. We will learn the Differentiation formulas of the following functions:

• Algebraic Function
• Exponential Function
• Logarithmic Function
• Trigonometric Function

Derivative of Algebraic Functions

y = f(x)	dy/dx
[Tex]\frac{d}{dx}(x^n)[/Tex]	[Tex]nx^{n-1}[/Tex]
[Tex] \frac{d}{dx} (\frac{1}{x})[/Tex]	[Tex]-\frac{1}{x^2}[/Tex]
[Tex]\frac{d}{dx} (√x) [/Tex]	[Tex]\frac{1}{2√x}[/Tex]

Derivative of Exponential Functions

y = f(x)	dy/dx
[Tex]\frac{d}{dx} (e^x)[/Tex]	ex
[Tex] \frac{d}{dx} (a^x) [/Tex]	ax logea

Derivative of Logarithmic Functions

y = f(x)	dy/dx
[Tex] \frac{d}{dx} ( log_ex) [/Tex]	1/x
[Tex]\frac{d}{dx} ( log_ax)[/Tex]	[Tex]\frac{1}{x log_ea}[/Tex]

Learn more about logarithmic differentiation.

Derivative of Trigonometric Functions

y = f(x)	dy/dx
[Tex]\frac{d}{dx} (sin x)               [/Tex]	cos x
[Tex]\frac{d}{dx}( cos x)               [/Tex]	-sin x
[Tex]\frac{d}{dx} ( tan x)               [/Tex]	sec2x
[Tex]\frac{d}{dx} (cot x)[/Tex]	-cosec2x
[Tex]\frac{d}{dx} (sec x)                [/Tex]	sec x.tan x
[Tex]\frac{d}{dx} (cosec x)               [/Tex]	-cosec x.cot x

The above formulas are used when the functions are present alone or when multiplied by a scalar number but when two functions are in product form or quotient form then we can’t simply differentiate each function separately but we need to follow some rules, particularly for product and quotient case. Hence, we will look at differentiation by parts.

Differentiation by Parts

In this we will learn the formulas for differentiation when two functions are in product or quotient form:

Differentiation of Product of Functions: Let us assume ‘u’ and ‘v’ are two functions in the product (u.v) then the Differentiation of u.v is given by product rule i.e.,

[Tex]\bold{\frac{d}{dx}(u.v)=u\frac{dv}{dx}+v\frac{du}{dx}}[/Tex]

Differentiation of Quotient of Functions: Let us assume ‘u’ and ‘v’ are two functions in the quotient (u/v) then the Differentiation of  u/v is given by quotient rule i.e.,

[Tex]\bold{\frac{d}{dx}(\frac{u}{v} ) = \frac{v\frac{du}{dx} – u \frac{dv}{dx}}{v^2}}[/Tex]

What is Integration?

Integration is a method to find the average rate of change of a function. As the name suggests, Integrate means adding all the functions’ points. Integration is actually anti-derivative of differentiating function. Differentiation and Integration are inverses of each other. We can integrate the function in two ways one is indefinite and the other is definite. In Indefinite Integration, we get a constant C with our expression but in Definite we are able to find the value of that constant C, by restricting its range or limit. The Integration of a function f(x) is given as

∫f(x)dx = F(x) + C

Where, 

• f(x) is Integrand,
• dx is Integrating Agent,
• F(x) is anti-derivative of f(x), and
• C is Constant.

How to Integrate Function

To explain we considered above result i.e. (1) – derivative of function f(x)

f'(x) = 2x 

Integrating both sides,

∫f'(x) = ∫2x 

Here,

We have to increase the power of derivative by 1 and also divide function with updated power of function, 

After that add an integral constant with it. 

Integration is called anti-derivative

[Tex]f(x) = 2 \frac{x^2}{2} + C = x^2 + C                                  [/Tex]

Integration Formulas

To integrate various types of functions we have different formulas for different types of functions. We will learn Integration Formulas for the following functions:

• Algebraic Function
• Exponential Function
• Trigonometric Function

Integration  of Algebraic Functions

Formulas for some Integration  of Algebraic Functions are

∫f'(x)	f(x)
∫xn dx	[Tex]\frac{x^n+1 }{n+1} + C[/Tex]
[Tex]∫\frac{1}{x}dx[/Tex]	logex + C

Integration  of Exponential Functions

Some commonly used formulas of Integration related to the exponential function are

∫f'(x)	f(x)
∫exdx	ex + C
∫ax logea dx	[Tex] log_ea \frac{a^x}{log_ea} + C  = a^x + C [/Tex]

Integration of Trigonometric Functions

Formula for integration of some common Trigonometric Functions are:

∫f'(x)	f(x)
∫ cos x dx	sin x + C
∫sin x dx	-cos x + C
∫ cot x dx	log|sin x | + C
∫ sec x dx	log|sec x + tan x | +C
∫ tan x dx	-log|cos x| + C
∫cosec x dx	log|cosec x – cot x | + C
∫sec2 x dx	tan x + C
∫sec x tan x dx	sec x + C

The above formulas are used when the functions are present alone or when multiplied by a scalar number but when two functions are in product form or quotient form then we can’t simply Integrate each function separately but we need to follow some rules, particularly for product and quotient case. Hence, we will look at Integration by Parts.

Integration By Parts

In integration by parts, we will learn the formulas for Integration when two functions are in product or quotient form:

Integration of Product of Functions: Let us assume ‘u’ and ‘v’ are two functions in the product (u.v) then the Integration of u.v is given as

 ∫u.v dx= u∫v dx – ∫ [(du/dx) ∫vdx] dx

Integration of Quotient of Functions: Let us assume ‘u’ and ‘v’ are two functions in the product (u/v) then the Integration of u.v is given as

∫u/v = u∫(1/v) dx – ∫ [(du/dx) ∫(1/v)dx)] dx

Area Under the Curve

Area Under the Curve refers to the region enclosed by the graph of a function and coordinate axes or the intersection region of two graphs. Here, we will not have a regular shape hence we can’t use regular formulas. To calculate the area in such a case we will use the concept of Integration. We will take an elemental area dx under the curve and Integrate it over the defined range x = a to x = b.

Differentiation & Integration Formulas

The formulas for Differentiation and Integration of some frequently used functions are tabulated below:

Functions	Differentiation Formula	Integration Formula
xn	d/dx(xn) = nx(n-1)	∫xn dx = xn+1/(n+1) + C
1/x	d/dx(1/x) = -1/x2	∫(1/x)dx = loge|x| + C
ex	d/dx(ex) = ex	∫ex dx = ex + C
sin x	d/dx(sin x) = cos x	∫sin x dx = -cos x + C
cos x	d/dx(cos x) = -sin x	∫cos x dx = sin x + C
tan x	d/dx(tan x) = sec2 x	∫tan x dx = -log|cos x| + C
cot x	d/dx(cot x) = -cosec2 x	∫cot x dx = log|sin x| + C
sec x	d/dx(sec x) = sec x.tan x	∫sec x dx = log |sec x + tan x| + C
cosec x	d/dx(cosec x) = -cosec x.cot x	∫cosec x dx = log |cosec x – cot x| + C

Properties of Differentiation and Integration

The Properties of Differentiation and Integration are listed below:

• Property of Scalar Multiplication: Both Differentiation and Integration follow the rule of scalar multiplication i.e.,

 d/dx{k.f(x)} = k.d/dx{f(x)} and ∫k.f(x)dx = k.∫f(x)dx 

Where k is a scalar quantity.

• Property of Addition and Subtraction: If functions are in Addition and Subtraction form we need to solve Differentiation and Integration as 

d/dx{f(x) ± g(x)} = d/dx{f(x)} ± d/dx{g(x)}

and

∫{f(x) ± g(x)} dx = ∫{f(x)}  dx± ∫{g(x)} dx

• Differentiation and Integration are the inverse processes of each other i.e., 

d/dx{f(x)} = f'(x) and ∫f'(x) dx = f(x)

• Differentiation follows the Chain Rule i.e. if we have the function of function then the derivative of it is given as the derivative of the first function multiplied by the derivative of the second function i.e.,

d/dx{f(g(x))} = f'(x).g'(x)

• Both Differentiation and Integration can be solved for a given limit.

Difference between Differentiation and Integration

The difference between Differentiation and Integration are as follows:

Differentiation	Integration
Differentiation involves the Division of component functions	Integration involves the Addition of components of a function
Reduces the power of the function	Increases the power of the function
Finds the gradient or slope of the curve	Finds the area under the curve
Calculated for a specific point in the function’s domain	Calculated for a range of points within the function’s domain
Derivative of a function is the anti-Integral of the function	Integral of a function is the anti-Derivative of the function

Solved Examples of Differentiation and Integration

Example 1: Differentiate [Tex]\bold{y= \frac{1}{3x+1}}   [/Tex] with respect to x.

Solution:  

Let [Tex]y = \frac{1}{3x+1}                       [/Tex]

⇒  [Tex]\frac{dy}{dx} = \frac{d}{dx}(\frac{1}{3x+1})    [/Tex]

⇒ [Tex]\frac{dy}{dx} = -\frac{3}{(3x+1)^2}[/Tex]

Example 2: Differentiate the following: i) x³ ii)[Tex] \bold{\frac{1}{x^3+1}}   [/Tex][Tex] \frac{1}{x^3+1}[/Tex]

Solution: 

i) Let y = x³

⇒\frac{dy}{dx} = \frac{d}{dx}(x^3)                                

⇒[Tex]\frac{dy}{dx} = 3x^2                               [/Tex]

 ii) Let[Tex] y = \frac{1}{x^3+1}                       [/Tex]

Using, Quotient Rule,                              

[Tex]\frac{dy}{dx} = \frac{-3x^2}{(x^3+1)^2}[/Tex]

Example 3: Find of derivative of [Tex]\bold{y = \frac{e^x-e^{-x}}{e^{-x}+e^x}}   [/Tex] With respect to x.

Solution:

Let [Tex]y = \frac{e^x-e^{-x}}{e^{-x}+e^x}                                  [/Tex]

⇒ [Tex]\frac{dy}{dx} =\frac{(e^{-x}+e^x)(e^x+e^{-x}) – (e^{-x}+e^x)(e^x+ e^{-x})}{(e^{-x}+e^x)^2}                                  [/Tex]

⇒ [Tex]\frac{dy}{dx} = \frac{e^{-2x}+e^{2x} -e^{-2x}-e^{2x}}{(e^{-x}+e^x)^2}                                  [/Tex]

⇒ [Tex]\frac{dy}{dx} = 0[/Tex]

Example 4: Differentiate [Tex]\bold{y = \frac{e^x-e^{-x}}{e^{-x}+e^x}}   [/Tex] with respect  to x. 

Solution: 

[Tex]y = \frac{e^x-e^{-x}}{e^{x}+e^{-x}}= \frac{e^{2x}-1}{e^{2x}+1}            [/Tex]

⇒[Tex] \frac{dy}{dx}=\frac{\frac{d}{dx}(e^{2x}-1)(e^{2x}+1)-\frac{d}{dx}(e^{2x}+1)(e^{2x}-1)}{(e^{2x}+1)^2}[/Tex]

⇒ [Tex]dy/dx = \frac{2e^{2x}(e^{2x}+1)-2e^{2x}(e^{2x}+1)}{(e^{2x}+1)^2}[/Tex]

⇒ [Tex]dy/dx = \frac{4e^{2x}}{(e^{2x}+1)^2}   [/Tex]

Example 5: Differentiate y = Sec²x with respect to x. 

Solution: 

Let y = sec²x           

⇒ [Tex]\frac{dy}{dx} = 2secx(secx tanx)                                  [/Tex]

⇒ [Tex]\frac{dy}{dx}   [/Tex] = 2 sec² x tan x

Example 6: Differentiate sec²x + cos2x.

Solution:

y = sec²x + cos2x                    

⇒ [Tex]\frac{dy}{dx} = 2sinx cosx + (-2sin2x)                                  [/Tex]

⇒ [Tex]\frac{dy}{dx} = 2sinx cosx -2sin2x[/Tex]

Example 7: Integrate √x with respect to x.

Solution:  

y = ∫√x dx

⇒ y = [Tex]∫x^{\frac{1}{2}} dx          [/Tex]

⇒ [Tex]y = \frac{ x^{\frac{1}{2}+1}}{\frac{1}{2}+1} + c                       [/Tex]

⇒ [Tex]y = \frac{2}{3}x^{\frac{3}{2}} + c[/Tex]

Example 8:  Integrate the following: 

(i) e^2x     (ii) e^ax

Solution:  

i) y=∫e^2x 

⇒ [Tex]y = \frac{e^{2x}}{2} + c                                  [/Tex]

ii) y=∫e^ax

⇒ [Tex]y = \frac{e^{ax}}{a} + c                                  [/Tex]

Example 9: Integrate  sin²x+ cos²x.

Solution:

y = ∫(sin²x + cos²x)dx          

⇒ y = ∫dx                      

⇒ y = x + c  

Example 10: Integrate sin 2x + cos 2x.

Solution: 

y = ∫(sin2x + cos2x)dx 

⇒ y = ∫sin2xdx + ∫cos2x dx

⇒ y = [Tex]\frac{-cos2x}{2}+ \frac{sin2x}{2}+ c    [/Tex]

⇒ [Tex]y = \frac{1}{2}(sin2x -cos2x) + c                                  [/Tex]

Example 11: Find the area bounded by the curve y = Sinx between x= 0 and x = 2π.

Solution: 

Let y = Sinx 

The graph of  y = sinx is like,

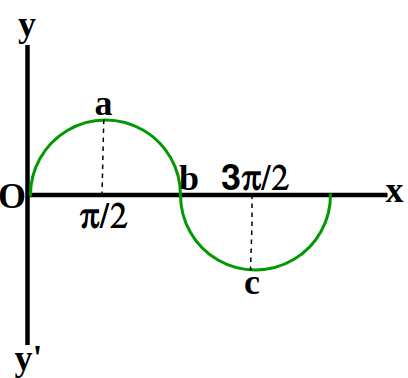
Required area = Area of OABO + Area of BCDB 

⇒ Required area [Tex]=  \int_{0}^{π}|sinx|dx + \int_{π}^{2π}|sinx|dx[/Tex]

⇒ Required area [Tex]= \int_{0}^{π}sinx  dx + \int_{π}^{2π}-sinxdx[/Tex]

⇒ Required area [Tex]= \left[ -cosx \right]_{0}^{π} + \left[ cosx \right]_{π}^{2π}[/Tex]

⇒ Required area = -cosπ + cos0 + cos2π- cosπ

⇒ Required area = 4 sq units. 

Example 12: The area bounded by the region of curve y² = x and the lines x = 1, x= 4, and the x-axis is :

Solution:

Let y² = x a curve region bounded by the lines x = 1 and x = 4 about x-axis. 

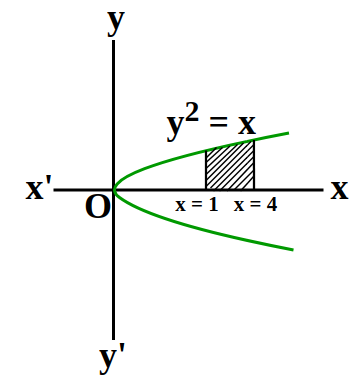
Required Area (Shaded Area)  =[Tex] \int_{1}^{4}|y|dx [/Tex]

⇒ Required area [Tex]= \int_{1}^{4}\sqrt{x}dx[/Tex]

⇒ Required area [Tex]= \left[\frac{ x^{\frac{3}{2}}}{\frac{3}{2}} \right]                         [/Tex]

⇒ Required area [Tex]= \frac{2}{3}\left[ {4}^{\frac{3}{2}} – 1 \right][/Tex]

⇒ Required area [Tex]= \frac{14}{3} sq. units.                                 [/Tex]

Example 13: The area of the region area, integrate x w.r.t. y and take y = 2 as the lower limit and y = 4 as the upper limit. The given curve x^2 = 4y is a parabola, which is symmetrical about the y-axis. 

Solution:  

The given curve is parabola x² = 4y which is symmetric to the y-axis. 

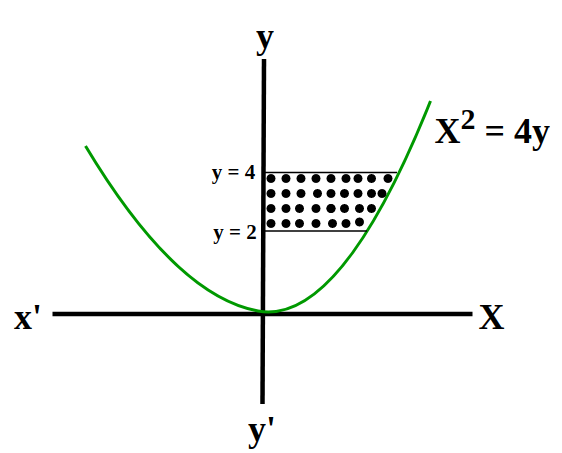
The area bounded by the curve is shaded portion of the graph.

Required  Area = [Tex]\int_{2}^{4}|x|dy[/Tex]

⇒ Required area [Tex]= \int_{2}^{4}2\sqrt{y}dy [/Tex]

⇒ Required area [Tex]= 2\left[\frac{y^{\frac{3}{2}}}{\frac{3}{2}}  \right]_{2}^{4}[/Tex]

⇒ Required area [Tex]= \frac{8}{3}\left[ 4 -\sqrt{2} \right] sq. units.[/Tex]

FAQs on Differentiation and Integration

Q1. What is Differentiation?

Answer:

Differentiation is a process of finding instantaneous change in function with respect to other quantity. It is given by d/dx{f(x)} = f'(x)

Q2. What is Integration?

Answer:

Integration refers to the process of adding small and continuous data of a function over a given range of limits. It is given by ∫f(x)dx = F(x) + C

Q3. What is the basic difference between Differentiation and Integration?

Answer:

The basic difference between Differentiation and Integration is that Differentiation divides the function and reduces it on a particular point in the domain while Integration sums up all the continuous data of the function over a given range of limits in the domain.

Q4. What is the Differentiation of log x?

Answer:

The differentiation of log x is given by d/dx(log x) which is equal to 1/x

Q5. What is Differentiation and Integration of e^x?

Answer:

The differentiation of e^x is e^x and the integration of e^x is e^x + C.