Ratio and Proportion

Ratio and Proportion are used for comparison in mathematics. Ratio is a comparison of two quantities while Proportion is a comparison of two ratios. When a fraction a/b is written a:b then it is termed as a ratio b. When two ratios let’s say a:b and c:d are equal a:b and c:d are said to be proportional to each other. Two proportional ratios are written as a:b::c:d. 

In this article, we will learn about Ratios and Proportions in detail with deep insights into various types of proportions, formulas to solve, examples, and FAQs based on it.

Ratio Definition

Ratio is a comparison of two quantities of the same unit. For example, you can compare your height with your friend’s height and your weight with your friend’s weight but you can’t compare your height with your friend’s weight because they don’t have the same units. So let’s say your height is 100 cm and that of your friend is 120 cm then you compare in the following manner

Let

• H₁ = 100 cm which is your height
• H₂ = 120 cm which is your friend’s height

Then a comparison between the two is done by 

H₁/H₂ = 100 cm/120 cm = 5/6

Now the above comparison we eliminated cm as both are the same units and also reduced 100/120 to its simplest form to 5/6. Hence, the comparison can be written in the form of a ratio as H₁:H₂ = 5:6.

Thus we got to know that ratio is a comparison between two quantities of the same type or unit and reduced to the simplest form. While calculating the ratio in the above case of 100/120 we eliminated 20 as a common factor from both the terms. 

Now think of a reverse case if someone says the ratio of heights of two objects is 5:6 then you can’t say exactly if it is 5 cm and 6 cm or 10 cm and 12 cm or 100 and 120 cm or any multiple of 5 and any multiple of 6. Thus we can say that ratio doesn’t give the exact value of the component, it just gives an idea of equivalent fractions multiplied with common factor k to both Numerator and Denominator of the fraction. Hence, in that case, the height of one object is 5k and that of the second object is 6k. This is what you assume the ratio to be k or x in questions where the two quantities are given in ratio.

Representation of Ratio

The ratio of two quantities is given by using the colon symbol (:). The ratio of two quantities a and b is given as 

a:b 

where,

• a is called Antecedent
• b is called Consequent

The ratio a:b means ak/bk where k is the common factor. k is multiplied to give equivalent fractions whose simplest form will be a/b. We can read a:b as ‘a ratio b’ or ‘a to b’.

Since we have learned the basic concept of ratio and its meaning now we will learn the properties of ratio.

Properties of Ratio

There are the following properties of the Ratio

• If a ratio is multiplied by the same term both in antecedent and consequent then there is no change in the actual ratio. For example, ak:bk = a:b
• If the antecedent and consequent of a ratio are divided by the same number then also there is no change in the actual ratio. For Example, a/k:b/k = a:b
• If two ratios are equal then their reciprocals are also equal i.e. if a:b = c:d then b:a = d:c
• If two ratios are equal then their cross-multiplications are also equal. For Example a:b = c:d ⇒ ad = bc
• The ratios for a pair of comparisons can be the same but the actual value may be different. For Example, 45:60 = 5:6 and 100:120 = 5:6 hence ratio 5:6 is same but actual value is different

Ratio Formulas

The ratio of any two quantities a:b is given by a/b where a/b must be in simplest form. In case, you are not able to find the common factor between a and b then find the HCF or a and b and divide a and b with HCF to reduce it to the simplest form. There are more formulas which we will see below:

Compound Ratios

When two ratios are multiplied then the new ratio is called the compound ratio. For example a:b and c:d are two ratios then ac:bd is a compound ratio.

Duplicate Ratios

For a:b, a²:b² is called duplicate ratios

For a:b, √a:√b is called sub-duplicate ratios

For a:b, a³:b³ is called triplicate ratios

Learn more about, Ratio Formulas

Proportion Definition

Proportion refers to the comparison of ratios. If two ratios are equal then they are said to be proportionate to each other. Let’s say a:b = c:d then a:b is said to be proportionate to c:d. Here it should be noted that a:b and c:d need not be the ratio of the same quantities i.e. if a:b is the ratio of heights then c:d can be the ratio of height or even weight. The magnitude of the ratio should be the same to call it proportionate. The proportion has applications in the Similarity of Triangles and in the Height and Distance chapter of trigonometry.

Representation of Proportion

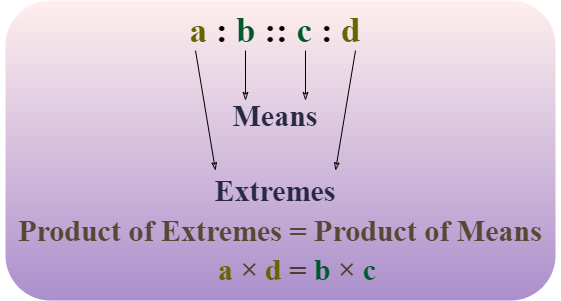
Two proportional ratios are represented by a double colon(::). If two ratios a:b and c:d are equal then they are represented as 

a:b::c:d

where 

• a and d are called extreme terms
• b and c are called mean terms

The image added below shows the property of the proportion.

Properties of Proportions

There are the following properties of Proportions

• For two ratios in proportion i.e. a/b = c/d, a/c = b/d holds true.
• For two ratios in proportion i.e. a/b = c/d, b/a = d/c holds true.
• For two ratios in proportion i.e. a:b::c:d, the product of mean terms is equal to the product of extreme terms i.e. ad = bc
• For two ratios in proportion i.e. a/b = c/d, (a + b)/b = (c + d)/d is true.
• For two ratios in proportion i.e. a/b = c/d, (a – b)/b = (c – d)/d is true.

Types of Proportions

There are three types of Proportions:

• Direct Proportion
• Inverse Proportion
• Continued Proportion

Let’s learn about them in detail.

Direct Proportion

When two quantities increase and decrease in the same ratio then the two quantities are said to be in Direct Proportion. It means if one quantity increases then the other will also increase and if one will decrease then the other will also decrease. It is represented as a ∝ b. For Example, Momentum is a product of mass and velocity hence, momentum is directly proportional to mass and velocity (p∝m or p∝v) which means if mass is constant and velocity is high then momentum will also be high and if velocity is constant then momentum will increase with an increase in mass.

Inverse Proportion

When two quantities are inversely related to each other i.e. increase in one leads to a decrease in the other or a decrease in the other leads to an increase in the first quantity then the two quantities are said to be Inversely Proportional to each other. For Example, Pressure is given by force divided by area (P = F/A). Here Pressure is inversely related to area which means a decrease in area will lead to an increase in pressure and an increase in area will lead to a decrease in pressure. Now you can understand why the strap of your school bag is broad which means there is a larger area and hence less pressure on your shoulder.

Continued Proportion

If the ratio a:b = b:c = c:d, then we see that the consequent of the first ratio is equal to the antecedent of the second ratio, and so on then the a:b:c:d is said to be in continued proportion. If the consequent and antecedent are not the same for two ratios then they can be converted into continued proportion by multiplying. For Example, in the case of a:b and c:d consequent and antecedent are not same then the continued proportion is given as ac:cb:bd.

In the continued proportion a:b:c:d., c is called the third proportion, and d is called the fourth proportion.

Proportion Formulas

There are the following formulas to solve problems of proportion.

• If a:b = c:d, then we can say that (a + c):(b + d), it is also called Addendo.
• If a:b = c:d, then we can say that (a – c):(b – d), it is also called Subtrahendo.
• If a:b = c:d, then we can say that (a – b):b = (c – d):d, it is also known as Dividendo.
• If a:b = c:d, then we can say that (a + b):b = (c + d):d, it is also known as Componendo.
• If a:b = c:d, then we can say that a:c = b:d, it is also known as Alternendo.
• If a:b = c:d, then we can say that b:a = d:c, it is also called Invertendo.
• If a:b = c:d, then we can say that (a + b):(a – b) = (c + d):(c – d), it is also known as Componendo and Dividendo.
• If a is proportional to b, then it means a = kb where k is a constant.
• If a is inversely proportional to b, then a = k/b, where k is a constant.
• Dividing or multiplying a ratio by a certain number gives an equivalent ratio.

Mean Proportion

Consider two ratios a:b = b:c then as per the rule of proportion product of the mean term is equal to the product of extremes, this means b² = ac, hence b = √ac is called mean proportion.

Difference between Ratio and Proportion

The difference between Ratio and Proportion is tabulated below

Ratio	Proportion
Ratio is used to compare two quantities of the same unit	Proportion is used to compare two ratios
Ratio is represented using (:), a/b = a:b	Proportion is represented using (::), a:b = c:d ⇒ a:b::c:d
Ratio is an expression	Proportion is an equation it equates two ratios

Read More,

• Percentage
• Ratios and Percentages
• Fractions

Ratio and Proportion Examples

Example 1. Is the ratio 5:10 proportional to 1:2?

Solution:

5:10 divided by 5 gives 1:2. Thus, they are same to each other. So we can say that 5:10 is proportional to 1:2.

Example 2. Given a constant k, such that k:5 is proportional to 10:25. Find the value of k.

Solution:

Since k:5 is proportional to 10:25, we can write,

k / 5 = 10 / 25

k = 10/25 × 5 = 2

So, the value of k is 2.

Example 3. Divide 100 into two parts such that they are proportional to 3:5.

Solution:

Let’s the value of two parts are 3k and 5k, where k is a constant.

Since the total sum of two parts is 100, we can write,

3k + 5k = 100

8k = 100

k = 12.5

So, the parts are 3k = 3 × 12.5 = 37.5 and 5k = 5 × 12.5 = 62.5

Example 4. If x² + 6y² = 5xy, then find the value of x/y.

Solution:

Given, x² + 6y² = 5xy. 

Dividing the equation by y², we get

(x/y)² + 6 = 5 (x/y)

Let’s x/y = t

So, we can write,

t² + 6 = 5t

t² – 5t + 6 = 0

(t – 2)(t – 3) = 0

t = 2 or t = 3

Since, t = x/y, we get

x/y = 2 or x/y = 3

Example 5. If a:b = c:d, then find the value of (a² + b²)/(c² + d²).

Solution:

Given, a/b = c/d. 

Squaring both sides, we get

a²/b² = c²/d²

a² = b²c²/d²

Putting the value of a² inside (a² + b²)/(c² + d²), we get

((b²c²/d²) + b²)/(c² + d²) =  b²(c² + d²)/(d² (c² + d²)) = b²/d²

Hence, our answer is b²/d².

Example 6. Find the mean proportion of 5 and 125

Solution:

Let the mean proportion be x 

⇒ 5:x = x:125

⇒ x² = 125 × 5

⇒ x = √625 = 25

FAQs on Ratio and Proportion

Q1: What is the Definition of Ratio?

Answer:

The comparison of two quantities of the same units obtained by division of the two quantities is called Ratio.

Q2: What is the Definition of Proportion?

Answer:

When two ratios are equivalent then the ratios are called to be in Proportion.

Q3: What is Direct Proportion?

Answer: 

When two quantities increase or decrease in the same ratio then the two quantities are said to be in direct proportion.

Q4: What is Continued Proportion?

Answer:

When the consequent of the first ratio is the antecedent of the second ratio then two ratios are said to be in Proportion. For Example, a:b and b:c are in continued proportion and can be written as a:b:c.

Q5: What is the formula of Ratio?

Answer:

The Ratio of two quantities a and b is given by a:b = a/b

Q6: What is the formula of Proportion?

Answer:

The formula for Proportion for two ratios a:b and c:d is a/b = c/d