L’ Hospital Rule in Calculus

L’Hospital Rule is one of the most frequently used tools in entire calculus, which helps us calculate the limit of those functions that seem indeterminate forms. For many years, these indeterminate forms have been considered impossible to solve for function but some scholars found out that some functions have limits which can be seen in the graph but the calculation seems to result in an indeterminate form. Hence, the L’hospital rule is born.

In this article, we will learn about the concept of the L’Hospital Rule in detail. Other than that, this article also covers indeterminate forms, the L’Hospital Rule formula, and proof of the L’Hospital Rule formula with examples as well.

What is L’Hospital Rule?

L’Hospital rule states that when the limit is applied to a fraction of two functions resulting in an indeterminate form then it is equal to the limit of the fraction formed by the individual derivatives of functions. The L’Hospital rule uses derivatives of each function to solve the limit which help us evaluate the limits which results in an indeterminate form.

Indeterminate Forms

The indeterminate forms are the forms with two functions whose limits cannot be determined by putting the limits in the function. The indeterminate form is the form that is undefined mathematically. The form whose value cannot be evaluated by directly applying the limits are called as indeterminate forms. Indeterminate form includes 0/0, ±∞/±∞, 0×∞, ∞-∞, 0⁰, 1^∞ etc. The 0/0, ±∞/±∞ are the most common indeterminate form in which the L’Hospital rule is applied.

L’Hospital Rule Formula

For two continuous and differentiable functions f(x) and g(x) if limits x tends to result in an indeterminate form, then the L’Hospital rule is applied and it states,

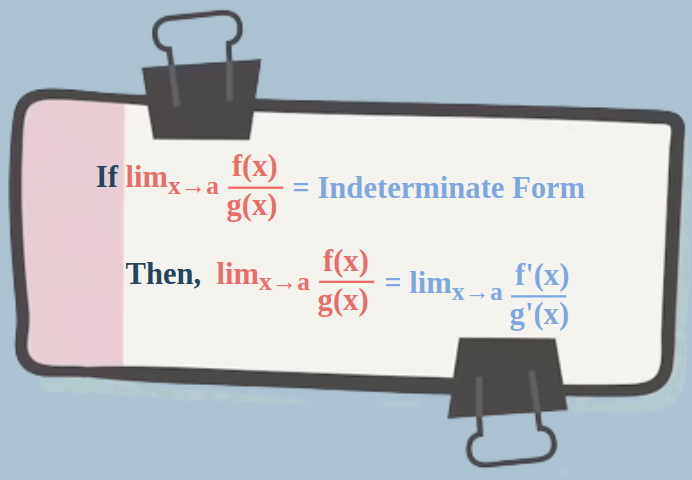
Where,

• a is any real number or infinity.
• f'(x) is derivative of f(x)
• g'(x) is derivative of g(x) and g(x) and g(a) ≠ 0

When to use L’Hospital Rule

The L’Hospital rule is used when the limits of two differentiable functions after applying the limit gives an indeterminate form. Commonly, for the indeterminate forms 0/0, ±∞/±∞ we apply the L’Hospital rule directly to evaluate the limit.

Conditions for L’Hospital Rule

Some necessary conditions for applying the L’Hospital rule

• f(x) and g(x) must be differentiable.
• The limit of the quotient of the derivatives of a given function should exist i.e.,

lim_x→a f'(x) / g'(x) = Some Finite Number

L’Hospital Rule Proof

The L’Hospital rule is applied when limits result in indeterminate form 0/0, ±∞/±∞. We can prove the L’Hospital rule by using Cauchy’s Mean Value Theorem.

Let f(x) and g(x) be two continuous functions on the interval [a, b] and differentiable on the interval (a, b) and we know for any function f(x), its derivative at x = c is given as

f'(c) = [f(x) – f(c)]/(x – c)

Assume that g(x) = 0 on (a, b), then there exists c in (a, b) such that

lim _x→b [f'(x) / g'(x)] = lim _x→b [{(f(x)- f(b)) / (x – b)} / {(g(x)- g(b)) / (x – b)}]

Let the functions f and g be differentiable at x = c satisfying where c belongs to the interval in which functions are defined. Let f(c) = g(c) = 0.

By Cauchy’s Mean Value Theorem,

lim_x→c [f(x) / g(x)] = lim _x→c[f(x) -f(c)]/[g(x) – g(c)]

⇒ lim _x→c [f(x) / g(x)] = lim _x→c[f(x) – 0] / [g(x) – 0]    {as f(c) = g(c) = 0}

⇒ lim _x→c [f(x) / g(x)] = lim _x→c[f(x) – f(c)] / [g(x) – g(c)]

⇒ lim_x→c [f(x) / g(x)] = lim _x→c [{(f(x)- f(c)) / (x – c)} / {(g(x)- g(c)) / (x – c)}]

⇒ lim_x→c [f(x) / g(x)] = lim_x→c [f'(x) / g'(x)]

Which is the required result.

Learn more about Mean Value Theorem.

How to Apply L’Hospital Rule?

L’Hospital rule is applied when we get an indeterminate form after applying the limits. For applying the L’Hospital Rule these are the following steps:

• First check after applying the limit we get indeterminate form or not. If we do not get indeterminate form then, we cannot apply L’Hospital rule.
• When we get indeterminate form, simplify the fraction before applying the rule.
• Find the derivatives of the functions separately.
• Determine the value of ratio of derivatives to get the result.

Example: Find lim_x→1 [(x¹⁰ – 1) / (x² – 1)]

Solution:

First apply the limit

lim_x→1 [(x¹⁰ – 1) / (x² – 1)] = [1¹⁰ -1] / [1² -1] = 0 / 0

After applying the limit, we get 0 / 0 form so, we apply L’Hospital rule

f(x) = x¹⁰ – 1

⇒ f'(x) = 10x⁹

g(x) = x² – 1

⇒ g'(x) = 2x

lim_x→1 [(x¹⁰ – 1) / (x² – 1)] = (10x⁹) / 2x

Now, put limit x = 1, we get

⇒ lim_x→1 [(x¹⁰ – 1) / (x² – 1)] = [(10×1⁹ )-1] / [2×1] = 10 / 2

⇒ lim_x→1 [(x¹⁰ – 1) / (x² – 1)] = 5

Applying L’Hospital Rule Multiple Times

When the L’Hospital rule is applied once and also after applying the limit we get an indeterminate form then after simplifying the fraction, we can again apply L’Hospital Rule. We can apply the rule multiple times until we get an indeterminate form. Before each application of the L’Hospital rule, we should simplify the fractions.

Example: Find lim_x→0 [(sinx – x) / x²]

Solution:

First apply the limit

lim_x→0 [(sinx – x) / x²] = (sin0 – 0) / 0² = 0/0

After applying the limit, we get 0 / 0 form so, we apply L’Hospital rule

f(x) = sin x – x

⇒ f'(x) = cos x -1

g(x) = x²

⇒ g'(x) = 2×0

lim_x→0 [(sinx – x) / x²] = (cos x -1) / 2x

Now, put limit x = 0, we get

lim_x→0 [(sinx – x) / x²] = (cos 0 -1) / 2×0 = 0 / 0

Again we get 0 / 0 form so, we again apply L’Hospital rule

f(x) = cos x – 1

⇒ f'(x) = -sinx

g(x) = 2x

⇒ g'(x) = 2

lim_x→0 [(sinx – x) / x²] = (-sin x) / 2

Now, put limit x = 0, we get

lim_x→0 [(sinx – x) / x²] = (- sin 0) / 2 = 0

⇒ lim_x→0 [(sinx – x) / x²] = 0

How to apply L’Hospital Rule for Exponents

When the variable is in power, we equate the limit with a variable and take the logarithm on both sides and then apply the L’Hospital rule.

Example: Evaluate: lim_x→∞ x^1/x

Solution:

The given limit gives ∞⁰

In this question we have variable in the exponent, so we take ln

y = x^1/x

Taking ln both sides in the above equation.

ln y = ln x^1/x

⇒ ln y = (1 / x) ln x

Applying limit

lim_x→∞ ln y = lim_x→∞ (1 / x) × ln x

⇒ lim_x→∞ ln y = lim_x→∞ (ln x) / x

Applying L’Hospital rule

lim_x→∞ ln y = lim_x→∞ (1 / x) / 1

⇒ lim_x→∞ ln y = lim_x→∞ (1 / x)

putting limit

lim_x→∞ ln y = 1 / ∞ = 0

⇒ ln [lim_x→∞ y] = 0

⇒ lim_x→∞ y = e⁰

⇒ lim_x→∞ y = 1

⇒ lim_x→∞ x^1/x = 1

Key Points to Remember

Some of the key points which we need to remember related to L’Hospital’s rule are:

• If the limit does not result in an indeterminate form after applying the limit, then we cannot apply the L’Hospital rule (it provides an incorrect result).
• Each time before applying the rule, we should simplify the rational expression.
• If the limit is given in product form like f(x). g(x) then, for applying the rule we can rewrite it as f(x) / 1 / g(x) or g(x) / 1 / f(x).
• While applying the L’Hospital rule multiple times always check if the resulting rational expression gives an indeterminate form or not.

Read More,

• Calculus
• Introduction to Limits
• Sandwich Theorem

Problems on L’Hospital Rule

Problem 1: Find lim_x→-2 [(x + 2) / (x² + 3x + 2)]

Solution:

First apply the limit

lim_x→-2 [(x + 2) / (x² + 3x + 2)] = lim_x→-2 [(- 2 + 2) / ((-2)² + 3×(-2) + 2)] = 0 / 0

After applying the limit, we get 0 / 0 form so, we apply L’Hospital rule

f(x) = x + 2

⇒ f'(x) = 1

g(x) = x² + 3x + 2

⇒ g'(x) = 2x + 3

lim_x→-2 [(x + 2) / (x² + 3x + 2)] = 1 / (2x + 3)

Now, put limit x = -2, we get

lim_x→-2 [(x + 2) / (x² + 3x + 2)] = 1 / [2×(-2) + 3] = 1 / (-4 + 3)

⇒ lim_x→-2 [(x + 2) / (x² + 3x + 2)] = -1

Problem 2: Find lim_x→0 [sin 3x / x]

Solution:

First apply the limit

lim_x→0 [sin 3x / x] = sin 3(0) / 0 = 0 / 0

After applying the limit, we get 0 / 0 form so, we apply L’Hospital rule

f(x) = sin3x

⇒ f'(x) = 3cos3x

g(x) = x

⇒ g'(x) = 1

lim_x→0 [sin 3x / x] = 3cos 3x / 1

Now, put limit x = 0, we get

lim_x→0 [sin 3x / x] = [3cos 3(0)] / 1 = 3 / 1

⇒ lim_x→0 [sin 3x / x] = 3

Problem 3: Find lim_x→∞ [x² / e^x]

Solution:

First apply the limit

lim_x→∞ [x² / e^x] = ∞² / e ^∞ = ∞ / ∞

After applying the limit, we get ∞ / ∞ form so, we apply L’Hospital rule

f(x) = x²

⇒ f'(x) = 2x

g(x) = e^x

⇒ g'(x) = e^x

⇒ lim_x→∞ [x² / e^x] = 2x / e^x

Now, put limit x = ∞, we get

lim_x→∞ [x² / e^x] = (2 × ∞)/ e^∞ = ∞ / ∞

Again we get ∞ / ∞ form so, we again apply L’Hospital rule

f(x) = 2x

⇒ f'(x) = x

g(x) = e^x

⇒ g'(x) = e^x

lim_x→∞ [x² / e^x] = 2 / e^x

Now, put limit x = ∞, we get

lim_x→∞ [x² / e^x] = 2 / e^x = 2 / e^∞ = 2 / ∞

⇒ lim_x→∞ [x² / e^x] = 0

Problem 4: Evaluate: lim_x→∞ x^x

Solution:

The given limit gives ∞^∞

In this question we have variable in the exponent, so we take ln

y = x^x

Taking ln

ln y = ln x^x

⇒ ln y = x ln x

Applying Limit

lim_x→∞ ln y = lim_x→∞ xlnx

⇒ lim_x→∞ ln y = lim_x→∞ [lnx / ( 1 / x)]

Applying L’Hospital rule

lim_x→∞ ln y = lim_x→∞ [(1 / x) / ( -1 / x²)]

⇒ lim_x→∞ ln y = lim_x→∞ [-(1 / x) / (1 / x)²]

⇒ lim_x→∞ ln y = lim_x→∞ [-1 / (1 / x)]

⇒ lim_x→∞ ln y = lim_x→∞ (-x)

putting limit

lim_x→∞ ln y = -∞

⇒ ln lim_x→∞ y = -∞

⇒ lim_x→∞ y = e^-∞

⇒ lim_x→∞ y = 0

⇒ lim_x→∞ x^x = 0

Practise Problems of L’Hospital Rule

Find the following limits.

• 
• 
• 
• 
• 
• 
• 
• 

FAQs on L’Hospital Rule

1. What is L’Hospital Rule?

L’Hospital rule states that when the limit is applied to ratio of two differentiable functions whose value after putting the limit results in indeterminate form is equal to the limit of the ratio of each function’s derivatives.

2. What is the Formula for L’Hospital Rule?

If  lim_x→a [f(x) / g(x)] = Indeterminate form, L’Hospital Rule Formula is given by:

lim_x→a [f(x) / g(x)] = lim_x→a [f'(x) / g'(x)]

Where,

• a is any real number or infinity,
• f'(x) is derivative of f(x), and
• g'(x) is derivative of g(x) and g(x) and g(a) ≠ 0.

3. When we apply L’Hospital Rule?

When for the given limits after applying the limit, we get indeterminate form (mostly 0/0, ±∞/±∞) and both functions are differentiable then, we apply L’Hospital rule.

4. Can L’Hospital Rule be applied multiple times?

Yes, L’Hospital rule can be applied multiple times if the resultant fraction gives indeterminate form again and again.

5. What are Indeterminate Forms?

Indeterminate forms are the forms with the ratio of two functions whose value in indeterminate after applying the limits. Some of the indeterminate forms are: 0/0, ±∞/±∞, 0×∞, ∞-∞, 0⁰, 1^∞ .

6. What are the Limitations of L’Hospital’s Rule?

One of the major limitations of L’Hospital’s Rule is that it can only be applied for indeterminate forms of either 0/0 or (+/-infinity)/(+/-infinity). All the other forms first need to be converted into these forms if possible.