Cauchy’s Mean Value theorem provides a relation between the change of two functions over a fixed interval with their derivative. Cauchy Mean Value Theorem is a special case of Lagrange Mean Value Theorem. Cauchy’s Mean Value theorem is also called the Extended Mean Value Theorem or the Second Mean Value Theorem.
In this article, we will learn about Cauchy’s Mean Value Theorem, its proof, some examples based on Cauchy’s Mean Value Theorem, and others in detail.
What is Mean Value Theorem?
According to the Mean Value Theorem, if a function passes through two points given as [a, f(a)] and [b, f(b)] then there exist a point through which tangent on the curve passes which is parallel to the secant passing through the two given points.
For the function f(x) continuous over [a, b] and differentiable over (a, b), there exists a point c in the interval (a, b) such that f'(c) = [f(b) – f(a)]/(b – a). The image for Mean Value Theorem is added below:
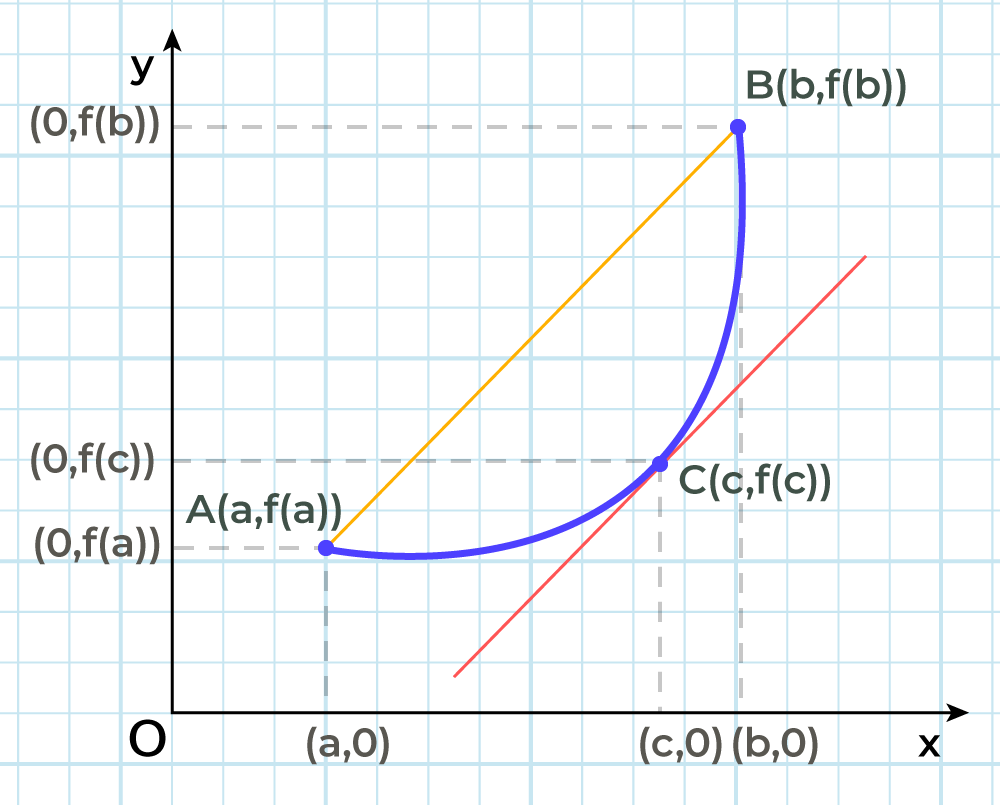
State Cauchy’s Mean Value Theorem
Cauchu’s Mean Value Theorem states that, for any two functions, f(x) and g(x) satisfying the following conditions,
- f(x), g(x) are continuous in the closed interval a ≤ x ≤ b, x ϵ [a, b]
- f(x), g(x) are differentiable in the open interval a < x < b, i.e. x ϵ (a, b)
- g'(x) != 0 for all x belongs to the open interval a < x < b, i.e. x ϵ (a, b)
Then there exists a point c in the open interval a < c < b such that,
[f(b) – f(a)] / [g(b) – g(a)] = f'(c) / g'(c)
Proof of Cauchy’s Mean Value Theorem
Cauchy’s mean value theorem is easily proved using the Rolle’s Mean Value Theorem. Cauchy’s mean value theorem states that, for any two function f(x) and g(x) continuous on [a, b] and defferentiable on (a, b) there exist a point in the interval (a, b) such that,
f'(c) / g'(c) = [f(b) – f(a)] / [g(b) – g(a)]
Now, let’s take the auxiliary function F(x) such that,
F(x) = f(x) + P×g(x)…(i)
In the above equation, P is chosen such that F(x) always satisfies the Rolle’s Theorem in [a, b], Now by definition of Rolle’s theorem,
F(a) = F(b)
⇒ f(a) + P×g(a) = f(b) + P×g(b)
⇒ f(b) – f(a) = P{g(b) – g(a)}
⇒ P = [f(b) – f(a)]/[g(b) – g(a)]
In eq.(i)
F'(x) = f'(x) – {[f(b) – f(a)]/[g(b) – g(a)]}×g'(x)
As, F(x) satisfies Rolles Theorem,
F(c) = 0 where, c ϵ (a, b)
⇒ f'(c) – {[f(b) – f(a)]/[g(b) – g(a)]}×g'(c) = 0
⇒ f'(c) / g'(c) = [f(b) – f(a)] / [g(b) – g(a)]
Thus, Cauchy’s Theorem is proved.
Limitations of Cauchy’s Mean Value Theorem
There are few limitations of the Cauchy’s Mean Value Theorem that are,
- Cauchy’s Mean Value Theorem is applicable only for continuous function.
- Cauchy’s Mean Value Theorem is applicable only for differentiable function.
Applications of Cauchy’s Mean Value Theorem
Various applications of Cauchy’s Mean Value theorem are,
- It is used to solve various problem in Real Analysis.
- It is used to predict the behaviours of various curves.
- It is used to derive Lagrange’s and Rolle’s Mean value theorems, etc.
Read More,
Solved Examples on Cauchy’s Mean Value Theorem
Example 1: Find ‘c’ of Cauchy’s Mean Value Theorem for the functions f(x) = 3x2 + 4x + 5 and g(x) = x2 – x + 25 in the interval [1, 2]
Solution:
Given,
- f(x) = 3x2 + 4x + 5
- g(x) = x2 – x + 25
f(x) and g(x) are polynomial functions and thus,
- f(x), g(x) are continuous in the closed interval a <= x <= b, x ϵ [1, 2]
- f(x), g(x) are differentiable in the open interval a < x < b, i.e. x ϵ (1, 2)
And g'(x) = 2x – 1, is not equal to zero(0) in the interval x ϵ [1, 2]
Thus, Cauchy’s Mean value theorem is applicable on f(x) and g(x)
- f(x) = 3x2 + 4x + 5
- g(x) = x2 – x + 25
Differentiating f(x) and g(x) with respect to x
- f'(x) = 6x + 4
- g'(x) = 2x – 1
Now,
f(a) = f(1) = 12,
f(b) = f(2) = 25,
g(a) = g(1) = 25,
g(b) = g(2) = 27,
f'(c) = 6c + 4, and
g'(c) = 2c – 1
Using Cauchy Mean Value Theorem,
f'(c) / g'(c) = [f(b) – f(a)] / [g(b) – g(a)]
⇒ (6c + 4)/(2c – 1) = (25 – 12)/(27 -25)
⇒ (6c + 4)/(2c – 1) = 13/2
⇒ (6c + 4).2 = 13.(2c – 1)
⇒ 12c + 8 = 26c – 13
⇒ 26c – 12c = 13 + 8
⇒ 14c = 21
⇒ c = 21/14 = 3/2 = 1.5 ϵ [1, 2]
Example 2: Find ‘c’ of Cauchy’s Mean Value Theorem for the functions f(x) = 2.ln x and g(x) = x2 – 1 in the interval [2, 3]
Solution:
Given,
f(x) is a polynomial functions and g(x) is a polynomial function both are continuous and differentiable on the given interval
- f(x), g(x) are continuous in the closed interval a <= x <= b, x ϵ [2, 3]
- f(x), g(x) are differentiable in the open interval a < x < b, i.e. x ϵ (2, 3)
And g'(x) = 2x, is not equal to zero(0) in the interval x ϵ [1, 2]
Thus, Cauchy’s Mean value theorem is applicable on f(x) and g(x)
Differentiating f(x) and g(x) with respect to x
Now,
f(a) = f(2) = 2. ln 2
f(b) = f(3) = 2. ln 3
g(a) = g(2) = 4
g(b) = g(3) = 9
f'(c) = 2/c
g'(c) = 2c
Using Cauchy Mean Value Theorem,
f'(c) / g'(c) = [f(b) – f(a)] / [g(b) – g(a)]
⇒ (2/c)/(2c)= (2. ln 3 – 2. ln 2)/(9 – 4)
⇒ 1/c2 = 2(ln 3 – ln 2)/5
⇒ 1/c2 = 2(ln 3/2)/5
⇒ 5/2[1/ln(3/2)] = c2
⇒ c = √[(5/2)/ln(3/2)]
⇒ c = 2.9 ϵ [2, 3]
FAQs on Cauchy’s Mean Value Theorem
What is Cauchy’s Mean Value Theorem?
Cauchy’s Mean Value Theorem is the fundamental theorem of mathematics which states that, for any function f(x) and g(x) if it is continuous on [a, b] and differntiable from (a, b) then,
[f(b) – f(a)] / [g(b) – g(a)] = f'(c) / g'(c)
where c belongs to (a, b)
Who Proved Cauchy’s Mean Value Theorem?
Cauchy’s Mean Value Theorem is proved by Augustin Louis Cauchy and hence the name Cauchy’s Mean Value Theorem.
What are Applications of Cauchy’s Mean Value Theorem?
Applications of Cauchy’s Mean Value theorem are,
- It is used to solve various problem in Real Analysis.
- It is used to predict the behaviors of various curves.
- It is used to derive Lagrange’s and Rooles Mean value theorems, etc.
State Lagrange’s Mean Value Theorem.
Lagrange’s Mean Value Theorem state that,
For any function f(x) defined on [a, b],
- If it is continuous on [a, b]
- If it is differentiable on (a, b)
Then, there exists a real number c ϵ (a, b) such that,
f'(c) = {f(b) – f(a)}/(b – a)
What is the other name of Cauchy Mean Value Theorem?
Cauchy Mean Value Theorem is also known as Second Mean Value Theorem or Extended Mean Value Theorem
Who proved the Cauchy Mean Value Theorem?
The Cauchy Mean Value Theorem was proved by the French Mathematician Augustin Louis Cauchy in 1823.
Like Article
Suggest improvement
Share your thoughts in the comments
Please Login to comment...