Class 8 RD Sharma Solutions – Chapter 5 Playing with Numbers – Exercise 5.3

Solve each of the following Cryptarithms:

Question 1.

    3       7
+ A      B   
   9       A

Solution:

In the unit’s place,
7 + B = A
In the ten’s place,
3 + A = 9 
From ten’s place A = 6 then B = −1
Hence, it is not possible
Thus, there should be one carry in ten’s place
7 + B > 9
Solve for ten’s place with one carry,
3 + A + 1 = 9
A = 9−1−3 = 5
In unit’s place subtracting 10 as one carry is given to ten’s place,
7 + B – 10= 5
B = 5 + 10 − 7 = 8
Therefore,
A = 5 and B = 8

Question 2.

   A       B
+ 3      7   
   9       A

Solution:

In the unit’s place,
B + 7 = A
In the ten’s place,
A + 3 = 9
From ten’s place A = 6 and B = −1 
It is not possible.
Thus there should be one carry in ten’s place, which means B + 7 > 9
Solve for ten’s place with one carry,
A + 3 + 1 = 9
A = 9 − 4 = 5
In unit’s place subtracting 10 as one carry is given to ten’s place,
B + 7 – 10 = 5
B = 5 + 10 − 7 = 8
Therefore,
 A = 5 and B = 8

Question 3.

   A      1
+ 1      B   
   B      0

Solution:

In the unit’s place,
1 + B = 0
Thus B = -1 
Which is not possible.
Thus, there should be one carry in ten’s place,
A + 1 + 1 = B —-> (equation 1)
For unit’s place, we need to subtract 10 as one carry is given in ten’s place,
1 + B – 10 = 0
B = 10 − 1 = 9
Substituting B = 9 in (equation 1),
A + 1 + 1 = 9
A = 9 − 1 − 1 = 7
Therefore, A = 7 and B = 9

Question 4.

    2      A      B
+ A      B      1   
    B      1      8

Solution:

In the unit’s place,
B + 1 = 8
B = 7
In the ten’s place,
A + B = 1
A + 7 = 1
A = −6 
Which is not possible.
Hence, A + B > 9
We know that now there should be one carry in hundred’s place, and so we need to subtract 10 from ten’s place,
That is,
A + B – 10 = 1
A + 7 = 11
A = 11 − 7 = 4
Now to check whether our values of A and B are correct, we should solve for hundred’s place.
2 + A + 1 = B
2 + 4 + 1 = 7
7 = 7
Hence, 
RHS = LHS
Therefore, 
A = 4 and B = 7

Question 5.

    1      2      A
+ 6      A      B   
    A     0      9

Solution:

In the unit’s place,
A + B = 9 —-> (equation 1)
With this condition we know that sum of 2 digits can be greater than 18.
So, there is no need to carry one from ten’s place.
In the ten’s place,
2 + A = 0
Which means A = −2 
Which is not possible
Hence, 
2 + A > 9
Now, there should be one carry in hundred’s place and hence we need to subtract 10 from ten’s place,
That is, 
2 + A – 10 = 0
A = 10 − 2 = 8
Now, substituting A=8 in (equation 1),
A + B = 9
8 + B = 9
B = 9 – 8
B = 1
Therefore, 
A = 8 and B = 1

Question 6.

    A      B      7
+ 7       A      B   
    A      0      9

Solution:

In the unit’s place,
The two conditions are, 
(i) 7 + B ≤ 9 
(ii) 7 + B > 9
For 7 + B ≤ 9
7 + B = A
A – B = 7 —->(equation 1)
In the ten’s place,
B + A = 8 —-> (equation 2)
Solve equation 1 and equation 2,
2A = 15 which means A = 7.5 
Which is not possible
Thus, first condition 7 + B ≤ 9 is wrong.
Therefore,
7 + B > 9 is correct condition
Hence, there should be one carry in ten’s place and subtracting 10 from unit’s place,
7 + B – 10 = A
B – A = 3 —-> (equation 3)
In the ten’s place,
B + A + 1 = 8
B + A = 8 − 1
B + A = 7 —-> (equation 4)
Solve equation 3 and equation 4,
2B = 10
B =  10  = 5
2
Substituting the value of B in equation 4
B + A = 7
5 + A = 7
A = 7 − 5 = 2
Therefore, 
B = 5 and A = 2

Question 7. Show that the Cryptarithm does not have any solution.

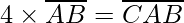
Solution:

If B is multiplied by 4 then only 0 satisfies the above condition.
So, for unit place to satisfy the above condition, we should have B = 0.
In ten’s place, only 0 satisfies the above condition.
But, AB cannot be 00 as 00 is not a two digit number.
So, A and B cannot be equal to 0
Therefore, 
There is no solution satisfying the condition