Program for Method Of False Position

Given a function f(x) on floating number x and two numbers ‘a’ and ‘b’ such that f(a)*f(b) < 0 and f(x) is continuous in [a, b]. Here f(x) represents algebraic or transcendental equation. Find root of function in interval [a, b] (Or find a value of x such that f(x) is 0).

Input: A function of x, for example x3 – x2 + 2.     
       And two values: a = -200 and b = 300 such that
       f(a)*f(b) < 0, i.e., f(a) and f(b) have
       opposite signs.
Output: The value of root is : -1.00
        OR any other value close to root.

We strongly recommend to refer below post as a prerequisite of this post. 
Solution of Algebraic and Transcendental Equations | Set 1 (The Bisection Method)
In this post The Method Of False Position is discussed. This method is also known as Regula Falsi or The Method of Chords. 
Similarities with Bisection Method:

1. Same Assumptions: This method also assumes that function is continuous in [a, b] and given two numbers ‘a’ and ‘b’ are such that f(a) * f(b) < 0.
2. Always Converges: like Bisection, it always converges, usually considerably faster than Bisection–but sometimes very much more slowly than Bisection.

Differences with Bisection Method:
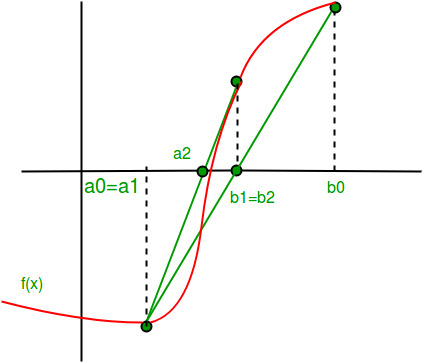
It differs in the fact that we make a chord joining the two points [a, f(a)] and [b, f(b)]. We consider the point at which the chord touches the x axis and named it as c. 
Steps:

1. Write equation of the line connecting the two points. 
   

y – f(a) =  ( (f(b)-f(a))/(b-a) )*(x-a)

Now we have to find the point which touches x axis. 
For that we put y = 0.

so x = a - (f(a)/(f(b)-f(a))) * (b-a)
   x = (a*f(b) - b*f(a)) / (f(b)-f(a)) 

This will be our c that is c = x. 

1. If f(c) == 0, then c is the root of the solution.
2. Else f(c) != 0
   1. If value f(a)*f(c) < 0 then root lies between a and c. So we recur for a and c
   2. Else If f(b)*f(c) < 0 then root lies between b and c. So we recur b and c.
   3. Else given function doesn’t follow one of assumptions.

Since root may be a floating point number and may converge very slow in worst case, we iterate for a very large number of times such that the answer becomes closer to the root.

Following is the implementation. 

C++

// C++ program for implementation of Regular Falsi Method for
// solving equations
#include<bits/stdc++.h>
using namespace std;
#define MAX_ITER 1000000

// An example function whose solution is determined using
// Regular Falsi Method. The function is x^3 - x^2  + 2
double func(double x)
{
    return x*x*x - x*x + 2;
}

// Prints root of func(x) in interval [a, b]
void regulaFalsi(double a, double b)
{
    if (func(a) * func(b) >= 0)
    {
        cout << "You have not assumed right a and b\n";
        return;
    }

    double c = a;  // Initialize result

    for (int i=0; i < MAX_ITER; i++)
    {
        // Find the point that touches x axis
        c = (a*func(b) - b*func(a))/ (func(b) - func(a));

        // Check if the above found point is root
        if (func(c)==0)
            break;

        // Decide the side to repeat the steps
        else if (func(c)*func(a) < 0)
            b = c;
        else
            a = c;
    }
    cout << "The value of root is : " << c;
}

// Driver program to test above function
int main()
{
    // Initial values assumed
    double a =-200, b = 300;
    regulaFalsi(a, b);
    return 0;
}

Java

// java program for implementation
// of Bisection Method for
// solving equations
import java.io.*;

class GFG {

    static int MAX_ITER = 1000000;

    // An example function whose
    // solution is determined using
    // Regular Falsi Method. The function
    // is x^3 - x^2 + 2
    static double func(double x)
    {
        return (x * x * x - x * x + 2);
    }

    // Prints root of func(x)
    // in interval [a, b]
    static void regulaFalsi(double a, double b)
    {
        if (func(a) * func(b) >= 0)
        {
            System.out.println("You have not assumed right a and b");
        }
        // Initialize result
        double c = a;

        for (int i = 0; i < MAX_ITER; i++)
        {
            // Find the point that touches x axis
            c = (a * func(b) - b * func(a))
                 / (func(b) - func(a));

            // Check if the above found point is root
            if (func(c) == 0)
                break;

            // Decide the side to repeat the steps
            else if (func(c) * func(a) < 0)
                b = c;
            else
                a = c;
        }
        System.out.println("The value of root is : " + (int)c);
    }

    // Driver program
    public static void main(String[] args)
    {
        // Initial values assumed
        double a = -200, b = 300;
        regulaFalsi(a, b);
    }
}

// This article is contributed by vt_m

Python3

# Python3 implementation of Bisection
# Method for solving equations

MAX_ITER = 1000000

# An example function whose solution
# is determined using Regular Falsi Method.
# The function is x^3 - x^2 + 2
def func( x ):
    return (x * x * x - x * x + 2)

# Prints root of func(x) in interval [a, b]
def regulaFalsi( a , b):
    if func(a) * func(b) >= 0:
        print("You have not assumed right a and b")
        return -1

    c = a # Initialize result

    for i in range(MAX_ITER):

        # Find the point that touches x axis
        c = (a * func(b) - b * func(a))/ (func(b) - func(a))

        # Check if the above found point is root
        if func(c) == 0:
            break

        # Decide the side to repeat the steps
        elif func(c) * func(a) < 0:
            b = c
        else:
            a = c
    print("The value of root is : " , '%.4f' %c)

# Driver code to test above function
# Initial values assumed
a =-200
b = 300
regulaFalsi(a, b)

# This code is contributed by "Sharad_Bhardwaj".

C#

// C# program for implementation
// of Bisection Method for
// solving equations
using System;

class GFG {

    static int MAX_ITER = 1000000;

    // An example function whose
    // solution is determined using
    // Regular Falsi Method. The function
    // is x^3 - x^2 + 2
    static double func(double x)
    {
        return (x * x * x - x * x + 2);
    }

    // Prints root of func(x)
    // in interval [a, b]
    static void regulaFalsi(double a, double b)
    {
        if (func(a) * func(b) >= 0)
        {
            Console.WriteLine("You have not assumed right a and b");
        }
        // Initialize result
        double c = a;

        for (int i = 0; i < MAX_ITER; i++)
        {
            // Find the point that touches x axis
            c = (a * func(b) - b * func(a))
                / (func(b) - func(a));

            // Check if the above found point is root
            if (func(c) == 0)
                break;

            // Decide the side to repeat the steps
            else if (func(c) * func(a) < 0)
                b = c;
            else
                a = c;
        }

        Console.WriteLine("The value of root is : " + (int)c);
    }

    // Driver program
    public static void Main(String []args)
    {
        // Initial values assumed
        double a = -200, b = 300;
        regulaFalsi(a, b);
    }
}

// This code is contributed by Sam007.

Javascript

<script>

// JavaScript program for implementation of Bisection Method for
// solving equations
  let MAX_ITER = 1000000

  // An example function whose solution is determined using
  // Regular Falsi Method. The function is x^3 - x^2  + 2
  function func(x){
      return x*x*x - x*x + 2;
  }

  // Prints root of func(x) in interval [a, b]
  function regulaFalsi( a, b){
      if (func(a) * func(b) >= 0){
          document.write("You have not assumed right a and b\n");
          return;
      }
// Initialize result
      let c = a;

      for (let i=0; i < MAX_ITER; i++)
      {
          // Find the point that touches x axis
          c = Math.floor((a*func(b) - b*func(a))/ (func(b) - func(a)));

          // Check if the above found point is root
          if (func(c)==0){
              break;
          }
          // Decide the side to repeat the steps
          else if (func(c)*func(a) < 0){
              b = c;
          }
          else{
              a = c;
          }
      }
      document.write("The value of root is : " + c);
  }

  // Driver program to test above function

  // Initial values assumed
  let a =-200;
  let b = 300;
  regulaFalsi(a, b);

</script>

Output

The value of root is : -1

This method always converges, usually considerably faster than Bisection. But worst case can be very slow. 
We will soon be discussing other methods to solve algebraic and transcendental equations.
References:
Introductory Methods of Numerical Analysis by S.S. Sastry
https://en.wikipedia.org/wiki/False_position_method
Please write comments if you find anything incorrect, or you want to share more information about the topic discussed above