Inequalities

Inequalities are the expressions which define the relation between two vales which are not equal. i.e., one side can be greater or smaller than the other. In mathematics along with equalities, inequalities are also important as the height of two individuals is not exactly the same, one is always taller or shorter than the other.

Inequalities

Therefore need for inequalities arises and using inequalities we can show these relations between objects based on the parameter such as taller and shorter, large and small, high and low, etc. 

In this article, we will learn about Inequalities including their symbols, rules/properties, types, and their graphical representations. Other than these topics, we will also learn how to solve different inequalities and systems of inequalities using graphs as well as algebra. So let’s start our learning on the topic of Inequalities.

What are Inequalities in Math?

The mathematical expression in which the LHS and RHS are unequal i.e., one is greater than the other or one is smaller than the other, is called inequalities. In other words, the statements in which both sides of the expression are related with an inequality symbol then it is called inequalities. 

As we already discussed, in inequalities, both sides are unequal means it can be greater than, less than, greater than equal to, less than equal to, or not equal.

Inequality Symbols

Inequality symbols are listed below:

Inequality Name	Symbol	Expression	Description
Greater than	>	x > a	x is greater than a
Less than	<	x < a	x is less than a
Greater than equal to	≥	x ≥ a	x is greater than or equal to a
Less than equal to	≤	x ≤ a	x is less than or equal to a
Not equal	≠	x ≠ a	x is not equal to a

Rules of Inequalities

There are various rules in inequalities to help us relate to and solve various different inequalities. Some of these rules are discussed as follows:

Rule 1

If a, b, and c are three numbers then, inequality between these numbers follows transitive property.

• If a > b and b > c, then a > c
• If a < b and b < c, then a < c
• If a ≥ b and b ≥ c, then a ≥ c
• If a ≤ b and b ≤ c, then a ≤ c

Rule 2

If the LHS and RHS of the expressions are exchanged, then the inequality reverses. It is called converse property.

• If a > b, then b < a
• If a < b, then b > a
• If a ≥ b, then b ≤ a
• If a ≤ b, then b ≥ a

Rule 3

If the same constant k is added or subtracted from both sides of the inequality, then both sides of the inequality are equal.

• If a > b, then a + k > b + k
• If a > b, then a – k > b – k

Similarly, for other inequalities.

• If a < b, then a + k < b + k
• If a < b, then a – k < b – k
• If a ≤ b, then a + k ≤ b + k
• If a ≤ b, then a – k ≤ b – k
• If a ≥ b, then a + k ≥ b + k
• If a ≥ b, then a – k ≥ b – k

The direction of the inequality does not change after adding or subtracting a constant.

Rule 4

If k is a positive constant that is multiplied or divided by both sides of the inequality, then there is no change in the direction of the inequality.

• If a > b, then ak > bk
• If a < b, then ak < bk
• If a ≤ b, then ak ≤ bk
• If a ≥ b, then ak ≥ bk

If k is a negative constant that is multiplied or divided by both sides of the inequality, then the direction of inequality gets reversed.

• If a > b, then ak < bk
• If a > b, then ak < bk
• If a ≥ b, then ak ≤ bk
• If a ≤ b, then ak ≥ bk

Rule 5

The square of any number is always greater than or equal to zero.

• a² ≥ 0

Rule 6

Taking square roots on both sides of the inequality does not change the direction of the inequality.

• If a > b, then √a > √b
• If a < b, then √a < √b
• If a ≥ b, then √a ≥ √b
• If a ≤ b, then √a ≤ √b

Graph for Inequalities

Inequalities are either with one variable or two or we have a system of inequalities, all of them can be graphed to the cartesian plane if it only contains two variables. Inequalities in one variable are plotted on real lines and two variables are plotted on the cartesian plane. 

Interval Notation for Inequalities

Important points for writing intervals for inequalities:

• In case of greater than and equal to (≥) or less than equal to (≤), the end values are included so, closed or square brackets [ ] are used.
• In case of greater than (>) or less than (<), the end values are excluded so, open brackets () are used.
• For both positive and negative infinity open brackets () are used.

The following table represents intervals for different inequalities:

Inequality	Interval
x > a	(a, ∞)
x < a	(-∞, a)
x ≥ a	[a, ∞)
x ≤ a	(-∞, a]
a < x ≤ b	(a, b]

Graph for Linear Inequalities with One Variable

From the following table we can understand, how to plot various Linear Inequalities with One Variable on a real line.

Inequality	Interval	Graph
x > 1	(1, ∞)
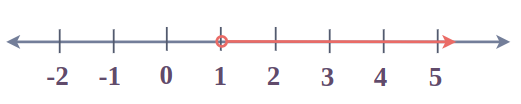
x < 1	(-∞, 1)
x ≥ 1	[1, ∞)
x ≤ 1	(-∞, 1]

Graph for Linear Inequalities with Two Variable

Let’s take an example of linear inequalities with two variables.

Consider the linear inequality 20x + 10y ≤ 60, as the possible solutions for given inequality are (0, 0), (0,1), (0, 2), (0,3), (0,4), (0,5), (0,6), (1,0), (1,1), (1,2), (1,3), (1,4), (2,0), (2,1), (2,2), (3,0), and also all the points beyond these points are also the solution of the inequality.

Let’s plot the graph from the given solutions.

The shaded region in the graph represents the possible solutions for the given inequality.

Types of Inequalities

There are various types of inequalities that can be classified as follows:

• Polynomial Inequalities: Polynomial inequalities are inequalities that can be represented in the form of polynomials. Example- 2x + 3 ≤ 10.
• Absolute Value Inequalities: Absolute value inequalities are the inequalities within the absolute value sign. Example- |y + 3| ≤ 4.
• Rational Inequalities: Rational inequalities are inequalities with fractions along with the variables. Example- (x + 4) / (x – 5) < 5.

How to Solve Inequalities

To solve the inequalities, we can use the following steps:

• Step 1: Write the inequality in the form of the equation.
• Step 2: Solve the equation and obtain the roots of the inequalities.
• Step 3: Represent the obtained values on the number line.
• Step 4: Represent the excluded values also on the number line with the open circles.
• Step 5: Find the intervals from the number line.
• Step 6: Take a random value from each interval and put these values in the inequality and check if it satisfies the inequality.
• Step 7: The solution for the inequality is the intervals that satisfy the inequality.

How to Solve Polynomial Inequalities

Polynomial Inequalities include linear inequalities, quadratic inequalities, cubic inequalities, etc. Here we will learn to solve linear and quadratic inequalities.

Solving Linear Inequalities

Linear inequalities can be solved like linear equations but according to the inequalities rule. Linear inequalities can be solved using simple algebraic operations.

One or two-step inequalities

One-step inequality is inequalities that can be solved in one step.

Example: Solve: 5x < 10

Solution:

⇒ 5x < 10 [Dividing both sides by 5]

⇒ x < 2 or (-∞, 2)

Two-step inequality are inequalities that can be solved in two steps.

Example: Solve: 4x + 2 ≥ 10

Solution:

⇒ 4x + 2 ≥ 10

⇒ 4x ≥ 8 [Subtracting 2 from both sides]

⇒ 4x ≥ 8 [Dividing both sides by 4]

⇒ x ≥ 2 or [2, ∞)

Compound Inequalities

Compound inequalities are inequalities that have multiple inequalities separated by “and” or “or”. To solve compound inequalities, solve the inequalities separately, and for the final solution perform the intersection of obtained solutions if the inequalities are separated by “and” and perform the union of obtained solutions if the inequalities are separated by “or”.

Example: Solve: 4x + 6 < 10 and 5x + 2 < 12

Solution:

First solve 4x + 6 < 10

⇒ 4x + 6 < 10 [Subtracting 6 from both sides]

⇒ 4x < 4

⇒ x < 1 or (-∞, 1) —–(i)

Second solve 5x + 2 < 12

⇒ 5x + 2 < 12 [Subtracting 2 from both sides]

⇒ 5x < 10

⇒ x < 2 or (-∞, 2) ——-(ii)

From (i) and (ii) we have two solutions x < 1 and x < 2.

We take intersection for the final solution as the inequalities are separated by and.

⇒ (-∞, 1) ∩ (-∞, 2)

⇒ (-∞, 1)

The final solution for given compound inequality is (-∞, 1).

Read More

• Compound Inequalities
• Word Problems of Linear Inequalities
• Triangle Inequality

Solving Quadratic Inequalities

Let’s take an example to solve absolute value inequalities.

Example: Solve the inequality: x² – 7x + 6 ≥ 0

Solution:

Following are the steps to solve inequality: x² – 7x + 6 ≥ 0

Step 1: Write the inequality in the form of equation:

x² – 7x + 6 = 0

Step 2: Solve the equation:

x² – 7x + 6 = 0

x² – 6x – x + 6 = 0

x(x – 6) – 1(x – 6) = 0

(x – 6) (x – 1) = 0

x = 6 and x = 1

From above step we obtain values x = 6 and x = 1

Step 3: From above values the intervals are (-∞, 1], [1, 6], [6, ∞)

Since, the inequality is ≥ that includes equal to, so we use closed bracket for the obtained values.

Step 4: Number line representation of above intervals.

Step 5: Take random numbers between each interval and check if it satisfies the value. If it satisfies, then include interval in the solution.

For interval (-∞, 1] let random value be -1.

Putting x = -1 in the inequality x² – 7x + 6 ≥ 0

⇒ (-1)² – 7(-1) + 6 ≥ 0

⇒ 1 + 7 + 6 ≥ 0

⇒ 14 ≥ 0 (True)

For interval [1, 6] let random value be 2.

Putting x = 0 in the inequality x² – 7x + 6 ≥ 0

⇒ 2² – 7(2) + 6 ≥ 0

⇒ 4 – 14 + 6 ≥ 0

⇒ -4 ≥ 0 (False)

For interval [6, ∞) let random value be 7.

Putting x = 7 in the inequality x² – 7x + 6 ≥ 0

⇒ 7² – 7(7) + 6 ≥ 0

⇒ 49 – 49 + 6 ≥ 0

⇒ 6 ≥ 0 (True)

Step 6: So, the solution for the absolute value inequality x² – 7x + 6 ≥ 0 is the interval (-∞, 1] ∪ [6, ∞) as it satisfies the inequality which can be plotted on the number line as:

How to Solve Absolute Value Inequalities

Let’s take an example to solve absolute value inequalities.

Example: Solve the inequality: |y + 1| ≤ 2

Solution:

Following are the steps to solve inequality: |y + 1| ≤ 2

Step 1: Write the inequality in the form of an equation:

|y + 1| = 2

Step 2: Solve the equation:

y + 1 = ∓ 2

y + 1 = 2 and y + 1 = – 2

y = 1 and y = -3

From above step we obtain values y = 1 and y = -3

Step 3: From above values the intervals are (-∞, -3], [-3, 1], [1, ∞)

Since, the inequality is ≤ that includes equal to, so we use closed bracket for the obtained values.

Step 4: Number line representation of above intervals.

Step 5: Take random numbers between each interval and check if it satisfies the value. If it satisfies, then include interval in the solution.

For interval (-∞, -3] let random value be -4.

Putting y = -4 in the inequality |y + 1| ≤ 2

⇒ |-4+ 1| ≤ 2

⇒ |-3| ≤ 2

⇒ 3 ≤ 2 (False)

For interval [-3, 1] let random value be 0.

Putting y = 0 in the inequality |y + 1| ≤ 2

⇒ |0+ 1| ≤ 2

⇒ |1| ≤ 2

⇒ 1 ≤ 2 (True)

For interval [1, ∞) let random value be 2.

Putting y = 2 in the inequality |y + 1| ≤ 2

⇒ |2+ 1| ≤ 2

⇒ |3| ≤ 2

⇒ 3 ≤ 2 (False)

Step 6: So, the solution for the absolute value inequality |y + 1| ≤ 2 is interval [-3, -1] as it satisfies the inequality which can be plotted on the number line as:

How to Solve Rational Inequalities

Let’s take an example to solve rational inequalities.

Example: Solve the inequality: (x + 3) / (x – 1) < 2

Solution:

Following are the steps to solve inequality:

Step 1: Write the inequality in the form of equation: (x + 3) / (x – 1) < 2

(x + 3) / (x – 1) = 2

Step 2: Solve the equation:

(x + 3) / (x – 1) = 2

(x + 3) = 2(x – 1)

x + 3 = 2x – 2

2x – x = 3 + 2

x = 5

From above step we obtain value x = 5

Step 3: From above values the intervals are (-∞,1), (1, 5), (5, ∞)

Since, the inequality is < that does not include equal to, so we use open bracket for the obtained value.

Since, for x = 1 the inequality is undefined, so we take open bracket for x = 1.

Step 4: Number line representation of above intervals.

Step 5: Take random numbers between each interval and check if it satisfies the value. If it satisfies, then include interval in the solution.

For interval (-∞, 1) let random value be 0.

Putting x = 0 in the inequality (x + 3) / (x – 1) < 2

⇒ (0 + 3) / (0 – 1) < 2

⇒ 3 / (-1) < 2

⇒ -3 < 2 (True)

For interval (1, 5) let random value be 2.

Putting x = 3 in the inequality (x + 3) / (x – 1) < 2

⇒ (3 + 3) / (3 – 1) < 2

⇒ 6 / 2 < 2

⇒ 3 < 2 (False)

For interval (5, ∞) let random value be 2.

Putting y = 6 in the inequality (x + 3) / (x – 1) < 2

⇒ (6 + 3) / (6 – 1) < 2

⇒ 9 / 5 < 2

⇒ 1.8 < 2 (True)

Step 6: So, the solution for the absolute value inequality (x + 3) / (x – 1) < 2 is interval (-∞, 1) ∪ (5, ∞) as it satisfies the inequality which can be plotted on the number line as:

How to Solve Linear Inequality with Two Variables

Let’s take an example to solve linear inequality with two variables.

Example: Solve: 20x + 10y ≤ 60

Solution:

Consider x = 0 and put it in the given inequality

⇒ 20x + 10y ≤ 60

⇒ 20(0) + 10y ≤ 60

⇒ 10y ≤ 60

⇒ y ≤ 6 ——(i)

Now, when x = 0, y can be 0 to 6.

Similarly, putting values in inequality and check it satisfies the inequality.

For x = 1, y can be 0 to 4.

For x = 2, y can be 0 to 2.

For x = 3, y can be 0.

The possible solution for given inequality is (0, 0), (0,1), (0, 2), (0,3), (0,4), (0,5), (0,6), (1,0), (1,1), (1,2), (1,3), (1,4), (2,0), (2,1), (2,2), (3,0).

Systems of Inequalities

The systems of inequalities are the set of two or more inequalities with one or more variables. Systems of inequalities contain multiple inequalities with one or more variables.

The system of inequalities is of the form:

a₁₁x₁ + a₁₂x₂ + a₁₃x₃ …….. + a_1nx_n < b₁

a₂₁x₁ + a₂₂x₂ + a₂₃x₃ …….. + a_2nx_n < b₂

┋ ┋ ┋ ┋ ┋

a_n1x₁ + a_n2x₂ + a_n3x₃ …….. + a_nnx_n < b_n

Graphical Representation of Systems of Inequalities

System of inequalities is a group of multiple inequalities. First, solve each inequality and plot the graph for each inequality. The intersection of the graph of all the inequalities represents the graph for systems of inequalities.

Consider an example,

Example: Plot graph for systems of inequalities

• 2x + 3y ≤ 6
• x ≤ 3
• y ≤ 2

Solution:

Graph for 2x + 3y ≤ 6

Shaded region of the graph represents 2x + 3y ≤ 6

Graph for x ≤ 3

Shaded region represents x ≤ 3

Graph for y ≤ 2

Shaded region represents y ≤ 2

Graph for given system of inequalities

Shaded region represents given system of inequalities.

Inequalities – FAQs

What are Inequalities?

Inequalities are the mathematical expressions in which the LHS and RHS of the expression are unequal.

What are the Symbols for Inequalities?

The symbols of inequalities are: >, <, ≥, ≤ and ≠.

What is the Transitive Property of Inequalities?

The transitive property of inequalities states that if a, b, c are three numbers then,

• If a > b and b > c, then a > c
• If a < b and b < c, then a < c
• If a ≥ b and b ≥ c, then a ≥ c
• If a ≤ b and b ≤ c, then a ≤ c

Mention the Conditions in which the Direction of Inequality Changes.

Conditions in which the direction of inequality changes:

• When a negative number is multiplied or divided with both the sides of the inequality.
• When the LHS and RHS of the inequality are exchanged.
• When we take reciprocal of both the sides of the inequality (when both sides are positive or when both sides are negative)

Give some Examples of Inequalities.

Some examples of inequalities are:

• 3x + 6 > 9
• 9x + 3y < 15
• 8x + 2 ≤ 18