Given a number N in decimal base, find number of its digits in any base (base b)

Given A Number n in a base 10, find the number of digits in its base b representation. 
Constraints : 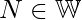Whole 
Examples :

Input : Number = 48 
        Base = 4
Output: 3
Explanation : (48)10 = (300)4

Input : Number = 1446
        Base = 7
Output: 4
Explanation : (446)10 = (4134)7

A simple approach: convert the decimal number into the given base r and then count number of digits.
An efficient approach : It resides on the relationship between the base of the number and number of digits of that number. 
Typically : Let n be a positive integer. The base 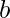representation of 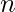has 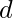digits if 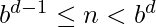, which is the case if 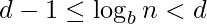or 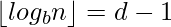.The number of digits in the base b representation of n is therefore 

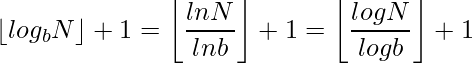
In above equation the base changing logarithmic property has been used. So we calculate the logarithm of the number in that base which we want to calculate the number of digits. And take its floor value and then add 1. 
This idea can be further used to find the number of digits of a given number n of base b in base r. All have to be done is to convert the number in base 10 and then apply the above formula of finding digits. It would be easier to calculate log of any base when number is in base 10. 

C++

// C++ program to Find Number of digits
// in base b.
#include <iostream>
#include <math.h>
using namespace std;

// function to print number of
// digits
void findNumberOfDigits(long n, int base)
{
    // Calculating log using base
    // changing property and then
    // taking it floor and then
    // adding 1.
    int dig = (int)(floor( log(n) /
                         log(base)) + 1);

    // printing output
    cout << "The Number of digits of "
         << "Number " << n << " in base "
         << base << " is " << dig;
}

// Driver method
int main()
{
    // taking inputs
    long n = 1446;
    int base = 7;

    // calling the method
    findNumberOfDigits(n, base);
    return 0;
}

// This code is contributed by Manish Shaw
// (manishshaw1)

Java

// Java program to Find Number
// of digits in base b.
import java.io.*;
public class GFG {

    // function to print number of digits
    static void findNumberOfDigits(long n, int base)
    {

        // Calculating log using base changing
        // property and then taking it
        // floor and then adding 1.
        int dig = (int)(Math.floor(
                        Math.log(n) / Math.log(base))
                        + 1);


        // printing output
        System.out.println("The Number of digits of Number "
                            + n + " in base " + base
                            + " is " + dig);
    }

    // Driver method
    public static void main(String[] args)
    {
        // taking inputs
        long n = 1446;
        int base = 7;

        // calling the method
        findNumberOfDigits(n, base);
    }
}

Python3

# Python3 program to Find Number of digits
# in base b.

import math

# function to print number of
# digits
def findNumberOfDigits(n, base):

    # Calculating log using base
    # changing property and then
    # taking it floor and then
    # adding 1.
    dig = (math.floor(math.log(n) /
                 math.log(base)) + 1)

    # printing output
    print ("The Number of digits of"
      " Number {} in base {} is {}"
            . format(n, base, dig))

# Driver method

# taking inputs
n = 1446
base = 7

# calling the method
findNumberOfDigits(n, base)

# This code is contributed by
# Manish Shaw (manishshaw1)

C#

// C# program to Find Number of digits
// in base b.
using System;

class GFG {

    // function to print number of
    // digits
    static void findNumberOfDigits(long n,
                                    int b)
    {
        // Calculating log using base
        // changing property and then
        // taking it floor and then
        // adding 1.
        int dig = (int)(Math.Floor(
          Math.Log(n) / Math.Log(b)) + 1);

        // printing output
        Console.Write("The Number of digits"
           + " of Number " + n + " in base "
                        + b + " is " + dig);
    }

    // Driver method
    public static void Main()
    {
        // taking inputs
        long n = 1446;
        int b = 7;

        // calling the method
        findNumberOfDigits(n, b);
    }
}

// This code is contributed by Manish Shaw
// (manishshaw1)

PHP

<?php
// PHP program to Find Number
// of digits in base b.

// function to print
// number of digits
function findNumberOfDigits($n, $b)
{
    // Calculating log using base
    // changing property and then
    // taking it floor and then
    // adding 1.
    $dig = (int)(floor(log($n) /
                       log($b)) + 1);

    // printing output
    echo ("The Number of digits".
               " of Number ". $n.
                  " in base ".$b.
                    " is ".$dig);
}

// Driver Code
$n = 1446;
$b = 7;

// calling the method
findNumberOfDigits($n, $b);

// This code is contributed by
// Manish Shaw (manishshaw1)
?>

Javascript

<script>

// Javascript program to Find Number of digits
// in base b.

// function to print number of
// digits
function findNumberOfDigits(n, base)
{
    // Calculating log using base
    // changing property and then
    // taking it floor and then
    // adding 1.
    var dig = parseInt(Math.floor( Math.log(n) /
                        Math.log(base)) + 1);

    // printing output
    document.write("The Number of digits of "
        + "Number " + n + " in base "
        + base + " is " + dig);
}

// Driver method

// taking inputs
var n = 1446;
var base = 7;

// calling the method
findNumberOfDigits(n, base);

</script>

Output :

The Number of digits of Number 1446 in base 7 is 4

Complexity Analysis:
Time complexity : O(logN)
Space complexity : O(1)

Optimized Approach: 

Here are few optimizations that can be made to the given program:

• Avoid using math.h library: The log function provided by the math.h library is a costly operation. Instead of using it, we can use the base changing property of logarithm and use log10 function to calculate the logarithm of n in base b. This will reduce the overhead of including the math.h library.
• Replace floor function with casting: Instead of using the floor function, we can cast the result of the logarithmic operation to an integer type. This is faster and simpler than calling the floor function.

Here’s the optimized version of the program with the above optimizations:

C++

#include <iostream>
using namespace std;

void findNumberOfDigits(long n, int base)
{
    int dig = 0;
    while (n > 0) {
        n /= base;
        dig++;
    }
    cout << "The Number of digits of "
         << "Number " << n << " in base "
         << base << " is " << dig;
}

int main()
{
    long n = 1446;
    int base = 7;
    findNumberOfDigits(n, base);
    return 0;
}

Java

import java.util.*;

public class Main {
    public static void findNumberOfDigits(long n, int base) {
        int dig = 0;
        while (n > 0) {
            n /= base;
            dig++;
        }
        System.out.println("The Number of digits of Number " + n + " in base " + base + " is " + dig);
    }

    public static void main(String[] args) {
        long n = 1446;
        int base = 7;
        findNumberOfDigits(n, base);
    }
}

Python3

def findNumberOfDigits(n, base):
    dig = 0
    while n > 0:
        n //= base
        dig += 1
    print(f"The Number of digits of Number {n} in base {base} is {dig}")

n = 1446
base = 7
findNumberOfDigits(n, base)

C#

using System;

public class Program
{
    public static void FindNumberOfDigits(long n, int baseVal)
    {
        int dig = 0;
        while (n > 0)
        {
            n /= baseVal;
            dig++;
        }
        Console.WriteLine("The Number of digits of Number " + n + " in base " + baseVal + " is " + dig);
    }

    public static void Main()
    {
        long n = 1446;
        int baseVal = 7;
        FindNumberOfDigits(n, baseVal);
    }
}

Javascript

function findNumberOfDigits(n, base) {
    let dig = 0;
    while (n > 0) {
        n = Math.floor(n / base);
        dig++;
    }
    console.log(`The Number of digits of Number ${n} in base ${base} is ${dig}`);
}

let n = 1446;
let base = 7;
findNumberOfDigits(n, base);

Output : 
The Number of digits of Number 1446 in base 7 is 4

Complexity Analysis:
Time complexity : O(logN)
Auxiliary Space : O(1)