Find Nth number in a sequence which is not a multiple of a given number

Given four integers A, N, L and R, the task is to find the N th number in a sequence of consecutive integers from L to R which is not a multiple of A. It is given that the sequence contain at least N numbers which are not divisible by A and the integer A is always greater than 1.

Examples:

Input: A = 2, N = 3, L = 1, R = 10 
Output: 5 
Explanation:
The sequence is 1, 2, 3, 4, 5, 6, 7, 8, 9 and 10. Here 5 is the third number which is not a multiple of 2 in the sequence.

Input: A = 3, N = 6, L = 4, R = 20 
Output: 11 
Explanation : 
11 is the 6th number which is not a multiple of 3 in the sequence. 

Naive Approach: The naive approach is to iterate over the range [L, R] in a loop to find the N^th number. The steps are:  

1. Initialize the count of non-multiple number and current number to 0.
2. Iterate over the range [L, R] until the count of the non-multiple number is not equal to N.
3. Increment the count of the non-multiple number by 1, If the current number is not divisible by A.

Below is the implementation of the above approach:  

C++

// C++ program for the above approach
#include <bits/stdc++.h>
using namespace std;

// Function to find Nth number not a
// multiple of A in the range [L, R]
void count_no (int A, int N, int L, int R)
{

    // To store the count
    int count = 0;
    int i = 0;

    // To check all the nos in range
    for(i = L; i < R + 1; i++)
    {
    if (i % A != 0)
        count += 1;

    if (count == N)
        break;
    }
    cout << i;
}

// Driver code
int main()
{

    // Given values of A, N, L, R
    int A = 3, N = 6, L = 4, R = 20;

    // Function Call
    count_no (A, N, L, R);
    return 0;
}

// This code is contributed by mohit kumar 29

Java

// Java program for the above approach
import java.util.*;
import java.io.*;

class GFG{

// Function to find Nth number not a
// multiple of A in the range [L, R]
static void count_no (int A, int N,
                      int L, int R)
{

    // To store the count
    int count = 0;
    int i = 0;

    // To check all the nos in range
    for(i = L; i < R + 1; i++)
    {
        if (i % A != 0)
            count += 1;

        if (count == N)
            break;
    }
    System.out.println(i);
}

// Driver code
public static void main(String[] args)
{

    // Given values of A, N, L, R
    int A = 3, N = 6, L = 4, R = 20;

    // Function call
    count_no (A, N, L, R);
}
}

// This code is contributed by sanjoy_62

Python3

# Python3 program for the above approach

# Function to find Nth number not a
# multiple of A in the range [L, R]
def count_no (A, N, L, R):

    # To store the count
    count = 0

    # To check all the nos in range
    for i in range ( L, R + 1 ):
        if ( i % A != 0 ):
            count += 1

        if ( count == N ):
            break
    print ( i )

# Given values of A, N, L, R
A, N, L, R = 3, 6, 4, 20

# Function Call
count_no (A, N, L, R)

C#

// C# program for the above approach
using System;

class GFG{

// Function to find Nth number not a
// multiple of A in the range [L, R]
static void count_no (int A, int N,
                      int L, int R)
{

    // To store the count
    int count = 0;
    int i = 0;

    // To check all the nos in range
    for(i = L; i < R + 1; i++)
    {
        if (i % A != 0)
            count += 1;

        if (count == N)
            break;
    }
    Console.WriteLine(i);
}

// Driver code
public static void Main()
{

    // Given values of A, N, L, R
    int A = 3, N = 6, L = 4, R = 20;

    // Function call
    count_no (A, N, L, R);
}
}

// This code is contributed by sanjoy_62

Javascript

<script>

// Javascript Program to implement
// the above approach

// Function to find Nth number not a
// multiple of A in the range [L, R]
function count_no (A, N, L, R)
{

    // To store the count
    let count = 0;
    let i = 0;

    // To check all the nos in range
    for(i = L; i < R + 1; i++)
    {
        if (i % A != 0)
            count += 1;

        if (count == N)
            break;
    }
   document.write(i);
}

// Driver Code

    // Given values of A, N, L, R
    let A = 3, N = 6, L = 4, R = 20;

    // Function call
    count_no (A, N, L, R);

    // This code is contributed by chinmoy1997pal.
</script>

Output:

11

Time Complexity: O(R – L)
Auxiliary Space: O(1)

Efficient Approach:
The key observation is that there are A – 1 numbers that are not divisible by A in the range [1, A – 1]. Similarly, there are A – 1 numbers not divisible by A in range [A + 1, 2 * A – 1], [2 * A + 1, 3 * A – 1] and so on. 
With the help of this observation, the N^th number which is not divisible by A will be: 

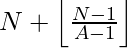
To find the value in the range [ L, R ], we need to shift the origin from ‘0’ to ‘L – 1’, thus we can say that the N^th number which is not divisible by A in the range will be : 

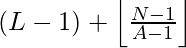
However there is an edge case, when the value of ( L – 1 ) + N + floor( ( N – 1 ) / ( A – 1 ) ) itself turns out to be multiple of a ‘A’, in that case Nth number will be : 

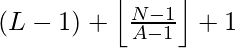
Below is the implementation of the above approach: 

C++

// C++ program for the above approach
#include <bits/stdc++.h>
using namespace std;

// Function to find Nth number
// not a multiple of A in range [L, R]
void countNo(int A, int N, int L, int R)
{

    // Calculate the Nth no
    int ans = L - 1 + N + floor((N - 1) /
                                (A - 1));

    // Check for the edge case
    if (ans % A == 0)
    {
        ans = ans + 1;
    }
    cout << ans << endl;
}

// Driver Code
int main()
{

    // Input parameters
    int A = 5, N = 10, L = 4, R = 20;

    // Function Call
    countNo(A, N, L, R);

    return 0;
}

// This code is contributed by avanitrachhadiya2155

Java

// Java program for the above approach
import java.io.*;

class GFG
{

  // Function to find Nth number
  // not a multiple of A in range [L, R]
  static void countNo(int A, int N, int L, int R)
  {

    // Calculate the Nth no
    int ans = L - 1 + N + (int)Math.floor((N - 1) / (A - 1));

    // Check for the edge case
    if (ans % A == 0)
    {
      ans = ans + 1;
    }
    System.out.println(ans);
  }

  // Driver Code
  public static void main (String[] args)
  {

    // Input parameters
    int A = 5, N = 10, L = 4, R = 20;

    // Function Call
    countNo(A, N, L, R);
  }
}

//  This code is contributed by rag2127

Python3

# Python3 program for the above approach
import math

# Function to find Nth number
# not a multiple of A in range [L, R]
def countNo (A, N, L, R):

    # Calculate the Nth no
    ans = L - 1 + N \
          + math.floor( ( N - 1 ) / ( A - 1 ) )

    # Check for the edge case
    if ans % A == 0:
        ans = ans + 1;
    print(ans)

# Input parameters
A, N, L, R = 5, 10, 4, 20

# Function Call
countNo(A, N, L, R)

C#

// C# program for the above approach
using System;
class GFG {

  // Function to find Nth number
  // not a multiple of A in range [L, R]
  static void countNo(int A, int N, int L, int R)
  {

    // Calculate the Nth no
    int ans = L - 1 + N + ((N - 1) / (A - 1));

    // Check for the edge case
    if (ans % A == 0)
    {
      ans = ans + 1;
    }
    Console.WriteLine(ans);
  }

  // Driver code
  static void Main()
  {

    // Input parameters
    int A = 5, N = 10, L = 4, R = 20;

    // Function Call
    countNo(A, N, L, R);
  }
}

// This code is contributed by divyesh072019.

Javascript

<script>

// Javascript program for
// the above approach

// Function to find Nth number
// not a multiple of A in range [L, R]
function countNo(A, N, L, R)
{

    // Calculate the Nth no
    var ans = L - 1 + N +
      Math.floor((N - 1) / (A - 1));

    // Check for the edge case
    if (ans % A == 0)
    {
        ans = ans + 1;
    }
    document.write(ans);
}

// Driver code

// Input parameters
var A = 5, N = 10, L = 4, R = 20;

// Function Call
countNo(A, N, L, R);

// This code is contributed by Khushboogoyal499

</script>

Output:

16

Time Complexity: O(1)
Auxiliary Space: O(1)