Eccentricity of Ellipse

Eccentricity of Ellipse: eccentricity is a measure that describes how much a conic section deviates from being circular. For any point on a conic section, eccentricity is defined as the ratio of the distance to a fixed point (focus) to the distance to a fixed line (directrix).

The eccentricity of an ellipse is the ratio of the distances from the centre of the ellipse to one of the foci and to one of the vertices of the ellipse. It is denoted using the letter, ‘e’ and is calculated as, e = c/a where a is the length of the semi-major axis and c is the distance from the centre to the foci.

In this article, we will learn about Ellipse, Eccentricity of Ellipse, Forumla for eccentricity of ellipse and others in detail.

What is an Ellipse?

An ellipse in mathematics is a special shape that we get when we cut through a cone at an angle. It looks like a stretched-out circle or an elongated oval. Imagine slicing through a cone sideways with a knife, not straight across but at a slant. The shape you get where the knife cuts through the cone is an ellipse.

Eccentricity of Ellipse

An ellipse is a shape formed by a point moving in a way that its distance from a fixed point and a fixed line always have a certain relationship. This is such that the ratio of the distance from the point to the distance from the line remains constant, and this constant ratio is always less than one. The constant ratio is denoted by ‘e’ and is known as the eccentricity of the ellipse.

The eccentricity of an ellipse is a measure of how stretched or elongated the ellipse is. It is a dimensionless parameter that ranges from 0 to 1. Suppose the distance of the focus from the centre of the ellipse is ‘c’ and the distance of the end of the ellipse from the centre is ‘a’, then eccentricity of the ellipse is found by the formula:

e = c/a

Eccentricity of Ellipse Formula

Eccentricity of an ellipse is defined as the ratio of the distance between the foci to the length of the major axis i.e the formula for eccentricity is given by:

e = c/a

where:

• e is Eccentricity
• c is Distance from Centre of each Focus
• a is Length of Semi-Major Axis

For any ellipse, let a be the length of its semi-major axis and b be the length of its semi-minor axis. In the coordinate system with the origin at the centre of ellipse and x-axis with the major axis, equation of ellipse is,

(x/a)² + (y/b)² = 1

with foci at coordinates (±c, 0) for

c = √ (a² – b²)

Formula of eccentricity of ellipse is:

e =√ (1 – b²/a²)

Eccentricity of Ellipse- Diagram

Diagram of Ellipse is shown in the image added below:

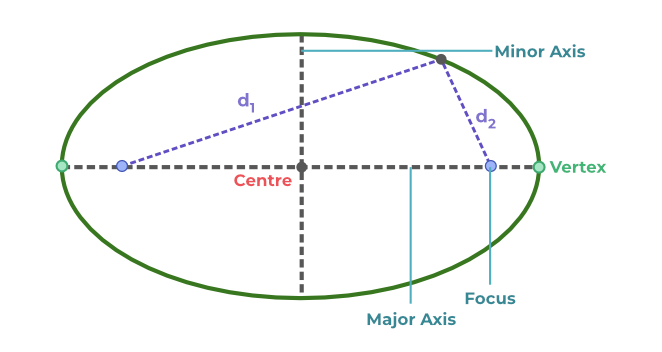
Diagram of Ellipse

Various results related to ellipse:

	x2/a2 + y2/b2 = 1, a > b	x2/a2 + y2/b2 = 1, a < b
Coordinates of Vertices	(a, 0) and (-a,0)	(0, b) and (0, -b)
Coordinates of Foci	(ae, 0) and (-ae,0)	(0, be) and (0, -be)
Length of Major Axis	2a	2b
Length of Minor Axis	2b	2a
Equation of Directrices	x = a/e and x = -a/e	y = b/e and y = -b/e
Length of Latus-Rectum	2b2/a	2a2/b
Eccentricity	e = √( 1 – b2/a2)	e = √( 1 – a2/b2)

Derivation of Eccentricity of Ellipse

Ratio of the distance between the foci of the ellipse to the length of the major axis is called eccentricity of ellipse.

Let’s consider an ellipse with a semi-major axis of length a and a semi-minor axis length b. The foci of the ellipse are located at a distance c from the center where c can be calculated using the relationship :

c = √(a²-b²)…(i)

And eccentricity is given by:

e = c/a…(ii)

Placing the value of c from (i) in (ii):

e = √(a²-b²) /a…(iii)

Squaring on both sides of (iii)

e² = {√(a²-b²) /a}²

e² = (a² – b²)/a²

Rearranging the above equation:

a²e² = a² – b²

a²e²+b² = a²

(a²e² + b²)/a² = 1

a²e²/a² + b²/a² = 1

e² + b²/a² = 1

e² = 1 – b²/a²

e = √(1 – b²/a²)…(iv)

e = √{ 1 – ( 2b/2a )²}

e = √( 1 – (minor axis/major axis)²)

Hence we have derived the equation (iv), known as the eccentricity formula for an ellipse.

People Also Read:

• Ellipse
• Eccentricity Formula of Circle, Parabola, Ellipse, Hyperbola
• Ellipse Formula
• How to find the Area of an Ellipse?

Eccentricity of Ellipse Examples

Example 1: For the following ellipse find the eccentricity of the ellipse:

1. 16x² + 25y² = 400
2. 3x² + 2y² = 6

Solution:

• 16x² + 25y² = 400

x²/25 + y²/16 = 1

This is of form (x/a)² + (y/b)² =1, where a = 5 and b = 4

e = √{ 1 – (b/a)²}

e = √( 1- 16/25)

e = 3/5

• 3x² + 2y² = 6

x²/2+ y²/3 = 1

This is of form (x/a)² + (y/b)² = 1, where a = 2 and b = 3

e = √{ 1 – (b/a)²}

e = √( 1- 2/3)

e = 1/√3

Example 2: If the latus rectum of an ellipse is equal to half of the minor axis, find its eccentricity.

Solution:

Latus-Rectum = Minor axis/2

2b²/a = 2b/2

2b = a

4b² = a²

4a²( 1-e² ) = a²

4 – 4e² = 1

4e² = 3

e = √3/2

Example 3: If the eccentricity of an ellipse is 5/8 and the distance between its foci is 10 , then find the latusrectum of the ellipse.

Solution:

Let the equation of required ellipse be x²/a² + y²/b² = 1 and its eccentricity is ‘e’

Given,

• e = 5/8
• 2ae = 10

e = 5/8 and ae = 5

e = 5/8 and a= 8

∴ b² = a²( 1 – e²)

b² = 64 (1- 25/64)

b² = 39

Hence, length of latusrectum = 2b²/a

L = 2 × 39 /8

L = 39 /4

Example 4: Find the distance between the directrices the ellipse x²/36 + y²/20 = 1

Solution:

Comparing the given equation with x²/a² + y²/b² = 1, we obtain a² = 36 and b² =20

Let e be the eccentricity of the ellipse. Then,

b² = a² (1 – e²)

20 = 36 (1 – e²)

36 e² = 16

e = 2/3

Distance between the directrices = 2a /e = 2×6/(2/3) = 18

Example 5: Find the equation of the ellipse whose axes are along the coordinate axes ,vertices are (±5,0) and foci at (±4,0) .

Solution:

x²/a² + y²/b² = 1

As we know that coordinates of its vertices and foci are (±a, 0) and (±ae, 0) respectively

a= 5 and ae = 4

e = 4/5

b² = a² (1- e²)

b² = 25 (1 – 16/25)

b² = 9

Hence, the equation is x²/25 + y²/9 = 1

Practice Problems on Eccentricity of Ellipse

1. Given an ellipse with a semi-major axis a = 5 units and a semi-minor axis b = 3 units, calculate the eccentricity of the ellipse.

2. An ellipse has an eccentricity of 0.8 and a semi-major axis of 10 units. Find the length of the semi-minor axis b

3. The distance between the foci of an ellipse is 8 units, and the length of the major axis is 10 units. Determine the eccentricity of the ellipse.

4. An ellipse has an eccentricity that is 1/2​ of its semi-major axis length. If the semi-minor axis is 6 units, find the eccentricity and the length of the semi-major axis.

FAQs on Eccentricity of Ellipse

What is eccentricity?

Eccentricity is the measure of how much the deviation of the curve has occurred from the circularity of the given shape.

What is Eccentricity of Ellipse?

Eccentricity of an ellipse is the ratio of the distances from the centre of the ellipse to one of the foci and to one of the vertices of the ellipse, it is denoted by ‘e’.

What does an eccentricity 0 mean for an ellipse?

An eccentricity of 0 indicates a circle. In this case ,the foci coincide at the center of the ellipse, and the shape is perfectly round.

Can the eccentricity of an ellipse be greater than 1?

No, eccentricity is always a value between 0 and 1 for an ellipse.

How does changing the eccentricity affect the focus of an ellipse?

As eccentricity increases ,the distance between the foci increases .Conversely ,as eccentricity decreases ,the foci move closer together.

Is Eccentricity of Ellipse greater or less than 1?

Eccentricity of an ellipse is always less than 1.

What is an eccentricity formula?

Eccentricity is calculated using the formula, Eccentricity = (Distance from Focus)/(Distance from Directrix)

What is eccentricity of ellipse formula in terms of a and b?

Formula for eccentricity is: e = √(1-b²/a²)