What is the formula of tan(A – B)?
Last Updated :
17 Aug, 2021
Trigonometry has always been part of advanced mathematics which is applicable in almost all fields whether it be architecture, geography, physics, astronomy, investigation, or daily life application. Although trigonometry is not used directly in real life it is applied to most of the appliances which come in daily use. It is used for programming, computing, navigating, medical imaging, measuring the heights of buildings and mountains, etc.
Trigonometry is a branch of standardized mathematics that deals with the relationship between lengths, heights, and angles.
Trigonometry includes its own trigonometric functions, expressions, angles, and their values which are used to solve trigonometric problems.
Addition Formulas of Trigonometry
Generally, there are six addition formulae in trigonometry. These all six formulae are interconnected as one is used to derive the other. These formulae are applicable for solving trigonometric problems. The first two addition formulae are of sine, the second two are related to cosine, and the third pair is of the tangent which is derived from four previous formulae.
Look into all the six formulae in the below given table:
| Expression | Derived Formula |
|---|
| sin (A + B) | sinAcosB + cosAsinB |
| sin(A – B) | sinAcosB – cosAsinB |
| cos(A + B) | cosAcosB – sinAsinB |
| cos(A – B) | cosAcosB + sinAsinB |
| tan(A + B) | 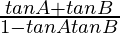 |
| tan(A – B) | 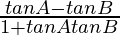 |
What is the formula of tan(A – B)?
Tan(A – B) is the sixth formula among the six addition formulae of trigonometry used to conduct trigonometric calculations. The formula is derived with the help of the previous four additional formulae of sine and cosine.
The result for tan(A – B) is derived in the terms of sines and cosines. The trigonometric formula is given by:
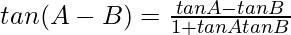
Now, let’s take a look into how the formula is derived from previous addition formulas of sines and cosines.
Derivation of the Formula
The formula can be derived from previous addition formulae as we know that
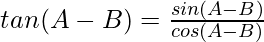
By putting in the formulae of sin(A-B) and cos(A-B), the result is

Now, dividing every term of right hand side with cosAcosB we get,
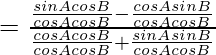
Canceling out the common factors we get,
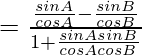
As we have tan = sin/cos
=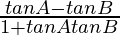
The same method of derivation is used to derive tan(A + B) from the other formulae.
Here, are some trigonometric problems using the tan(A – B) formula.
Sample Questions
Question 1: Find an expression for tan 15°?
Answer:
From the question we can assume the expression 15° = 60° – 45°.
The value of tan60°=√3 and tan45° = 1
Now, following the expression
tan15° = tan(60° – 45°)
=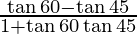
=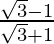
Multiplying both numerator and denominator by √3-1
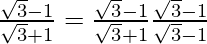
=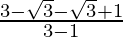
= 2 – √3
Question 2: If the tanA = 1/2 and tanB = 1/3, find the value for expression tan(A + B).
Answer:
As per the formula
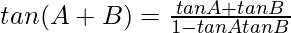
putting in the given values of tanA and tanB,
=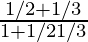

Therefore, tan(A + B) = 1
which is tan (A + B)= tan 45°
Question 3: If tan A = 5 and tan B = 2, find the value for tan(A – B).
Answer:
As per the formula
tan(A + B) = 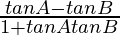
= 5 – 2/1 + 5×2
= 3/11
Therefore, the value of tan(A – B) is 3/11.
Share your thoughts in the comments
Please Login to comment...