Root 2 Value | How to Find the Square Root of 2?
Last Updated :
31 Jan, 2024
Square root of 2 is represented as √2 or 2½. When multiplied by itself, √2 will result in the number 2. The value of the square root of 2 is 1.414.
Let’s learn the methods to find the square root of 2 value in detail.
Root 2 Value
The numerical value of square root 2 up to 50 decimal places is as follows:
√2 = 1.41421356237309504880168872420969807856967187537694…
The value of the square root of 2 is widely accepted as 1.414. The reason for this is that it contains an infinite number of decimals and to make mathematical computations easy, we use only 3 digits after decimal places.
Sometimes 99/70 is also used as a value for Square Root of 2.
How to Find the Square Root of 2?
The square root of a number is the value that when multiplied by itself, results in the number taken as input. To compute the square root, we first need to check if the number is a perfect square. Perfect Squares are the numbers, the roots of which are whole numbers.
For example, 4, 9, 25, 36, 49, etc. It is easier to compute the square root of a perfect square number as compared to a non-perfect square number.
To compute the root of a non-perfect number, we usually apply one of these two methods:
- Long division Method
- Estimation Method (Guess and Check Method)
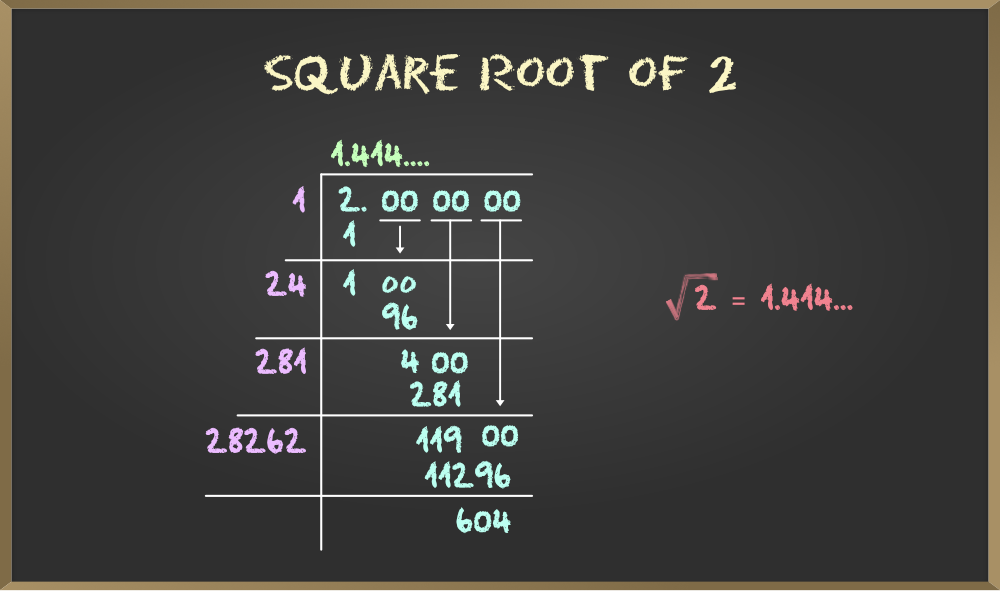
Square Root 2 Value by Long Division Method
It is always easier to compute the square root of perfect squares. But to compute the square root of a non-perfect square, we need to perform the long division method.
To compute the square root of 2, we need to follow the steps given below:
Step 1: Write 2 as 2.000000 to make it easier to divide
Step 2: Now look for the perfect square less than 2 i.e. 1 and divide the number with it.
Step 3: Now the quotient and the remainder are 1. We will place a decimal in the quotient and bring down the pair of zeroes for further division.
Step 4: Now add the quotient with the existing divisor, this will become the digit at the tens place for our next divisor.
Step 5: For the unit’s place, we need to find a value that can be placed at the unit place of both quotient and the divisor such that the new divisor when multiplied with the unit digit of the quotient results in the highest number less than the remainder.
Now. bring down the next pair of zeros and repeat the steps 4th and 5th. This can be done for infinite steps as the exact value of the square root of 2 goes up to infinite decimal places.
We can compute the result up to 4 decimal places as that can be used for approx. value of the square root.
Read More On
Square Root 2 Value by Estimation Method
In the Estimation Method for calculating the square root of 2 involves, we make an initial guess and then refine it to get closer to the actual value.
Let’s discuss how it works.
- Begin with a reasonable guess for the square root of 2. A common starting point is 1, because 12 = 1, which is close to 2.
- Use the following formula to improve your guess:
New Estimate= (Old Estimate + 2 ∕Old Estimate) ∕ 2
3. Repeat the refining step using the new estimate as the old estimate in the next iteration.
Example:
- Start with 1 as the initial guess.
- Apply the formula: New Estimate = (1 + 2/1) / 2 = 1.5.
- Use 1.5 as the new estimate and apply the formula again: New Estimate = (1.5 + 2/1.5) / 2 ≈ 1.4167.
- Continue this process until the estimate squares to a value as close to 2 as needed for your precision requirements.
With each iteration, our estimate will get closer to the actual square root of 2.
Is Square Root of 2 Rational or Irrational?
Let’s find out whether the root 2 value is rational or irrational.
Rational numbers are numbers that can be expressed as a fraction, where the denominator is not zero. They include integers, fractions, and finite or repeating decimals.
On the other hand, irrational numbers cannot be expressed as a simple fraction. Their decimal expansions are non-terminating and non-repeating.
The value of √2 is 1.414… value can’t be determined exactly because it can not be represented as a fraction i.e. in the form of a/b where a and b are integers and b can’t be zero.
So, the square root of 2 is an an irrational number.
Read More On,
Root 2 Value Calculation- FAQs
1. What is the Value of Square Root of 2?
The value of the square root of 2, is approximately 1.41421.
2. Why is Square Root of 2 an Irrational Number?
The square root of 2 is considered an irrational number because it cannot be expressed as a simple fraction or ratio of two integers.
4. What is root 2 symbol?
Root 2, is represented by the square root symbol √ and written as √2.
5. Is the number 2 a Perfect Square?
2 is a prime number this implies that the square root of 2 cannot be expressed as a product of two equal integers. Therefore, 2 is not a perfect square.
6. What is root 3 value ?
√3 is an irrational number so its exact value is never determined. But it can be approximated to √3 = 1.732(approx)
7. Does a Square Root Have Two Values?
Yes, a square root can have two values, one positive and one negative. This is because squaring either a positive or a negative number results in a positive value.
Share your thoughts in the comments
Please Login to comment...