Python | Percentage increase in hemisphere volume if radius is increased
Last Updated :
20 Feb, 2023
Given that the radius of a hemisphere is increased by a fixed percentage so, the target is to calculate the percentage increase in the volume of the hemisphere.
Examples:
Input :
20
Output :
72.8 %
Input :
70
Output :
391.3 %
Approach:
Let, the radius of the hemisphere = 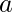
Given percentage increase = 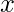
Volume before increase = 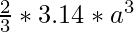
New radius after increase = 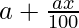
So, new volume = 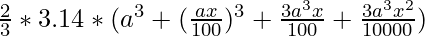
Change in volume = 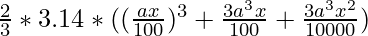
Percentage increase in volume = 
Below is the Python code implementation of the above mentioned approach.
Python3
def newvol(x):
print('percentage increase in the volume of the hemisphere is ', pow(x, 3) / 10000 + 3 * x
+ (3 * pow(x, 2)) / 100, '%')
x = 10.0
newvol(x)
|
Output :
percentage increase in the volume of the hemisphere is 33.1 %
Time Complexity: O(log x) because pow function would take logarithmic time
Auxiliary Space: O(1)
Like Article
Suggest improvement
Share your thoughts in the comments
Please Login to comment...