Polytime Manyone reduction: Clique to E-TM

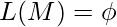
Prerequisite – Clique is NP A Polynomial-time reduction is a method for solving one problem using another. E-TM = {<M> : M is a TM and } CLIQUE = {<G, k> : graph G has a clique with at least k vertices}. Note – Since CLIQUE is NP => some NDTM_CLIQUE accepts CLIQUE.

Reduction(<G, k>)
    construct the following machine M
    M(x):
        1. Run NDTMCLIQUE on input <G, k>.
    2. If NDTMCLIQUE accepts; M rejects x.
    3. Else; M accepts x.
    return <M>

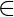
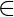
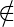
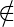
We convert the instance <G, k> CLIQUE to a TM <M> E-TM. And <G, k> CLIQUE to a TM <M> E-TM. Correctness:

i. <G, k> 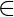 CLIQUE => M rejects all input x => L(M)=  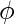=> <M>  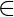E-TM.
ii. <G, k> 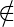 CLIQUE => M accepts all input x => L(M) 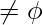=> <M>  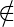E-TM.

Hence, reduction is correct. Polytime – The reduction involves describing the construction of a new Turing machine M for input <G, k>. We don’t run the machine on the input. Hence, the reduction is polytime.