Parallelogram is a two-dimensional geometrical shape whose opposite sides are equal in length and parallel. The opposite angles of a parallelogram are equal in measure.
In this article, we will learn about the definition of a parallelogram, its properties, types, theorem and formulas on the area and perimeter of a parallelogram in detail.
Parallelogram Definition
A parallelogram is defined as a quadrilateral whose two pairs of opposite sides are parallel and equal. The opposite angles of a parallelogram are also equal in measure.
Below is the diagram of a parallelogram ABCD having adjacent sides ‘a’ and ‘b’ and height ‘h’.
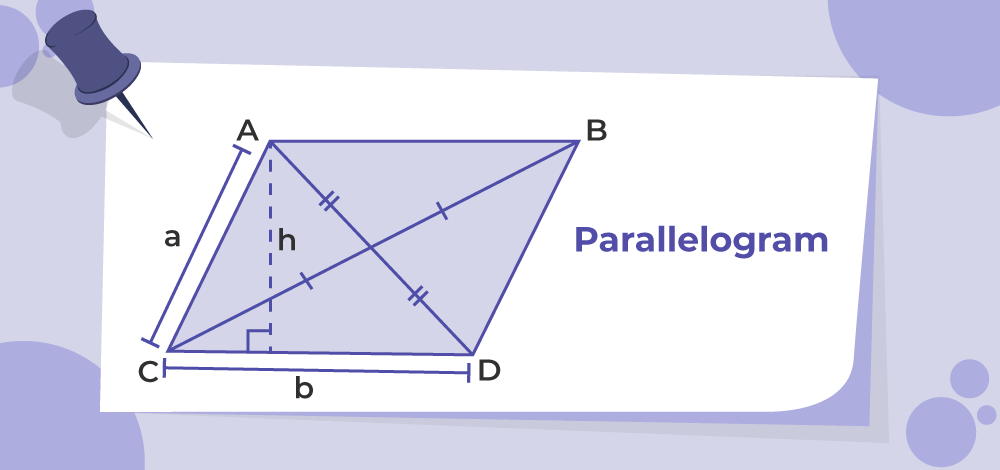
Diagram of a parallelogram
Also Read,
Shape of Parallelogram
A parallelogram is a two-dimensional closed shape with four sides, i.e. it is a quadrilateral.
Note- All rectangles and squares can be considered parallelograms but the opposite is not true, i.e. all parallelograms are not considered squares or rectangles.
Angles of Parallelogram
Parallelogram is a quadrilateral i.e. a polygon with four sides and four angles and the opposite pair of angles are equal in the parallelogram. i.e. in a parallelogram ABCD ∠A is equal to ∠C and ∠B is equal to ∠D.
The sum of all the angles in the quadrilateral is 360°. As a parallelogram is a quadrilateral so sum of all the angles of the parallelogram ABCD equals 360°. Now,
∠A + ∠B + ∠C + ∠D = 360°
in parallelogram ∠A = ∠C and ∠B = ∠D
Thus,
∠A + ∠B + ∠A + ∠B = 360°
2(∠A + ∠B) = 360°
∠A + ∠B = 180°
Similarly, ∠C + ∠D = 180°
Thus, adjacent angles are supplementry in a parallelogram.
Properties of Parallelogram
A parallelogram has some special properties of its own. The properties of a parallelogram are as follows :
- The opposite sides of a parallelogram are parallel and equal.
- The opposite angles of a parallelogram are congruent.
- If said, one of the angles of a parallelogram is 90°. Then all the angles are 90°, and it becomes a rectangle.
- The diagonals of the parallelogram bisect each other.
- The consecutive angles of a parallelogram are supplementary.
Also Check: Properties of Parallelogram
Types of Parallelogram
There are three types of parallelogram, based on the properties of parallelogram. They are :
Now let’s discuss all these three types of parallelogram in some detail.
Rectangle
A rectangle is a parallelogram with two pairs of equal and parallel opposite sides, along with four right angles.
Observe the rectangle ABCD and associate it with the following properties,
- Two pairs of parallel sides. Here AB || DC and AD || BC
- Four right angles ∠A = ∠B = ∠C = ∠D = 90°.
- The opposite sides are the same length, where AB = DC and AD = BC.
- Two equal diagonals where AC = BD.
- Diagonals that bisect each other.
To learn about rectangles in detail, head over to Rectangle.

Diagram of a Rectangle
Square
A square is a parallelogram with four equal sides and four equal angles.
Observe the square ACDB and associate it with the following properties:
- Four equal sides are AB = BC = CD = DA.
- Right angles are ∠A = ∠B = ∠C = ∠D = 90°.
- There are two pairs of parallel sides. Here AB || DC and AD || BC.
- Two identical diagonals where AD = BC.
- Diagonals are perpendicular and bisect each other; AD is perpendicular to BC.
To learn about squares in detail, head over to Square.
.png)
Diagram of a Square
Rhombus
A parallelogram with four equal sides and equal opposite angles is called a rhombus. Consider the diamond ABCD and assign it the following attributes,
- In the given figure, the four equal sides are AB = CD = BC = AD.
- The two pairs of parallel sides are AB || CD and BC || AD.
- The equal opposite angles are ∠A = ∠B and ∠C = ∠D.
- Here, the diagonals (AC and BD) are perpendicular to each other and bisect at right angles.
.png)
Diagram of a Rhombus
To learn about rhombus in detail, head over to Rhombus.
All 2D shapes have two basic formulas for area and perimeter. Parallelogram is a basic 2-dimensional figure which is widely used in mathematics.
All the formulas on parallelogram can be subdivided into two parts:
- Area of Parallelogram
- Perimeter of Parallelogram
Let us discuss these two formulas of a parallelogram in detail.
Area of Parallelogram
The area of a parallelogram is the space covered between the four sides of the parallelogram.
It can be calculated by knowing the length of the base and the height of the parallelogram and measuring it in square units such as cm2, m2, or inch2. Note the following parallelogram representing the base and height.
.png)
Area of a Parallelogram
Consider a parallelogram ABCD with a base (b) and a height (h).
Then, the area of a parallelogram is calculated by the formula:
Parallelogram area = base (b) × height (h)
Also Check- Area of a Parallelogram
Area of Parallelogram without Height
When the height of the parallelogram is not known, the area of the parallelogram can still be found, provided the angle is known to us.
The formula for the area of a parallelogram without height is given as:
Parallelogram area = ab Sinθ
Where a and b are the sides of the parallelogram and θ is the angle between them.
Also Check- Height of a parallelogram
Perimeter of Parallelogram
Perimeter of a parallelogram is the length of its boundary, so it is equal to the sum of all sides.
In a parallelogram, the opposite sides are equal. Let’s say the sides are a and b. Then, the perimeter (P) of the parallelogram with edges is in units of P = 2 (a + b).
Perimeter of Parallelogram = 2 (a + b)
Do Check- Perimeter of a Parallelogram
Parallelogram Theorem
Let’s understand the theorem on parallelogram and how to prove it.
Theorem: Parallelograms on the same base and between the same parallels are equal in area.
To Prove: Area of parallelogram ABCD = Area of parallelogram ABEF
Proof: Let’s assume two parallelograms ABCD and ABEF with the same base DC and between the same parallel lines AB and FC
In the figure given below, the two parallelograms, ABCD and ABEF, lie between the same parallel lines and have the same base. Area ABDE is common between both parallelograms.
Taking a closer look at the two triangles, △BCD and △AEF might be congruent.
.png)
Parallelogram Theorem
BC = AE (Opposite sides of a parallelogram),
∠BCD = ∠AEF (These are corresponding angles because BC || AE and CE are the transversal).
∠BDC = ∠AFE (These are corresponding angles because BD || AF and FD are the transversals).
Thus, by the ASA criterion of congruent triangles. These two triangles are congruent, and they must have equal areas.
area(BCD) = area(AEF)
area(BCD) + area(ABDE) = area(AEF) + area(ABDE)
area(ABCD) = area(ABEF)
Hence, parallelograms lying between the same parallel lines and having a common base have equal areas.
Difference Between Parallelogram and Rectangle
Rectangle and parallelogram are both quadrilaterals. The rectangle is a parallelogram as it has all the properties of a parallelogram and more but a parallelogram is not always a rectangle.
Here we have tabulated some of the basic differences between the properties of a parallelogram and those of a rectangle.
|
Properties
|
Parallelogram
|
Rectangle
|
|
Parallelogram vs Rectangle
|
| Sides |
The opposite sides of a parallelogram are equal. |
The opposite sides of a rectangle are equal. |
| Diagonals |
The diagonals of a parallelogram bisect each other, but the diagonals are not equal. |
The diagonals of a rectangle bisect each other, and the diagonals are equal to each other as well. |
| Angles |
The opposite angles of a parallelogram are equal, and the adjacent angles are supplementary. |
All the angles of a rectangle are equal to each other and equal to 90°. |
Do Check,
Solved Examples on Parallelogram
We have solved some select questions on parallelogram down below. They will help you better improve your understanding of the concepts in this chapter.
Example 1: Find the length of the other side of a parallelogram with a base of 12 cm and a perimeter of 60 cm.
Solution:
Given perimeter of a parallelogram = 60cm.
Base length of given parallelogram = 12 cm.
P = 2 (a + b) units
Where b = 12cm and P = 40cm.
60 = 2 (a + 12)
60 = 2a + 24
2a = 60-24
2a = 36
a = 18cm
Therefore, the length of the other side of the parallelogram is 18 cm.
Example 2: Find the perimeter of a parallelogram with the base and side lengths of 15cm and 5cm, respectively.
Solution:
Base length of given parallelogram = 15 cm
Side length of given parallelogram = 5 cm
Perimeter of a parallelogram is given by,
P = 2(a + b) units.
Putting the values, we get
P = 2(15 + 5)
P = 2(20)
P = 40 cm
Therefore, the perimeter of a parallelogram will be 40 cm.
Example 3: The angle between two sides of a parallelogram is 90°. If the lengths of two parallel sides are 5 cm and 4 cm, respectively, find the area.

Solution:
If one angle of the parallelogram is 90°. Then, the rest of the angles are also 90°. Therefore, the parallelogram becomes a rectangle. The area of the rectangle is length times breadth.
Area of parallelogram = 5 × 4
Area of parallelogram = 20cm2
Example 4: Find the area of a parallelogram when the diagonals are given as 8 cm, and 10 cm, the angle between the diagonals is 60°.
Solution:
In order to find the area of the parallelogram, the base and height should be known, lets’s first find the base of the parallelogram, applying the law of cosines,
b2 = 42 + 52 – 2(5)(4)cos(120°)
b2 = 16 + 25 – 40(0.8)
b2 = 9
b = 3cm
Finding the height of the parallelogram,
.png)
4/sinθ = b/sin120
4/sinθ = 3/-0.58
sinθ = -0.773
θ = 50°
Now, to find the height,
Sinθ = h/10
0.76 = h/10
h = 7.6cm
Area of the parallelogram = 1/2 × 3 × 7.6
= 11.4 cm2
Example 5: Prove that a parallelogram circumscribing a circle is a rhombus.

Solution:
Given:
- Parallelogram ABCD
- Circle PQRS
To prove: ABCD is a rhombus.
Proof:
We know that the tangents drawn from an exterior point to a circle are equal to each other. Therefore:
AP = AS ⇢ (1)
BP = BQ ⇢ (2)
DS = DR ⇢ (3)
CR = CQ ⇢ (4)
Adding the LHS and RHS of equations 1, 2, 3, and 4:
AP + BP + DS + CR = AS + BQ + DR + CQ
AB + DR + CR = AS + DS + BC
AB + CD = AD + BC
Since the opposite angles of a parallelogram are equal:
2AB = 2BC
AB = BC, and similarly, CD = AD.
Therefore: AB = CD = BC = AD.
Since all the sides are equal, ABCD is a rhombus.
Real-Life Examples of a Parallelogram
Various examples of parallelogram as observed in our daily life includes:
- We came across various things in our daily life which resembles a parallelogram such as the computer screen, books, buildings, and tiles all are considered to be in a parallelogram shape.
- The parallelogram is the most common shape which we encounter daily.
- Rectangle and square both can be considered a parallelogram and are easily seen in our daily life.
FAQs on Introduction to Parallelogram
What is a parallelogram?
A parallelogram is a quadrilateral whose opposite sides are equal in length and parallel to each other. The opposite angles of a parallelogram are equal in measure.
What is the area of a Parallelogram?
The space occupied inside the boundary of the triangle is termed the area of the parallelogram. It can be calculated using the formula,
Area of Parallelogram = Base (b) × Height (h)
What is the Perimeter of a Parallelogram?
The length of all the boundaries of the triangle is termed the perimeter of the parallelogram. It can be calculated using the formula,
Perimeter of Parallelogram = 2(l + b)
Does a parallelogram have equal diagonals?
No, the diagonals of a parallelogram are not equal. However, the diagonals of a parallelogram bisect each other.
How many lines of symmetry parallelogram have?
In general, a parallelogram has 0 lines of symmetry. But in special cases of parallelogram line of symmetry is present. Lines of symmetry of special types of parallelograms are,
| Square |
4 |
| Rhombus |
2 |
| Rectangle |
2 |
Does a parallelogram have equal sides?
Yes, all parallelograms have equal pairs of opposite sides but not all sides are equal in a parallelogram.
Is a rhombus a parallelogram?
Yes, a rhombus is a parallelogram. A rhombus has all the properties of the parallelogram and more.
How is a parallelogram different from a quadrilateral?
All parallelograms are quadrilaterals, but not all quadrilaterals are necessarily parallelograms. For example, a trapezoid is a quadrilateral, not a parallelogram. For a quadrilateral to be a parallelogram, all of its opposite sides must be parallel and equal.
Is a square a parallelogram?
Yes, a square is a parallelogram as it has all the properties of a parallelogram and more. However, a parallelogram is not always a square.
Is a rectangle a parallelogram?
Yes, a rectangle is a parallelogram. Rectangle is a parallelogram as it has all the properties of a parallelogram and more. However, a parallelogram is not always a rectangle.
Like Article
Suggest improvement
Share your thoughts in the comments
Please Login to comment...