Nth root of a number using log
Last Updated :
18 Sep, 2022
Given two integers N and K, the task is to find the Nth root of the K.
Examples:
Input: N = 3, K = 8
Output: 2.00
Explanation:
Cube root of 8 is 2. i.e. 23 = 8
Input: N = 2, K = 16
Output: 4.00
Explanation:
Square root of 16 is 4, i.e. 42 = 16
Approach: The idea is to use logarithmic function to find the Nth root of K.
Let D be our Nth root of the K,
Then, 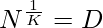
Apply logK on both the sides –
=> 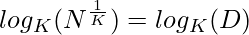
=> 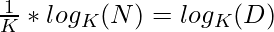
=> 
Below is the implementation of the above approach:
C++
#include <bits/stdc++.h>
double kthRoot(double n, int k)
{
return pow(k,
(1.0 / k)
* (log(n)
/ log(k)));
}
int main(void)
{
double n = 81;
int k = 4;
printf("%lf ", kthRoot(n, k));
return 0;
}
|
Java
import java.util.*;
class GFG {
static double kthRoot(double n, int k)
{
return Math.pow(k, ((1.0 / k) *
(Math.log(n) /
Math.log(k))));
}
public static void main(String args[])
{
double n = 81;
int k = 4;
System.out.printf("%.6f", kthRoot(n, k));
}
}
|
Python3
import numpy as np
def kthRoot(n, k):
return pow(k, ((1.0 / k) *
(np.log(n) /
np.log(k))))
n = 81
k = 4
print("%.6f" % kthRoot(n, k))
|
C#
using System;
class GFG {
static double kthRoot(double n, int k)
{
return Math.Pow(k, ((1.0 / k) *
(Math.Log(n) /
Math.Log(k))));
}
public static void Main(String []args)
{
double n = 81;
int k = 4;
Console.Write("{0:F6}", kthRoot(n, k));
}
}
|
Javascript
<script>
function kthRoot(n, k)
{
return Math.pow(k, ((1.0 / k) *
(Math.log(n) /
Math.log(k))));
}
var n = 81;
var k = 4;
var x = kthRoot(n, k)
document.write(x.toFixed(6));
</script>
|
Time Complexity: O(1)
Auxiliary Space: O(1)
Like Article
Suggest improvement
Share your thoughts in the comments
Please Login to comment...