Law of Action and Reaction is the other name for Newton’s Third Law of Motion. There are three basic laws given by famous English Physicist Issac Newton that are helpful in defining the motion of any object in an inertial frame of reference. The third law of Netwon is also called the Law of Action and Reaction. As its name suggests it explains, “For any action to an object we have an equal and opposite reaction.”
This could be explained by the example that suppose we have a ball that strikes a wall with a force of F1 (action force), and the wall applies a force of F2 (reaction force) then the action force is always equal to the reaction force, i.e. F1 = F2
In this article, we will learn about Newton’s Third Law of Motion (Law of Action and Reaction), its Examples, and others in detail.
Law of Action and Reaction (Newton’s Third Law of Motion)
Law of Action and Reaction also called Newton’s Third Law of Motion states that,
“For every action there is always an equal and opposite reaction.”
Action and reaction forces are applied on different bodies at the same instance of time. Due to this action and reaction pair, the system is always in equilibrium. The image added below shows an action and reaction pair. In the image, a gun fires a bullet and the gun applies a force on the bullet and the bullet also applies a force on the gun which is called recoil force.

According to Law of Action and Reaction, when two bodies engage, they apply forces to each other that are equal in magnitude and opposite in direction. This law is useful in studying the motion of objects that are in static equilibrium or the objects that are in linear motion.
This can be explained by the example as, a book resting on a table exerts a force on the table and the table exerts a normal force on the book, the action force by the book and the reaction force by the book both are equal. Mathematically, law of action and reaction is shown below:

Here, F12 is the force applied by body 1 on body 2 and F21 is the force applied by body 2 on body 1. The minus sign indicates that the two forces are applied in a mutually opposite direction.
Forces always occur in pairs. If two bodies A and B exist within a system, then the force of A on B and force of B and A become internal forces of the system, and they cancel each other since they are opposite in direction and equal. As a result, the system maintains its equilibrium.
Action and Reaction Pair in Nature
There are various examples around us that supports the law of action and reaction and they are added below,
Swimming of a Fish:
A fish uses its fins to push water backward to simulate its motion. In turn, the water exerts an equal reacting force by pushing the fish forward, propelling the fish through the water.
The magnitude of the force exerted by the fish on the water is equivalent to the size of the force on the fish. The direction of the force on the water which is backward in nature is opposite the direction of the forward force on the fish.
Flying of Birds in Air:
The flying motion of birds is governed by the birds pushing down on the air with their wings, while the air in return pushes their wings up and gives them lift. The direction of the force on the air which is backward in nature is opposite the direction of the forward force on the birds. Action-reaction force pairs make it possible for birds to fly.
Proof that Action and Reaction are Equal and Opposite
To understand the concept of the action and reaction forces, let us consider a system of two spring balances A and B connected together.
The spring balance B is fixed to any rigid support. A force is then applied in the loose and free end, by pulling the spring balance A. As an effect of the application of force, both the spring balances show the same readings.
This concludes that both the spring balances witness equal magnitude forces. It also shows that the force exerted by both the spring balances, A on B is equal but opposite in direction to the force exerted by spring balance B on A.
Here, he force exerted on the acting body (the spring balance A on B) is termed as action, and the force exerted by the reacting body (spring balance B on A) can be termed as reaction.

Application of Action and Reaction Pair
There are various applications and examples that shows the action and reaction pairs are important. Some of them are,
Recoil of A Gun
When a bullet is fired from a gun, it exerts a forward force (action) on the bullet and the bullet exerts an equal and opposite force on the gun (reaction), and the gun recoils. The reaction force is experienced on the hand of the firing person.

Sailor and Boat
When a sailor jumps out of a boat, he pushed the boat backward by exerting a backward force of the boat (action) and he jumps forward. Thereby, the boat exerts an equal and opposite force on the sailor called reaction.

Flying of Hot Air Ballon
When we release an air-filled balloon, the force of the air (gases) coming out of the balloon is the action, which exerts an equal and opposite force on the balloon called reaction by moving upward.

Rocket Propulsion
When a rocket is fired, the force of the burning gases coming out (action) exerts an equal and opposite force on the rocket (reaction) and it moves upward.
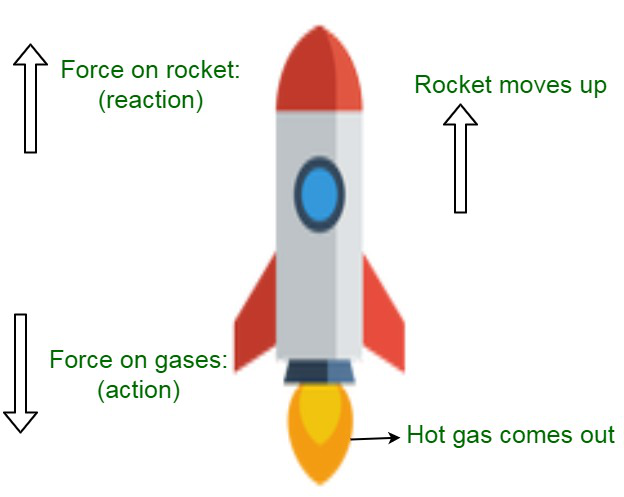
Different kinds of fuels are burned in the rocket’s engine, producing hot gases. These gases push against the inside tube of the rocket. After igniting and burning through the inside tube, the gases escape from the bottom of the rocket. As these gases flow downward, the rocket rises in the sky towards the upward direction. Therefore, as the gases and rocket move in the opposite direction w.r.t each other. The reaction of a rocket is the application of the third law of motion.
Mathematical Interpretation of Action and Reaction Pair
Action and reaction pair states that every action has equal and opposite reaction. For a system of two bodies, A and B, let us assume FAB is a force of body A acting on B and FBA is force by B on body A. This is shown in the image added below,

The mathematical expression w.r.t the forces is given by,
FAB = – FBA
where,
- FAB is an action on B
- FBA is reaction of body B on A
Negative sign indicates that force acting on body A is in opposite direction to the force which is acting on body B.
Derivation of Law of Action and Reaction
Derivation of Law of Action and Reaction from Newton’s Second Law of Motion is added below in this article,
Let us assume an isolated system with no external forces acting upon them, consisting of two massive bodies A & B mutually interacting with each other. Now, Let us consider both bodies to be in effect of a force under the influence of each other, that is, FAB, to be the force exerted on body B by body A and FBA be the force exerted by body B on A.
Due to these forces, FAB and FBA, let us assume dp1/dt and dp2/dt to be the rate of the change of momentum in the effect of these bodies respectively. Then,
FBA = dp1/dt and FAB = dp2/dt
Adding these equations as:
FBA + FAB = dp1/dt + dp2/dt
= d(p1 + p2) / dt
Since, no external force acts on the system, therefore:
d(p1 + p2) / dt = 0
or
FBA + FAB = 0
FBA = -FAB
The above equation represents Newton’s third law of motion (i.e, for every action there, is an equal and opposite reaction).
Read, More
Problems on Law and Action and Reaction
Problem 1: A car with a mass of 1250 Kg traveling by an acceleration of 10 m/s2 hits a bike. What force does the car experience?
Solution:
According to Newton’ second law,
The force on the bike, F = m × a
= 1250 kg × 10 m/s2
= 12500 N
Now according to Newton’s third law, for every action, there is an equal and opposite reaction.
Thus, the car experiences a force of 10 N.
Problem 2: A Dog of mass 10 kg jumps on a table of mass 60 kg. As the Dog walks around on the table, what is the average force that the table applies to the Dog? Use g = 10 m/s2.
Solution:
The force that the dog applies to the table is its weight. As per Newton’s third law, the table also applies a force to the dog of the same magnitude.
The force on the dog from the table is:
Fs = FN = ma = 10 kg × 10 m/s2 = 100 N
Problem 3: A boy is riding his scooter and pushes off the ground with his foot. Thus this causes him to accelerate at a rate of 8 m/s2. Boy’s weight is 600 N. What is the strength of his push off the ground? Use g = 10 m/s2.
Solution:
Boy’s weight, F is 600 N.
The formula to calculate the force on an object is,
F = ma
where m is the mass and a is the acceleration.
or
m = F / a
= 600 N / 10 m/s2
= 60 kg
Boy accelerates at 8 m/s2. so, he is pushed by a force of
F = ma = 60 kg × 8 m/s2 = 480 N
Problem 4: Two bodies apply forces to each other. The force on one of the bodies as a function of time in the x-direction is kt + b, where k and b are constants. What’s the force as a function of time in the x-direction on the other body? Consider no other forces are present besides the forces the bodies apply to each other.
Solution:
According to Newton’s third law, Every action has an equal and opposite reaction.
Thus, the force has an equal and opposite force on the other body.
Mathematically, this just means to negative the force. Therefore, the force as a function of time in the x-direction on the other body is -ky – b.
FAQs on Law of Action and Reaction
1. What is Law of Action and Reaction?
Law of Action and Reaction also called Newton’s Third Law of Motion states that,
“Every Action has it equal and Opposite Reaction”
2. What is Law of Interia?
Law of Inertia also called the Newton’s First Law of Motion. This law states that,
“An object in the state of rest or an object in the state of motion always remains in their native state unless and until any force is applied to the object.”
3. Give some examples of Actiona and Reaction Pairs?
Some examples of action reaction pairs are,
- Bullet Fired form a Gun and Gun
- Gases coming out from a Rocket and Rocket, etc.
4. What is a Force?
To “Push or Pull” anything is called the force. Force applied to any body can do a lot of things it can change its shape, it can change its speed, it can change its direction, etc.
5. What is Formula for Force?
The formula used to calculate the force acting on any object is,
F = m.a
where,
- F is Force acting on the Body
- m is Mass of the Body
- a is Acceleration of the Body
6. What is SI unit of Force?
The SI unit of Force is Newton. Other units of force are, kg/m2, kgf, etc.
Share your thoughts in the comments
Please Login to comment...