Generate all cyclic permutations of a number

Given a number N, our task is to generate all the possible cyclic permutations of the number. 
A cyclic permutation shifts all the elements of a set by a fixed offset. For a set with elements , , 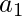…, , 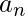a cyclic permutation of one place to the left would y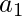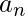ield , …, , , and a cyclic permutation of one place to the right would yield , , , ….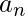
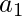Examples:

Input :  123
Output : 123
         312
         231

Input :  5674
Output : 5674
         4567
         7456
         6745

The idea is to generate next permutation of a number using below formula. 

    rem = num % 10;
    div = num / 10;
    num = (pow(10, n - 1)) * rem + div;

While repeating above steps, if we come back to original number, we stop and return. 

C++

// Program to generate all cyclic permutations
// of number
#include <bits/stdc++.h>
using namespace std;

// Function to count the total number of digits
// in a number.
int countdigits(int N)
{
    int count = 0;
    while (N) {
        count++;
        N = N / 10;
    }
    return count;
}

// Function to generate all cyclic permutations
// of a number
void cyclic(int N)
{
    int num = N;
    int n = countdigits(N);

    while (1) {
        cout << num << endl;

        // Following three lines generates a
        // circular permutation of a number.
        int rem = num % 10;
        int div = num / 10;
        num = (pow(10, n - 1)) * rem + div;

        // If all the permutations are checked
        // and we obtain original number exit
        // from loop.
        if (num == N)
            break;
    }
}

// Driver Program
int main()
{
    int N = 5674;
    cyclic(N);
    return 0;
}

Java

// Java Program to generate all
// cyclic permutations of number
public class GFG
{

    // Function to count the total number
    // of digits in a number.
    static int countdigits(int N)
    {
        int count = 0;
        while (N>0) {
            count++;
            N = N / 10;
        }
        return count;
    }

    // Function to generate all cyclic
    // permutations of a number
    static void cyclic(int N)
    {
        int num = N;
        int n = countdigits(N);

        while (true) {
            System.out.println(num);

            // Following three lines generates a
            // circular permutation of a number.
            int rem = num % 10;
            int dev = num / 10;
            num = (int)((Math.pow(10, n - 1)) *
                                rem + dev);

            // If all the permutations are
            // checked and we obtain original
            // number exit from loop.
            if (num == N)
                break;
        }
    }

    // Driver Program
    public static void main (String[] args) {
    int N = 5674;
    cyclic(N);
    }
}

/* This code is contributed by Mr. Somesh Awasthi */

Python3

# Python3 Program to
# generate all cyclic
# permutations of number
import math

# Function to count the
# total number of digits
# in a number.
def countdigits(N):
    count = 0;
    while (N):
        count = count + 1;
        N = int(math.floor(N / 10));
    return count;

# Function to generate
# all cyclic permutations
# of a number
def cyclic(N):
    num = N;
    n = countdigits(N);
    while (1):
        print(int(num));

        # Following three lines
        # generates a circular
        # permutation of a number.
        rem = num % 10;
        div = math.floor(num / 10);
        num = ((math.pow(10, n - 1)) *
                           rem + div);

        # If all the permutations
        # are checked and we obtain
        # original number exit from loop.
        if (num == N):
            break;

# Driver Code
N = 5674;
cyclic(N);

# This code is contributed by mits

C#

// C# Program to generate all
// cyclic permutations of number
using System;

class GFG
{
    // Function to count the total number
    // of digits in a number.
    static int countdigits(int N)
    {
        int count = 0;
        while (N > 0) {
            count++;
            N = N / 10;
        }
        return count;
    }

    // Function to generate all cyclic
    // permutations of a number
    static void cyclic(int N)
    {
        int num = N;
        int n = countdigits(N);

        while (true) {
            Console.WriteLine(num);

            // Following three lines generates a
            // circular permutation of a number.
            int rem = num % 10;
            int dev = num / 10;
            num = (int)((Math.Pow(10, n - 1)) *
                                    rem + dev);

            // If all the permutations are
            // checked and we obtain original
            // number exit from loop.
            if (num == N)
                break;
        }
    }

    // Driver Program
    public static void Main ()
    {
      int N = 5674;
      cyclic(N);
    }
}

// This code is contributed by nitin mittal

PHP

<?php
// PHP Program to generate all
// cyclic permutations of number

// Function to count the total
// number of digits in a number.
function countdigits($N)
{
    $count = 0;
    while ($N)
    {
        $count++;
        $N = floor($N / 10);
    }
    return $count;
}

// Function to generate all
// cyclic permutations of a number
function cyclic($N)
{
    $num = $N;
    $n = countdigits($N);

    while (1)
    {
        echo ($num);
        echo "\n" ;

        // Following three lines generates a
        // circular permutation of a number.
        $rem = $num % 10;
        $div = floor($num / 10);
        $num = (pow(10, $n - 1)) * $rem + $div;

        // If all the permutations are checked
        // and we obtain original number exit
        // from loop.
        if ($num == $N)
            break;
    }
}

    // Driver Code
    $N = 5674;
    cyclic($N);

// This code is contributed by nitin mittal
?>

Javascript

<script>

// javascript Program to generate all
// cyclic permutations of number
// Function to count the total number
// of digits in a number.

function countdigits(N)
{
    var count = 0;
    while (N>0) {
        count++;
        N = parseInt(N / 10);
    }
    return count;
}

// Function to generate all cyclic
// permutations of a number
function cyclic(N)
{
    var num = N;
    var n = countdigits(N);

    while (true) {
        document.write(num+"<br>");

        // Following three lines generates a
        // circular permutation of a number.
        var rem = num % 10;
        var dev = parseInt(num / 10);
        num = parseInt(((Math.pow(10, n - 1)) *
                            rem + dev));

        // If all the permutations are
        // checked and we obtain original
        // number exit from loop.
        if (num == N)
            break;
    }
}

// Driver Program
var N = 5674;
cyclic(N);

// This code is contributed by Amit Katiyar
</script>

Output

5674
4567
7456
6745

Time Complexity: O(N), where N is the number of digits
Auxiliary Space: O(1)  

This article is contributed by Vineet Joshi. If you like GeeksforGeeks and would like to contribute, you can also write an article using write.geeksforgeeks.org or mail your article to review-team@geeksforgeeks.org. See your article appearing on the GeeksforGeeks main page and help other Geeks.
Please write comments if you find anything incorrect, or you want to share more information about the topic discussed above.