Find the type of triangle from the given sides

Last Updated : 13 Sep, 2021

Given three integers A, B, and C which denote the sides of a triangle, the task is to check that the triangle is a right-angled, acute-angled, or obtuse-angled triangle.
Examples:

Input: A = 1, B = 4, C = 3
Output: Obtuse-angled Triangle
Explanation:
Triangle with the sides 1, 2 and 3 is an obtuse-angled triangle
Input: A = 2, B = 2, C = 2
Output: Acute-angled Triangle
Explanation:
Triangle with the sides 2, 2, and 2 is an acute-angled triangle

Approach: The idea is to use the facts from the cosine law to check the type of triangle using these formulae –
$c^2 &= a^2 + b^2 - 2*a*b*cos\gamma$
It generalizes the Pythagorean Theorem, which states that for a right-angled triangle square of the hypotenuse is equal to the sum of squares of the base and height of the triangle, which is 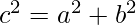$c^2 &= a^2 + b^2$
Similarly, It can be observed that
For acute-angled triangle
$c^2 \textless a^2 + b^2$
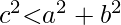
For Obtuse-angled triangle
$c^2 \textgreater a^2 + b^2$
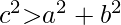
Below is the implementation of the above approach:

C++

// C++ implementation to find
// the type of triangle with
// the help of the sides
 
#include <bits/stdc++.h>
using namespace std;
 
// Function to find the type of
// triangle with the help of sides
void checkTypeOfTriangle(int a,
                int b, int c){
    int sqa = pow(a, 2);
    int sqb = pow(b, 2);
    int sqc = pow(c, 2);
     
    if (sqa == sqb + sqc ||
        sqb == sqc + sqa ||
        sqc == sqa + sqb){
        cout << "Right-angled Triangle";
    }
    else if(sqa > sqc + sqb ||
            sqb > sqa + sqc ||
            sqc > sqa + sqb){
        cout << "Obtuse-angled Triangle";
    }
    else{
        cout << "Acute-angled Triangle";
    }
}
 
// Driver Code
int main()
{
    int a, b, c;
    a = 2;
    b = 2;
    c = 2;
     
    // Function Call
    checkTypeOfTriangle(a, b, c);
    return 0;
}

Java

// Java implementation to find
// the type of triangle with
// the help of the sides
import java.util.*;
 
class GFG
{
 
// Function to find the type of
// triangle with the help of sides
static void checkTypeOfTriangle(int a,
                int b, int c){
    int sqa = (int)Math.pow(a, 2);
    int sqb = (int)Math.pow(b, 2);
    int sqc = (int)Math.pow(c, 2);
     
    if (sqa == sqa + sqb ||
        sqb == sqa + sqc ||
        sqc == sqa + sqb){
        System.out.print("Right-angled Triangle");
    }
    else if(sqa > sqc + sqb ||
            sqb > sqa + sqc ||
            sqc > sqa + sqb){
        System.out.print("Obtuse-angled Triangle");
    }
    else{
        System.out.print( "Acute-angled Triangle");
    }
}
 
// Driver Code
public static void main (String []args)
{
    int a, b, c;
    a = 2;
    b = 2;
    c = 2;
     
    // Function Call
    checkTypeOfTriangle(a, b, c);
}
}
 
// This code is contribute by chitranayal

Python3

# Python3 implementation to find
# the type of triangle with
# the help of the sides
 
# Function to find the type of
# triangle with the help of sides
def checkTypeOfTriangle(a,b,c):
    sqa = pow(a, 2)
    sqb = pow(b, 2)
    sqc = pow(c, 2)
 
    if (sqa == sqa + sqb or
        sqb == sqa + sqc or
        sqc == sqa + sqb):
        print("Right-angled Triangle")
 
    elif(sqa > sqc + sqb or
            sqb > sqa + sqc or
            sqc > sqa + sqb):
        print("Obtuse-angled Triangle")
 
    else:
        print("Acute-angled Triangle")
 
# Driver Code
if __name__ == '__main__':
    a = 2
    b = 2
    c = 2
 
    # Function Call
    checkTypeOfTriangle(a, b, c)
 
# This code is contributed by mohit kumar 29

C#

// C# implementation to find
// the type of triangle with
// the help of the sides
using System;
 
class GFG
{
  
// Function to find the type of
// triangle with the help of sides
static void checkTypeOfTriangle(int a,
                int b, int c){
    int sqa = (int)Math.Pow(a, 2);
    int sqb = (int)Math.Pow(b, 2);
    int sqc = (int)Math.Pow(c, 2);
      
    if (sqa == sqa + sqb ||
        sqb == sqa + sqc ||
        sqc == sqa + sqb){
        Console.Write("Right-angled Triangle");
    }
    else if(sqa > sqc + sqb ||
            sqb > sqa + sqc ||
            sqc > sqa + sqb){
        Console.Write("Obtuse-angled Triangle");
    }
    else{
        Console.Write( "Acute-angled Triangle");
    }
}
  
// Driver Code
public static void Main(String []args)
{
    int a, b, c;
    a = 2;
    b = 2;
    c = 2;
      
    // Function Call
    checkTypeOfTriangle(a, b, c);
}
}
 
// This code is contributed by 29AjayKumar

Javascript

<script>
 
// JavaScript implementation to find
// the type of triangle with
// the help of the sides
 
// Function to find the type of
// triangle with the help of sides
function checkTypeOfTriangle(a,b,c)
{
    let sqa = Math.floor(Math.pow(a, 2));
       let sqb = Math.floor(Math.pow(b, 2));
    let sqc = Math.floor(Math.pow(c, 2));
       
    if (sqa == sqa + sqb ||
        sqb == sqa + sqc ||
        sqc == sqa + sqb){
        document.write("Right-angled Triangle");
    }
    else if(sqa > sqc + sqb ||
            sqb > sqa + sqc ||
            sqc > sqa + sqb){
        document.write("Obtuse-angled Triangle");
    }
    else{
        document.write( "Acute-angled Triangle");
    }
}
 
// Driver Code
let a, b, c;
a = 2;
b = 2;
c = 2;
 
// Function Call
checkTypeOfTriangle(a, b, c);
 
// This code is contributed by rag2127
 
</script>

Output:

Acute-angled Triangle

My Personal Notes

Related Articles

Table of Contents

Find the type of triangle from the given sides

C++

Java

Python3

C#

Javascript

Start Your Coding Journey Now!