Hyperbola | Equation, Definition & Properties

A Hyperbola is a smooth curve in a plane with two branches that mirror each other, resembling two infinite bows. It is a conic section formed by intersecting a right circular cone with a plane at an angle such that both halves of the cone are intersected.

Let’s learn about Hyperbola in detail, including its Equation, Formulas, Properties, Graphs, and Derivation.

Hyperbola

What is Hyperbola?

A hyperbola is the locus of points whose difference in the distances from two foci is a fixed value. This difference is obtained by subtracting the distance of the nearer focus from the distance of the farther focus.

If P (x, y) is a point on the hyperbola and F, F’ are two foci, then the locus of the hyperbola is 

PF – PF’ = 2a

Hyperbola Definition

A hyperbola is the locus of all those points in a plane such that the difference in their distances from two fixed points in the plane is a constant

Hyperbola Equation

The equation of a hyperbola in its standard form depends on its orientation and whether it’s centered at the origin or another point. Here are the two primary forms for hyperbolas centered at the origin, one opening horizontally and the other opening vertically:

x²/a² – y²/b² = 1

This equation represents a hyperbola that opens to the left and right. The points (±a,0) are the vertices of the hyperbola, located on the x-axis.

Parts of Hyperbola

A hyperbola is a conic section that is developed when a plane cuts a double right circular cone at an angle such that both halves of the cone are joined. It can be described using concepts like foci, directrix, latus rectum, and eccentricity.

Parts of Hyperbola	Description
Foci	Two foci with coordinates F(c, 0) and F'(-c, 0)
Centre	Midpoint of the line joining the two foci, denoted as O
Major Axis	Length of the major axis is 2a units
Minor Axis	Length of the minor axis is 2b units
Vertices	Intersection points with the axis, (a, 0) and (-a, 0)
Transverse Axis	Line that passes through the two foci and the centre of the hyperbola
Conjugate Axis	Line that passes through the centre and is perpendicular to the transverse axis
Asymptotes	Equations of asymptotes are y = (b/a)x and y = -(b/a)x, lines that approach the hyperbola but never touch it
Directrix	Fixed straight line perpendicular to the axis of a hyperbola

Hyperbola Eccentricity

The eccentricity of a hyperbola is the ratio of the distance of a point from the focus to its perpendicular distance from the directrix. It is denoted by the letter ‘e‘.

• The eccentricity of a hyperbola is always greater than 1, i.e., e >1.
• We can easily find the eccentricity of the hyperbola by the formula :

e = √[1 + (b²/a²)]

where,

• a is Length of Semi-major axis
• b is Length of Semi-minor axis

Read More: Eccentricity

Standard Equation of Hyperbola

The standard equations of a hyperbola are:

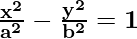
OR

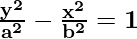
A hyperbola has two standard equations. These equations of a hyperbola are based on its transverse axis and conjugate axis. 

• Standard equation of the hyperbola is [(x²/a²) – (y²/b²)] = 1, where the X-axis is the transverse axis and the Y-axis is the conjugate axis. 
• Furthermore, another standard equation of the hyperbola is [(y²/a²)- (x²/b²)] = 1, where the Y-axis is the transverse axis and the X-axis is the conjugate axis.
• Standard equation of the hyperbola with centre (h, k) and the X-axis as the transverse axis and the Y-axis as the conjugate axis is,

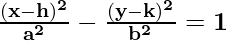
• Furthermore, another standard equation of the hyperbola with centre (h, k) and the Y-axis as the transverse axis and the X-axis as the conjugate axis is

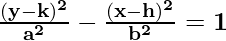
Latus Rectum of Hyperbola

Latus rectum of a hyperbola is a line passing through any of the foci of a hyperbola and perpendicular to the transverse axis of the hyperbola. The endpoints of a latus rectum lie on the hyperbola, and its length is 2b²/a.

Derivation of Hyperbola Equation

Let us consider a point P on the hyperbola whose coordinates are (x, y). From the definition of the hyperbola, we know that the difference between the distance of point P from the two foci F and F’ is 2a, i.e., PF’-PF = 2a.

Let the coordinates of the foci be F (c, o) and F ‘(-c, 0).

Now, by using the coordinate distance formula, we can find the distance of point P (x, y) to the foci F (c, 0) and F ‘(-c, 0).

√[(x + c)² + (y – 0)²] – √[(x – c)² + (y – 0)²] = 2a

√[(x + c)² + y²] = 2a + √[(x – c)² + y²] 

Now, by squaring both sides, we get

(x + c)² + y² = 4a² + (x – c)² + y² + 4a√[(x – c)² + y²] 

4cx – 4a² = 4a√[(x – c)² + y²] 

cx – a² = a√[(x – c)² + y²] 

Now, by squaring on both sides and simplifying, we get

[(x²/a²) – (y²/(c² – a²))] = 1

We have, c² = a² + b², so by substituting this in the above equation, we get

x²/a² – y²/b² = 1

Hence, the standard equation of the hyperbola is derived.

Similarly, we can derive the standard equations of the other hyperbola, i.e., [y²/a² – x²/b²] = 1

Hyperbola Formula

Following hyperbola formulas are widely used in finding the various parameters of the hyperbola which include, the equation of hyperbola, the major and minor axis, eccentricity, asymptotes, vertex, foci, and semi-latus rectum.

Property	Formula
Equation of Hyperbola	(x-xo)2 / a2 – (y-yo)2 / b2 = 1
Major Axis	y = y0​; Length = 2a
Minor Axis	x = x0​; Length = 2b
Eccentricity	​ e = √(1 + b2/a2)
Asymptotes	y= y0 ​±(b/a)(x − x0​)
Vertex	(a, y0) and (−a, y0)
Focus (Foci)	(a, √(a2 + b2)y0) and  (−a, √(a2 + b2)y0)
Semi-Latus Rectum (p)	p = b2/a
Equation of Tangent	(xx1)/a2 – (yy1)/b2 = 1,
Equation of Normal	y−y1​=(−y1​a2)​(x−x1​) / (x1​b2), at point (x1,y1) where, x1​ ≠ 0

where,

• ( x₀ ​, y₀​) is the Centre Point
• a is the Semi-major Axis
• b is the Semi-minor Axis.

Graph of Hyperbola

Hyperbola is a curve which has two unbounded curves which are mirror images of each other. The graph of the hyperbola shows that curve in the 2-D plane. We can observe the different parts of a hyperbola in the hyperbola graphs for standard equations given below:

Equation of the Hyperbola	Graph of Hyperbola	Parameters of Hyperbola
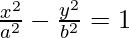
		Coordinates of the centre: (0, 0) Coordinates of the vertex: (a, 0) and (-a, 0) Coordinates of foci: (c, 0) and (-c, 0) The length of the transverse axis = 2a The length of the conjugate axis = 2b The length of the latus rectum = 2b2/a Equations of asymptotes:  y = (b/a) x and y = -(b/a) x Eccentricity (e) = √[1 + (b2/a2)]
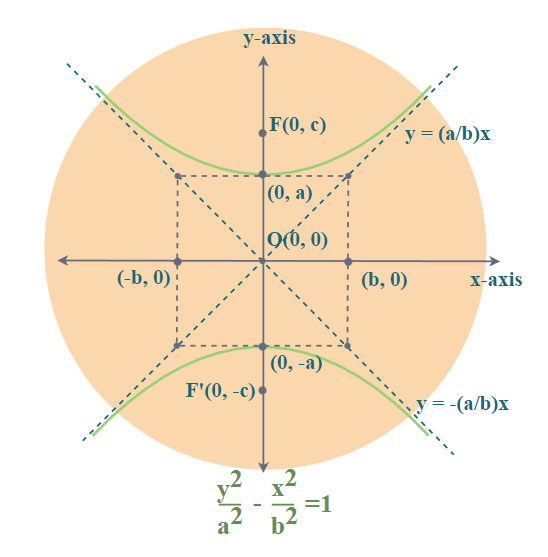		Coordinates of the centre: (0, 0) Coordinates of the vertex: (0, a) and (0, -a) Coordinates of foci: (0, c) and (0, -c) The length of the transverse axis = 2b The length of the conjugate axis = 2a The length of the latus rectum = 2b2/a Equations of asymptotes: y = (a/b) x and y = -(a/b) x Eccentricity (e) = √[1 + (b2/a2)]

Conjugate Hyperbola

Conjugate Hyperbola are 2 hyperbolas such that the transverse and conjugate axes of one hyperbola are the conjugate and transverse axis of the other hyperbola respectively.

Conjugate hyperbola of (x² / a²) – (y² /b²) = 1 is,

(x² / a²) – (y² / b²) = 1

where,

• a is Semi-major axis
• b is Semi-minor axis
• e is Eccentricity of Parabola
• a² = b² (e² − 1)

Properties of Hyperbola

• If the eccentricities of the hyperbola and its conjugate are e₁, and e₂ then,

(1 / e₁²) + (1 / e₂²) = 1

• Foci of a hyperbola and its conjugate are concyclic and form the vertices of a square.
• Hyperbolas are equal if they have the same latus rectum.

Auxiliary Circles of Hyperbola

Auxiliary Circle is a circle which is drawn with centre C and diameter as a transverse axis of the hyperbola. The auxiliary circle of the hyperbola equation is,

x² + y² = a²

Rectangular Hyperbola

A hyperbola with a transverse axis of 2a units and a conjugate axis of 2b units of equal length is called the Rectangular Hyperbola. i.e. in rectangular hyperbola,

2a = 2b

⇒ a = b

Equation of a Rectangular Hyperbola is given as follows:

x² – y² = a²

Note: Eccentricity of Rectangular hyperbola is √2.

Parametric Representation of Hyperbola

Parametric Representation of auxiliary circles of the hyperbola is:

x = a sec θ, y = b tan θ

People Also Read

• Conic Section
• Parabola
• Circle
• Ellipse

Hyperbola Class 11

In Class 11 mathematics, the study of hyperbolas forms a part of the conic sections in analytic geometry. Understanding hyperbolas at this level involves exploring their definition, standard equations, properties, and various elements associated with them.

Class 11 curriculum typically includes deriving these equations and properties, sketching hyperbolas based on given equations, and solving problems related to the hyperbola’s elements and positions. Mastery of these concepts provides a strong foundation in analytic geometry, preparing students for further studies in mathematics and related fields.

Summary – Hyperbola

A hyperbola is a type of conic section that forms when a plane intersects a cone at an angle such that two separate curves are produced. Characterized by its mirror symmetry, a hyperbola consists of two disconnected branches, each curving away from the other. It can be defined mathematically in a coordinate plane using a standard equation, which varies based on its orientation—either horizontal or vertical—and whether its center is at the origin or another point. The standard forms are x²/a² – y²/b² = 1 for a hyperbola opening horizontally and y²/a² – x²/b² = 1 for one opening vertically, with variations to accommodate a center moved to (h,k). Key features of hyperbolas include vertices, the closest points on each branch to the center; foci, points from which distances to any point on the hyperbola have a constant difference; and asymptotes, lines that the branches approach but never touch. The properties of hyperbolas make them significant in various fields, including astronomy, physics, and engineering, for modeling and analyzing hyperbolic trajectories and behaviors.

Solved Examples on Hyperbola

Question 1: Determine the eccentricity of the hyperbola x²/64 – y²/36 = 1.

Solution:

Equation of hyperbola is x²/64 – y²/36 = 0

By comparing given equation with standard equation of the hyperbola x²/a² – y²/b² = 1, we get

a² = 64, b² = 36

⇒ a = 8, b = 6

We have,

Eccentricity of a hyperbola (e) = √(1 + b²/a²)

⇒ e = √(1 + 6²/8²)

⇒ e = √(1 + 36/64)

⇒ e = √(64 + 36)/64) = √(100/64)

⇒ e = 10/8 = 1.25‬

Hence, Eccentricity of given hyperbola is 1.25‬.

Question 2: If the equation of the hyperbola is [(x-4)²/25] – [(y-3)²/9] = 1, find the lengths of the major axis, minor axis, and latus rectum. 

Solution:

Equation of hyperbola is [(x-4)²/25] – [(y-3)²/9] = 1

By comparing given equation with the standard equation of the hyperbola, (x – h)²/a² – (y – k)²/b² = 1

Here, x = 4 is the major axis and y = 3 is the minor axis.

a² = 25 a = 5

b² = 9 b = 3

Length of major axis = 2a = 2 × (5) = 10 units

Length of minor axis = 2b = 2 × (3) = 6 units

Length of latus rectum = 2b²/a = 2(3)²/5 = 18/5 = 3.6 units

Question 3: Find the vertex, asymptote, major axis, minor axis, and directrix if the hyperbola equation is [(x-6)²/7²]-[(y-2)²/4²] = 1.

Solution:

Equation of hyperbola is [(x-6)²/7²] – [(y-2)²/4²] = 1

By comparing given equation with standard equation of hyperbola, (x – h)²/a² – (y – k)²/b² = 1

h = 6, k = 2, a = 7, b = 4

Vertex of a Hyperbola: (h + a, k) and (h – a, k) = (13, 2) and (-1, 2)

Major axis of Hyperbola is x = h x = 6

Minor axis of Hyperbola is y = k y = 2

Equations of asymptotes of hyperbola are 

y = k − (b / a)x + (b / a)h and y = k+ (b / a)x – (b / a)h

⇒ y = 2 – (4/7)x + (4/7)6 and y = 2 + (4/7)x – (4/7)6

⇒ y = 2 – 0.57x + 3.43 and y = 2 + 0.57x – 3.43

⇒ y = 5.43 – 0.57x and y = -1.43 + 0.57x

Equation of the directrix of a hyperbola is x = ± a²/√(a² + b²)

⇒ x = ± 7²/√(7² + 4²) 

⇒ x= ± 49/√65 

⇒ x = ± 6.077

Question 4: Find the eccentricity of the hyperbola whose latus rectum is half of its conjugate axis.

Solution:

Length of latus rectum is half of its conjugate axis

Let, Equation of hyperbola be [(x² / a²) – (y² / b²)] = 1

Conjugate axis = 2b

Length of Latus rectum = (2b² / a)

From given data, (2b² / a) = (1/2) × 2b

2b = a

We have,

Eccentricity of Hyperbola (e) = √[1 + (b²/a²)]

Now, substitute a = 2b in the formula of eccentricity

⇒ e = √[1 + (b²/(2b)²]

⇒ e = √[1 + (b²/4b²)] = √(5/4) 

⇒ e = √5/2

Hence, required eccentricity is √5/2.

Practice Problems on Hyperbola

P1. Find the standard form equation of the hyperbola with vertices at (-3, 2) and (1, 2), and a focal length of 5.

P2. Determine the center, vertices, and foci of the hyperbola with the equation 9x² – 4y² = 36.

P3. Given the hyperbola with the equation (x – 2)²/16 – (y + 1)²/9 = 1, find the coordinates of its center, vertices, and foci.

P4. Write the equation of the hyperbola with a horizontal major axis, center at (0, 0), a vertex at (5, 0), and a focus at (3, 0).

FAQs on Hyperbola

What is Hyperbola in Maths?

The locus of a point in a plane such that the ratio of its distance from a fixed point to that from a fixed line is a constant greater than 1 is called Hyperbola.

What is Standard Equation of Hyperbola?

Standard Equation of Hyperbola is 

(x²/a²) – (y²/b²) = 1

What is Eccentricity of Hyperbola?

Eccentricity of a hyperbola is the ratio of the distance of a point from the focus to its perpendicular distance from the directrix. For Hyperbola the eccentricity is always greater than 1.

What is Formula of Eccentricity of Hyperbola?

Formula for Eccentricity of Hyperbola is e = √(1 + (b²/a²))

What are Foci of Hyperbola?

A hyperbola has two foci. For the hyperbola (x²/a²) – (y²/b²) = 1, the foci are given by (ae, 0) and (-ae, 0)

What is Transverse Axis of Hyperbola?

For hyperbola (x²/a²) – (y²/b²) = 1, transverse axis is along x axis. Its length is given by 2a. Line passing through centre and foci of hyperbola are called transverse axis of a hyperbola.

What are Asymptotes of Hyperbola?

Lines parallel to hyperbola which meet hyperbola at infinity are called the asymptotes of hyperbola.

How many Asymptotes does Hyperbola have?

A hyperbola has 2 asymptotes. Asymptotes is a line tangent to the hyperbola which meets the hyperbola at infinity.

What is Hyperbola used for?

Hyperbolas find applications in various fields such as astronomy, physics, engineering, and economics. They are used in satellite trajectories, radio transmission patterns, artillery targeting, financial modeling, and celestial mechanics, among other areas.

What is Difference between Parabola and Hyperbola in Standard Form?

In standard form, the equation of a parabola involves terms raised to the power of 1 and 2, while the equation of a hyperbola involves terms raised to the power of 2 and -2. Also, the parabola is characterized by a single focus point, while the hyperbola has two.

What is Basic Equation of Hyperbola Graph?

Basic equation of a hyperbola graph is:

(x – h)² / a² – (y – k)² / b² = 1

Or

(y – k)² / b² – (x -h)² / a² = 1

What are Types of Hyperbola?

Hyperbolas can be classified into three types based on their orientation: horizontal, vertical, and oblique hyperbolas.

How do you identify a Hyperbola equation?

A hyperbola equation typically involves terms with both x and y variables, with a difference between the squares of x and y coefficients, and the coefficients of these terms are positive and negative, respectively.

What is formula of B in Hyperbola?

In the standard form of a hyperbola equation, B represents the length of the conjugate axis, and its formula is B = 2b, where b is the distance from the center to the vertices along the conjugate axis.

How to draw a Hyperbola?

To draw a hyperbola, you typically start by plotting the center point, then mark the vertices, foci, asymptotes, and other key points based on the given equation or properties. Finally, sketch the hyperbola’s curves using these points as guides.