Corollaries of Binomial Theorem
Last Updated :
28 Jun, 2022
The expression 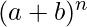 denotes
denotes 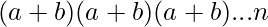 times. This can be evaluated as the sum of the terms involving
times. This can be evaluated as the sum of the terms involving  for k = 0 to n, where the first term can be chosen from n places, second term from (n-1) places,
for k = 0 to n, where the first term can be chosen from n places, second term from (n-1) places, 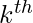 term from (n-(k-1)) places and so on. This is expressed as
term from (n-(k-1)) places and so on. This is expressed as 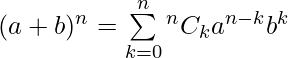 . The binomial expansion using Combinatorial symbols is
. The binomial expansion using Combinatorial symbols is
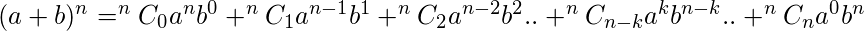
- The degree of each term
 [Tex]b^{n-k} [/Tex]in the above binomial expansion is of the order n.
[Tex]b^{n-k} [/Tex]in the above binomial expansion is of the order n. - The number of terms in the expansion is n+1.
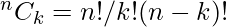 Similarly
Similarly 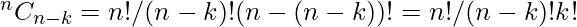 Hence it can be concluded that
Hence it can be concluded that 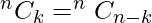 .
.
Substituting a = 1 and b = x in the binomial expansion, for any positive integer n we obtain 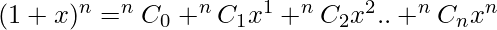 . Corollary 1:
. Corollary 1:
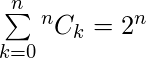
for any non-negative integer n. Replacing x with 1 in the above binomial expansion, We obtain 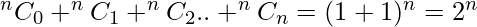 . Corollary 2:
. Corollary 2:
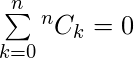
for any positive integer n. Replacing x with -1 in the above binomial expansion, We obtain 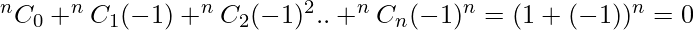 . Corollary 3: Replacing x with 2 in the above binomial expansion, we obtain
. Corollary 3: Replacing x with 2 in the above binomial expansion, we obtain  In general, it can be said that
In general, it can be said that
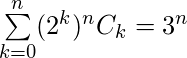
Additionally, one can combine corollary 1 and corollary 2 to get another result, 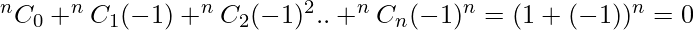 [Tex]^nC_0 + ^nC_2 + .. = ^nC_1 + ^nC_3 + … [/Tex]Sum of coefficients of even terms = Sum of coefficients of odd terms. Since
[Tex]^nC_0 + ^nC_2 + .. = ^nC_1 + ^nC_3 + … [/Tex]Sum of coefficients of even terms = Sum of coefficients of odd terms. Since  , 2(
, 2(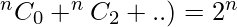 [Tex]^nC_0 + ^nC_2 + .. = 2^{n-1} [/Tex]
[Tex]^nC_0 + ^nC_2 + .. = 2^{n-1} [/Tex]
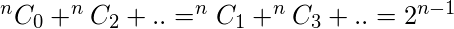
Counting The coefficients of the terms in the expansion 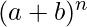 correspond to the terms of the pascal’s triangle in row n.
correspond to the terms of the pascal’s triangle in row n.
Like Article
Suggest improvement
Share your thoughts in the comments
Please Login to comment...