During the game, you may have witnessed two billiard balls collide with each other. Collision is the violent coming together of two distinct bodies. What happens when two objects collide? Can we identify the colliding bodies’ velocity or trajectory? Let us investigate!
A collision occurs when two things come into touch with one other for a brief period of time. In other terms, a collision is a short-term reciprocal contact between two masses in which the momentum and energy of the colliding masses change. You may have seen the effect of a striker on coins when they collided while playing caroms.
Coefficient of Restitution
The ratio of final velocity to the initial velocity of the interacting particles after the occurrence of a collision between them is termed the coefficient of restitution. The coefficient of restitution is denoted by ‘e’ with a value ranging from 0 to 1. Since the coefficient of restitution is a constant commodity, it doesn’t have any dimensions. It provides more information about the elasticity of the collision. The perfectly elastic collision where there is no loss of overall kinetic energy of the system. It is basically an integer value, which depicts the measure of the nature of colliding materials.
The maximum value of the coefficient of restitution is e =1.
The formula for the coefficient of restitution is,
e = Relative Speed before the Collision / Relative Speed after the Collision
Range of Values for e
Since the coefficient of restitution lies between the interval 0 to 1, it can contain the following range of values :
- For e = 0, refers to a perfectly inelastic collision. Maximum kinetic energy is lost during the occurrence of such kind of collision.
- If 0 < e < 1, refers to a real-world inelastic collision, that is, in these types of collision, some kinetic energy is lost.
- If e = 1, refers to a perfectly elastic collision in which no kinetic energy is dissipated. The objects rebound with the same speed with which they approach each other.
Types Of Collision
According to the law of conservation of momentum, there is no loss of energy during the collision of objects with individual masses. However, there may be certain collisions without following the conservation of kinetic energy. Based on the energy conservation being followed during collisions, the following categories can be devised:
(1) Elastic Collision
Elastic collisions conserve the total momentum and total energy. The total kinetic energy may or may not be conserved. Since the forces involved during the collision are conserved in nature, the form of mechanical energy is not converted to any other form of energy.
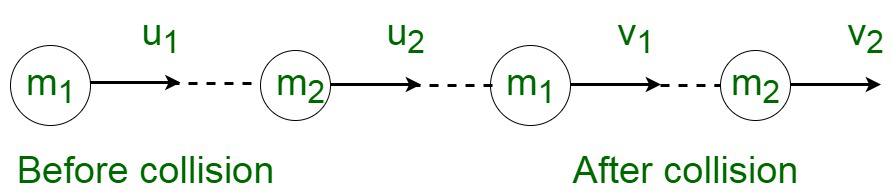
Let us assume two objects with masses, m1 and m2 traveling with speeds u1 and u2 respectively. Let the final velocities after the collision of these two objects, be v1 and v2.
According to the law of conservation of momentum, we have:
m1u1 + m2u2 = m1v1 + m2v2
According to the conservation of kinetic energy:
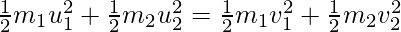
Examples of Elastic Collision are:
- The collision between two billiards balls.
- Throwing and catching back a ball.
Applications of Elastic Collision are:
The collision time is inversely proportional to the force acting between the interacting bodies. In order to maximize the force acting between two bodies, the collision time must be reduced. The same is possible for the other case. This implies, that in order to minimize the force between two bodies, the collision time must be increased.
The concepts are visible in the concept of the introduction of airbags in vehicles. The idea is to provide a larger collapse time in order to minimize the effect of force on objects during a collision. Airbags in cars achieve this by increasing the time frame required to halt the momentum of both the passenger and the driver of the automobile.
(2) Inelastic Collision
Inelastic collisions conserve the total momentum and total energy. The total kinetic energy may or may not be conserved. The energy is transformed into other forms of energy, that is, heat and light. The interacting objects may either stick to each other or begin moving aligned in the same direction.
By the law of conservation of momentum, we have,
Since the objects move in the same direction, both the objects move with the same speed, v,
m1u1 + m2u2 = (m1 + m2)v

- The kinetic energy of the masses before the collision is : K.E1 =
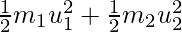
- The kinetic energy after the collision is: K.E2 = 1/2 (m1+ m2) v2
By the law of conservation of energy,
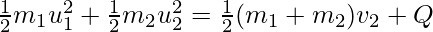
where ‘Q’ refers to the change in energy resulting in the production of heat or sound.
Examples of Inelastic Collision are:
- Accident of two vehicles.
- A car hitting a tree.
- The ball dropped from a certain height unable to rise to its original height.
One Dimensional Collision
The collision in which both the initial and final velocities of the involved masses lie in one line. All the variables involved in the motion occur in a single dimension.
(1) Elastic One Dimensional Collision
In the elastic collisions the momentum and internal kinetic energy are conserved. Since elastic collisions can be simulated with only microscopic particles like, electrons or neutrons. Consider two protons with the masses m1 and m2
By the law of conservation of momentum, we have,
m1u1 + m2u2 = m1v1 + m2v2
Because of kinetic energy conservation,
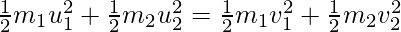
This implies,
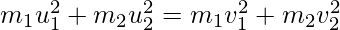 (Factoring out 1/2)
(Factoring out 1/2)
Rearranging we get: 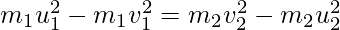
Therefore,
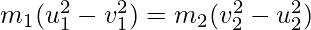
On expansion, it becomes,
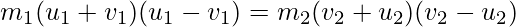
By the conservation of momentum :

Grouping it using the same masses :

Therefore,

On dividing the two equations:
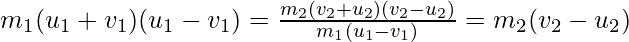
We imply,

Now,

Substituting the value of 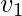
![Rendered by QuickLaTeX.com v_2 = \frac{[2 m_1 u_1 + u_2 (m_2-m_1)] }{ (m_1 + m_2 )}](https://www.geeksforgeeks.org/wp-content/ql-cache/quicklatex.com-71df423c39e45bb7c42f4a07ed2f54ff_l3.png) …….(1)
…….(1)
Now using the value of v2 in equation 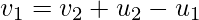
![Rendered by QuickLaTeX.com v_1 = \frac{[2 m_1 u_1 + u_2 (m_2-m_1)] }{ (m_1 + m_2)} + u_2- u_1\\ v_1 = \frac{[2 m_1 u_1 + u_2 (m_2-m_1) + u_2 (m_1 + m_2 ) - u_1(m_1 + m_2 )] }{ (m_1 + m_2 ) }](https://www.geeksforgeeks.org/wp-content/ql-cache/quicklatex.com-875be5aca7cb7ada848d6602b95121e7_l3.png) …….(2)
…….(2)
On reduction, we obtain,
![Rendered by QuickLaTeX.com v_1 = \frac{[2m_2 u_2 + u_1 ( m_1 - m_2)] }{ (m_1 +m_2 )}](https://www.geeksforgeeks.org/wp-content/ql-cache/quicklatex.com-3f3e4317a4af6cbaa6ca3bec36fdc69c_l3.png)
Most of the time after collision, these masses exchange their velocities. When masses of both the bodies are equal ,
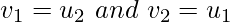
In case, the second mass object is at rest and the first mass object collides with it, after the occurrence of a collision, there is an interchange in the velocities, the first mass comes to rest, and the second mass moves with the speed equal to the first mass.
Therefore, 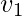 = 0 and
= 0 and 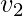 =
=  . In case if
. In case if  then,
then, 
This implies that the lighter body (the body with lesser mass) will tend to bombard back with its own velocity, whereas the heavier mass will remain static at its position.
Also, we have, in case,
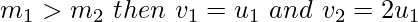
Some Special Cases are:
Case I:
In case of equal mass objects, that is, 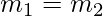
Using equation (1) and (2), we have,
 .
.
Therefore, we can conclude that if two bodies of equal masses suffer a head-on elastic collision, then there is a velocity exchange between the particles. Also, the exchange of momentum between two particles is maximum, in this scenario.
Case II:
Consider the target particle is at rest i,e 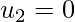
Using equation (1) and (2), we have,
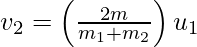 …..(3)
…..(3)
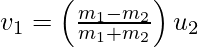 ……(4)
……(4)
The magnitude of KE transformed, is given by,
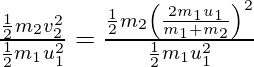
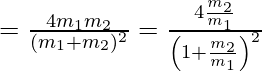 ……(5)
……(5)
when 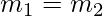 ,then in this condition
,then in this condition 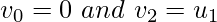
and part of the KE transferred would be
=1
Therefore, after the collision the respective states and velocities of the particles get interchanged, the first particle comes to rest and the second particle begins moving with the velocity of the first particle.
In this scenario, where 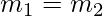 the transfer of energy is full, that is 100%.
the transfer of energy is full, that is 100%.
And, in case 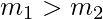 or
or  ,then the energy transformation is not equivalent to 100%.
,then the energy transformation is not equivalent to 100%.
Case III:
If 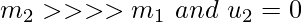
Using equation (3) and (4), we have,
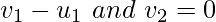 ……..(6)
……..(6)
Case IV:
If 
Using equation (3) and (4), we have,
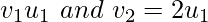 ………(7)
………(7)
Therefore,
We can conclude that when a particle with a larger mass undergoes a collision with a negligibly lighter mass particle at rest, then after the collision, the heavy particle maintains the same velocity and the light particle begins movement with a velocity double that of a heavier particle mass object.
(2) Inelastic One Dimensional Collision
The momentum of the involved particles remains conserved, which the kinetic energy is changed to different forms of energy.
By the law of conservation of momentum, we have,
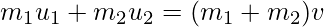
Since in inelastic one dimensional collision, both the objects tend to move with the same velocity v, we have,

The loss in kinetic energy can be equated to be :

Sample Problem
Problem 1. An object with mass m moving with velocity V m/s undergoes a collision with another body twice of its own mass originally at rest. Compute the ratio of K.E before and after the collision.
Solution:
Mass of first object = m
Mass of second object = 2m
The ratio of K.E before and after the collision will be 9:1.
Problem 2: Calculate the coefficient of restitution when a rubber ball falls from the ceiling situated at a height of 10 m. It rebounds twice and attains a final height of 2.5 m.
Solution:
Coefficient of restitution is given by,
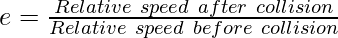
On calculating, we obtain,
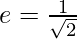
Problem 3: Consider two perfectly elastic particles A and B of equal masses, m with initial velocities of 15 m/sec and 10 m/sec respectively. What will be their final velocities?
Solution:
The velocities of the bodies will interchange after the collision, therefore, the final velocities of the masses will be,
Problem 4. Consider a body to be initially at the rest position. It accelerates constantly in one-dimensional motion. Explain the relation between the dissipated power and time.
Solution:
By the second law of motion,
v = u + at
v = 0 + at = at
Since, we know power is equivalent to ,
P = F × v
Therefore, we have,
P = (ma) × at = ma2t
Since m and n are constants,
Hence, Power is directly proportional to time.
P ∝ t.
Problem 5: Consider a body to be initially at the rest position. It accelerates constantly in one-dimensional motion with constant power. Explain the relationship between displacement and time.
Solution:
Since, we know,
p = force × velocity
[p] = [F] [v] = [MLT-2][LT-1]
[p] = [ML2T-3]
L2T-3 = constant
⇒ 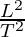 = constant
= constant
L2 ∝ T3
⇒ L ∝ 
Like Article
Suggest improvement
Share your thoughts in the comments
Please Login to comment...