Question 1. Recall that two circles are congruent if they have the same radii. Prove that equal chords of congruent circles subtend equal angles at their centres.
Solution:
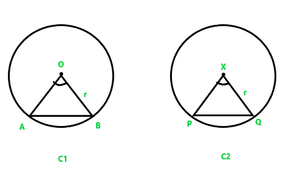
Given:
Two Congruent Circles C1 and C2
AB is the chord of C1
and PQ is the chord of C2
AB = PQ
To Prove: Angle subtended by the Chords AB and PQ are equal i.e. ∠AOB = ∠PXQ
Proof:
In â–³AOB & â–³PXQ
AO = PX (Radius of congruent circles are equal)
BO = QX (Radius of congruent circles are equal)
AB = PQ (Given)
â–³AOB â© â–³PXQ (SSS congruence rule)
Therefore, ∠AOB = ∠PXQ (CPCT)
Question 2. Prove that if chords of congruent circles subtend equal angles at their centres, then the chords are equal.
Solution:
Given:
Two Congruent circles C1 and C2
AB is the chord of C1 and PQ is chord of C2
& ∠AOB = ∠PXQ
To prove :
In â–³AOB and â–³PXQ ,
AO = PX (Radius of congruent circles are equal)
∠AOB = ∠PXQ (Given)
BO = QX (Radius of congruent circles are equal)
â–³AOB â© â–³PXQ (SAS congruence rule)
Therefore, AB = PQ (CPCT)
Whether you're preparing for your first job interview or aiming to upskill in this ever-evolving tech landscape,
GeeksforGeeks Courses are your key to success. We provide top-quality content at affordable prices, all geared towards accelerating your growth in a time-bound manner. Join the millions we've already empowered, and we're here to do the same for you. Don't miss out -
check it out now!
Last Updated :
20 Dec, 2021
Like Article
Save Article